Analytical Methods for Engineers: Trigonometric Methods TMA 2 Solution
VerifiedAdded on 2023/06/11
|9
|980
|371
Homework Assignment
AI Summary
This document presents a comprehensive solution to a Trigonometric Methods assignment, covering topics such as trigonometric functions, Pythagoras theorem, sinusoidal functions, and complex numbers. It includes detailed step-by-step calculations and explanations for each question, addressi...

Trigonometric Methods 1
Trigonometric Methods
Student’s Name
Course
Professor’s Name
University
City (State)
Date
Trigonometric Methods
Student’s Name
Course
Professor’s Name
University
City (State)
Date
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
Trigonometric Methods 2
Trigonometric Methods
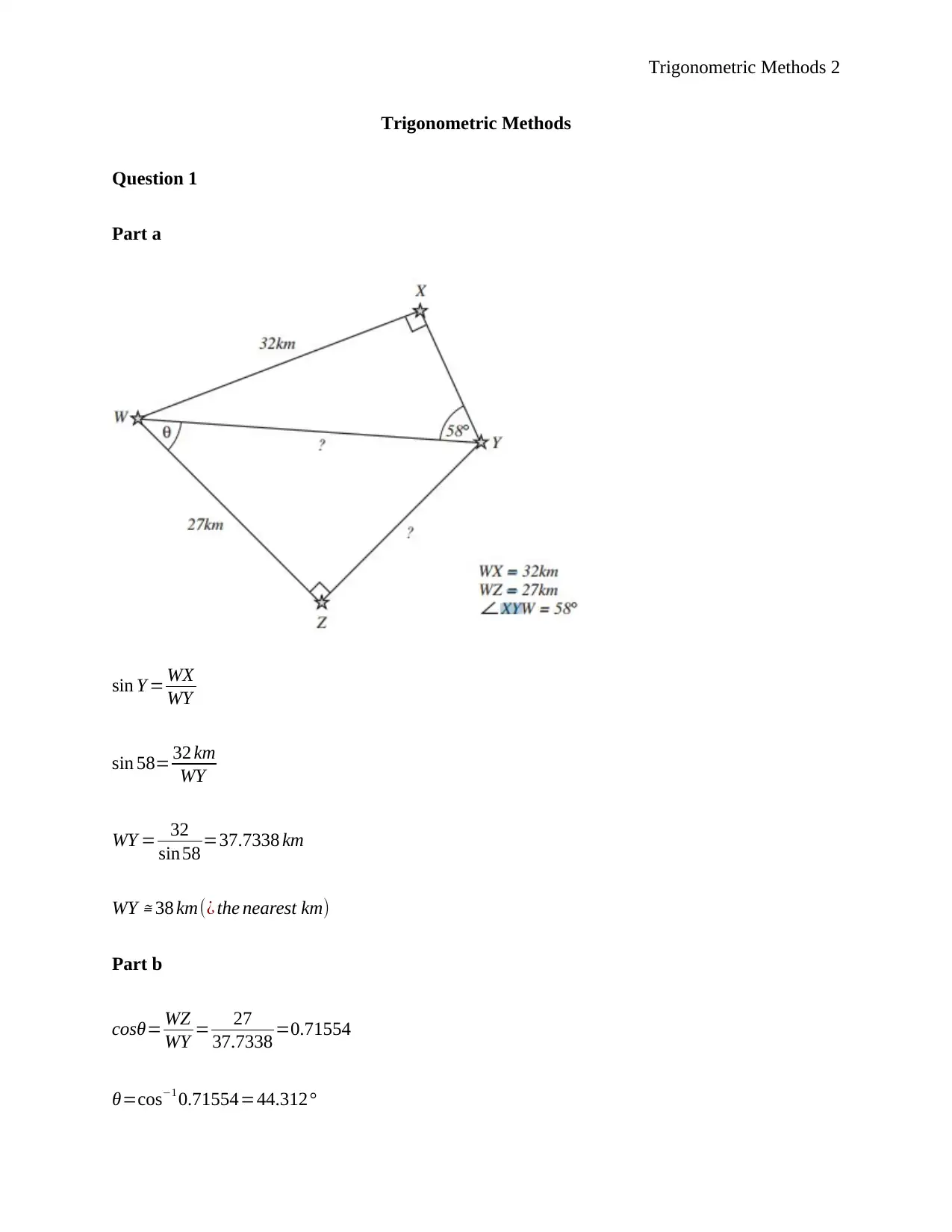
Question 1
Part a
sin Y = WX
WY
sin 58= 32 km
WY
WY = 32
sin58 =37.7338 km
WY ≅ 38 km(¿ the nearest km)
Part b
cosθ= WZ
WY = 27
37.7338 =0.71554
θ=cos−1 0.71554=44.312°
Trigonometric Methods
Question 1
Part a
sin Y = WX
WY
sin 58= 32 km
WY
WY = 32
sin58 =37.7338 km
WY ≅ 38 km(¿ the nearest km)
Part b
cosθ= WZ
WY = 27
37.7338 =0.71554
θ=cos−1 0.71554=44.312°

Trigonometric Methods 3
θ ≅ 44 ° (¿ the nearest degree)
Part c
Using Pythagoras theorem,
YZ= √ WY 2−WZ 2= √ 37.73382−272=26.3598 km
YZ ≅ 26 km(¿ the nearest km)
Question 2
i=15 sin(100 πt+ 0.6)
The equation is in the form y¿ asin(bt +c )
a) amplitude ¿ a=15 Amperes
b) period ¿ b=100 π
c) Frequency¿ 1
period = 1
100 π
d) Phase angle¿ c=0.6 radians= (0.6 × 180
π )°=34.3775 °
e) When t=2.5 s ,i=15 sin(100 π × 2.5+ 0.6)
¿ 15 sin ( 250 π + 0.6 )=15 × 0.5646=8.4696 Amperes
f) The current reaches the maximum level when sin ( 100 πt +0.6 )=1 so that,
sin−1 1=(100 πt +0.6)
100 πt =sin−1 1−0.6=1.5706−0.6=0.9708
t= 0.9708
100 π =0.00309 seconds
θ ≅ 44 ° (¿ the nearest degree)
Part c
Using Pythagoras theorem,
YZ= √ WY 2−WZ 2= √ 37.73382−272=26.3598 km
YZ ≅ 26 km(¿ the nearest km)
Question 2
i=15 sin(100 πt+ 0.6)
The equation is in the form y¿ asin(bt +c )
a) amplitude ¿ a=15 Amperes
b) period ¿ b=100 π
c) Frequency¿ 1
period = 1
100 π
d) Phase angle¿ c=0.6 radians= (0.6 × 180
π )°=34.3775 °
e) When t=2.5 s ,i=15 sin(100 π × 2.5+ 0.6)
¿ 15 sin ( 250 π + 0.6 )=15 × 0.5646=8.4696 Amperes
f) The current reaches the maximum level when sin ( 100 πt +0.6 )=1 so that,
sin−1 1=(100 πt +0.6)
100 πt =sin−1 1−0.6=1.5706−0.6=0.9708
t= 0.9708
100 π =0.00309 seconds
⊘ This is a preview!⊘
Do you want full access?
Subscribe today to unlock all pages.

Trusted by 1+ million students worldwide

Trigonometric Methods 4
t=0.00309 ×1000=3.09 milliseconds
Question 3
Part a (i)
cos (270 °−θ)
270 °−θ=180+( 90−θ)
cos ( 270 °−θ ) =cos (180+(90−θ))
But we know that, cos ( 180+∅ ) =−cos ∅ so that, cos ( 270 °−θ ) =−cos (90−θ)
But again, −cos ( 90−θ )=−( sinθ )
Hence, cos ( 270 °−θ )=−sinθ
Part a (ii)
sin (270 °−θ)
270 °−θ=180+( 90−θ)
sin ( 270 °−θ ) =sin (180+(90−θ))
But we know that, sin ( 180+∅ )=−sin∅ so that, sin ( 270 °−θ ) =−sin (90−θ)
But again, −sin ( 90−θ )=−(cosθ )
Therefore, sin ( 270 °−θ )=−cosθ
Part a (iii)
cos (270 °+θ)
t=0.00309 ×1000=3.09 milliseconds
Question 3
Part a (i)
cos (270 °−θ)
270 °−θ=180+( 90−θ)
cos ( 270 °−θ ) =cos (180+(90−θ))
But we know that, cos ( 180+∅ ) =−cos ∅ so that, cos ( 270 °−θ ) =−cos (90−θ)
But again, −cos ( 90−θ )=−( sinθ )
Hence, cos ( 270 °−θ )=−sinθ
Part a (ii)
sin (270 °−θ)
270 °−θ=180+( 90−θ)
sin ( 270 °−θ ) =sin (180+(90−θ))
But we know that, sin ( 180+∅ )=−sin∅ so that, sin ( 270 °−θ ) =−sin (90−θ)
But again, −sin ( 90−θ )=−(cosθ )
Therefore, sin ( 270 °−θ )=−cosθ
Part a (iii)
cos (270 °+θ)
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

Trigonometric Methods 5
270 ° +θ=180+(90+θ)
cos ( 270 ° +θ ) =cos (180+(90+θ))
But we know that, cos ( 180+∅ ) =−cos ∅ so that, cos ( 270 ° +θ ) =−cos (90+θ)
But again, −cos ( 90+θ ) =−(−sinθ)
Hence, cos ( 270 ° +θ ) =sinθ
Part 3b
V 1=3 sin (ωt )
V 2=2 cos (ωt )
V 3=V 1 +V 2=3 sin ( ωt ) +2 cos ( ωt)
but R sin ( ωt +α ) =(Rcosα )sin ( ωt ) +( Rsinα) cos( ωt)
Which implies that,
Rcosα =3 and Rsinα=2
Rsinα
Rcosα = 2
3 =tanα
α =tan−1
( 2
3 )=33.69°
Rcosα =3 , R= 3
cosα = 3
cos 33.69 ° =3.6056
So, V 3=R sin ( ωt +α ) =3.6056 sin (ωt +33.69 °)
270 ° +θ=180+(90+θ)
cos ( 270 ° +θ ) =cos (180+(90+θ))
But we know that, cos ( 180+∅ ) =−cos ∅ so that, cos ( 270 ° +θ ) =−cos (90+θ)
But again, −cos ( 90+θ ) =−(−sinθ)
Hence, cos ( 270 ° +θ ) =sinθ
Part 3b
V 1=3 sin (ωt )
V 2=2 cos (ωt )
V 3=V 1 +V 2=3 sin ( ωt ) +2 cos ( ωt)
but R sin ( ωt +α ) =(Rcosα )sin ( ωt ) +( Rsinα) cos( ωt)
Which implies that,
Rcosα =3 and Rsinα=2
Rsinα
Rcosα = 2
3 =tanα
α =tan−1
( 2
3 )=33.69°
Rcosα =3 , R= 3
cosα = 3
cos 33.69 ° =3.6056
So, V 3=R sin ( ωt +α ) =3.6056 sin (ωt +33.69 °)

Trigonometric Methods 6
Hence, we can see that frequency of V 1 =frequency of V 2=frequency of V 3= 1
ω
Question 4a
Z= Z1 Z2
Z1 +Z2
Z1 =4+ j 10
Z2 =12− j 3
Z= (4+ j 10)(12− j3)
( 4+ j10 )+(12− j 3)= 4 (12− j 3 )− j10(12− j 3)
4 +12+ j 10− j3 =78+ j108
16+ j7
Converting the numerator into polar form we get,
r = √ 782+¿1082
=133.2216 , θ=tan−1
( 108
78 ) =54.1623° ¿
Hence, 78+ j108=¿133.2216¿ 54.1623 °
Converting the denominator into polar form we get,
r = √162+¿72
=17.4642, θ=tan−1
( 7
16 )=23.6294 ° ¿
Hence, 16+ j7=¿17.4642¿ 23.6294 °
78+ j 108
16 + j 7 =¿133.2216¿ 54.1623 ° ÷17.4642¿ 23.6294 °
Z=¿7.6283¿ 30.5329 °
r =7.6283 ,θ=30.532 9
Hence, we can see that frequency of V 1 =frequency of V 2=frequency of V 3= 1
ω
Question 4a
Z= Z1 Z2
Z1 +Z2
Z1 =4+ j 10
Z2 =12− j 3
Z= (4+ j 10)(12− j3)
( 4+ j10 )+(12− j 3)= 4 (12− j 3 )− j10(12− j 3)
4 +12+ j 10− j3 =78+ j108
16+ j7
Converting the numerator into polar form we get,
r = √ 782+¿1082
=133.2216 , θ=tan−1
( 108
78 ) =54.1623° ¿
Hence, 78+ j108=¿133.2216¿ 54.1623 °
Converting the denominator into polar form we get,
r = √162+¿72
=17.4642, θ=tan−1
( 7
16 )=23.6294 ° ¿
Hence, 16+ j7=¿17.4642¿ 23.6294 °
78+ j 108
16 + j 7 =¿133.2216¿ 54.1623 ° ÷17.4642¿ 23.6294 °
Z=¿7.6283¿ 30.5329 °
r =7.6283 ,θ=30.532 9
⊘ This is a preview!⊘
Do you want full access?
Subscribe today to unlock all pages.

Trusted by 1+ million students worldwide

Trigonometric Methods 7
x=rcosθ=7.6283 cos 30.5329=6.5705
y=r sin θ=7.6283 sin 30.5329=3.8754
z=x +i y =6.5705+ j 3.8754
Z=¿7.6283¿ 30.5329 °
Question 4b
Z1 =2+ j 2
Z2 =1+ j 5
Z3 = j 6
Y = 1
Z1
+ 1
Z2
+ 1
Z3
= 1
2+ j 2 + 1
1+ j 5 + 1
j6
1
j 6 = 1 × j
j 6 × j =− j
6
Y = 6 ( 1+ j5 ) +6 ( 2+ j2 ) − j ( 2+ j 2 ) ( 1+ j5 )
6(2+ j2)(1+ j 5)
Y = 6+ j30+ 12+ j12+ j 8+12
6(−8+ j 12) = 30+ j 50
−48+ j 72
30+ j50= √ 302+502 ¿ tan−1 (50/30) ¿ √ 3400 ¿ 59.0362
−48+ j 72= √ 482 +722 ¿ tan−1 (72/−48) ¿ √7488 ¿−56.3099
Y = √3400 ¿ 59.0362 ÷ √7488 ¿−56.3099
Y =¿0.6738 ¿ ( 59.0362+56.3099 )−180
x=rcosθ=7.6283 cos 30.5329=6.5705
y=r sin θ=7.6283 sin 30.5329=3.8754
z=x +i y =6.5705+ j 3.8754
Z=¿7.6283¿ 30.5329 °
Question 4b
Z1 =2+ j 2
Z2 =1+ j 5
Z3 = j 6
Y = 1
Z1
+ 1
Z2
+ 1
Z3
= 1
2+ j 2 + 1
1+ j 5 + 1
j6
1
j 6 = 1 × j
j 6 × j =− j
6
Y = 6 ( 1+ j5 ) +6 ( 2+ j2 ) − j ( 2+ j 2 ) ( 1+ j5 )
6(2+ j2)(1+ j 5)
Y = 6+ j30+ 12+ j12+ j 8+12
6(−8+ j 12) = 30+ j 50
−48+ j 72
30+ j50= √ 302+502 ¿ tan−1 (50/30) ¿ √ 3400 ¿ 59.0362
−48+ j 72= √ 482 +722 ¿ tan−1 (72/−48) ¿ √7488 ¿−56.3099
Y = √3400 ¿ 59.0362 ÷ √7488 ¿−56.3099
Y =¿0.6738 ¿ ( 59.0362+56.3099 )−180
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
Trigonometric Methods 8
Y =¿0.6738 ¿−64.6539
r =0.6738 ,θ=−64.6539
x=rcosθ=0.6738 cos (−64.6539)=0.2884
y=rsinθ=0.6738 sin ( −64.6539 ) =−0.6089
Y =x +iy=0.2884− j 0.6089
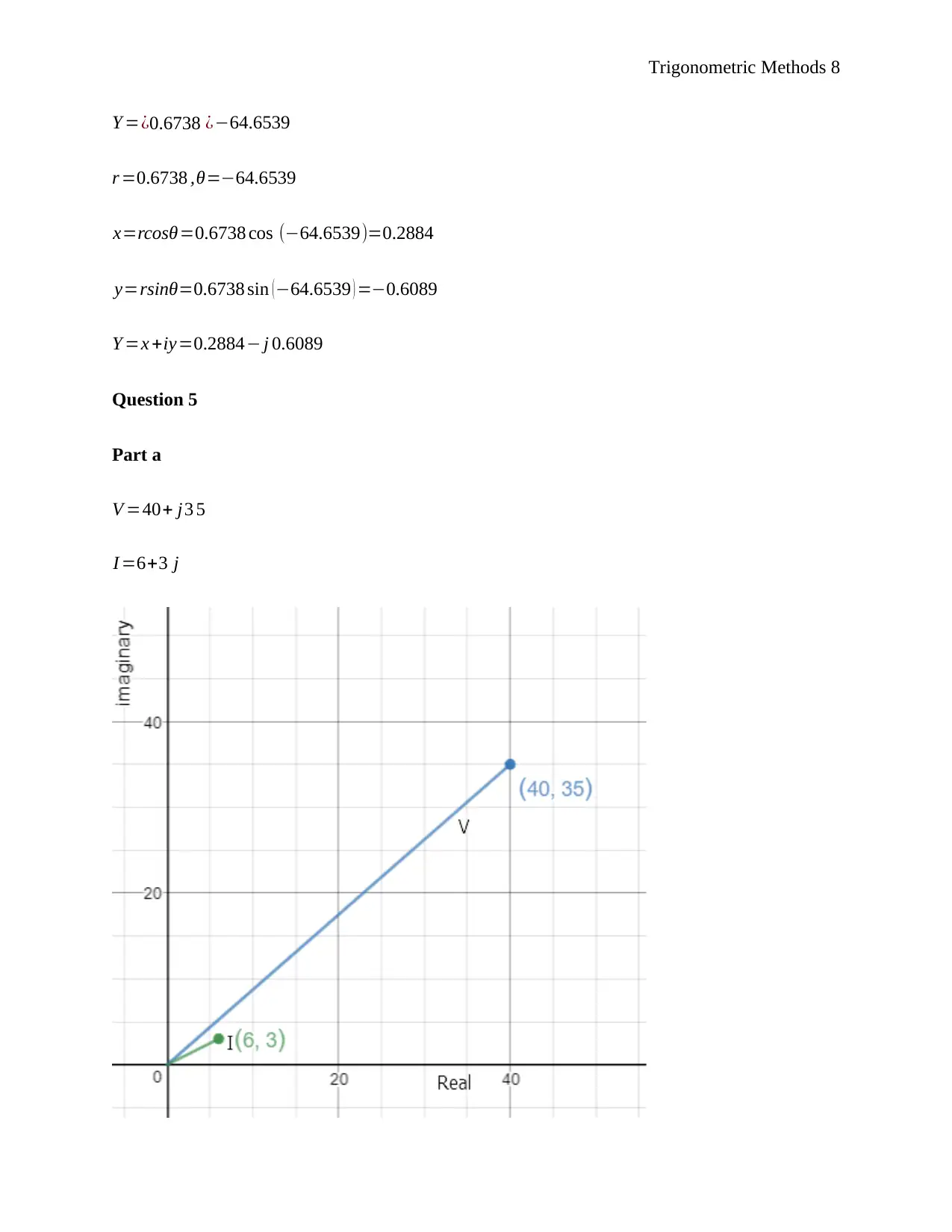
Question 5
Part a
V =40+ j3 5
I =6+3 j
Y =¿0.6738 ¿−64.6539
r =0.6738 ,θ=−64.6539
x=rcosθ=0.6738 cos (−64.6539)=0.2884
y=rsinθ=0.6738 sin ( −64.6539 ) =−0.6089
Y =x +iy=0.2884− j 0.6089
Question 5
Part a
V =40+ j3 5
I =6+3 j

Trigonometric Methods 9
Part b
θV =tan−1
( 35
40 )=tan−1 0.875=41.1859 °
θi =tan−1
( 3
6 )=tan−1 0.5=26.5651 °
Phase difference=θV −θi=41.1859−26.1859=14.6208 °
Part c
Power=¿ V ∨¿ I ∨cos ∅
|V |= √ 4 02+ 352=53.1507
|I |= √32 +62=6.7082
∅ =Phase difference=14.6208 °
Power=|V ||I |cos ∅ =53.1507 ×6.7082 cos 14.6208 °=345 Watts
Part b
θV =tan−1
( 35
40 )=tan−1 0.875=41.1859 °
θi =tan−1
( 3
6 )=tan−1 0.5=26.5651 °
Phase difference=θV −θi=41.1859−26.1859=14.6208 °
Part c
Power=¿ V ∨¿ I ∨cos ∅
|V |= √ 4 02+ 352=53.1507
|I |= √32 +62=6.7082
∅ =Phase difference=14.6208 °
Power=|V ||I |cos ∅ =53.1507 ×6.7082 cos 14.6208 °=345 Watts
⊘ This is a preview!⊘
Do you want full access?
Subscribe today to unlock all pages.

Trusted by 1+ million students worldwide
1 out of 9
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.
