Numeracy and Data Analysis: Wind Speed Analysis of Glasgow City
VerifiedAdded on 2023/06/10
|10
|1739
|392
AI Summary
This report focuses on the wind speed analysis of Glasgow city using numeracy and data analysis techniques such as mean, median, mode, range, and standard deviation. Linear forecasting model is also used to forecast future events. The report includes the arrangement of data in tabular form, wind speed chart, and calculation of m and c values for linear forecasting. The subject is Numeracy and Data Analysis, and the course code and college/university are not mentioned.
Contribute Materials
Your contribution can guide someone’s learning journey. Share your
documents today.

Data Numeracy
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

Contents
INTRODUCTION...........................................................................................................................3
TASK...............................................................................................................................................3
1.Arrangement of data in tabular form:.......................................................................................3
2.The wind speed of city Glasgow in following chart formats:..................................................4
3.Various types of data analysis is given below -........................................................................4
4.The value of m and c is calculated with the help of linear forecasting formula which is y =
mx + c..........................................................................................................................................7
CONCLUSION................................................................................................................................9
REFERENCES..............................................................................................................................10
INTRODUCTION...........................................................................................................................3
TASK...............................................................................................................................................3
1.Arrangement of data in tabular form:.......................................................................................3
2.The wind speed of city Glasgow in following chart formats:..................................................4
3.Various types of data analysis is given below -........................................................................4
4.The value of m and c is calculated with the help of linear forecasting formula which is y =
mx + c..........................................................................................................................................7
CONCLUSION................................................................................................................................9
REFERENCES..............................................................................................................................10
INTRODUCTION
Data analysis is the interpretation about a set of data on the basis of statements given. Data
analysis is a process of extracting, modelling, transporting data to discover important information
for decision making in business organisation (Dennis and Barnes, 2020). In this report, the wind
speed of city Glasgow has taken for consecutive 10 days to analyse the data for better decision
making with the help of numeracy and data analysis. There are various ways to determine the
data but in numeracy, data is calculated with the help of mean, median, mode, standard deviation
and range. The liner forecasting model of Glasgow has taken into consideration for forecasting
the future events with consideration of past values. Here also, the wind speed of Day 11 and Day
13 is forecasted with the help of forecasting model.
TASK
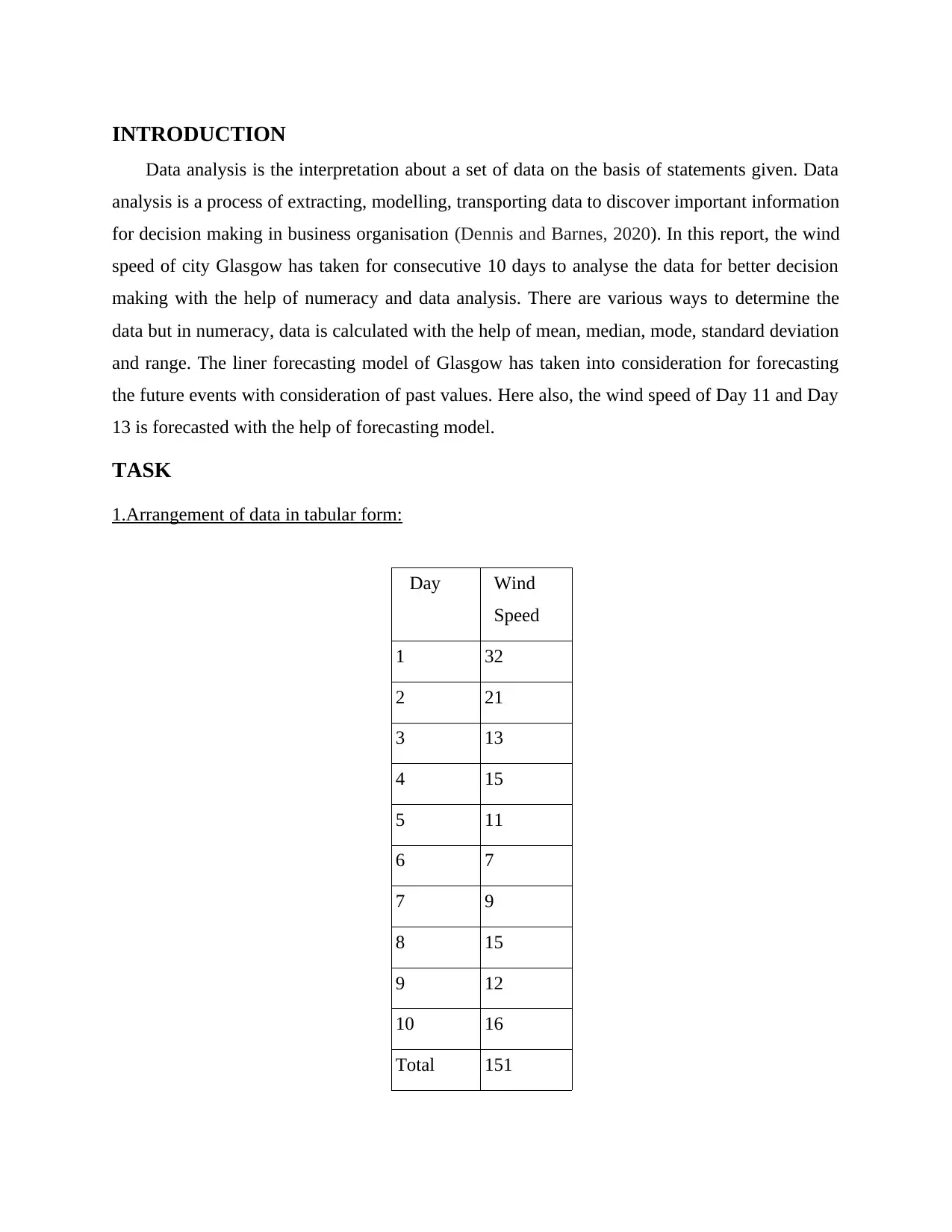
1.Arrangement of data in tabular form:
Day Wind
Speed
1 32
2 21
3 13
4 15
5 11
6 7
7 9
8 15
9 12
10 16
Total 151
Data analysis is the interpretation about a set of data on the basis of statements given. Data
analysis is a process of extracting, modelling, transporting data to discover important information
for decision making in business organisation (Dennis and Barnes, 2020). In this report, the wind
speed of city Glasgow has taken for consecutive 10 days to analyse the data for better decision
making with the help of numeracy and data analysis. There are various ways to determine the
data but in numeracy, data is calculated with the help of mean, median, mode, standard deviation
and range. The liner forecasting model of Glasgow has taken into consideration for forecasting
the future events with consideration of past values. Here also, the wind speed of Day 11 and Day
13 is forecasted with the help of forecasting model.
TASK
1.Arrangement of data in tabular form:
Day Wind
Speed
1 32
2 21
3 13
4 15
5 11
6 7
7 9
8 15
9 12
10 16
Total 151
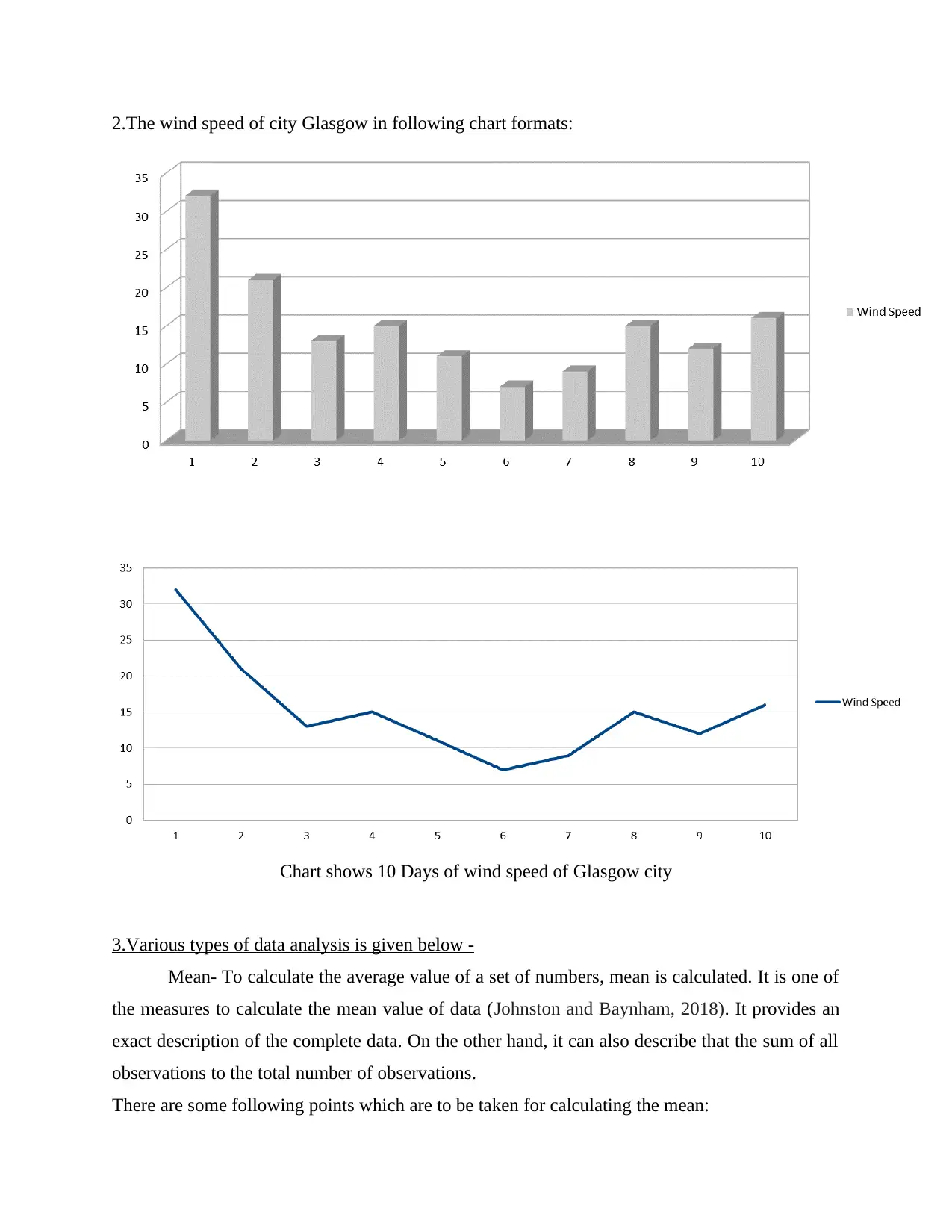
2.The wind speed of city Glasgow in following chart formats:
Chart shows 10 Days of wind speed of Glasgow city
3.Various types of data analysis is given below -
Mean- To calculate the average value of a set of numbers, mean is calculated. It is one of
the measures to calculate the mean value of data (Johnston and Baynham, 2018). It provides an
exact description of the complete data. On the other hand, it can also describe that the sum of all
observations to the total number of observations.
There are some following points which are to be taken for calculating the mean:
Chart shows 10 Days of wind speed of Glasgow city
3.Various types of data analysis is given below -
Mean- To calculate the average value of a set of numbers, mean is calculated. It is one of
the measures to calculate the mean value of data (Johnston and Baynham, 2018). It provides an
exact description of the complete data. On the other hand, it can also describe that the sum of all
observations to the total number of observations.
There are some following points which are to be taken for calculating the mean:
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

Point 1: Gather the available data.
Point 2: Compute the overall sum of data.
Point 3: Formulate the total number of data.
Point 4: Divide the overall sum of data with the total value of data.
Mean of Wind Speed = Sum of all observations / Total number of observations
Mean = 151 / 10
Mean = 15.1
Median: This data analytics method helps to find out the mid-value of the given list of
information after arranged in a proper order. The arrangement of information or interpretation
can be also done in ascending or descending in an appropriate manner. The formula of median is
very different for even and odd values of observations.
There are some following points to calculate the value of median:
Point 1: Categorize the data in ascending or descending order.
Point 2: Sum of total number from the data set.
Point 3: Identifying the value of median weather it is eve or odd
Point 4: If the total value of observations is provided in odd numbers. Formula to calculate the
median:
Median = N+1/2
Point 5: If the total value of observations is available in even. Then, the formula to calculate is:
Median = N/2
Here is the Median calculation of wind speed:
32, 21, 13, 15, 11, 7, 9, 15, 12, 16
7, 9, 11, 12, 13, 15, 15, 16, 21, 32
Median = 11 / 2
Median = 5.5th position
Median = 13+15 / 2
Median = 14
Mode: This data analytics method of mode is also one of the measures of central
tendency for identifying the value in a given set of data. Mode is that type of observation which
occurs highest times or has the maximum frequency in the given set of particular data.
Point 2: Compute the overall sum of data.
Point 3: Formulate the total number of data.
Point 4: Divide the overall sum of data with the total value of data.
Mean of Wind Speed = Sum of all observations / Total number of observations
Mean = 151 / 10
Mean = 15.1
Median: This data analytics method helps to find out the mid-value of the given list of
information after arranged in a proper order. The arrangement of information or interpretation
can be also done in ascending or descending in an appropriate manner. The formula of median is
very different for even and odd values of observations.
There are some following points to calculate the value of median:
Point 1: Categorize the data in ascending or descending order.
Point 2: Sum of total number from the data set.
Point 3: Identifying the value of median weather it is eve or odd
Point 4: If the total value of observations is provided in odd numbers. Formula to calculate the
median:
Median = N+1/2
Point 5: If the total value of observations is available in even. Then, the formula to calculate is:
Median = N/2
Here is the Median calculation of wind speed:
32, 21, 13, 15, 11, 7, 9, 15, 12, 16
7, 9, 11, 12, 13, 15, 15, 16, 21, 32
Median = 11 / 2
Median = 5.5th position
Median = 13+15 / 2
Median = 14
Mode: This data analytics method of mode is also one of the measures of central
tendency for identifying the value in a given set of data. Mode is that type of observation which
occurs highest times or has the maximum frequency in the given set of particular data.

Basic points to formulate the mode which are as follows:
Point 1: Arrange the values in ascending or descending order.
Point 2: Examine the given data.
Point 3: identify the value which occurs more in the given set of particular data.
Point 4: Then the most common number comes here, is our value of mode.
7, 9, 11, 12, 13, 15, 15, 16, 21, 32
Mode = 15
Range: This method shows the difference between the highest and the lowest value of the
range (Schwenger, 2018). This coming performance is known as the range of observation.
Various points to formulate the range which are as follows:
Point 1: Arrange the information in an appropriate manner
Point 2: Find the maximum and the minimum value.
Point 3: Formulate the distinguish between the highest and lowest
Point 4: Then, at the end the value of range is measured.
Formulate the range of wind speed:
Range = Highest value -Lowest value
= 32 – 7
= 25
Standard deviation: This method is the Degree of dispersion which tells how the values
are spread across in the sample of given data in comparison to the amount of mean (Westwood,
2021).
Following points to formulate the standard deviation:
Point 1: From very first, it formulates the value of mean.
Point 2: After this calculate the deviation for each and every provided value of mean.
Point 3: Measure the overall sum of all squares.
Point 4: Then, divide by the total sum of data.
Point 5: After measuring the above value, take a square root of the result.
Point 1: Arrange the values in ascending or descending order.
Point 2: Examine the given data.
Point 3: identify the value which occurs more in the given set of particular data.
Point 4: Then the most common number comes here, is our value of mode.
7, 9, 11, 12, 13, 15, 15, 16, 21, 32
Mode = 15
Range: This method shows the difference between the highest and the lowest value of the
range (Schwenger, 2018). This coming performance is known as the range of observation.
Various points to formulate the range which are as follows:
Point 1: Arrange the information in an appropriate manner
Point 2: Find the maximum and the minimum value.
Point 3: Formulate the distinguish between the highest and lowest
Point 4: Then, at the end the value of range is measured.
Formulate the range of wind speed:
Range = Highest value -Lowest value
= 32 – 7
= 25
Standard deviation: This method is the Degree of dispersion which tells how the values
are spread across in the sample of given data in comparison to the amount of mean (Westwood,
2021).
Following points to formulate the standard deviation:
Point 1: From very first, it formulates the value of mean.
Point 2: After this calculate the deviation for each and every provided value of mean.
Point 3: Measure the overall sum of all squares.
Point 4: Then, divide by the total sum of data.
Point 5: After measuring the above value, take a square root of the result.

Formulate the value of standard deviation of wind speed:
Standard Deviation= √∑ (xi – μ) 2 / N
= √454.9 / 10
= √45.49
= 6.744
4.The value of m and c is calculated with the help of linear forecasting formula which is y = mx
+ c.
Linear Forecasting Model: This model of forecasting is helpful in future to evaluate the
future forecasting by using past events (Wright, 2019).
There are some steps in linear forecasting theory which are as follows:
Step1: Firstly, search the problem.
Step2: Through surveys, information is collected.
Step3: Find out the information from start.
Step4: Selection of model which is useful for future activities.
Step5: Carefully analyse the information.
y = mx + C
where, 'y' is referring to Dependent Factor,
'mx' is refers to Independent factor and
'c' refers to constant Factor
There are some steps involved in calculation of ‘m’:
Step1: First, find the value of total number and then multiply both the term and the count of total
number.
Step2: Sum up the 'x' and 'y' variable terms separately.
Step3: Then, subtracting the Step2 form step1.
Step4: Calculate the square of x and multiply with count of total number.
Step5: Also the square of sum of 'x’ is calculated.
Step6: Then, subtract the Step5 from Step4.
Step7: At last, divide the value of Step3 with Step6.
Standard Deviation= √∑ (xi – μ) 2 / N
= √454.9 / 10
= √45.49
= 6.744
4.The value of m and c is calculated with the help of linear forecasting formula which is y = mx
+ c.
Linear Forecasting Model: This model of forecasting is helpful in future to evaluate the
future forecasting by using past events (Wright, 2019).
There are some steps in linear forecasting theory which are as follows:
Step1: Firstly, search the problem.
Step2: Through surveys, information is collected.
Step3: Find out the information from start.
Step4: Selection of model which is useful for future activities.
Step5: Carefully analyse the information.
y = mx + C
where, 'y' is referring to Dependent Factor,
'mx' is refers to Independent factor and
'c' refers to constant Factor
There are some steps involved in calculation of ‘m’:
Step1: First, find the value of total number and then multiply both the term and the count of total
number.
Step2: Sum up the 'x' and 'y' variable terms separately.
Step3: Then, subtracting the Step2 form step1.
Step4: Calculate the square of x and multiply with count of total number.
Step5: Also the square of sum of 'x’ is calculated.
Step6: Then, subtract the Step5 from Step4.
Step7: At last, divide the value of Step3 with Step6.
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

= 10*721 – 55*151 / 10*385 – 55*55
= 7210 – 8305 / 3850 – 3025
= -1095 / 825
= -1.33
Steps for calculation of 'c':
Step1: To measure the sum of 'y' variable
Step2: Then, compute the value of ‘m’
Step3: Calculate the sum of 'x' variable and then multiply both 'm' and sum of 'x'
Step4: Then, deducted the sum of 'mx' from sum of 'y'
Step5: After that divide the value of Step4 by 'n'
= 151 - (-1.33) (55) / 10
= 151 - (- 73.15)
= 151 + 73.15 / 10
= 224.15 / 10
c = 22.415
Wind Speed on Day11:
m = -1.33, c = 22.415, x = 11
y = mx + c
= -1.33 * 11 + 22.415
= -14.63 + 22.415
m = 7.785
Wind Speed on Day13:
m = -1.33, c = 22.415, x = 13
= 7210 – 8305 / 3850 – 3025
= -1095 / 825
= -1.33
Steps for calculation of 'c':
Step1: To measure the sum of 'y' variable
Step2: Then, compute the value of ‘m’
Step3: Calculate the sum of 'x' variable and then multiply both 'm' and sum of 'x'
Step4: Then, deducted the sum of 'mx' from sum of 'y'
Step5: After that divide the value of Step4 by 'n'
= 151 - (-1.33) (55) / 10
= 151 - (- 73.15)
= 151 + 73.15 / 10
= 224.15 / 10
c = 22.415
Wind Speed on Day11:
m = -1.33, c = 22.415, x = 11
y = mx + c
= -1.33 * 11 + 22.415
= -14.63 + 22.415
m = 7.785
Wind Speed on Day13:
m = -1.33, c = 22.415, x = 13

y = mx + c
= -1.33 * 13 + 22.415
= -17.29 + 22.415
= 5.125
CONCLUSION
As it is concluded from the above report that the mean, median, mode, range and standard
deviation is very much useful to assess and calculation of statistical data. The information which
has been taken into account is of wind speed for 10 consecutive days of Glasgow. The data so far
gathered is being showing with the assistance of charts and graphs that becomes easier to
understand the forecasted futuristic conditions.
= -1.33 * 13 + 22.415
= -17.29 + 22.415
= 5.125
CONCLUSION
As it is concluded from the above report that the mean, median, mode, range and standard
deviation is very much useful to assess and calculation of statistical data. The information which
has been taken into account is of wind speed for 10 consecutive days of Glasgow. The data so far
gathered is being showing with the assistance of charts and graphs that becomes easier to
understand the forecasted futuristic conditions.

REFERENCES
Books and Journals
Dennis, M. and Barnes, M., 2020. Math and numeracy in young adults with spina bifida and
hydrocephalus. Developmental Neuropsychology. 21(2). pp.141-155.
Johnston, B. and Baynham, M., 2018. 'Invention within limits': numeracy practices of young
unemployed people. Literacy and Numeracy Studies. 8(1). pp.51-65.
Schwenger, B., 2018. Research on training wheels–embedding academic literacy and numeracy
in vocational pedagogy through action research. Educational Action Research. 26(2).
pp.288-300.
Westwood, P., 2021. Numeracy and learning difficulties: Approaches to teaching and
assessment. David Fulton Publishers.
Wright, K., 2019. A written assessment is an invalid test of numeracy skills. British Journal of
Nursing. 16(13). pp.828-831.
Books and Journals
Dennis, M. and Barnes, M., 2020. Math and numeracy in young adults with spina bifida and
hydrocephalus. Developmental Neuropsychology. 21(2). pp.141-155.
Johnston, B. and Baynham, M., 2018. 'Invention within limits': numeracy practices of young
unemployed people. Literacy and Numeracy Studies. 8(1). pp.51-65.
Schwenger, B., 2018. Research on training wheels–embedding academic literacy and numeracy
in vocational pedagogy through action research. Educational Action Research. 26(2).
pp.288-300.
Westwood, P., 2021. Numeracy and learning difficulties: Approaches to teaching and
assessment. David Fulton Publishers.
Wright, K., 2019. A written assessment is an invalid test of numeracy skills. British Journal of
Nursing. 16(13). pp.828-831.
1 out of 10
Related Documents
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.


