Numeracy and Data Analysis for Wind Speed Data Table
VerifiedAdded on 2023/06/12
|9
|1602
|344
AI Summary
This report includes the calculation of mean, median, mode, range and standard deviation of wind speed data table of a particular country. It also includes two types of charts and linear forecasting theory for the computation of value 'm' and 'c' which helpful to calculate the day11 and day 13 wind speed.
Contribute Materials
Your contribution can guide someone’s learning journey. Share your
documents today.

Numeracy and Data
Analysis
Analysis
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

Contents
INTRODUCTION...........................................................................................................................3
MAIN BODY..................................................................................................................................3
1. Compute the data table of wind speed (Gayle and Lambert., 2018).......................................3
2. Wind Speed data of the country in Chart Form:......................................................................3
3. There are several types to data analysis are as follows:..........................................................4
4. Compute the value of m and c with the help of linear forecasting formula which is y = mx +
c....................................................................................................................................................6
CONCLUSION................................................................................................................................8
REFERENCES................................................................................................................................9
INTRODUCTION...........................................................................................................................3
MAIN BODY..................................................................................................................................3
1. Compute the data table of wind speed (Gayle and Lambert., 2018).......................................3
2. Wind Speed data of the country in Chart Form:......................................................................3
3. There are several types to data analysis are as follows:..........................................................4
4. Compute the value of m and c with the help of linear forecasting formula which is y = mx +
c....................................................................................................................................................6
CONCLUSION................................................................................................................................8
REFERENCES................................................................................................................................9
INTRODUCTION
It is tool of statistics which help to prepare the data systematically and understandable.
The following report shows the speed of wind of a particular country on the basis of numeracy
and data analysis where it includes tabular data sheet and two types of charts for calculating
mean, median, mode, range and standard deviation. It is also including the linear forecasting
theory for the computation of value 'm' and 'c' which helpful to calculate the day11 and day 13
wind speed. This data is useful as a base of future forecasting for knowledge and skills.
MAIN BODY
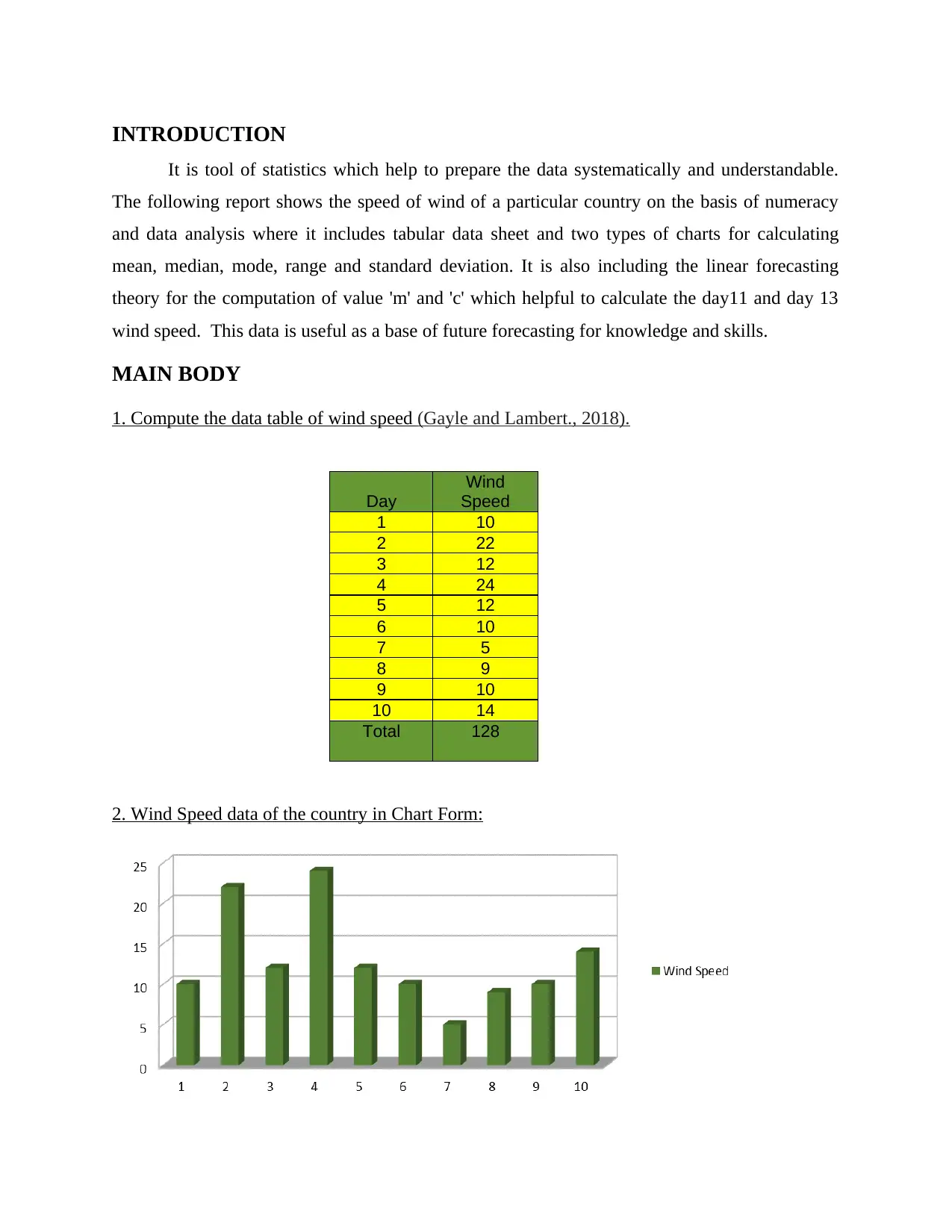
1. Compute the data table of wind speed (Gayle and Lambert., 2018).
Day
Wind
Speed
1 10
2 22
3 12
4 24
5 12
6 10
7 5
8 9
9 10
10 14
Total 128
2. Wind Speed data of the country in Chart Form:
It is tool of statistics which help to prepare the data systematically and understandable.
The following report shows the speed of wind of a particular country on the basis of numeracy
and data analysis where it includes tabular data sheet and two types of charts for calculating
mean, median, mode, range and standard deviation. It is also including the linear forecasting
theory for the computation of value 'm' and 'c' which helpful to calculate the day11 and day 13
wind speed. This data is useful as a base of future forecasting for knowledge and skills.
MAIN BODY
1. Compute the data table of wind speed (Gayle and Lambert., 2018).
Day
Wind
Speed
1 10
2 22
3 12
4 24
5 12
6 10
7 5
8 9
9 10
10 14
Total 128
2. Wind Speed data of the country in Chart Form:
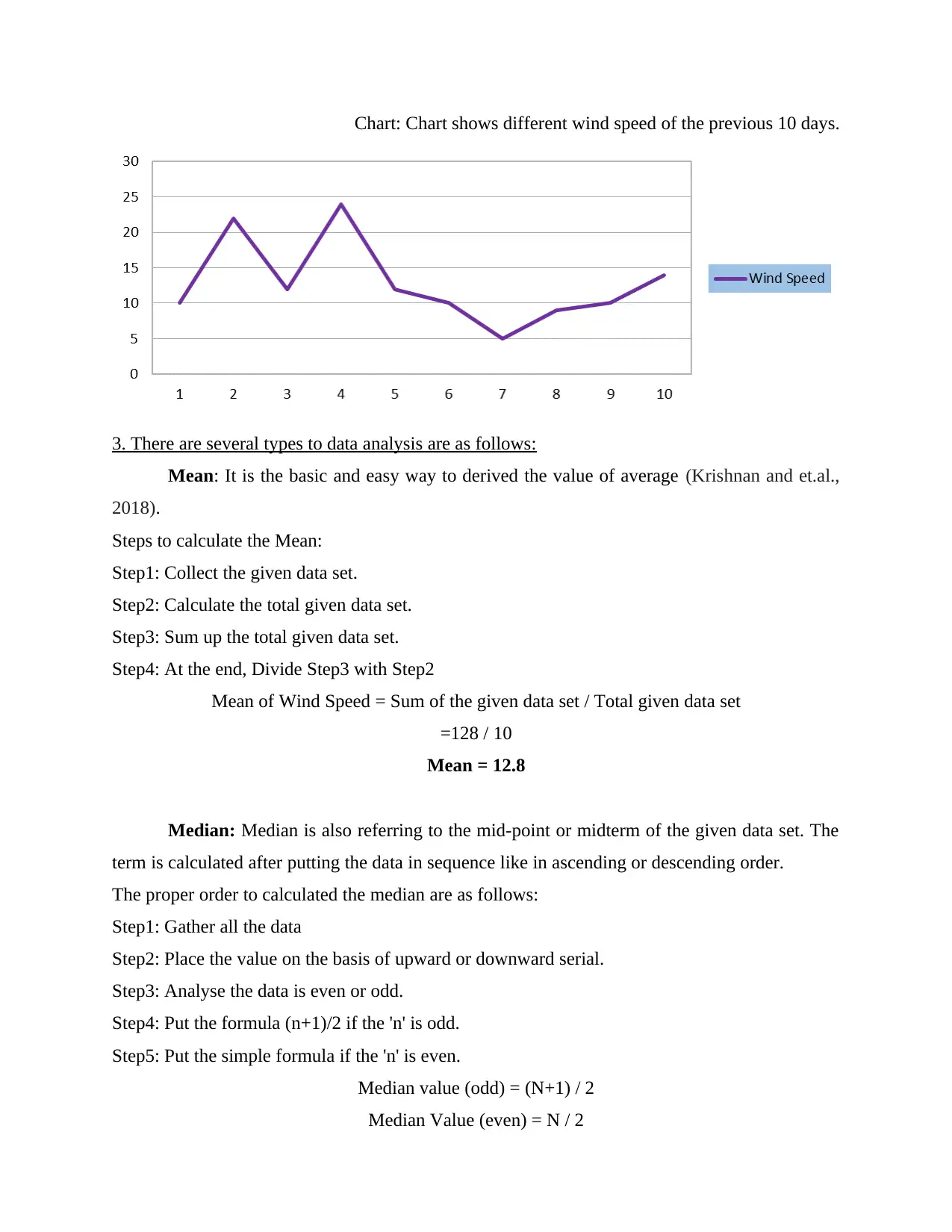
Chart: Chart shows different wind speed of the previous 10 days.
3. There are several types to data analysis are as follows:
Mean: It is the basic and easy way to derived the value of average (Krishnan and et.al.,
2018).
Steps to calculate the Mean:
Step1: Collect the given data set.
Step2: Calculate the total given data set.
Step3: Sum up the total given data set.
Step4: At the end, Divide Step3 with Step2
Mean of Wind Speed = Sum of the given data set / Total given data set
=128 / 10
Mean = 12.8
Median: Median is also referring to the mid-point or midterm of the given data set. The
term is calculated after putting the data in sequence like in ascending or descending order.
The proper order to calculated the median are as follows:
Step1: Gather all the data
Step2: Place the value on the basis of upward or downward serial.
Step3: Analyse the data is even or odd.
Step4: Put the formula (n+1)/2 if the 'n' is odd.
Step5: Put the simple formula if the 'n' is even.
Median value (odd) = (N+1) / 2
Median Value (even) = N / 2
3. There are several types to data analysis are as follows:
Mean: It is the basic and easy way to derived the value of average (Krishnan and et.al.,
2018).
Steps to calculate the Mean:
Step1: Collect the given data set.
Step2: Calculate the total given data set.
Step3: Sum up the total given data set.
Step4: At the end, Divide Step3 with Step2
Mean of Wind Speed = Sum of the given data set / Total given data set
=128 / 10
Mean = 12.8
Median: Median is also referring to the mid-point or midterm of the given data set. The
term is calculated after putting the data in sequence like in ascending or descending order.
The proper order to calculated the median are as follows:
Step1: Gather all the data
Step2: Place the value on the basis of upward or downward serial.
Step3: Analyse the data is even or odd.
Step4: Put the formula (n+1)/2 if the 'n' is odd.
Step5: Put the simple formula if the 'n' is even.
Median value (odd) = (N+1) / 2
Median Value (even) = N / 2
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

Calculation of Wind speed median are as follows:
10, 22, 12, 24, 12, 10, 5, 9, 10, 14
5, 9, 10, 10, 10, 12, 12, 14, 22, 24
Median = 10/2
Median = 5th term
Median= 10
Mode: It calculate the value of mode by selecting the term which is highly repeatable in a
given data set (Nalawade, More and Bhola., 2019).
Steps to calculated the Mode:
Step1: Place the term according to the ascending or descending order.
Step2: Observe the data deeply
Step3: Observe the repeated number in a given data set.
Step4: Select the highly repeated number.
5, 9, 10, 10, 10, 12, 12, 14, 22, 24
Mode of Wind Speed = 10
Range: The Range is the difference of highest and lowest value.
Following are the steps to calculate the range:
Step1: Position the data set on the basis of sorting.
Step2: Pick the value topmost and bottom most value.
Step3: Compute the difference between topmost and bottom most value.
Step4: After compute the Step3 the value of range is derived.
Calculation of Wind Speed Range:
Range = Highest value – Lowest Value
= 24 – 5
Range = 19
Standard Deviation: The standard deviation is value is determining the total number of
data is spread in relation to the average value or mean.
Following are the standard deviation steps:
Step1: Pick out the mean value.
Step2: Compute all data set value through deviation from mean.
Step3: Compute the sum of all squares.
Step4: Then divide by the total count of data.
10, 22, 12, 24, 12, 10, 5, 9, 10, 14
5, 9, 10, 10, 10, 12, 12, 14, 22, 24
Median = 10/2
Median = 5th term
Median= 10
Mode: It calculate the value of mode by selecting the term which is highly repeatable in a
given data set (Nalawade, More and Bhola., 2019).
Steps to calculated the Mode:
Step1: Place the term according to the ascending or descending order.
Step2: Observe the data deeply
Step3: Observe the repeated number in a given data set.
Step4: Select the highly repeated number.
5, 9, 10, 10, 10, 12, 12, 14, 22, 24
Mode of Wind Speed = 10
Range: The Range is the difference of highest and lowest value.
Following are the steps to calculate the range:
Step1: Position the data set on the basis of sorting.
Step2: Pick the value topmost and bottom most value.
Step3: Compute the difference between topmost and bottom most value.
Step4: After compute the Step3 the value of range is derived.
Calculation of Wind Speed Range:
Range = Highest value – Lowest Value
= 24 – 5
Range = 19
Standard Deviation: The standard deviation is value is determining the total number of
data is spread in relation to the average value or mean.
Following are the standard deviation steps:
Step1: Pick out the mean value.
Step2: Compute all data set value through deviation from mean.
Step3: Compute the sum of all squares.
Step4: Then divide by the total count of data.

Step5: After the above calculated value take a square root of that result.
Calculation of wind speed standard deviation:
Standard Deviation= √∑ (xi – μ) 2 / N
= √311.6 / 10
= √31.16
Standard deviation = 5.582
4. Compute the value of m and c with the help of linear forecasting formula which is y = mx + c.
Linear Forecasting Model: It is the theory which is helpful for future forecasting with
the help of past reports (O'Neill, Booth and Lamb., 2018.).
Linear Forecasting theory steps are as follows:
Step1: Observe the problem arising.
Step2: Gather the information through surveys.
Step3: Find out the information form starting.
Step4: Choose the model which is helpful for future activities.
Step5: Observe all the information carefully.
y = mx + C
where, 'y' is referring to Dependent Factor,
'mx' is refers to Independent factor and
'c' refers to constant Factor
There is sequence to calculate the 'm':
Step1: First, find the count of total number and then multiply both the term and the count of total
number
Step2: Sum up the 'x' and 'y' variable term separately
Step3: Then Subtract the Step2 form step1.
Step4: Calculate the square of x and multiply with count of total number.
Step5: Also calculate the square of sum of 'x'.
Step6: Then subtract the Step5 from Step4.
Step7: At the end, Divide the value of Step3 with Step6.
Calculation of wind speed standard deviation:
Standard Deviation= √∑ (xi – μ) 2 / N
= √311.6 / 10
= √31.16
Standard deviation = 5.582
4. Compute the value of m and c with the help of linear forecasting formula which is y = mx + c.
Linear Forecasting Model: It is the theory which is helpful for future forecasting with
the help of past reports (O'Neill, Booth and Lamb., 2018.).
Linear Forecasting theory steps are as follows:
Step1: Observe the problem arising.
Step2: Gather the information through surveys.
Step3: Find out the information form starting.
Step4: Choose the model which is helpful for future activities.
Step5: Observe all the information carefully.
y = mx + C
where, 'y' is referring to Dependent Factor,
'mx' is refers to Independent factor and
'c' refers to constant Factor
There is sequence to calculate the 'm':
Step1: First, find the count of total number and then multiply both the term and the count of total
number
Step2: Sum up the 'x' and 'y' variable term separately
Step3: Then Subtract the Step2 form step1.
Step4: Calculate the square of x and multiply with count of total number.
Step5: Also calculate the square of sum of 'x'.
Step6: Then subtract the Step5 from Step4.
Step7: At the end, Divide the value of Step3 with Step6.

m = (10*643 – 55*128) / (10*385 – 3025)
= (6430 – 7040) / (3850 – 3025)
= -610 / 825
m = 0.74
Compute the worth of 'c' by following steps (Sio and Ismail., 2019):
Step1: Add the total terms of 'y' variable
Step2: Find the value of 'm' then calculate the 'm' with sum of 'x' variable.
Step4: Compute the difference between Step2 and Step1.
Step5: Calculate the total count of 'x' variable.
Step6: Then the result is find out by dividing the outcome of step 4 with 'n'.
c = (128 – 0.74*55) / 10
= (128 – 40.7) / 10
= 87.3 / 10
c = 8.73
Wind Speed of Day 11
m = 0.74, c = 8.73, x = 11
y = mx + c
= (0.74*11) + 8.73
=8.14 + 8.73
y = 16.87
Wind Speed of Day 13
m = 0.74, c = 8.73, x = 13
y = mx + c
= (0.74*13) + 8.73
= 9.62+ 18.35
y = 27.97
= (6430 – 7040) / (3850 – 3025)
= -610 / 825
m = 0.74
Compute the worth of 'c' by following steps (Sio and Ismail., 2019):
Step1: Add the total terms of 'y' variable
Step2: Find the value of 'm' then calculate the 'm' with sum of 'x' variable.
Step4: Compute the difference between Step2 and Step1.
Step5: Calculate the total count of 'x' variable.
Step6: Then the result is find out by dividing the outcome of step 4 with 'n'.
c = (128 – 0.74*55) / 10
= (128 – 40.7) / 10
= 87.3 / 10
c = 8.73
Wind Speed of Day 11
m = 0.74, c = 8.73, x = 11
y = mx + c
= (0.74*11) + 8.73
=8.14 + 8.73
y = 16.87
Wind Speed of Day 13
m = 0.74, c = 8.73, x = 13
y = mx + c
= (0.74*13) + 8.73
= 9.62+ 18.35
y = 27.97
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

CONCLUSION
The above result shows the numeracy and data analysis which include mean, median,
mode, range and standard deviation calculation of a particular table of wind speed data of 10
days and in relation to data table it is also shows the two types of charts. Using Linear
forecasting theory, the value of 'm' and worth of 'c' is calculated which help to calculate the wind
Speed of the Day 11 and Day 13.
The above result shows the numeracy and data analysis which include mean, median,
mode, range and standard deviation calculation of a particular table of wind speed data of 10
days and in relation to data table it is also shows the two types of charts. Using Linear
forecasting theory, the value of 'm' and worth of 'c' is calculated which help to calculate the wind
Speed of the Day 11 and Day 13.

REFERENCES
Books and Journals
Gayle, V. and Lambert, P., 2018. What is quantitative longitudinal data analysis?. Bloomsbury
Publishing.
Krishnan, S., Rohman, A., Welter, J. and Dozor, A.J., 2018. Relationship between health literacy
in parents and asthma control in their children: a prospective study in a diverse suburban
population. Pediatric Allergy, Immunology, and Pulmonology, 31(4), pp.221-225.
Nalawade, R.K., More, D.K. and Bhola, S.S., 2019. Employability Skills Required for
Functional Areas of Management. IUP Journal of Soft Skills, 13(1).
O'Neill, M.M., Booth, S.R. and Lamb, J.T., 2018. Using NVivo™ for literature reviews: The
eight step pedagogy (N7+ 1). Qualitative Report: an online journal dedicated to qualitative
research since 1990, pp.21-39.
Sio, J. and Ismail, R., 2019. Binary logistic regression analysis of instructional leadership factors
affecting English language literacy in primary schools. 3L, Language, Linguistics,
Literature, 25(2).
Books and Journals
Gayle, V. and Lambert, P., 2018. What is quantitative longitudinal data analysis?. Bloomsbury
Publishing.
Krishnan, S., Rohman, A., Welter, J. and Dozor, A.J., 2018. Relationship between health literacy
in parents and asthma control in their children: a prospective study in a diverse suburban
population. Pediatric Allergy, Immunology, and Pulmonology, 31(4), pp.221-225.
Nalawade, R.K., More, D.K. and Bhola, S.S., 2019. Employability Skills Required for
Functional Areas of Management. IUP Journal of Soft Skills, 13(1).
O'Neill, M.M., Booth, S.R. and Lamb, J.T., 2018. Using NVivo™ for literature reviews: The
eight step pedagogy (N7+ 1). Qualitative Report: an online journal dedicated to qualitative
research since 1990, pp.21-39.
Sio, J. and Ismail, R., 2019. Binary logistic regression analysis of instructional leadership factors
affecting English language literacy in primary schools. 3L, Language, Linguistics,
Literature, 25(2).
1 out of 9
Related Documents
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.
