Coordinate Geometry: Determining Points and Mirror Images on X-Axis

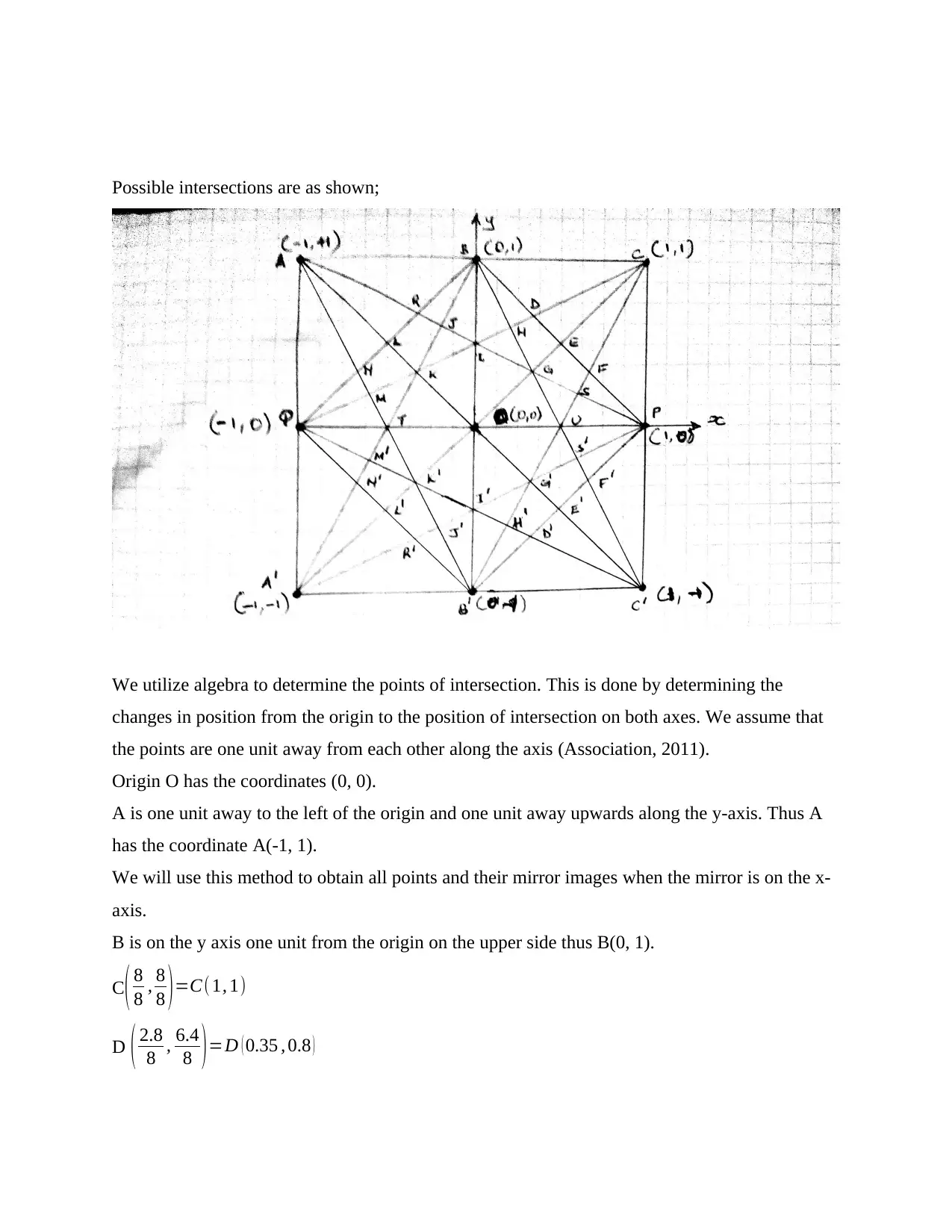
This assignment focuses on coordinate geometry, specifically determining points of intersection and their mirror images across the x-axis. It uses algebraic methods to calculate the coordinates of various points and their reflections. The origin is set as (0, 0), and other points are located relative to it. By reflecting points across the x-axis, the y-coordinates are inverted while the x-coordinates remain the same. The document includes a calculation of the number of possible combinations of selecting two points from a set of nine, demonstrating combinatorial principles in geometry. Desklib provides this solution and other resources for students seeking assistance with their assignments.

Possible intersections are as shown;
We utilize algebra to determine the points of intersection. This is done by determining the
changes in position from the origin to the position of intersection on both axes. We assume that
the points are one unit away from each other along the axis (Association, 2011).
Origin O has the coordinates (0, 0).
A is one unit away to the left of the origin and one unit away upwards along the y-axis. Thus A
has the coordinate A(-1, 1).
We will use this method to obtain all points and their mirror images when the mirror is on the x-
axis.
B is on the y axis one unit from the origin on the upper side thus B(0, 1).
C( 8
8 , 8
8 )=C(1, 1)
D ( 2.8
8 , 6.4
8 )=D ( 0.35 , 0.8 )

E ( 4
8 , 4
8 )=E ( 0.5 , 0.5 )
F (5.4
8 , 2.8
8 )=F ( 0.675 ,0.35 )
G ( 2.8
8 , 2.8
8 )=G(0.35 , 0.35)
H ( 1.6
8 , 4.8
8 )=H (0.2 , 0.6)
I ( 0
8 , 4
8 )=I ( 0 , 0.5 )
J ( −1.6
8 , 4.8
8 )=J (−0.2, 0.6)
K (−2.8
8 , 2.8
8 )=K (−0.35 , 0.35)
L (−4
8 , 4
8 )=L(−0.5 ,0.5)
M (−4.8
8 , 1.6
8 )=M (−0.6 ,0.2)
N (−5.4
8 , 2.8
8 )=N (−0.675 , 0.35)
O (0, 0)
P( 8
8 , 0
8 )=P(1 , 0)
Q (−8
8 , 0
8 )=Q(−1 , 0)
R (−2.8
8 , 6.4
8 )=R(−0.35 ,0.8)
S ( 4.8
8 , 1.6
8 )=S(0.6 ,0.2)
T ( −4
8 , 0
8 ) =T (−0.5 , 0)
Having a mirror on the x-axis, we get the following coordinates of the points below;
A’ (-1, -1)

B’ (0, -1)
C’ (1, -10
D’ (0.35, -0.8)
E’ (0.5, -0.5)
F’ (0.675, -0.35)
G’ (0.35, -0.35)
H’ (0.2, -0.6)
I’ (0, -0.5)
J’ (-0.2, -0.6)
K’ (-0.35, -0.35)
L’ (-0.5, -0.5)
M’ (-0.6, -0.2)
N’ (-0.675, -0.35)
R’ (-0.35, -0.8)
S’ (0.6, -0.2)
There are 9 pins on the board and we choose any two points to choose from. Therefore, there are
9C2 possibilities (Jerome Rosner, 2008).
9C2 = 9∗8
1∗2 =36

References
Association, A. O., 2011. Journal of the American Optometric Association. California: American
Optometric Association.
Jerome Rosner, J. R., 2008. Pediatric optometry. second ed. Michigan: Butterworths Tolley Limited.