Calculus 1 Assignment: Comprehensive Solutions and Explanations

This document provides comprehensive solutions to a Calculus 1 homework assignment. The solutions cover a range of calculus concepts, including finding critical points, determining domains, analyzing monotone intervals, applying differentiation rules (quotient rule, power rule), finding inflection points, solving optimization problems (perimeter and area), calculating derivatives of complex functions (using product rule, chain rule, and logarithmic rules), evaluating integrals, and determining consumer surplus. Each solution is presented with detailed steps and explanations, making it a valuable resource for students studying calculus. The assignment addresses problems related to derivatives, integrals, and applications of calculus in various contexts. The solutions are designed to help students understand the concepts and improve their problem-solving skills. The document is contributed by a student and is available on Desklib, a platform offering AI-based study tools.

Running head: CALCULUS 1
Calculus
Student Name
Institution

CALCULUS 2
Question 1
Step 1: Identify the critical points by equating the derivative to zero
F(x) = x3+x2-5x+2
dy
dx = 3 x2 +2x-5
3 x2 +2x-5 = 0
Use the equation:
X=−b ± √b2−4 ac
2a = −2± √ 22−4∗3∗−5
2∗3 =−5
3 , 1
Step 2: find the domain
Since this is a quadratic equation, the domain is: −∞<¿x¿ ∞
Step 3: check the behavior of the monotone intervals
Since the critical points fall within the domain, we form the monotone intervals as:
−∞<¿X← 5
3 , −5
3 <x<1, 1<x< ∞
For −∞<¿x← 5
3 ;
We pick a value within the range (x=-1) and check the sign value of dy
dx
F(x) = 3(−1)2 +2(-1)-5=-4
For −5
3 <x<1;
We pick a value within the range (x=0) and check the sign value of dy
dx
F(x) = 3(0)2 +2(0)-5=-5
We pick a value within the range (x=2) and check the sign value of dy
dx

CALCULUS 3
F(x) = 3(2)2 +2(2)-5=11
−∞ <¿x← 5
3
−5
3 <x<1 1<x<∞
Sign - - +
Behavior decreasing Decreasing Increasing
Question 2
Step 1: Find the first derivative
f(x) = x−2
x+ 1
Using quotient rule of differentiation;
d
dx ( x −2 ) ( x +1 ) − d
dx ( x +1 ) ( x −2 )
( x +1)2
= 1 ( x +1 ) −1( x−2)
(x +1)2 = 3
( x+1 )2
f ‘(x) = 3
( x+1 )2
Step 1: equate first derivative to zero and find where the first derivative is undefined
3
( x+1 ) 2 =0
The function 3
( x+1 )2 is undefined at x=-1 since we cannot divide by zero
Step 2: finding the domain
Domain: x<-1 or x>-1
Step 3: finding singularity points
x¿-1
Since f(x) is not defined at x¿-1, then no inflection points. Inflection points exists where a
function, f(x) is defined at all points within the domain (Hayter, 2013; Stephens, 2011).

CALCULUS 4
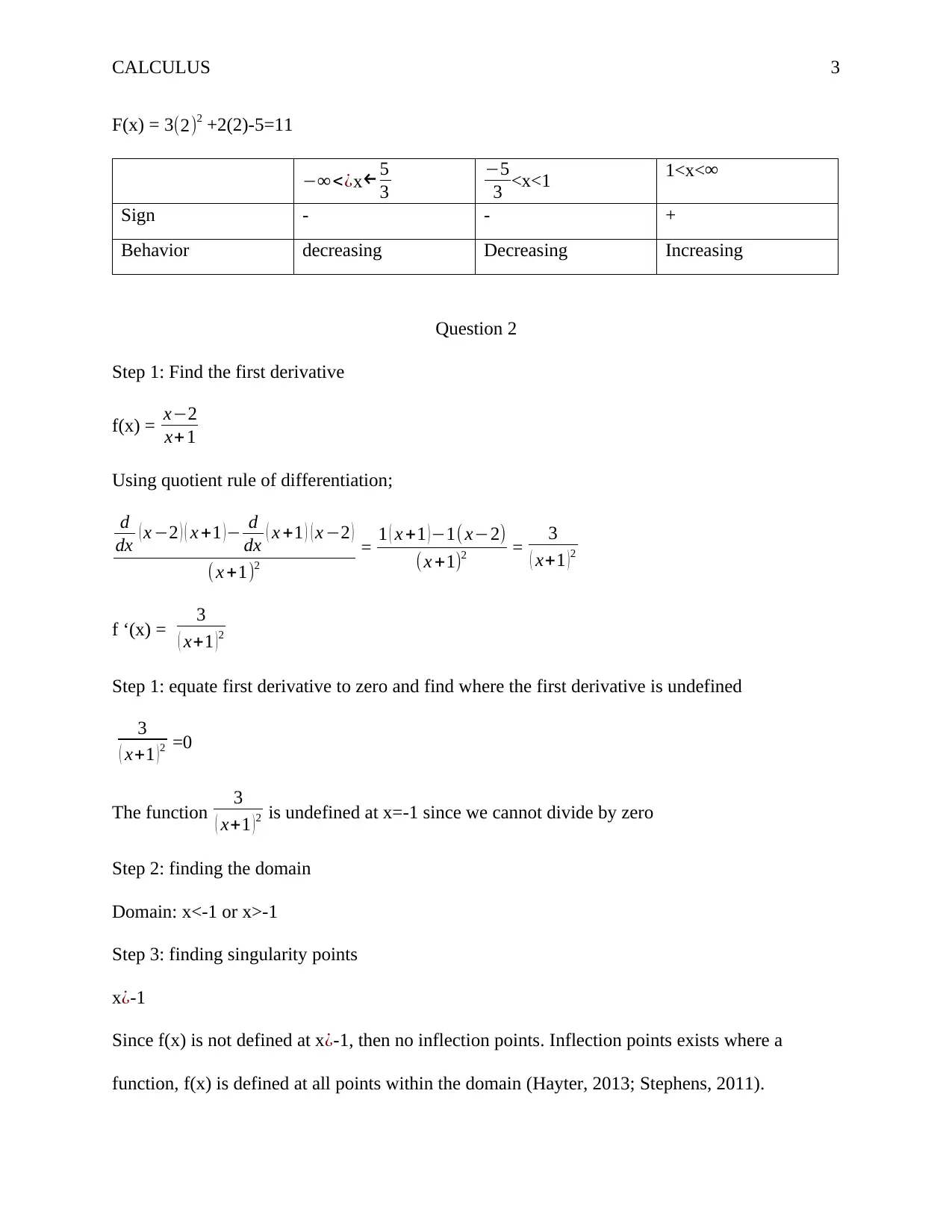
Question 3
Question 4
Step 1: Perimeter of the fence:
L+W = 80
Step 2: Making L the subject of the formula
L = 80-w
Step 3: Calculate the area
Area = L*W
= (80-W)W

CALCULUS 5
A = 80W-W 2
Step 4: differentiate and equate to zero
dA
dW = 80-2W
80-2W=0
W= 40
L= 80-W = 80-40 = 40
Maximum area = L*W = 40*40 = 1600 ft2
Question 5
Step 1: expressing width in terms of x
32 = 2x+w
W= 32-2x
Step 2: calculating area with width (w) expressed in x
Area, A = L*W
A = x*w
A = x(32-2x) = 32x - 2x2
Step 3: calculating the x values which maximizes cross-sectional area by differentiating area (A)
function
dA
dx = 32 – 4x
Step 4: equating dA
dx to zero
32 – 4x = 0
4x= 32
Thus

CALCULUS 6
X= 8
W= 32-2x = 32-2(8) = 16
Step 4: checking whether the extreme point is maximum by calculating the second derivative of
area function
d2 A
d x2 = – 4
Thus the extreme point is maximum
Step 5: calculating the maximum cross-sectional area
A = L*W
= 16*8
= 128 inch2
Question 6
a)
( 6 x8 ) ( 3 x−4 ) = 18( x−4 ) ( x8 )
Using the power rule, we simplify
18( x−4 ) ( x8 )= 18( x−4 +8 )=18 ( x4 )
Therefore,
( 6 x8 ) ( 3 x−4 ) = 18( x4 )
b)
3 a−5
27 a−7 = a−5
9 a−7
= 1
9 a(−5− (−7 ))
= 1
9 a(−5 +7)

CALCULUS 7
= 1
9 a2
= ( 1
3 a )
2
Question 7
log x
3 x +1
Step 1: apply logarithm rule: logc ( a
b ) = logc ( a ) -logc ( b )
log ( x
3 x +1 ) = log10 ( x )-log10 ( 3 x+1 )
=log10 ( x )-log10 ( 3 x+1 )
Question 8
a)
Q(t) = 410e0.0035t
At t=0;
Q(t) = 410 e0.0035(0)= 410
b)
At t=50;
Q(t) = 410 e0.0035(50) = 488.411
Question 9

CALCULUS 8
y=ex x2lnx
Let f= ex , g=x2 ln (x)
Apply product rule: (f ∙ g)’ = f' ∙g +f∙g
d
dx (ex ) x2 lnx + d
dx ( x2 lnx ) ex ………………………………………………… Equation (1)
But
d
dx (ex ) = ex
d
dx (ex ) x2 lnx= ¿) x2 lnx …………………………………………………...Equation (2)
For d
dx ( x2 lnx ) ex ,
Apply exponent rule: ab = ebln(a)
x2 ln (x) = e2 ln ( x ) ln (x)
d
dx ( x2 lnx ) ex = d
dx (e2 ln ( x ) ln (x) )
Then, apply chain rule to differentiate ( x2 lnx ) ex
Let x= eu and d
dx ( 2 ln ( x ) ln ( x) )
d
du (eu ¿ = eu
And
d
dx ( 2 ln ( x ) ln (x) )= 2( 1
x ln ( x ) + 1
x ln ( x ) )
Substitute u = 2ln(x)ln(x)
e2 ln ( x ) ln (x) * 2( 1
x ln ( x )+ 1
x ln ( x ) )e x ……. …………………………………Equation (3)

CALCULUS 9
Adding equation (2) and (3) gives;
¿) x2 lnx+e2 ln ( x ) ln (x) * 2( 1
x ln ( x )+ 1
x ln ( x ) )e x
Therefore,
d
dx (ex x2 lnx )= ¿) x2 lnx+e2 ln ( x ) ln (x) * 2( 1
x ln ( x )+ 1
x ln ( x ) )e x
Question 10
∫ ( 25 x4 +4 x +3 )dx = 25∫ x4dx +4∫ xdx +3∫ 1dx
= 25[ x4 +1
4+1 ]+ 4[ x1+1
1+1 ] + 3 [ x0 +1
0+1 ]+C
=25 [ x5
5 ]+ 4 [ x2
2 ] + 3[ x
1 ]+C
= 5 x5+2x2+3x +C
Question 11
P=-0.01 x2 – 0.2x+10
Calculating the Equilibrium price when x=0
dP
dx = -0.02x-0.2
x=-10
Demand when x=-10
P=-0.01(−10)2 – 0.2(-10)+10 = $11
Demand when x=2
P=-0.01(2)2 – 0.2(2)+10 = $9.56
Consumer surplus = 11-9.56 = $1.44
Question 12

CALCULUS 10
∫t (t +2)−6dt
Step 1 : Simplify (t +2)−6
(t +2)−6 = t
(t +2)6
Thus
∫t (t +2)−6dt = ∫ t
( t+2)6 dt
Step 2: use substitution method of integration
Let u=t+2
t=u-2
Substitute t+2 with u
∫ t
( t+2)6 dt = ∫ u−2
(u)6 du where u=t+2 and t=u-2
Step 3: expand u−2
(u)6
u−2
(u)6 = 1
u5 - 2
u6
∫ ( 1
u5 − 2
u6 ) du = ∫ 1
u5 du - ∫ 2
u6 du
= ∫u−5du - 2∫ u−6du
= −1
4 u4 - ( −2
5 u2 )
Step 3: substitute u=t+2 in −1
4 u4 - ( −2
5 u2 )
−1
4 u4 - ( −2
5 u2 ) = −1
4 (t+ 2)4 +( 2
5(t +2)2 ) +C

CALCULUS 11
Therefore,
∫t (t +2)−6dt = −1
4 (t+ 2)4 + ( 2
5(t +2)2 ) +C
References
Hayter, A. (2013). Probability and statistics for engineers and scientists. Australia: Duxbury.
Stephens, L. (2011). Statistics for engineers. New York: McGraw-Hill Companies.

CALCULUS 12