Calculus Assignment: Derivatives, Integrals, and Functions
VerifiedAdded on 2023/01/19
|6
|1304
|79
Homework Assignment
AI Summary
This document presents a comprehensive solution to a calculus homework assignment. The solution includes detailed steps for finding the derivative of a function, determining intervals of increase and decrease, and evaluating limits using L'Hopital's rule. It also covers the calculation of distance from velocity using integration, solving differential equations with initial conditions, and evaluating definite integrals. Furthermore, the assignment includes problems on exponential growth, finding the inverse of a function, optimization problems involving area, and linear approximation techniques for evaluating functions. The solutions demonstrate a strong understanding of calculus concepts and problem-solving skills.
2)
g ( x ) = x−4
x2 +20
Derivative of function is:
g ' ( x )= d
dx ( x−4
x2+20 )= ( x2 +20 ) ( x−4 ) '− ( x −4 ) ( x2 +20 )
( x2+20 )2 =−x2+ 8 x+20
( x2 +20 )2
The function increases when g' ( x ) >0 and decreases when g' ( x ) <0
First let us find the location of g' ( x ) =0:
(−x2 +8 x+20)
¿ ¿
These are the locations of local maxima and minima.
local minima: x=−2
local maxima: x=10
Thus, the function decreases in the interval ( −∞ ,−2] then increases in the interval (-2,10] and
further decreases in the interval (-10,∞)
3)
li mx →1+ ¿ x
1
x2−1 ¿
Exponent rule: ax=eln ( ax ) =ex· ln ( a )
therefore, x
1
x2−1 =e
1
x2−1 ln ( x )
li mx →1+ ¿ x
1
x2−1=li mx →1+¿ e
1
x 2−1
ln ( x )
¿
¿
Move limit under exponent:
¿ e
lim x→1+¿ ln ( x )
x2−1
¿
Indeterminate form, x=1 : ln ( x )
x2−1 = 0
0 , use L’Hopital’s rule:
¿ e
lim x→1+¿ d
dx
ln (x )
x2−1
=eli m x →1+¿ 1
2 x
=e
1
2¿
¿
g ( x ) = x−4
x2 +20
Derivative of function is:
g ' ( x )= d
dx ( x−4
x2+20 )= ( x2 +20 ) ( x−4 ) '− ( x −4 ) ( x2 +20 )
( x2+20 )2 =−x2+ 8 x+20
( x2 +20 )2
The function increases when g' ( x ) >0 and decreases when g' ( x ) <0
First let us find the location of g' ( x ) =0:
(−x2 +8 x+20)
¿ ¿
These are the locations of local maxima and minima.
local minima: x=−2
local maxima: x=10
Thus, the function decreases in the interval ( −∞ ,−2] then increases in the interval (-2,10] and
further decreases in the interval (-10,∞)
3)
li mx →1+ ¿ x
1
x2−1 ¿
Exponent rule: ax=eln ( ax ) =ex· ln ( a )
therefore, x
1
x2−1 =e
1
x2−1 ln ( x )
li mx →1+ ¿ x
1
x2−1=li mx →1+¿ e
1
x 2−1
ln ( x )
¿
¿
Move limit under exponent:
¿ e
lim x→1+¿ ln ( x )
x2−1
¿
Indeterminate form, x=1 : ln ( x )
x2−1 = 0
0 , use L’Hopital’s rule:
¿ e
lim x→1+¿ d
dx
ln (x )
x2−1
=eli m x →1+¿ 1
2 x
=e
1
2¿
¿
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
4
velocity of the object is given as:
v=5 t2 ft
s
Distance can be calculated as:
d= ∫ v · dt
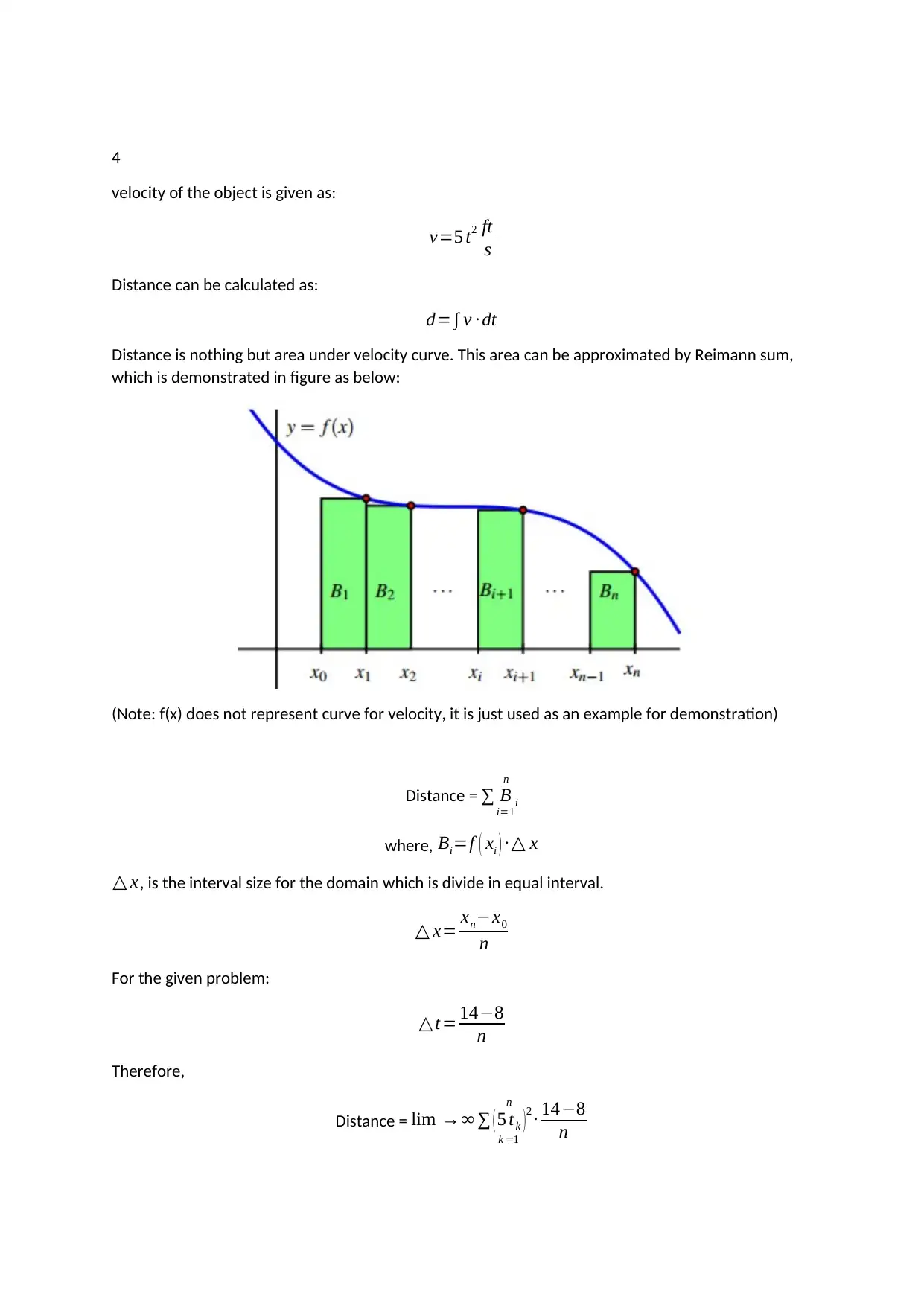
Distance is nothing but area under velocity curve. This area can be approximated by Reimann sum,
which is demonstrated in figure as below:
(Note: f(x) does not represent curve for velocity, it is just used as an example for demonstration)
Distance = ∑ B
i=1
n
i
where, Bi=f ( xi ) · △ x
△ x, is the interval size for the domain which is divide in equal interval.
△ x= xn−x0
n
For the given problem:
△ t= 14−8
n
Therefore,
Distance = lim →∞ ∑ ( 5 tk )
k =1
n 2 · 14−8
n
velocity of the object is given as:
v=5 t2 ft
s
Distance can be calculated as:
d= ∫ v · dt
Distance is nothing but area under velocity curve. This area can be approximated by Reimann sum,
which is demonstrated in figure as below:
(Note: f(x) does not represent curve for velocity, it is just used as an example for demonstration)
Distance = ∑ B
i=1
n
i
where, Bi=f ( xi ) · △ x
△ x, is the interval size for the domain which is divide in equal interval.
△ x= xn−x0
n
For the given problem:
△ t= 14−8
n
Therefore,
Distance = lim →∞ ∑ ( 5 tk )
k =1
n 2 · 14−8
n

f ( tk ), is evaluated at the right end point of each sub interval.
1)
g ' ' ( x ) =5 sin ( x ) −3 ex +2
Integrating w.r.t x:
g ' ( x )=−5 cos ( x )−3 e x+2 x+ C1
Using condition: g ' ( 0 ) =50:
g' ( 0 ) =50=−5 cos ( 0 ) −3 e0+2 (0)+ C1
⇒C1=58
Integrating g ' ( x ) w.r.t x:
g ( x ) =−5 sin ( x ) −3 ex +x2+58 x +C2
Using condition: g ( 0 )=20:
g ( 0 ) =20=−5 sin ( 0 ) −3 e0+02+58(0)+C2
⇒ C2=23
Therefore,
g ( x )=−5 sin ( x )−3 ex + x2+58 x +23
2)
I =∫
2
8
2 f ( x )+10 dx
Let us simplify:
I =∫
2
8
2 f ( x ) dx+∫
2
8
10 dx
Simplify using properties of integral:
I =2 · (∫
0
8
f ( x ) dx−∫
1
2
f ( x ) dx−∫
0
1
f ( x ) dx )+60
¿ 2 · ( 40− (−15 )−5 ) +60=160
1)
g ' ' ( x ) =5 sin ( x ) −3 ex +2
Integrating w.r.t x:
g ' ( x )=−5 cos ( x )−3 e x+2 x+ C1
Using condition: g ' ( 0 ) =50:
g' ( 0 ) =50=−5 cos ( 0 ) −3 e0+2 (0)+ C1
⇒C1=58
Integrating g ' ( x ) w.r.t x:
g ( x ) =−5 sin ( x ) −3 ex +x2+58 x +C2
Using condition: g ( 0 )=20:
g ( 0 ) =20=−5 sin ( 0 ) −3 e0+02+58(0)+C2
⇒ C2=23
Therefore,
g ( x )=−5 sin ( x )−3 ex + x2+58 x +23
2)
I =∫
2
8
2 f ( x )+10 dx
Let us simplify:
I =∫
2
8
2 f ( x ) dx+∫
2
8
10 dx
Simplify using properties of integral:
I =2 · (∫
0
8
f ( x ) dx−∫
1
2
f ( x ) dx−∫
0
1
f ( x ) dx )+60
¿ 2 · ( 40− (−15 )−5 ) +60=160
⊘ This is a preview!⊘
Do you want full access?
Subscribe today to unlock all pages.

Trusted by 1+ million students worldwide
3)
ln ( 5−x )+ln (5+ x )=0
Use rule: ln ( ab )=ln ( a ) +ln ( b )
therefore,
ln ( ( 5−x ) ( 5+ x ) ) =0
Use: ln ( 1 )=0,
( 5−x ) ( 5+ x ) =1
therefore,
25−x2 =1⇒ x=± √24
As log function cannot intake negative numbers, correct solution is:
x= √24
4)
Bacteria culture triples every 2 hours. Initial population is 300.
This is an exponential growth problem.
This growth can be modelled using function:
P=P0 3 ( t
2 )
P is population at any time, P0 is start population. Factor 3 ( t
2 ) ensures that growth triples every 2
hours.
Time when P = 25000
25000=300∗3
( t
2 )
3
t
2 = 25000
300 ⇒ t
2 ln ( 3 ) =ln ( 250
3 ) ⇒ t=8.052hours
1)
ln ( 5−x )+ln (5+ x )=0
Use rule: ln ( ab )=ln ( a ) +ln ( b )
therefore,
ln ( ( 5−x ) ( 5+ x ) ) =0
Use: ln ( 1 )=0,
( 5−x ) ( 5+ x ) =1
therefore,
25−x2 =1⇒ x=± √24
As log function cannot intake negative numbers, correct solution is:
x= √24
4)
Bacteria culture triples every 2 hours. Initial population is 300.
This is an exponential growth problem.
This growth can be modelled using function:
P=P0 3 ( t
2 )
P is population at any time, P0 is start population. Factor 3 ( t
2 ) ensures that growth triples every 2
hours.
Time when P = 25000
25000=300∗3
( t
2 )
3
t
2 = 25000
300 ⇒ t
2 ln ( 3 ) =ln ( 250
3 ) ⇒ t=8.052hours
1)
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
Find Inverse of:
g ( x ) =e8+ 3
√ x
i) y=e8 + 3
√x
ii) Switch x and y: x=e8+ 3
√ y
iii) Solve for y: ln ( x ) =8+ 3
√ y ⇒ y = ( ln ( x ) −8 ) 3
iv) Replace y with g−1 ( x ) : g−1 ( x )= ( ln x−8 )3
v) Verify: ( g−1 o g ) ( x )= ( ln g ( x )−8 )3
= ( ln (e8+ 3
√x )−8 )3
= ( 8+ 3
√ x−8 )3
=x, therefore
verified.
vi)
3.
Area of poster (printable) is 1500 sq. cm
Margins are 3 cm on left and right side and 5 cm on top and bottom.
Let the length of printable poster be ‘x’ cm. It’s height therefore will be 1500
x
Total area of poster with margins will be:
A= ( x+3+ 3 ) · ( 1500
x +5+5 )= ( x +6 ) · ( 1500
x + 10 )=1500+10 x+ 9000
x +60
For area to be minimum:
d
dx A=0⇒ 10− 9000
x2 =0 ⇒ x=30
Therefore, length of printable area is 30 and height is 50.
f ( x )= 3
8 √ x3+1 , 0≤ x ≤ 2
This is a decreasing function in the interval [0,2]
Let, M =f ( 0 ) = 3
8 √ 0+1 =0.375 and m=f ( 2 )= 3
8 √8+1 =0.125
As the function decreases, the inequality: m ≤ f ( x ) ≤ M holds true.
Therefore,
g ( x ) =e8+ 3
√ x
i) y=e8 + 3
√x
ii) Switch x and y: x=e8+ 3
√ y
iii) Solve for y: ln ( x ) =8+ 3
√ y ⇒ y = ( ln ( x ) −8 ) 3
iv) Replace y with g−1 ( x ) : g−1 ( x )= ( ln x−8 )3
v) Verify: ( g−1 o g ) ( x )= ( ln g ( x )−8 )3
= ( ln (e8+ 3
√x )−8 )3
= ( 8+ 3
√ x−8 )3
=x, therefore
verified.
vi)
3.
Area of poster (printable) is 1500 sq. cm
Margins are 3 cm on left and right side and 5 cm on top and bottom.
Let the length of printable poster be ‘x’ cm. It’s height therefore will be 1500
x
Total area of poster with margins will be:
A= ( x+3+ 3 ) · ( 1500
x +5+5 )= ( x +6 ) · ( 1500
x + 10 )=1500+10 x+ 9000
x +60
For area to be minimum:
d
dx A=0⇒ 10− 9000
x2 =0 ⇒ x=30
Therefore, length of printable area is 30 and height is 50.
f ( x )= 3
8 √ x3+1 , 0≤ x ≤ 2
This is a decreasing function in the interval [0,2]
Let, M =f ( 0 ) = 3
8 √ 0+1 =0.375 and m=f ( 2 )= 3
8 √8+1 =0.125
As the function decreases, the inequality: m ≤ f ( x ) ≤ M holds true.
Therefore,
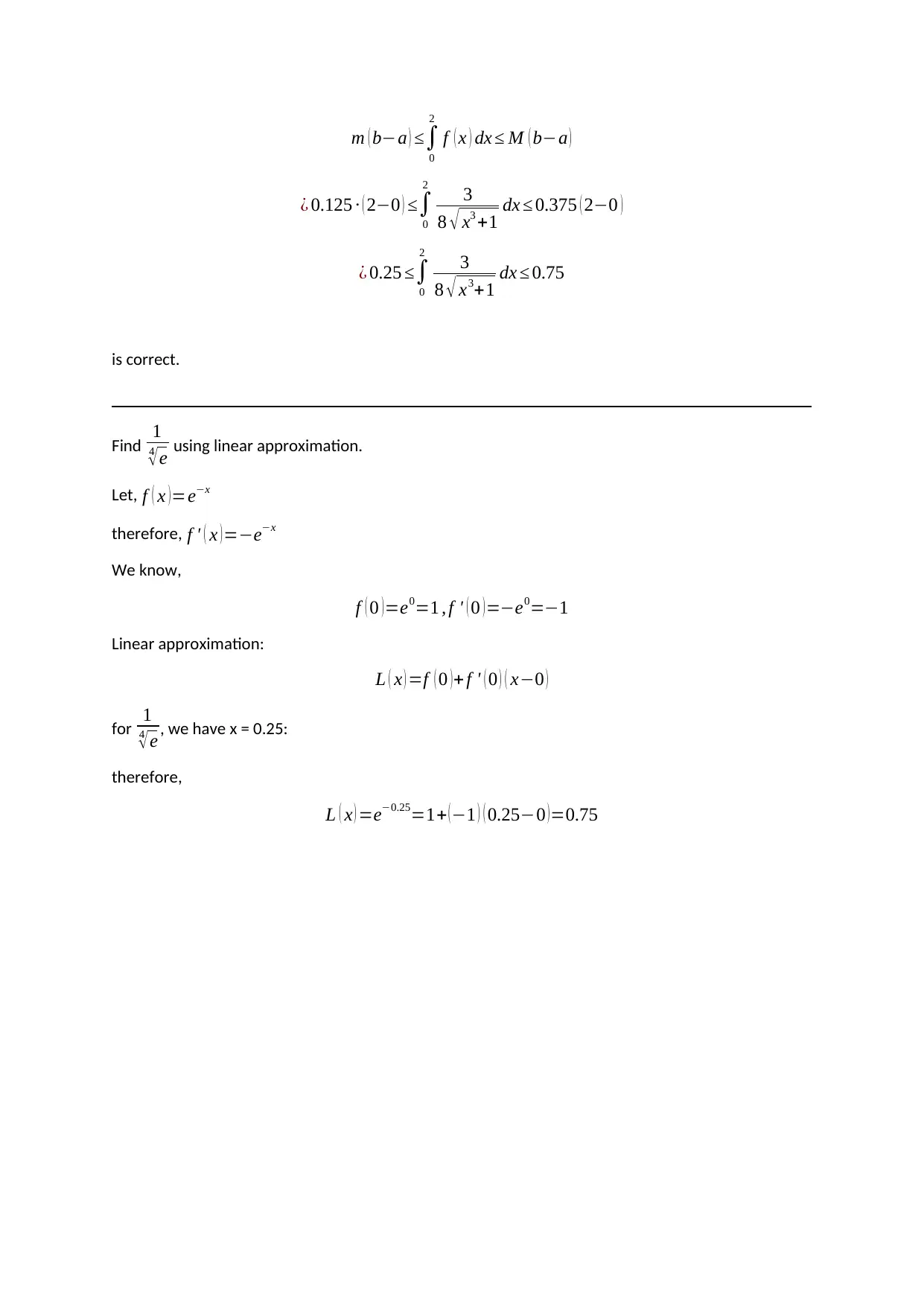
m ( b−a ) ≤∫
0
2
f ( x ) dx ≤ M ( b−a )
¿ 0.125 · ( 2−0 ) ≤∫
0
2
3
8 √ x3 +1 dx ≤ 0.375 ( 2−0 )
¿ 0.25 ≤∫
0
2
3
8 √ x3+1 dx ≤ 0.75
is correct.
Find 1
4
√e using linear approximation.
Let, f ( x )=e−x
therefore, f ' ( x )=−e−x
We know,
f ( 0 )=e0=1 , f ' ( 0 )=−e0=−1
Linear approximation:
L ( x ) =f ( 0 )+ f ' ( 0 ) ( x−0 )
for 1
4
√e , we have x = 0.25:
therefore,
L ( x ) =e−0.25=1+ (−1 ) ( 0.25−0 )=0.75
0
2
f ( x ) dx ≤ M ( b−a )
¿ 0.125 · ( 2−0 ) ≤∫
0
2
3
8 √ x3 +1 dx ≤ 0.375 ( 2−0 )
¿ 0.25 ≤∫
0
2
3
8 √ x3+1 dx ≤ 0.75
is correct.
Find 1
4
√e using linear approximation.
Let, f ( x )=e−x
therefore, f ' ( x )=−e−x
We know,
f ( 0 )=e0=1 , f ' ( 0 )=−e0=−1
Linear approximation:
L ( x ) =f ( 0 )+ f ' ( 0 ) ( x−0 )
for 1
4
√e , we have x = 0.25:
therefore,
L ( x ) =e−0.25=1+ (−1 ) ( 0.25−0 )=0.75
⊘ This is a preview!⊘
Do you want full access?
Subscribe today to unlock all pages.

Trusted by 1+ million students worldwide
1 out of 6
Related Documents
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
Copyright © 2020–2025 A2Z Services. All Rights Reserved. Developed and managed by ZUCOL.





