Maths 1 Assignment: Detailed Solutions for Calculus and Analysis
VerifiedAdded on 2022/08/08
|17
|1593
|31
Homework Assignment
AI Summary
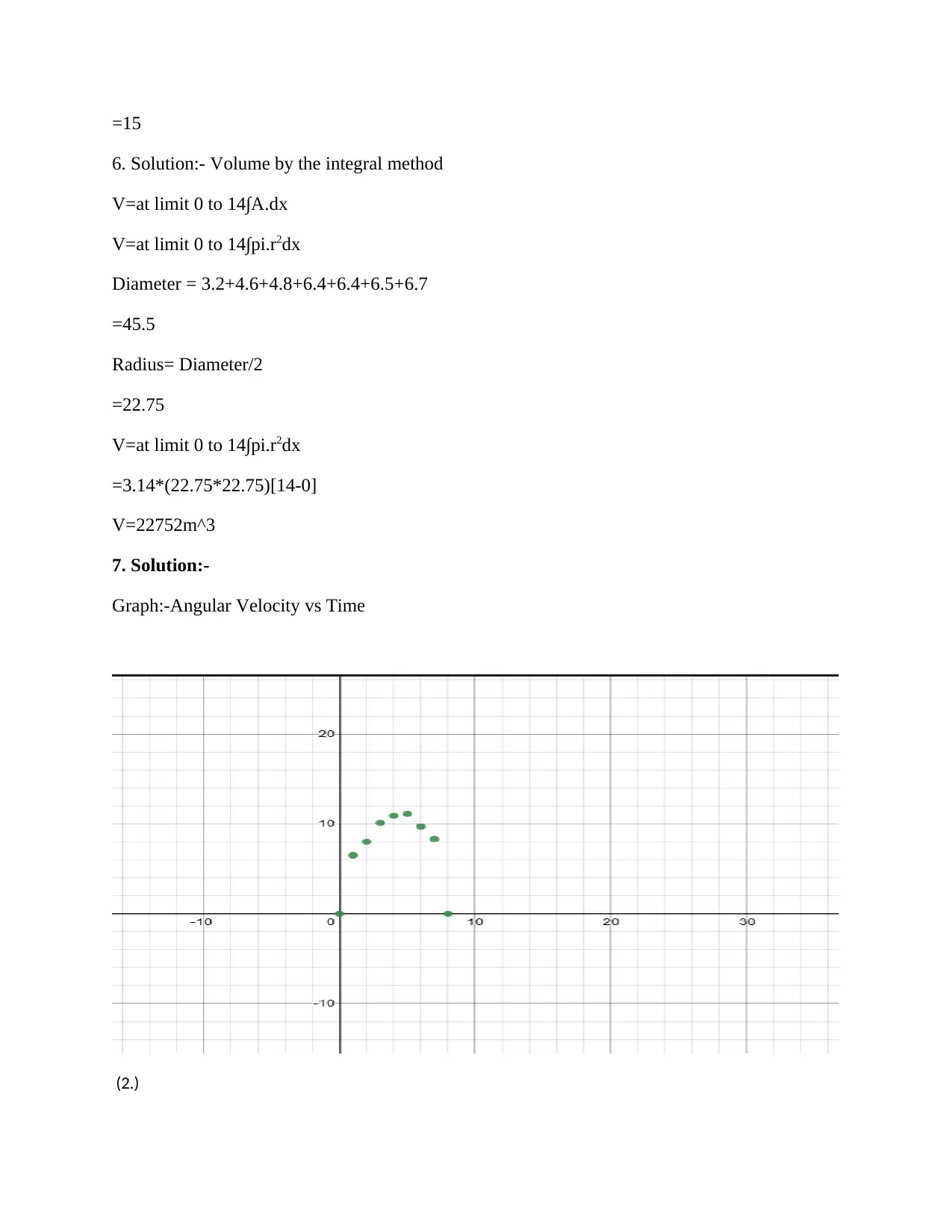
This document presents a comprehensive solution to a Maths 1 assignment, encompassing a variety of calculus and analysis problems. The solutions cover topics such as plotting graphs of different function types (algebraic, trigonometric, and exponential), including the identification of extreme points and asymptotes. It also includes the analysis of even and odd functions, along with problems related to exponential decay, trigonometric functions, and the application of integration to find average values and volumes. Furthermore, the assignment delves into the Simpson's rule for integration and numerical methods like the bisection and Newton-Raphson methods for finding roots of equations. The solutions demonstrate the application of various formulas and techniques, providing a detailed step-by-step approach to solve each problem.
1 out of 17
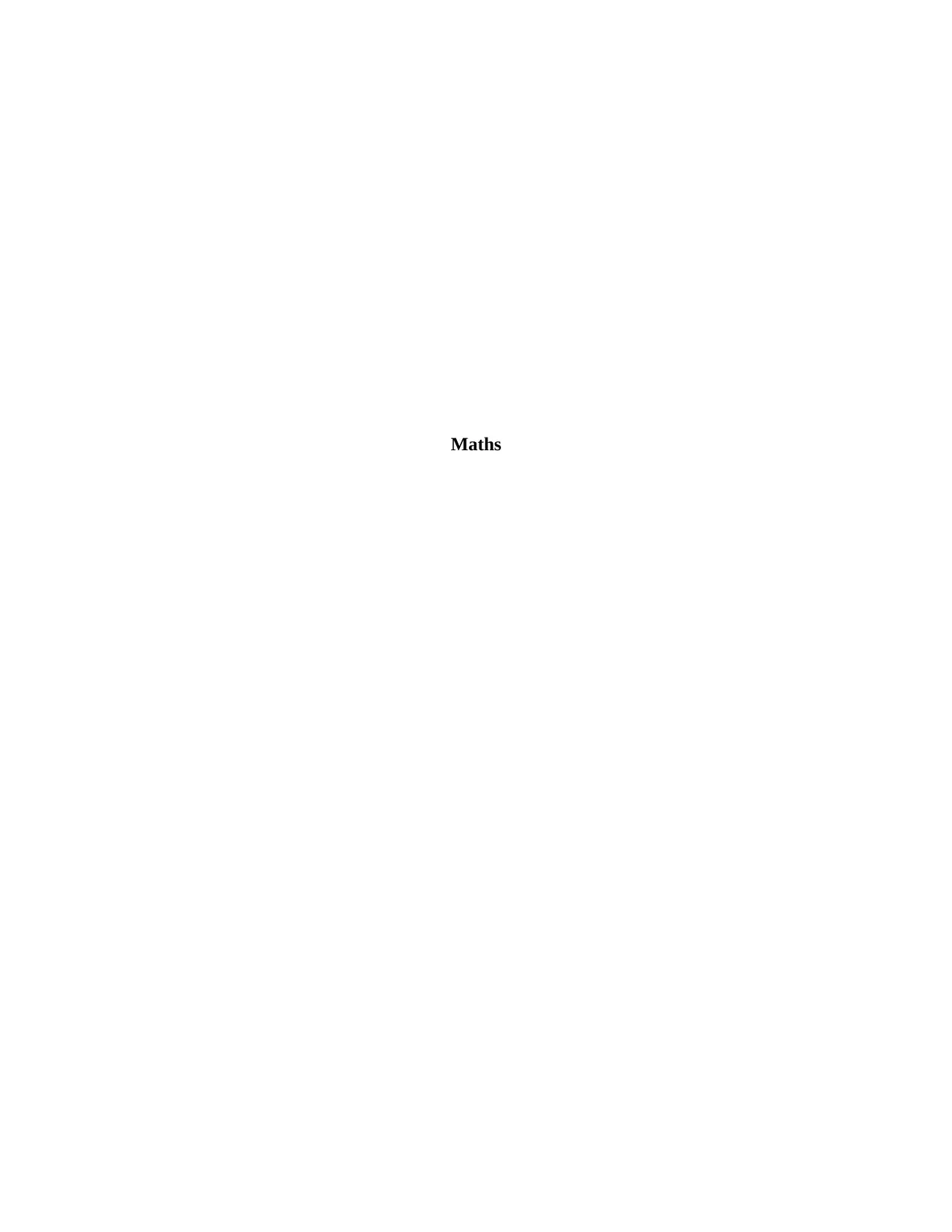
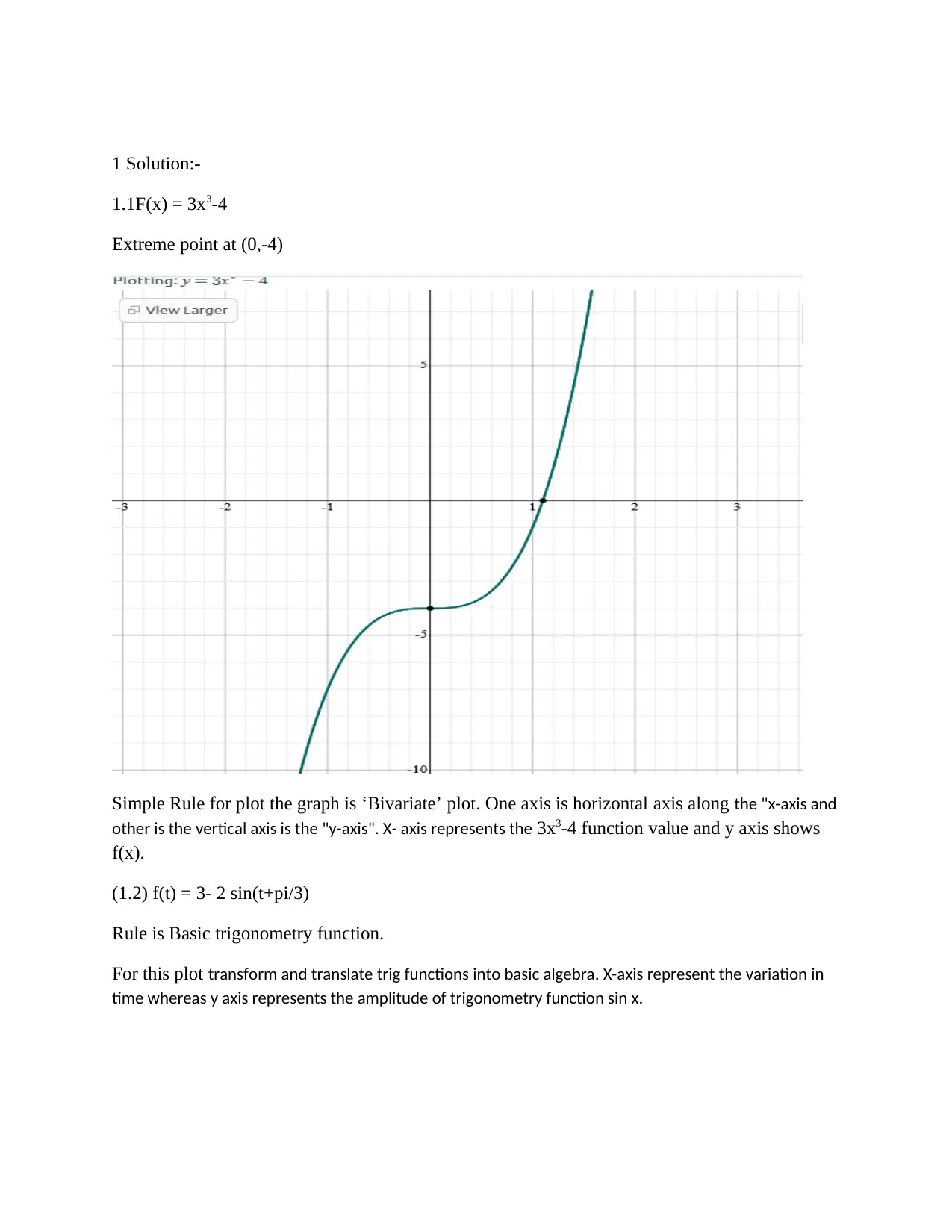
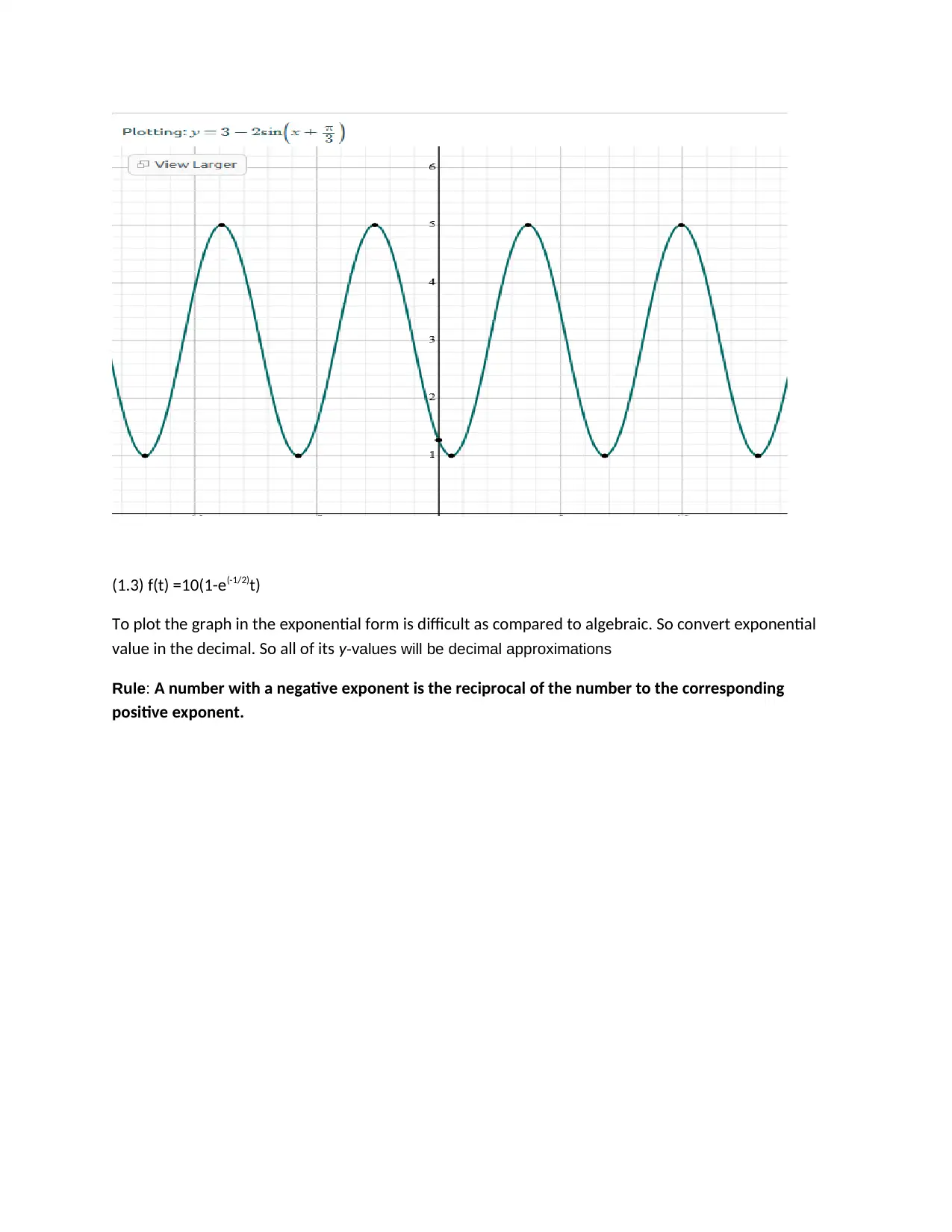

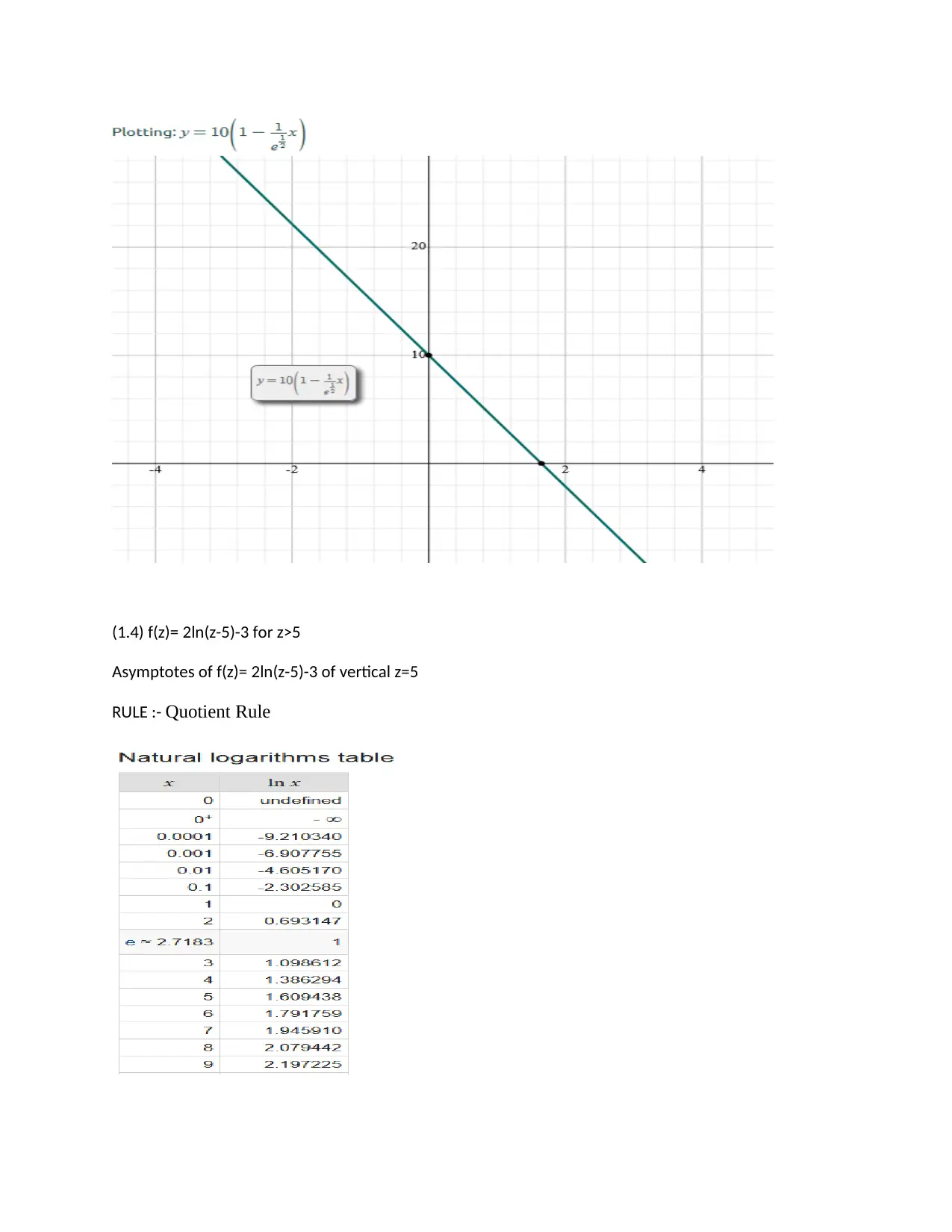
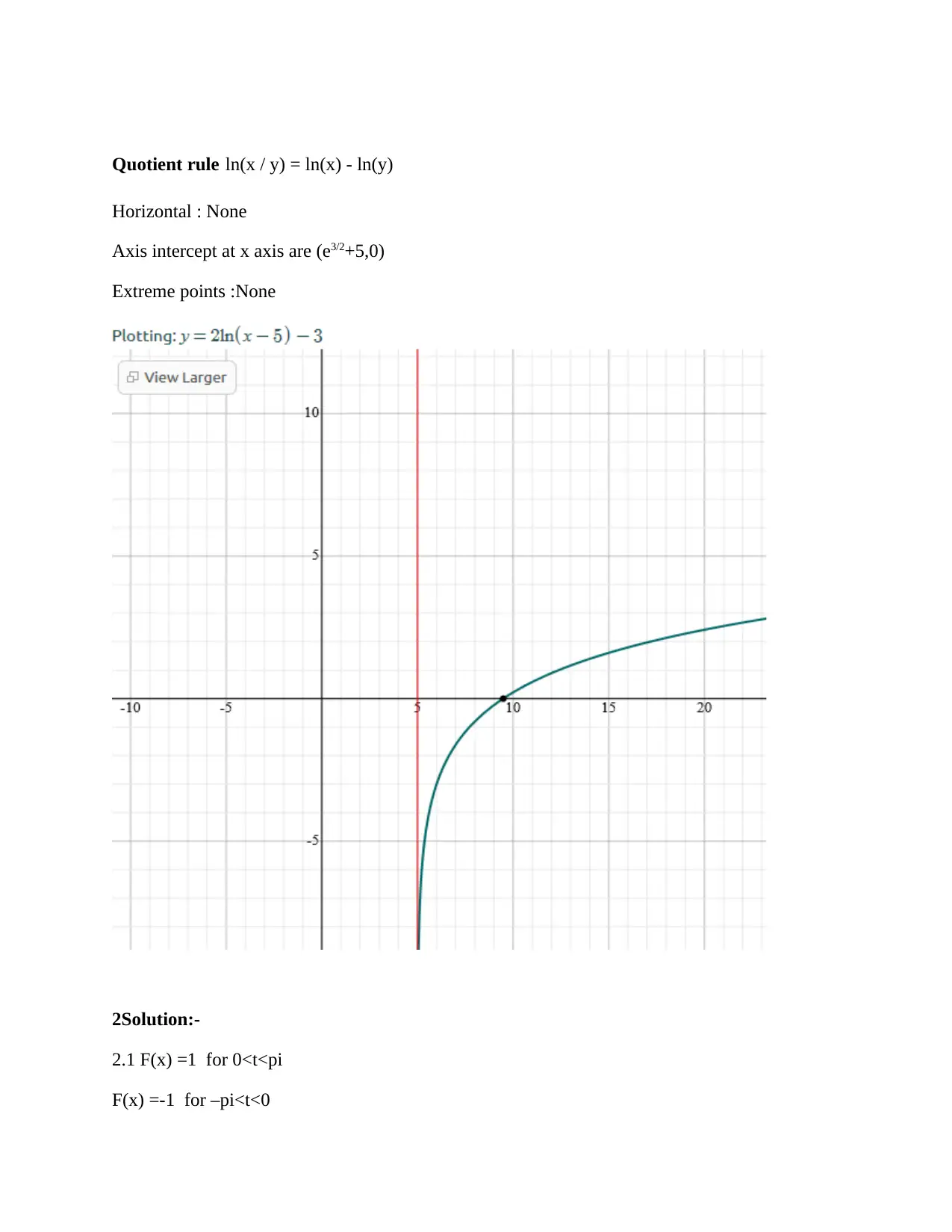
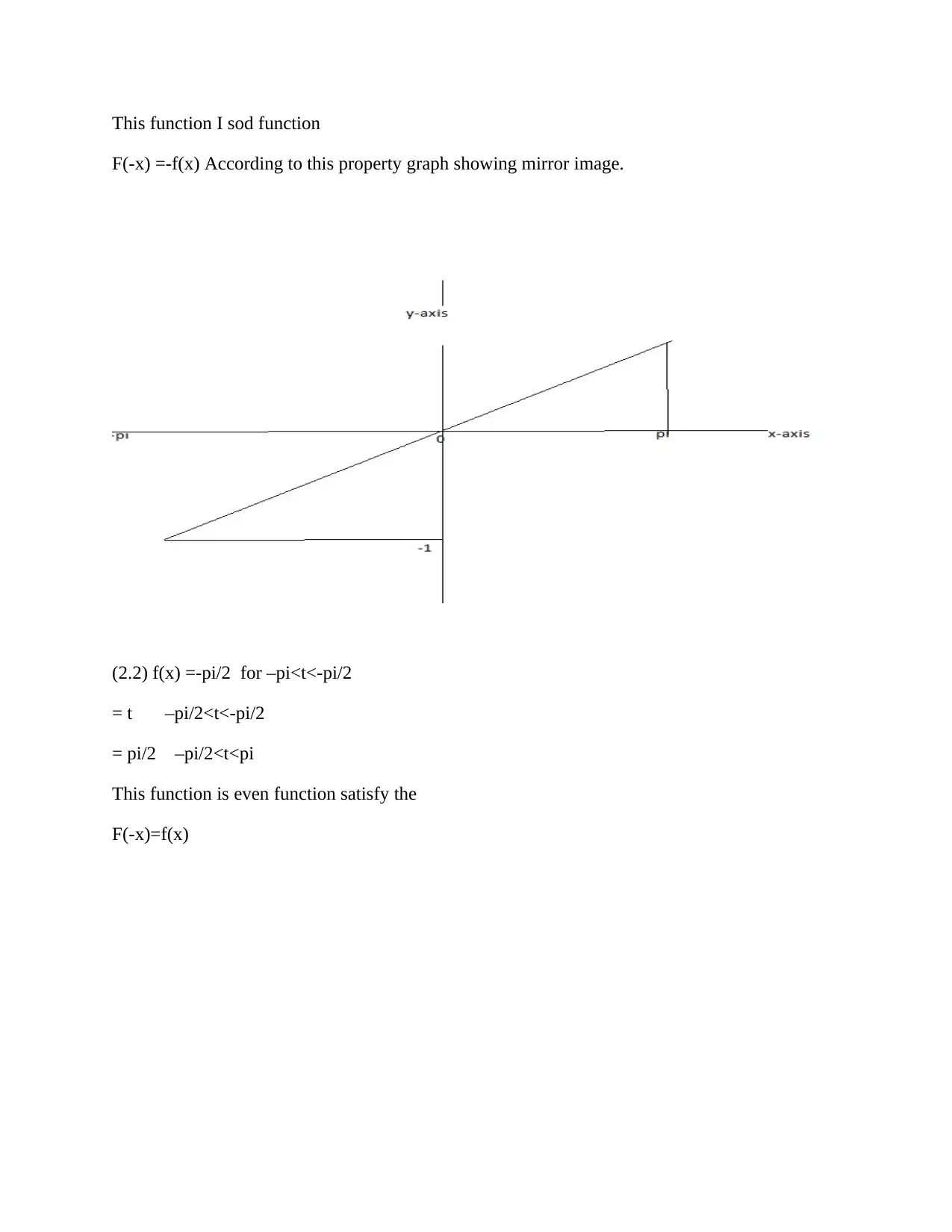
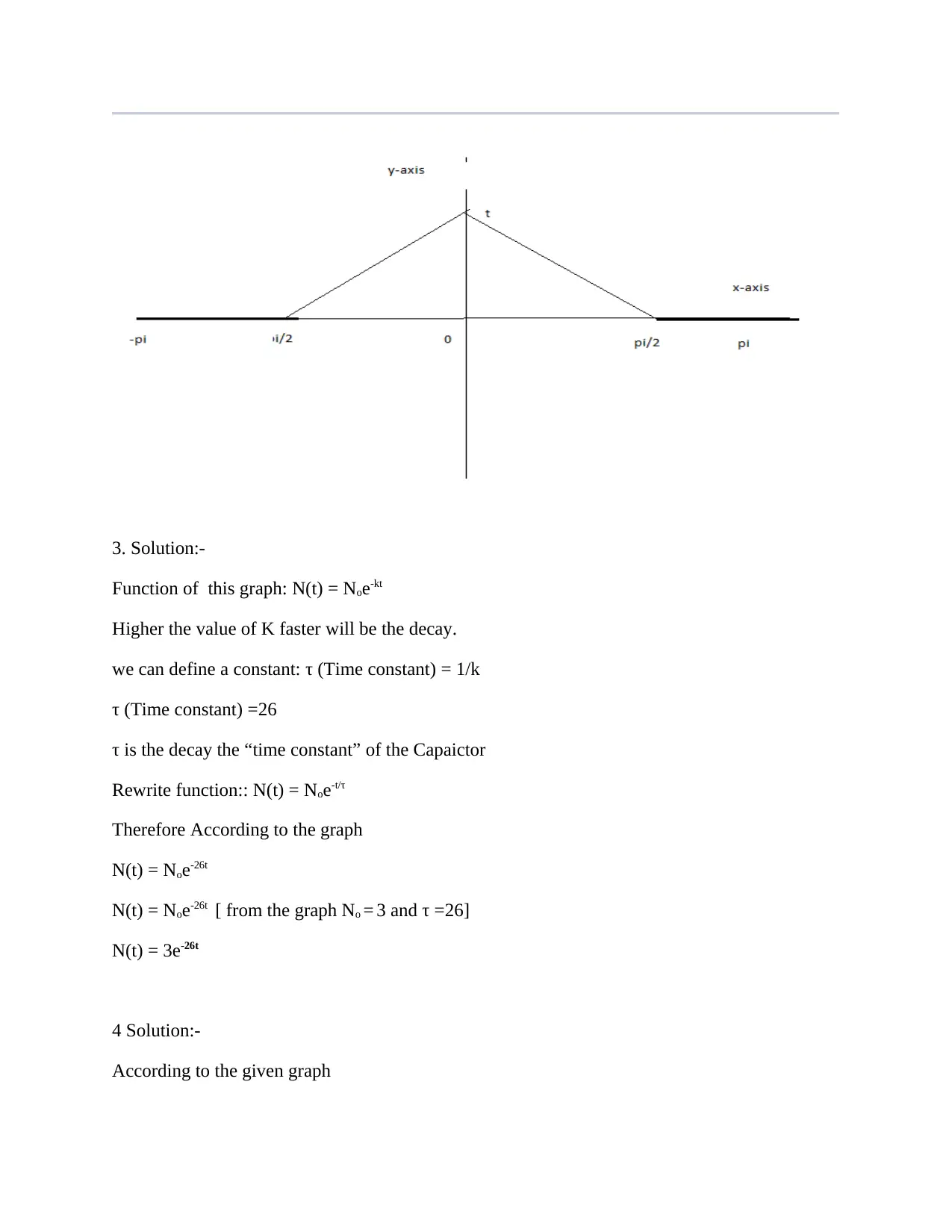

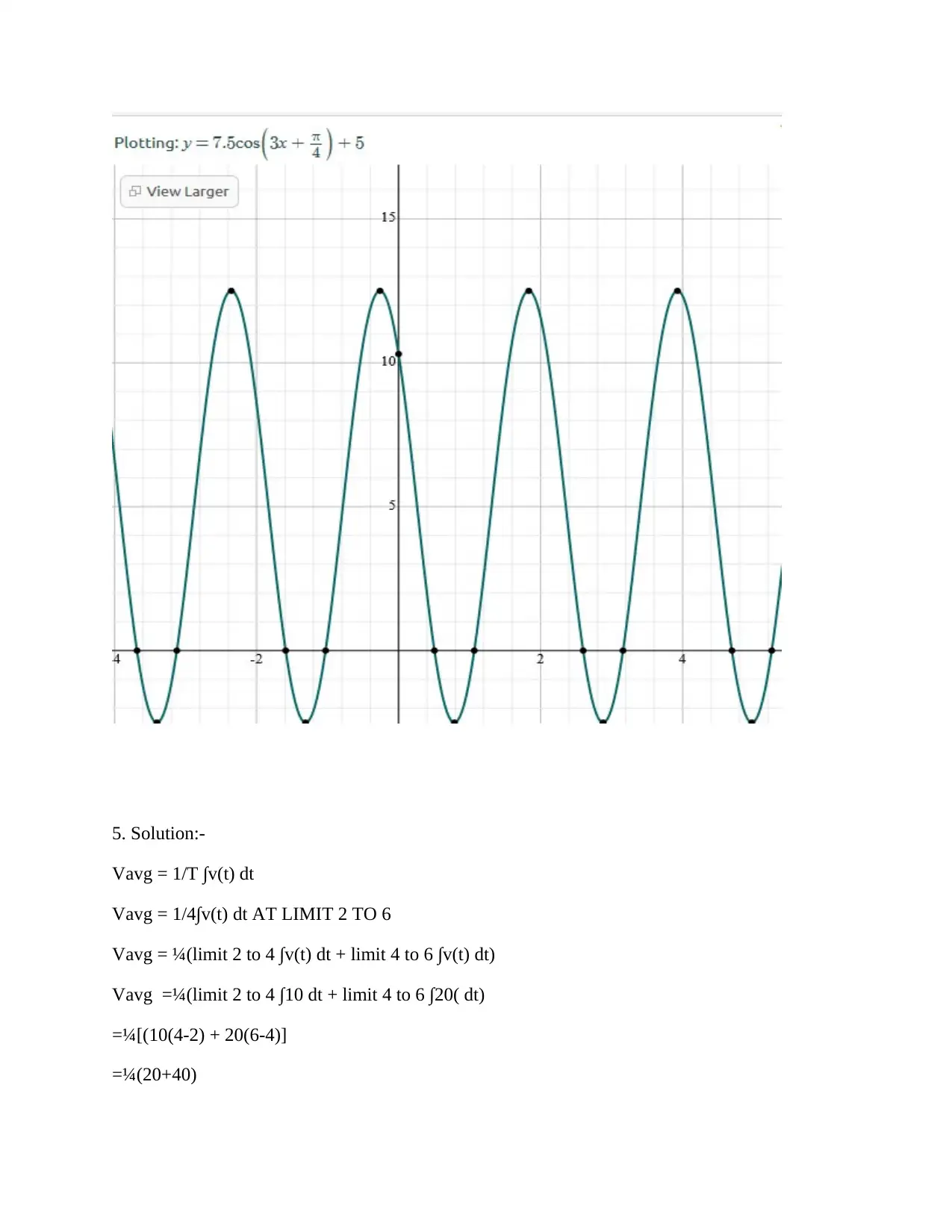







![[object Object]](/_next/static/media/star-bottom.7253800d.svg)