Calculus Assignment: Differentiation, Integration, Matrices, and More
VerifiedAdded on 2021/04/24
|11
|783
|60
Homework Assignment
AI Summary
This document presents solutions to a Calculus assignment, encompassing a range of topics. The solutions begin with differentiation problems, including the application of product, quotient, and chain rules. It proceeds to integration techniques such as substitution and integration by parts. The assignment also includes problems involving the calculation of areas under curves using definite integrals. Furthermore, it explores matrix operations, including matrix multiplication, finding the inverse of a matrix, and solving systems of linear equations using matrices. The solutions are detailed, providing step-by-step explanations for each problem, making it a valuable resource for students studying calculus.

Question 1
Differentiating
(a). f(x)=6x^2ln(4x)
f’(x)=d/dx(6x^2ln(4x))
First we remove the constant
f’(x)=6 d/dx(x^2ln(4x))
Second the product rule
(f*g)’=f’*g+f*g’
f=x^2 , g=ln(4x)
f’=d/dx(x^2)=2x , g’=d/dx(ln(4x))=1/x
Third substitute the values into the product rule
f’(x)=6 (2xln(4x)+(1/x)*x^2)
Final Result
f’(x)=6(2xln(4x)+x)
(b). f(x)= e−2 x−1
cos (2 x )
f’(x)=d/dx( e−2 x−1
cos (2 x ))
First use the quotient rule
(f/g)’=(f’*g-f*g’)/g^2
f=e^-2x-1 , g=cos(2x)
f’=2e^-2x , g’=-2sin(2x)
Second substitute the values in the quotient rule
Differentiating
(a). f(x)=6x^2ln(4x)
f’(x)=d/dx(6x^2ln(4x))
First we remove the constant
f’(x)=6 d/dx(x^2ln(4x))
Second the product rule
(f*g)’=f’*g+f*g’
f=x^2 , g=ln(4x)
f’=d/dx(x^2)=2x , g’=d/dx(ln(4x))=1/x
Third substitute the values into the product rule
f’(x)=6 (2xln(4x)+(1/x)*x^2)
Final Result
f’(x)=6(2xln(4x)+x)
(b). f(x)= e−2 x−1
cos (2 x )
f’(x)=d/dx( e−2 x−1
cos (2 x ))
First use the quotient rule
(f/g)’=(f’*g-f*g’)/g^2
f=e^-2x-1 , g=cos(2x)
f’=2e^-2x , g’=-2sin(2x)
Second substitute the values in the quotient rule
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

f’(x)= ( −2 e−2 x ) cos ( 2 x ) −(e−2 x−1)(−2sin ( 2 x ) )
( cos ( 2 x ) )
2
Final Result
f’(x)= −2e−2 x cos ( 2 x ) +(2 sin ( 2 x ) )(e−2 x−1)
( cos ( 2 x ) ) 2
(c). h(t)=√ (t3 +sin ( 2t ))
h’(t)= √(t3 +sin ( 2t ))
First use the chain rule
dfh(u)/dt=dh/du*du/dt
h= √u , u=t3+ sin ¿)
d/du( √u ¿=1/2 √u
d/dt( t3+sin ¿))=3 t2+cos ( 2t )∗2
Second substitute the values in the chain rule
h’(t)=1/2 √u *¿)
h’(t)=1/(2 √ t3 +sin (2 t))*¿)
Final Result
h’(t)= 3t2 +2 cos (2t )
2 √ t3 +sin (2 t)
Question 2
Closed box
(a). V=L*W*H
V=2x*x*h
V=2 x2 h
( cos ( 2 x ) )
2
Final Result
f’(x)= −2e−2 x cos ( 2 x ) +(2 sin ( 2 x ) )(e−2 x−1)
( cos ( 2 x ) ) 2
(c). h(t)=√ (t3 +sin ( 2t ))
h’(t)= √(t3 +sin ( 2t ))
First use the chain rule
dfh(u)/dt=dh/du*du/dt
h= √u , u=t3+ sin ¿)
d/du( √u ¿=1/2 √u
d/dt( t3+sin ¿))=3 t2+cos ( 2t )∗2
Second substitute the values in the chain rule
h’(t)=1/2 √u *¿)
h’(t)=1/(2 √ t3 +sin (2 t))*¿)
Final Result
h’(t)= 3t2 +2 cos (2t )
2 √ t3 +sin (2 t)
Question 2
Closed box
(a). V=L*W*H
V=2x*x*h
V=2 x2 h

Expression of h in terms of x if V=1000
h=1000/2 x2
(b). A=2LW+2LH+2WH
A=2(2x*x)+2(2x*h)+2(x*h)
Final result
A= 4 x2 + 4 xh+2 xh
A=4 x2 + 4 x ( 1000
2 x2 )+ 2 x ( 1000
2 x2 )
A=4 x2 + 2000
x + 1000
x
A=4 x2 + 3000
x
(c). A’= 8 x−3000 x−2
A’=8 x−3000 x−2
0= 8 x−3000 x−2
x=7.211248
A”=8+6000/x^3
A”=24
h=1000/2x^2
h=9.615
Question 3
∫(1−sin2 x
sin2 x )dx
Expansion of the expression
h=1000/2 x2
(b). A=2LW+2LH+2WH
A=2(2x*x)+2(2x*h)+2(x*h)
Final result
A= 4 x2 + 4 xh+2 xh
A=4 x2 + 4 x ( 1000
2 x2 )+ 2 x ( 1000
2 x2 )
A=4 x2 + 2000
x + 1000
x
A=4 x2 + 3000
x
(c). A’= 8 x−3000 x−2
A’=8 x−3000 x−2
0= 8 x−3000 x−2
x=7.211248
A”=8+6000/x^3
A”=24
h=1000/2x^2
h=9.615
Question 3
∫(1−sin2 x
sin2 x )dx
Expansion of the expression
⊘ This is a preview!⊘
Do you want full access?
Subscribe today to unlock all pages.

Trusted by 1+ million students worldwide

1−sin2 x
sin2 x = 1
sin2 x −1
∫( 1
sin2 x −1)dx
Using the Sum rule
=∫ 1
sin2 x dx -∫−1 dx
=∫ 1
sin2 x dx=-cos(x)
=∫−1 dx=x
Final result
=-cos(x)-x+C
Question 4
(a). ∫( sin ( x )
( 3 cos ( x ) −4 )
4 ) dx
First using substitution
U=cos(x)
=∫−( 1
( 3u−4 )4 )du
By taking out the constant
=−∫( 1
( 3 u−4 )4 )du
Applying substitution with V=3u-4
=−∫( 1
3 v 4 )du
Taking the constant out
sin2 x = 1
sin2 x −1
∫( 1
sin2 x −1)dx
Using the Sum rule
=∫ 1
sin2 x dx -∫−1 dx
=∫ 1
sin2 x dx=-cos(x)
=∫−1 dx=x
Final result
=-cos(x)-x+C
Question 4
(a). ∫( sin ( x )
( 3 cos ( x ) −4 )
4 ) dx
First using substitution
U=cos(x)
=∫−( 1
( 3u−4 )4 )du
By taking out the constant
=−∫( 1
( 3 u−4 )4 )du
Applying substitution with V=3u-4
=−∫( 1
3 v 4 )du
Taking the constant out
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

=−1
3 ∫( 1
v 4 )du
=−1
3 ∫( v−4 )du
Applying the power rule
=
−1
3 ∗v−3
−3
Substituting back all the values
V=3u-4 and u=cos(x)
=
−1
3 ∗(3 cos ( x )−4)−3
−3
Final result
= 1
9 ( 3 cos ( x )−4 )3 +C
(b). ∫
0
1
2 x3 e2 x4
dx
Taking the constant out
=2.∫
0
1
x3 e2 x4
dx
Making u=2x^4 and substituting
=2.∫
0
1
eu
8 du
= 2∗1
8 ∗∫
0
1
eu du
=∫
0
1
1
4 e2 x4
3 ∫( 1
v 4 )du
=−1
3 ∫( v−4 )du
Applying the power rule
=
−1
3 ∗v−3
−3
Substituting back all the values
V=3u-4 and u=cos(x)
=
−1
3 ∗(3 cos ( x )−4)−3
−3
Final result
= 1
9 ( 3 cos ( x )−4 )3 +C
(b). ∫
0
1
2 x3 e2 x4
dx
Taking the constant out
=2.∫
0
1
x3 e2 x4
dx
Making u=2x^4 and substituting
=2.∫
0
1
eu
8 du
= 2∗1
8 ∗∫
0
1
eu du
=∫
0
1
1
4 e2 x4

We end up with
( 1
4 e2 x4
¿1−( 1
4 e2 x4
) 0
e2
4 − 1
4 =1.59726
Final Answer
1.597
Question 5
Using integration by parts
∫ ( x +3 )2 sin ( x ) dx
Let u= ( x +3 )2 and V=sin(x)
V’=-cos(x) , u’=2(x+3)
Hence
=( x +3 ) 2 ¿
Further integrating the second part
∫−2 ( x +3 ) cos ( x)dx =−2 ¿
Hence
( x +3 ) 2 ¿
Simplifying
=−x2 cos ( x ) −6 xcos ( x ) +2 xsin ( x )−7 cos ( x ) +6 sin (x)
Final result
=−x2 cos ( x ) −6 xcos ( x ) +2 xsin ( x )−7 cos ( x ) +6 sin (x)+C
Question 6
( 1
4 e2 x4
¿1−( 1
4 e2 x4
) 0
e2
4 − 1
4 =1.59726
Final Answer
1.597
Question 5
Using integration by parts
∫ ( x +3 )2 sin ( x ) dx
Let u= ( x +3 )2 and V=sin(x)
V’=-cos(x) , u’=2(x+3)
Hence
=( x +3 ) 2 ¿
Further integrating the second part
∫−2 ( x +3 ) cos ( x)dx =−2 ¿
Hence
( x +3 ) 2 ¿
Simplifying
=−x2 cos ( x ) −6 xcos ( x ) +2 xsin ( x )−7 cos ( x ) +6 sin (x)
Final result
=−x2 cos ( x ) −6 xcos ( x ) +2 xsin ( x )−7 cos ( x ) +6 sin (x)+C
Question 6
⊘ This is a preview!⊘
Do you want full access?
Subscribe today to unlock all pages.

Trusted by 1+ million students worldwide

Area under a curve
(a). The value of f(x) for both x’s
f(x)= ( 7 π
5 +1 )sin ( 5∗7 π
5 )
f(x)=2.021
f(x)= ( 8 π
5 +1 ) sin ( 5∗8 π
5 )
f(x)=2.56
Hence, the region has positive value meaning it is above the x-axis
(b) Upper limit ( ¿-Lower limit (¿
Where the limits are the given values for upper and lower bounds
(c). ∫
7 π
5
8 π
5
( x +1 ) sin ( 5 x ) dx
Integration by part
∫ ( x +1 ) sin ( 5 x ) dx ¿ ¿
Where
U=(x+1), V=sin (5x)
U’=1, V’=-1/5cos(5x)
Hence
= 1
5 ( x +1 ) cos ( 5 x )−∫ −1
5 cos(5 x) dx
Integrating the second part
(a). The value of f(x) for both x’s
f(x)= ( 7 π
5 +1 )sin ( 5∗7 π
5 )
f(x)=2.021
f(x)= ( 8 π
5 +1 ) sin ( 5∗8 π
5 )
f(x)=2.56
Hence, the region has positive value meaning it is above the x-axis
(b) Upper limit ( ¿-Lower limit (¿
Where the limits are the given values for upper and lower bounds
(c). ∫
7 π
5
8 π
5
( x +1 ) sin ( 5 x ) dx
Integration by part
∫ ( x +1 ) sin ( 5 x ) dx ¿ ¿
Where
U=(x+1), V=sin (5x)
U’=1, V’=-1/5cos(5x)
Hence
= 1
5 ( x +1 ) cos ( 5 x )−∫ −1
5 cos(5 x) dx
Integrating the second part
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

∫ −1
5 cos (5 x) dx =−1
25 sin (5 x )
Finally
= 1
5 ( x +1 ) cos ( 5 x ) + 1
25 sin (5 x)+C
After integration
¿) for 7 π
5 = 7 π +5
25
¿) for 8 π
5 = 8 π +5
25
= 8 π +5
25 −7 π +5
25
= 1
5 (−2−3 π)
Area=-2.285
Question 8
(a)
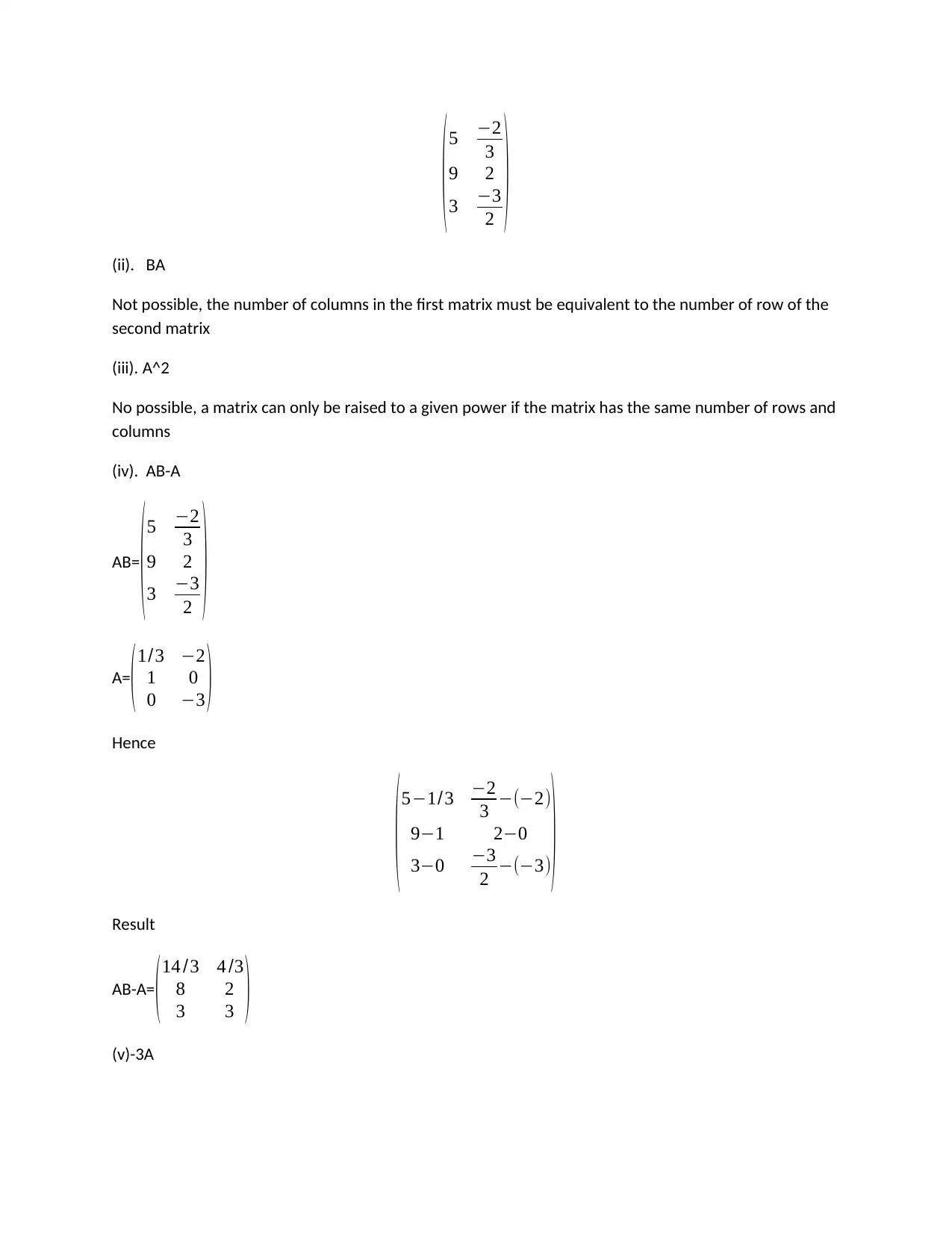
(i) AB
(1/3 −2
1 0
0 −3 )∗( 9 1
−1 1/ 2 )
( 1
3∗9+(−2∗−1) 1
3 ∗1+(−2∗1
2 )
1∗9+(0∗−1) 1∗1+( 0∗1
2 )
0∗9+(−3∗−1) 0∗1+(−3∗1
2 ) )
5 cos (5 x) dx =−1
25 sin (5 x )
Finally
= 1
5 ( x +1 ) cos ( 5 x ) + 1
25 sin (5 x)+C
After integration
¿) for 7 π
5 = 7 π +5
25
¿) for 8 π
5 = 8 π +5
25
= 8 π +5
25 −7 π +5
25
= 1
5 (−2−3 π)
Area=-2.285
Question 8
(a)
(i) AB
(1/3 −2
1 0
0 −3 )∗( 9 1
−1 1/ 2 )
( 1
3∗9+(−2∗−1) 1
3 ∗1+(−2∗1
2 )
1∗9+(0∗−1) 1∗1+( 0∗1
2 )
0∗9+(−3∗−1) 0∗1+(−3∗1
2 ) )
( 5 −2
3
9 2
3 −3
2 )
(ii). BA
Not possible, the number of columns in the first matrix must be equivalent to the number of row of the
second matrix
(iii). A^2
No possible, a matrix can only be raised to a given power if the matrix has the same number of rows and
columns
(iv). AB-A
AB=
( 5 −2
3
9 2
3 −3
2 )
A= (1/3 −2
1 0
0 −3 )
Hence
( 5−1/3 −2
3 −(−2)
9−1 2−0
3−0 −3
2 −(−3))
Result
AB-A= (14 /3 4 /3
8 2
3 3 )
(v)-3A
3
9 2
3 −3
2 )
(ii). BA
Not possible, the number of columns in the first matrix must be equivalent to the number of row of the
second matrix
(iii). A^2
No possible, a matrix can only be raised to a given power if the matrix has the same number of rows and
columns
(iv). AB-A
AB=
( 5 −2
3
9 2
3 −3
2 )
A= (1/3 −2
1 0
0 −3 )
Hence
( 5−1/3 −2
3 −(−2)
9−1 2−0
3−0 −3
2 −(−3))
Result
AB-A= (14 /3 4 /3
8 2
3 3 )
(v)-3A
⊘ This is a preview!⊘
Do you want full access?
Subscribe today to unlock all pages.

Trusted by 1+ million students worldwide
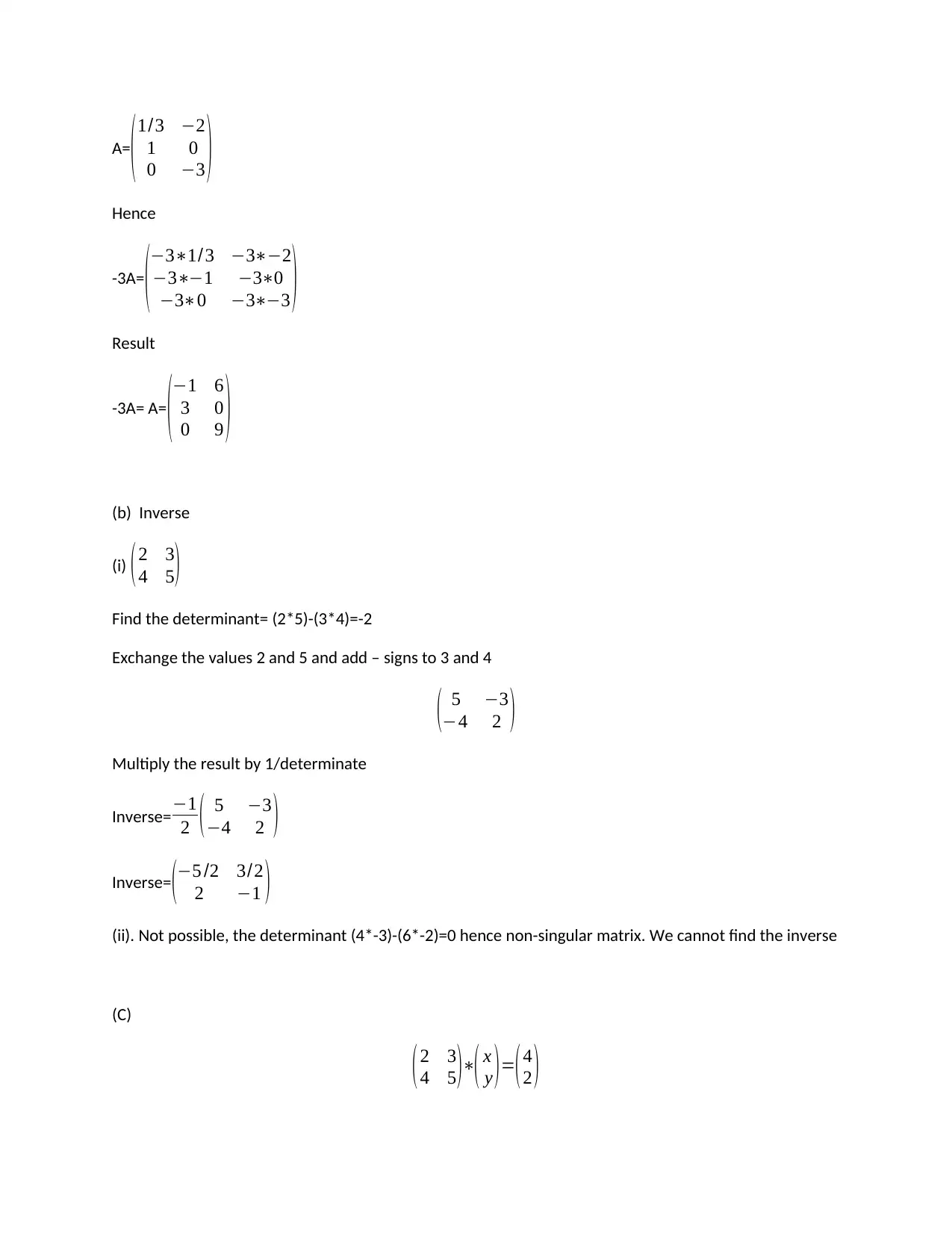
A= (1/3 −2
1 0
0 −3 )
Hence
-3A=
(
−3∗1/3 −3∗−2
−3∗−1 −3∗0
−3∗0 −3∗−3 )
Result
-3A= A=
(−1 6
3 0
0 9 )
(b) Inverse
(i) ( 2 3
4 5)
Find the determinant= (2*5)-(3*4)=-2
Exchange the values 2 and 5 and add – signs to 3 and 4
( 5 −3
−4 2 )
Multiply the result by 1/determinate
Inverse=−1
2 ( 5 −3
−4 2 )
Inverse=(−5 /2 3/2
2 −1 )
(ii). Not possible, the determinant (4*-3)-(6*-2)=0 hence non-singular matrix. We cannot find the inverse
(C)
( 2 3
4 5 )∗( x
y ) =( 4
2 )
1 0
0 −3 )
Hence
-3A=
(
−3∗1/3 −3∗−2
−3∗−1 −3∗0
−3∗0 −3∗−3 )
Result
-3A= A=
(−1 6
3 0
0 9 )
(b) Inverse
(i) ( 2 3
4 5)
Find the determinant= (2*5)-(3*4)=-2
Exchange the values 2 and 5 and add – signs to 3 and 4
( 5 −3
−4 2 )
Multiply the result by 1/determinate
Inverse=−1
2 ( 5 −3
−4 2 )
Inverse=(−5 /2 3/2
2 −1 )
(ii). Not possible, the determinant (4*-3)-(6*-2)=0 hence non-singular matrix. We cannot find the inverse
(C)
( 2 3
4 5 )∗( x
y ) =( 4
2 )
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

Hence multiply both sides by the inverse of the first matrix on the left
(−5 /2 3/2
2 −1 )( 2 3
4 5 )∗( x
y )= ( −5
2
3
2
2 −1 )∗( 4
2 )
( 1 0
0 1 )∗( x
y )= (−7
9 )
Result
X=-7
Y=9
Question 10
The form is missing from page 8-9
Question 11
This Part is supposed to be BLANK
(−5 /2 3/2
2 −1 )( 2 3
4 5 )∗( x
y )= ( −5
2
3
2
2 −1 )∗( 4
2 )
( 1 0
0 1 )∗( x
y )= (−7
9 )
Result
X=-7
Y=9
Question 10
The form is missing from page 8-9
Question 11
This Part is supposed to be BLANK
1 out of 11
Related Documents
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
Copyright © 2020–2025 A2Z Services. All Rights Reserved. Developed and managed by ZUCOL.



