University Complex Analysis Homework on Series and Functions

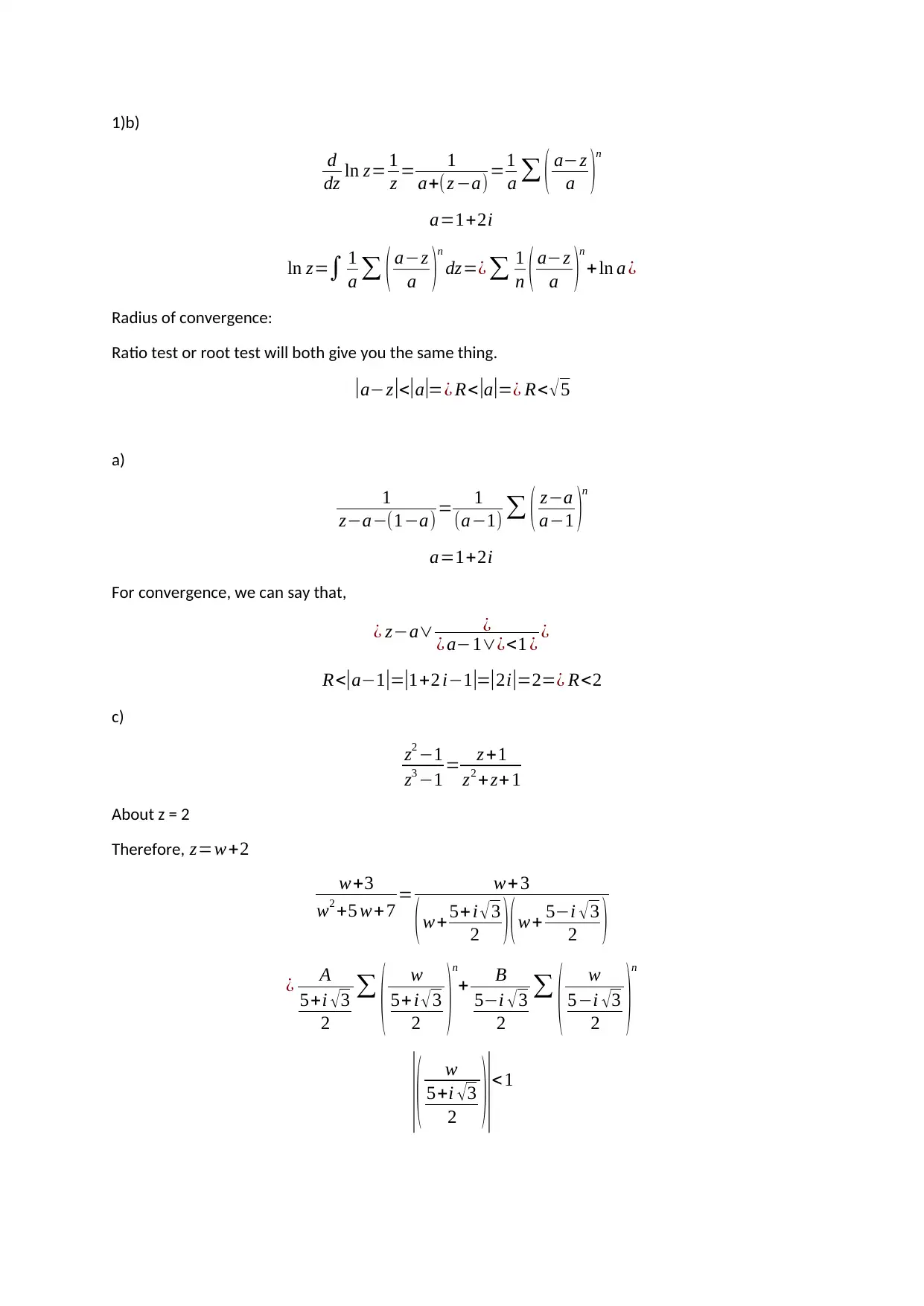
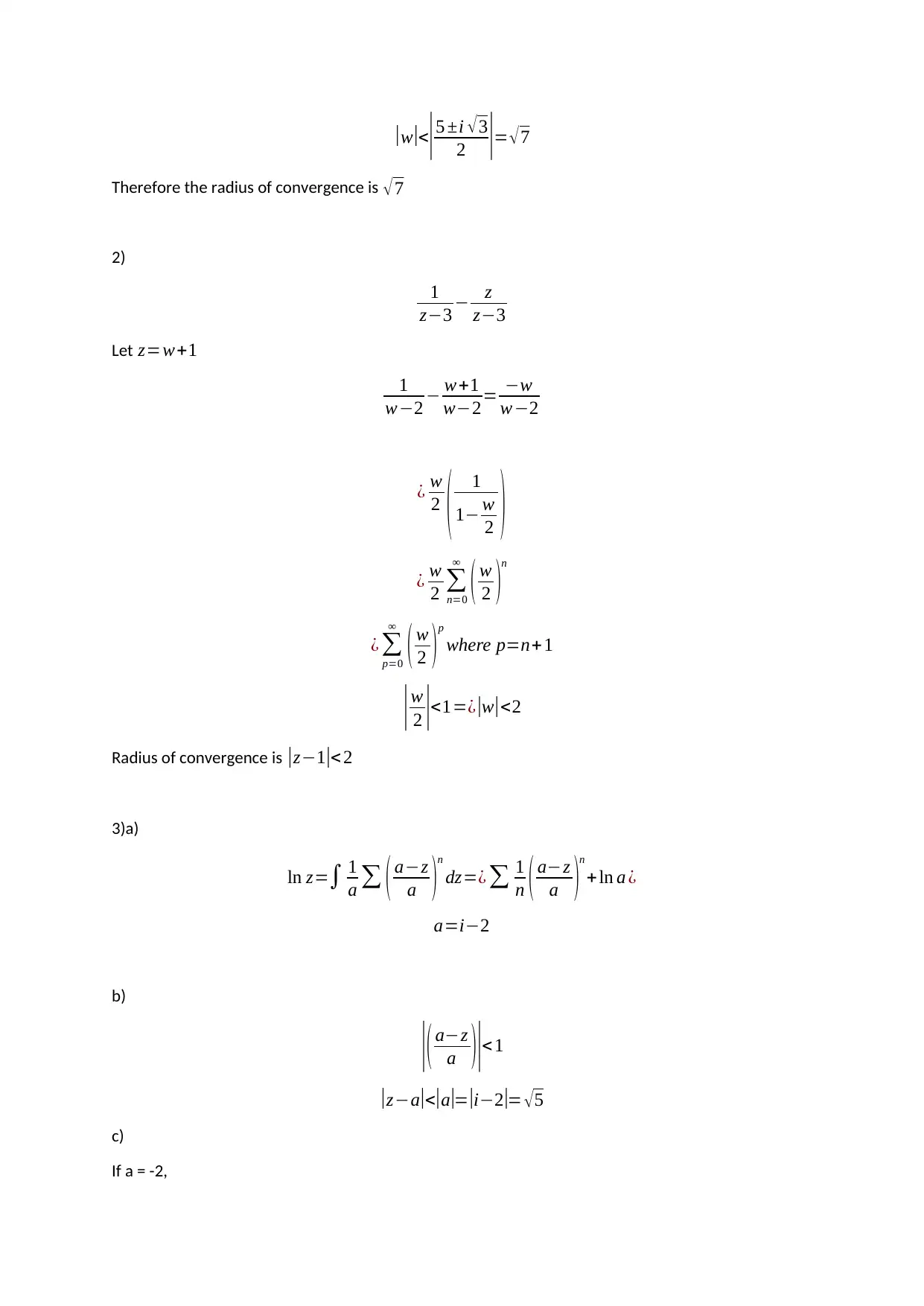
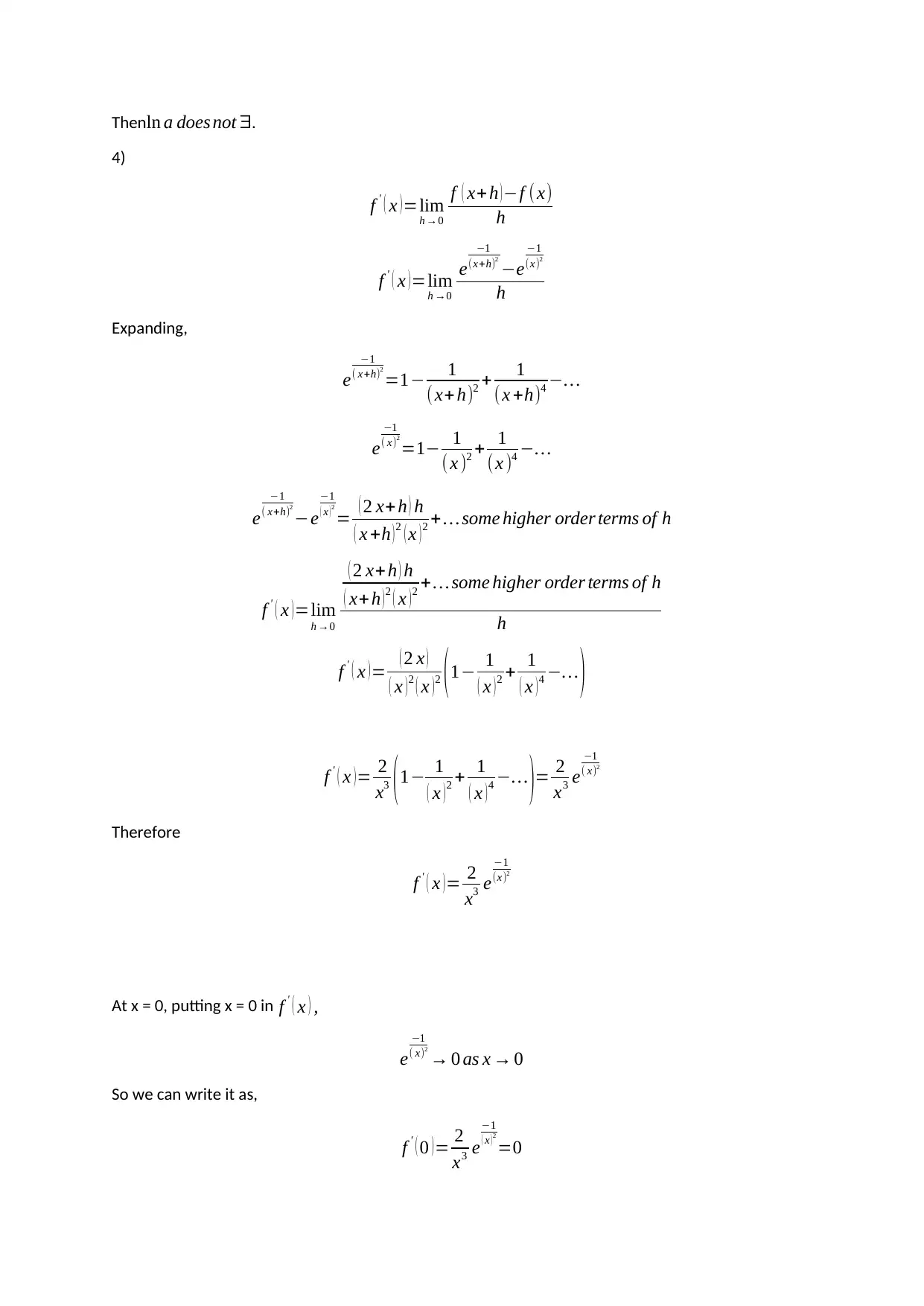
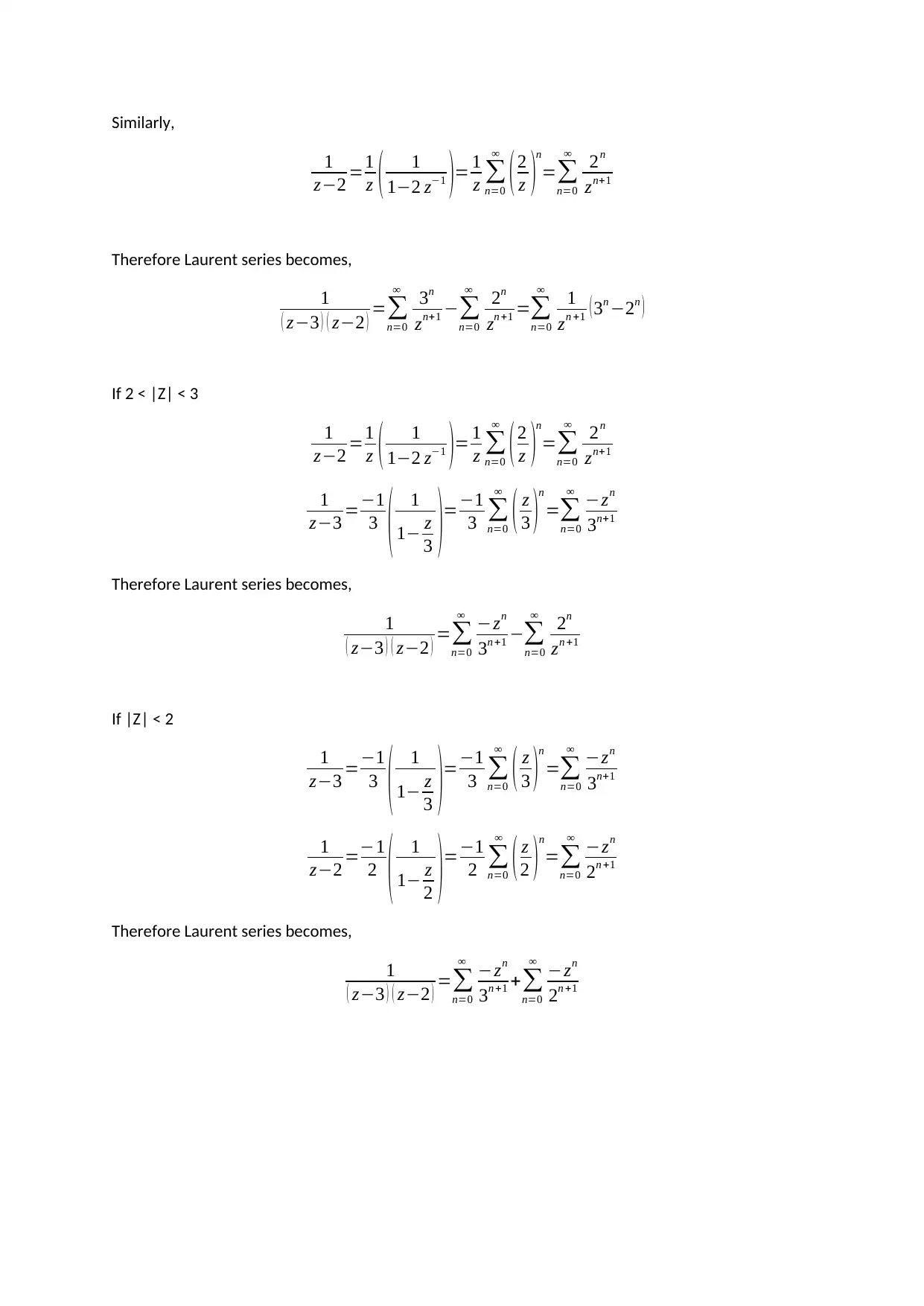
This document presents solutions to a complex analysis homework assignment. The solutions cover several key areas, including determining the radius of convergence for series, specifically using the ratio test. The assignment involves the manipulation and analysis of complex functions, including finding the derivatives and Taylor series. The solutions also explore the concepts of continuity and discontinuity, providing detailed steps and explanations. Furthermore, the assignment delves into Laurent series, with solutions provided for different regions of convergence, demonstrating the ability to expand functions in series representations based on the modulus of the complex variable. These solutions offer a comprehensive guide to understanding complex analysis concepts and solving related problems.

1)b)
d
dz ln z= 1
z = 1
a+(z −a)=1
a ∑ ( a−z
a )
n
a=1+2i
ln z=∫ 1
a ∑ ( a−z
a )
n
dz=¿ ∑ 1
n ( a−z
a )
n
+ln a ¿
Radius of convergence:
Ratio test or root test will both give you the same thing.
|a−z|<|a|=¿ R<|a|=¿ R< √ 5
a)
1
z−a−(1−a)= 1
(a−1) ∑ ( z−a
a−1 )n
a=1+2i
For convergence, we can say that,
¿ z−a∨ ¿
¿ a−1∨¿<1 ¿ ¿
R<|a−1|=|1+2 i−1|=|2i|=2=¿ R<2
c)
z2 −1
z3 −1 = z +1
z2 +z+ 1
About z = 2
Therefore, z=w+2
w+3
w2 +5 w+7 = w+3
( w+ 5+ i √ 3
2 )( w+ 5−i √ 3
2 )
¿ A
5+i √3
2
∑ ( w
5+ i √3
2 )n
+ B
5−i √3
2
∑ ( w
5−i √3
2 )n
|( w
5+i √3
2 )|<1

|w|<| 5 ±i √ 3
2 |= √ 7
Therefore the radius of convergence is √7
2)
1
z−3 − z
z−3
Let z=w+1
1
w−2 − w+1
w−2= −w
w−2
¿ w
2 ( 1
1− w
2 )
¿ w
2 ∑
n=0
∞
( w
2 )n
¿ ∑
p=0
∞
( w
2 )p
where p=n+1
|w
2 |<1=¿|w|<2
Radius of convergence is |z−1|<2
3)a)
ln z=∫ 1
a ∑ ( a−z
a )
n
dz=¿ ∑ 1
n ( a−z
a )
n
+ln a ¿
a=i−2
b)
|( a−z
a )|< 1
|z−a|<|a|=|i−2|= √5
c)
If a = -2,

Thenln a does not ∃.
4)
f ' ( x ) =lim
h →0
f ( x+ h ) −f (x)
h
f ' ( x ) =lim
h →0
e
−1
(x+h)2
−e
−1
(x)2
h
Expanding,
e
−1
( x+h)2
=1− 1
(x+ h)2 + 1
(x +h)4 −…
e
−1
( x)2
=1− 1
( x )2 + 1
(x )4 −…
e
−1
( x+h)2
−e
−1
( x ) 2
= ( 2 x+ h ) h
( x +h ) 2 ( x ) 2 + … some higher order terms of h
f ' ( x )=lim
h →0
( 2 x+ h ) h
( x+ h )2 ( x )2 + … some higher order terms of h
h
f ' ( x ) = ( 2 x )
( x ) 2 ( x ) 2 ( 1− 1
( x ) 2 + 1
( x ) 4 −… )
f ' ( x )= 2
x3 (1− 1
( x )2 + 1
( x )4 −… )= 2
x3 e
−1
( x)2
Therefore
f ' ( x )= 2
x3 e
−1
(x)2
At x = 0, putting x = 0 in f ' ( x ) ,
e
−1
( x)2
→ 0 as x → 0
So we can write it as,
f ' ( 0 ) = 2
x3 e
−1
( x ) 2
=0

b)
With the help of induction we can show that higher order derivatives are also zero so,
f ' ( 0 )=f ' ' ( 0 )=f '' ' ( 0 )=…=0
Now if we find the Taylor series of f ( x) at 0,
f ( 0+ h ) =f ( 0 ) +h f ' ( 0 ) + h2
2 f ' ' ( 0 ) + h3
6 f ' ' ' ( 0 ) + …
Putting the values of the derivatives we get,
f ( 0+ h )=0
c)
lim
h→ 0
f ( 0+h ) −f ( 0 ) =lim
h→ 0
e
−1
h2
As h is imaginary so h2 will give a negative value so it will become -h2
lim
h→ 0
e
1
h2
=∞ ≠ f ( 0 )
Therefore it is not continuous proved.
d)
e
−1
z2
=1+−1
z2 + 1
2 ! z4 + −1
3 ! z6 + …
¿ ∑
n=0
∞ (−1)n
n !(z2 )n =∑
n=0
∞ 1
n ! ( −1
z2 )
n
It is valid in the domain of |z| > 0
5)
1
z2 −5 z +6 = 1
( z−3 ) ( z−2 ) = 1
z−3 − 1
z−2
If |Z|> 3
1
z−3 = 1
z ( 1
1−3 z−1 )=1
z ∑
n =0
∞
( 3
z )n
=∑
n=0
∞ 3n
zn +1

Similarly,
1
z−2 =1
z ( 1
1−2 z−1 )= 1
z ∑
n=0
∞
( 2
z )
n
=∑
n=0
∞ 2n
zn+1
Therefore Laurent series becomes,
1
( z−3 ) ( z−2 ) =∑
n=0
∞ 3n
zn+1 −∑
n=0
∞ 2n
zn +1 =∑
n=0
∞ 1
zn +1 ( 3n −2n )
If 2 < |Z| < 3
1
z−2 =1
z ( 1
1−2 z−1 )= 1
z ∑
n=0
∞
(2
z )n
=∑
n=0
∞ 2n
zn+1
1
z−3 =−1
3 ( 1
1− z
3 )=−1
3 ∑
n=0
∞
( z
3 )
n
=∑
n=0
∞ −zn
3n+1
Therefore Laurent series becomes,
1
( z−3 ) ( z−2 ) =∑
n=0
∞ −zn
3n +1 −∑
n=0
∞ 2n
zn +1
If |Z| < 2
1
z−3 =−1
3 ( 1
1− z
3 )=−1
3 ∑
n=0
∞
( z
3 )n
=∑
n=0
∞ −zn
3n+1
1
z−2 =−1
2 ( 1
1− z
2 )=−1
2 ∑
n=0
∞
( z
2 )n
=∑
n=0
∞ −zn
2n +1
Therefore Laurent series becomes,
1
( z−3 ) ( z−2 ) =∑
n=0
∞ −zn
3n +1 +∑
n=0
∞ −zn
2n +1