Mathematics Assignment: Sequence, Series, Complex Numbers Solutions
VerifiedAdded on 2021/04/24
|10
|1152
|84
Homework Assignment
AI Summary
This document presents a comprehensive solution to a mathematics homework assignment. It covers a range of topics including sequences and series, where problems involving geometric progressions, recurrence systems, and determining the nth term are addressed. The solution also delves int...

Ans.1. (a) Here the first term is a= 2.6 and Common Ratio (r) =?
We have, ar= 4.68, ar^2 =8.424, ar^3= 15.1632
r=ar/a= 4.68/2.6= 1.8
r=ar^2/ar=8.424/4.68=1.8
r=ar^3/ar^2=15.1632/8.424=1.8
Therefore the First term is 2.6 and Common Ratio= 1.8
The recurrence System is ( a∗r(n−1) )=¿2.6*(1.8)^(n-1) ; n=1,…..
(b) The closed form for this sequence is 2.6*(1.8)^(n-1) ; n=1,…..
(c) Tenth Term= a*r^(10-1) = 2.6*(1.8)^(9)=515.734
(d)
Let the number be ‘n’.
a*r^(n-1) ≤ 39000
or, 2.6*(1.8)^(n-1)≤39000
or, (1.8)^(n-1)≤15000
or, (n-1)ln(1.8)≤ln(15000)
or, n-1≤16.35
or, n≤17.35
Since ‘n’ should be a natural number, so it should be n=17
Ans.2. The sequence converges to -26
Reason:
lim
n → ∞
{52∗( 0.91 )n−26 }
¿ lim
n → ∞
{52∗( 0.91 )n }−26
=0-26 =-26
Ans.3. It is an Arithmetic Progression
Here 1st Term a= 6 (From Feb 2016)
And Common Difference = 4
Number of terms = 14
Therefore, the required number of people=
12+ ∑
n =1
14
{6+ ( n−1 )∗4 }
We have, ar= 4.68, ar^2 =8.424, ar^3= 15.1632
r=ar/a= 4.68/2.6= 1.8
r=ar^2/ar=8.424/4.68=1.8
r=ar^3/ar^2=15.1632/8.424=1.8
Therefore the First term is 2.6 and Common Ratio= 1.8
The recurrence System is ( a∗r(n−1) )=¿2.6*(1.8)^(n-1) ; n=1,…..
(b) The closed form for this sequence is 2.6*(1.8)^(n-1) ; n=1,…..
(c) Tenth Term= a*r^(10-1) = 2.6*(1.8)^(9)=515.734
(d)
Let the number be ‘n’.
a*r^(n-1) ≤ 39000
or, 2.6*(1.8)^(n-1)≤39000
or, (1.8)^(n-1)≤15000
or, (n-1)ln(1.8)≤ln(15000)
or, n-1≤16.35
or, n≤17.35
Since ‘n’ should be a natural number, so it should be n=17
Ans.2. The sequence converges to -26
Reason:
lim
n → ∞
{52∗( 0.91 )n−26 }
¿ lim
n → ∞
{52∗( 0.91 )n }−26
=0-26 =-26
Ans.3. It is an Arithmetic Progression
Here 1st Term a= 6 (From Feb 2016)
And Common Difference = 4
Number of terms = 14
Therefore, the required number of people=
12+ ∑
n =1
14
{6+ ( n−1 )∗4 }
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

= 12 + ∑
n =1
14
{ 6 } + ∑
n =1
14
{ ( n−1 )∗4 }
= 12+6*14 + ∑
n =1
14
{ n∗4 } - ∑
n =1
14
{ 4 }
=12 +14*6+4*14*15/2-14*4
=460
Ans.4. ∑
n=11
38
{ n3 −3∗n2 }
= ∑
n =1
38
{n3−3∗n2 } - ∑
n =1
11
{n3−3∗n2 }
= ∑
n =1
38
{n3 } - ∑
n =1
38
{3∗n2 } - ∑
n =1
11
{n3 } + ∑
n =1
11
{3∗n2 }
=(38*39/2)^2 -3*38*39*77/6 – (11*12/2)^2 + 3*11*12*23/6
=549081-57057- 4356+ 1518
=489186
Ans.5. We have the 8th term as
14C8 *((3*a^2)^8)*(9*a^3)^(-6)
Therefore the required coefficient is
14C8*3^(-4)
Ans.8.(a) We have f(x)=( 1
4 + x)^(-1/4)
Therefore differentiating both sides w.r.t. x we get,
f (x′ )=(-1/4)( 1
4 + x)^(-5/4)
or, g(x)=(-4)f′(x)= ( 1
4 + x)^(-5/4)
Now, we are having
f(x)=√2 -√2x+ 5 √ 2
2 x^2 - 15 √ 2
2 x^3 +….
Differentiating, both sides w.r.t. x and multiplying by -4 we get,
g(x)=(-4)f′(x)= 4√2+20√2x -+90√2 x^2+…. Which is the required Taylor Series for g(x)= ( 1
4 + x)^(-5/4).
n =1
14
{ 6 } + ∑
n =1
14
{ ( n−1 )∗4 }
= 12+6*14 + ∑
n =1
14
{ n∗4 } - ∑
n =1
14
{ 4 }
=12 +14*6+4*14*15/2-14*4
=460
Ans.4. ∑
n=11
38
{ n3 −3∗n2 }
= ∑
n =1
38
{n3−3∗n2 } - ∑
n =1
11
{n3−3∗n2 }
= ∑
n =1
38
{n3 } - ∑
n =1
38
{3∗n2 } - ∑
n =1
11
{n3 } + ∑
n =1
11
{3∗n2 }
=(38*39/2)^2 -3*38*39*77/6 – (11*12/2)^2 + 3*11*12*23/6
=549081-57057- 4356+ 1518
=489186
Ans.5. We have the 8th term as
14C8 *((3*a^2)^8)*(9*a^3)^(-6)
Therefore the required coefficient is
14C8*3^(-4)
Ans.8.(a) We have f(x)=( 1
4 + x)^(-1/4)
Therefore differentiating both sides w.r.t. x we get,
f (x′ )=(-1/4)( 1
4 + x)^(-5/4)
or, g(x)=(-4)f′(x)= ( 1
4 + x)^(-5/4)
Now, we are having
f(x)=√2 -√2x+ 5 √ 2
2 x^2 - 15 √ 2
2 x^3 +….
Differentiating, both sides w.r.t. x and multiplying by -4 we get,
g(x)=(-4)f′(x)= 4√2+20√2x -+90√2 x^2+…. Which is the required Taylor Series for g(x)= ( 1
4 + x)^(-5/4).

(b)
We have f(x)=( 1
4 + x)^(-1/4)
Therefore integrating both sides w.r.t. x we get,
F(x)=(4/3)( 1
4 + x)^(3/4)
or, h(x)=F(x)=(4/3) ( 1
4 + x)^(3/4)
Now, we are having
f(x)=√2 -√2x+ 5 √ 2
2 x^2 - 15 √ 2
2 x^3 +….
Integrating, both sides w.r.t. x and multiplying by ¾ we get,
h(x)=F(x)= ¾ √2x – 3/8 √2 x^2 + 5/3 √2 x^3 – 15/8 √2 x^4 , which is the required Taylor Series for
h(x)= ( 1
4 + x)^(3/4).
Ans.9.
The required equation is:
z^2 – (3/4 +2i +3/4 -2i)z + (3/4 + 2i)(3/4-2i)=0
or, z^2 –(6/4)z + (73/16)=0
Ans.10.
z^4 = -2√3-2i
= -2(√3 + i)
=-4( √ 3
2 + i
2)
=(2i)^2 (cos(π/6)+sin(π/6))
=((2i)^2)*(e^(iπ/6))
Or, z^2 = ±(2*i)*(e^(iπ/12))
Or, z= ±(√(2*i))*(e^(iπ/24))
We have f(x)=( 1
4 + x)^(-1/4)
Therefore integrating both sides w.r.t. x we get,
F(x)=(4/3)( 1
4 + x)^(3/4)
or, h(x)=F(x)=(4/3) ( 1
4 + x)^(3/4)
Now, we are having
f(x)=√2 -√2x+ 5 √ 2
2 x^2 - 15 √ 2
2 x^3 +….
Integrating, both sides w.r.t. x and multiplying by ¾ we get,
h(x)=F(x)= ¾ √2x – 3/8 √2 x^2 + 5/3 √2 x^3 – 15/8 √2 x^4 , which is the required Taylor Series for
h(x)= ( 1
4 + x)^(3/4).
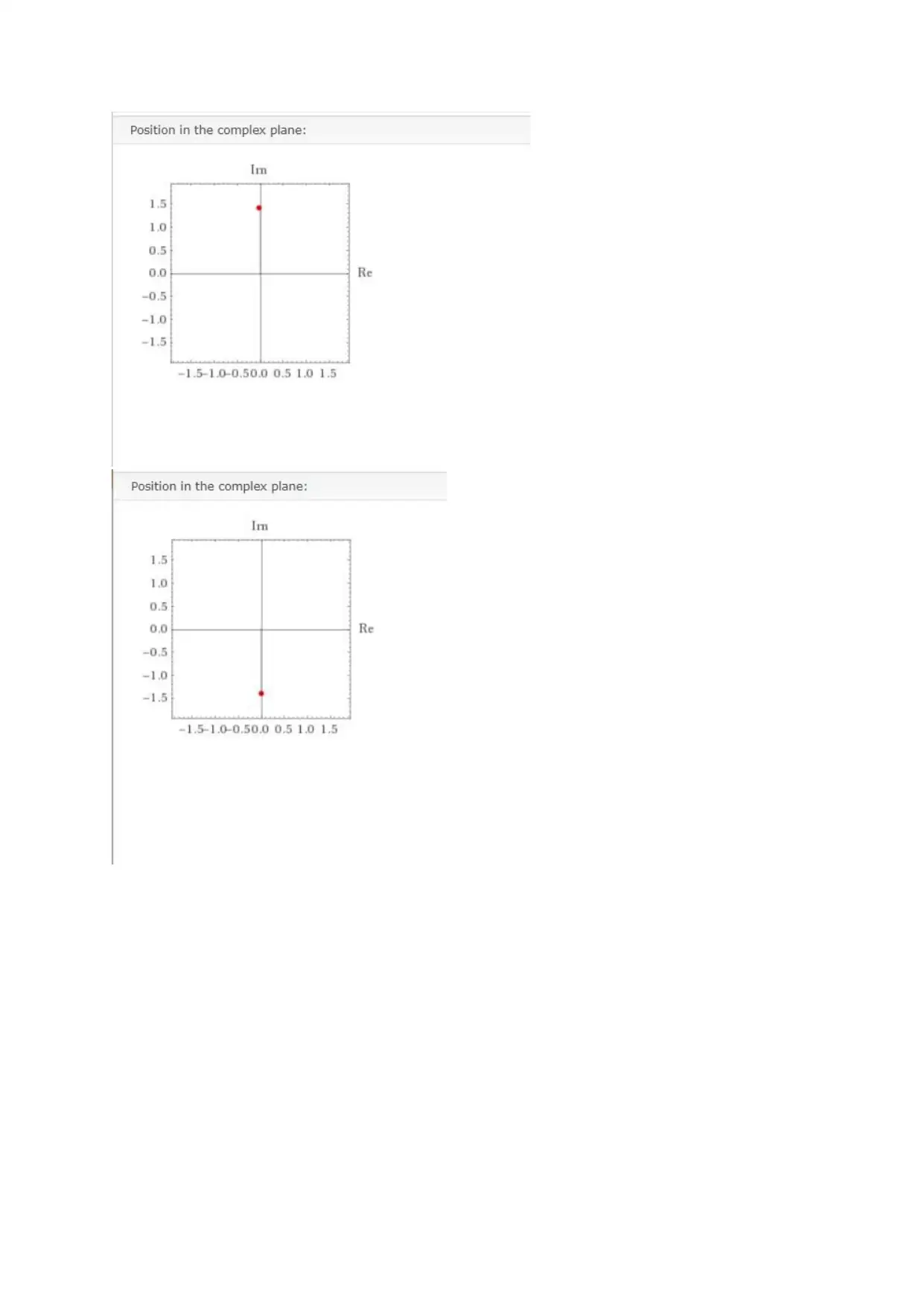
Ans.9.
The required equation is:
z^2 – (3/4 +2i +3/4 -2i)z + (3/4 + 2i)(3/4-2i)=0
or, z^2 –(6/4)z + (73/16)=0
Ans.10.
z^4 = -2√3-2i
= -2(√3 + i)
=-4( √ 3
2 + i
2)
=(2i)^2 (cos(π/6)+sin(π/6))
=((2i)^2)*(e^(iπ/6))
Or, z^2 = ±(2*i)*(e^(iπ/12))
Or, z= ±(√(2*i))*(e^(iπ/24))
⊘ This is a preview!⊘
Do you want full access?
Subscribe today to unlock all pages.

Trusted by 1+ million students worldwide
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
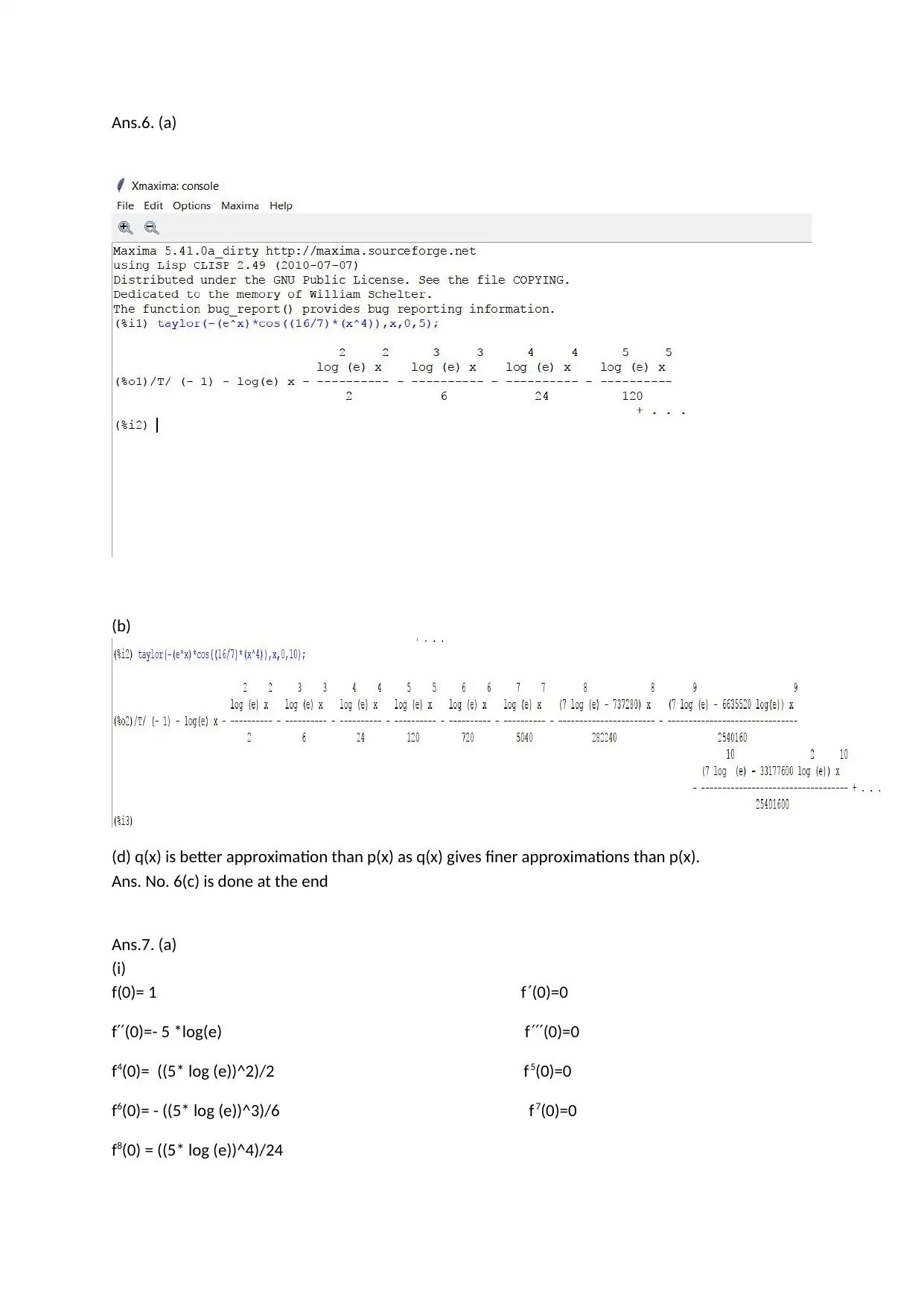
Ans.6. (a)
(b)
(d) q(x) is better approximation than p(x) as q(x) gives finer approximations than p(x).
Ans. No. 6(c) is done at the end
Ans.7. (a)
(i)
f(0)= 1 f ′(0)=0
f′′(0)=- 5 *log(e) f′′′(0)=0
f4(0)= ((5* log (e))^2)/2 f5(0)=0
f6(0)= - ((5* log (e))^3)/6 f7(0)=0
f8(0) = ((5* log (e))^4)/24
(b)
(d) q(x) is better approximation than p(x) as q(x) gives finer approximations than p(x).
Ans. No. 6(c) is done at the end
Ans.7. (a)
(i)
f(0)= 1 f ′(0)=0
f′′(0)=- 5 *log(e) f′′′(0)=0
f4(0)= ((5* log (e))^2)/2 f5(0)=0
f6(0)= - ((5* log (e))^3)/6 f7(0)=0
f8(0) = ((5* log (e))^4)/24

Therefore, the required Taylor Series is:
T(x)= 1 - 5 *log(e) * x^2 + ((5* log (e))^2)/2 * x^4 - ((5* log (e))^3)/6 * x^6 + ((5* log (e))^4)/24 *
x^8+ ……
(ii) The interval of validity for the series is
|-5*x^2|≤1
or, |x^2|≤1/5
or, - 1
√ 5 ≤ x ≤ 1
√ 5
(b) (i) 5
2 x +3 = 5
2 x +5−2 = 5
2(x−1)+5 = 1
2(x−1)/5+1 =
1
1+ 2 ( x−1 )
5
(ii) f(1)= 1
f′(1)= -(2/5)
f′′(1)= (4/25)
f′′′(1)= -(8/125)
Therefore the required Taylor Polynomial is
T(x)= 1 -(2/5) * (x-1) + (4/25) * (x-1)^2 - (8/125) * (x-1)^3 + ……
(iii)
The interval of validity is:
| 2(x−1)
5 |≤1
or, |x-1|≤ 5
2
or, -−5
2 ≤x-1≤ 5
2
or, -−3
2 ≤x≤ 7
2
Ans.11.
L.H.S.=
T(x)= 1 - 5 *log(e) * x^2 + ((5* log (e))^2)/2 * x^4 - ((5* log (e))^3)/6 * x^6 + ((5* log (e))^4)/24 *
x^8+ ……
(ii) The interval of validity for the series is
|-5*x^2|≤1
or, |x^2|≤1/5
or, - 1
√ 5 ≤ x ≤ 1
√ 5
(b) (i) 5
2 x +3 = 5
2 x +5−2 = 5
2(x−1)+5 = 1
2(x−1)/5+1 =
1
1+ 2 ( x−1 )
5
(ii) f(1)= 1
f′(1)= -(2/5)
f′′(1)= (4/25)
f′′′(1)= -(8/125)
Therefore the required Taylor Polynomial is
T(x)= 1 -(2/5) * (x-1) + (4/25) * (x-1)^2 - (8/125) * (x-1)^3 + ……
(iii)
The interval of validity is:
| 2(x−1)
5 |≤1
or, |x-1|≤ 5
2
or, -−5
2 ≤x-1≤ 5
2
or, -−3
2 ≤x≤ 7
2
Ans.11.
L.H.S.=
⊘ This is a preview!⊘
Do you want full access?
Subscribe today to unlock all pages.

Trusted by 1+ million students worldwide

(sinΘ)^4= ( 1
2∗i )^4 (e^(i*Θ)- e^(-i*Θ) )^4
= 1
16 ( (e^(2*i* Θ))^2+ (e^(-2*i* Θ))^2 + 2^2 – 2* (e^(2*i* Θ))*2 – 2* (e^(-2*i* Θ)) +6)
= 1
16( (e^(4*i* Θ))+ (e^(-4*i* Θ) +6 – 4 * (e^(2*i* Θ)) – 4* (e^(-2*i* Θ)))
= 1
16∗{ei∗4∗θ +e−i∗4∗θ−4∗ei∗2∗θ−4∗e−i∗2∗θ +6 }
R.H.S.= 1
8 (cos(4*Θ)- 4*cos(2*Θ)+ 3)
= 1
8 {1
2 ( ei∗4∗Θ +e−i∗4∗Θ )− 4
2 ( ei∗2∗Θ+ e−i∗2∗Θ ) +3 }
=
1
8 ∗1
2 {ei∗4∗θ +e−i∗4∗θ−4∗ei∗2∗θ−4∗e−i∗2∗θ +6 }
= 1
16∗{ei∗4∗θ +e−i∗4∗θ−4∗ei∗2∗θ−4∗e−i∗2∗θ +6 }
L.H.S.= R.H.S.
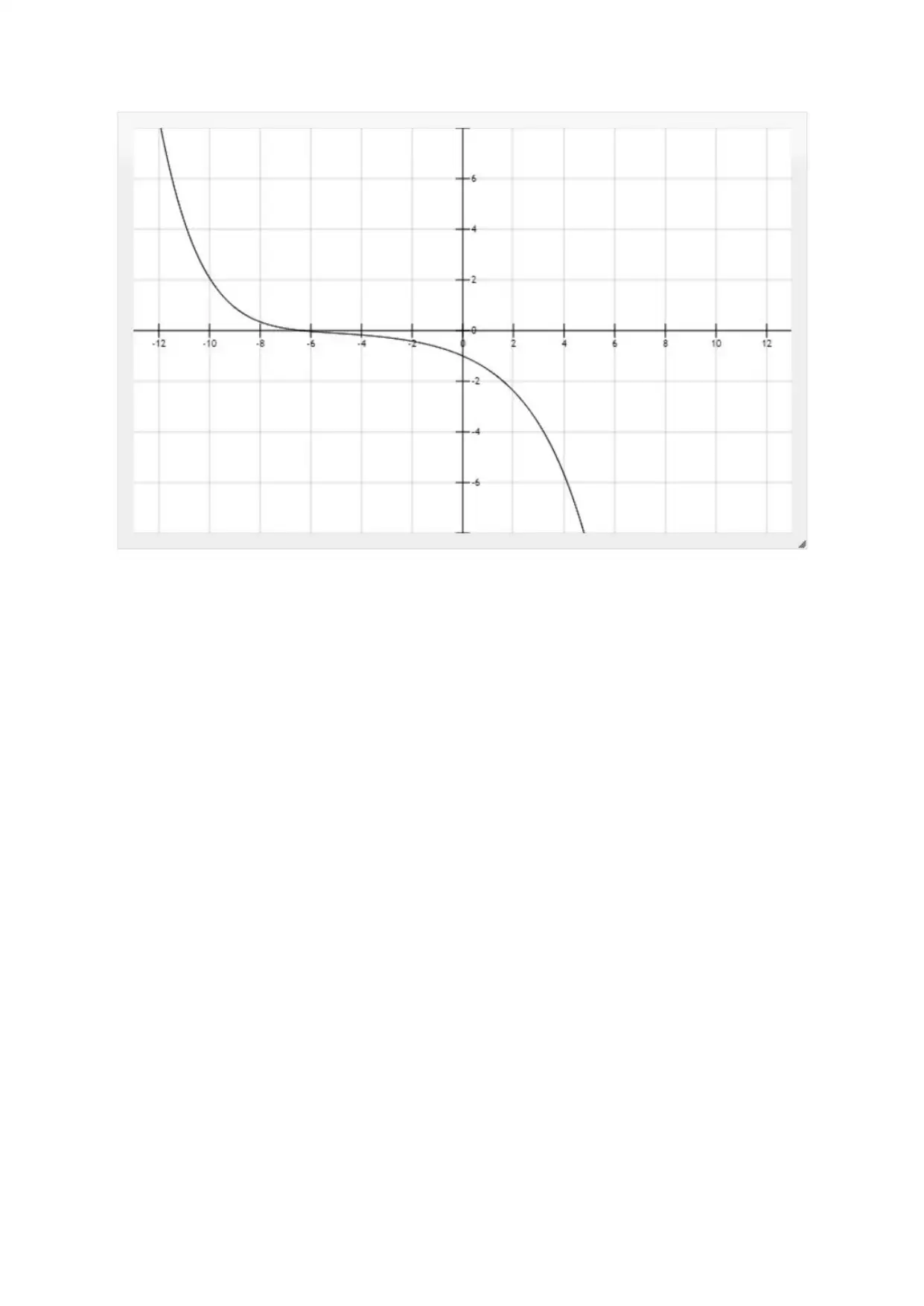
Ans.6. (c)
Graph of f:
2∗i )^4 (e^(i*Θ)- e^(-i*Θ) )^4
= 1
16 ( (e^(2*i* Θ))^2+ (e^(-2*i* Θ))^2 + 2^2 – 2* (e^(2*i* Θ))*2 – 2* (e^(-2*i* Θ)) +6)
= 1
16( (e^(4*i* Θ))+ (e^(-4*i* Θ) +6 – 4 * (e^(2*i* Θ)) – 4* (e^(-2*i* Θ)))
= 1
16∗{ei∗4∗θ +e−i∗4∗θ−4∗ei∗2∗θ−4∗e−i∗2∗θ +6 }
R.H.S.= 1
8 (cos(4*Θ)- 4*cos(2*Θ)+ 3)
= 1
8 {1
2 ( ei∗4∗Θ +e−i∗4∗Θ )− 4
2 ( ei∗2∗Θ+ e−i∗2∗Θ ) +3 }
=
1
8 ∗1
2 {ei∗4∗θ +e−i∗4∗θ−4∗ei∗2∗θ−4∗e−i∗2∗θ +6 }
= 1
16∗{ei∗4∗θ +e−i∗4∗θ−4∗ei∗2∗θ−4∗e−i∗2∗θ +6 }
L.H.S.= R.H.S.
Ans.6. (c)
Graph of f:
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
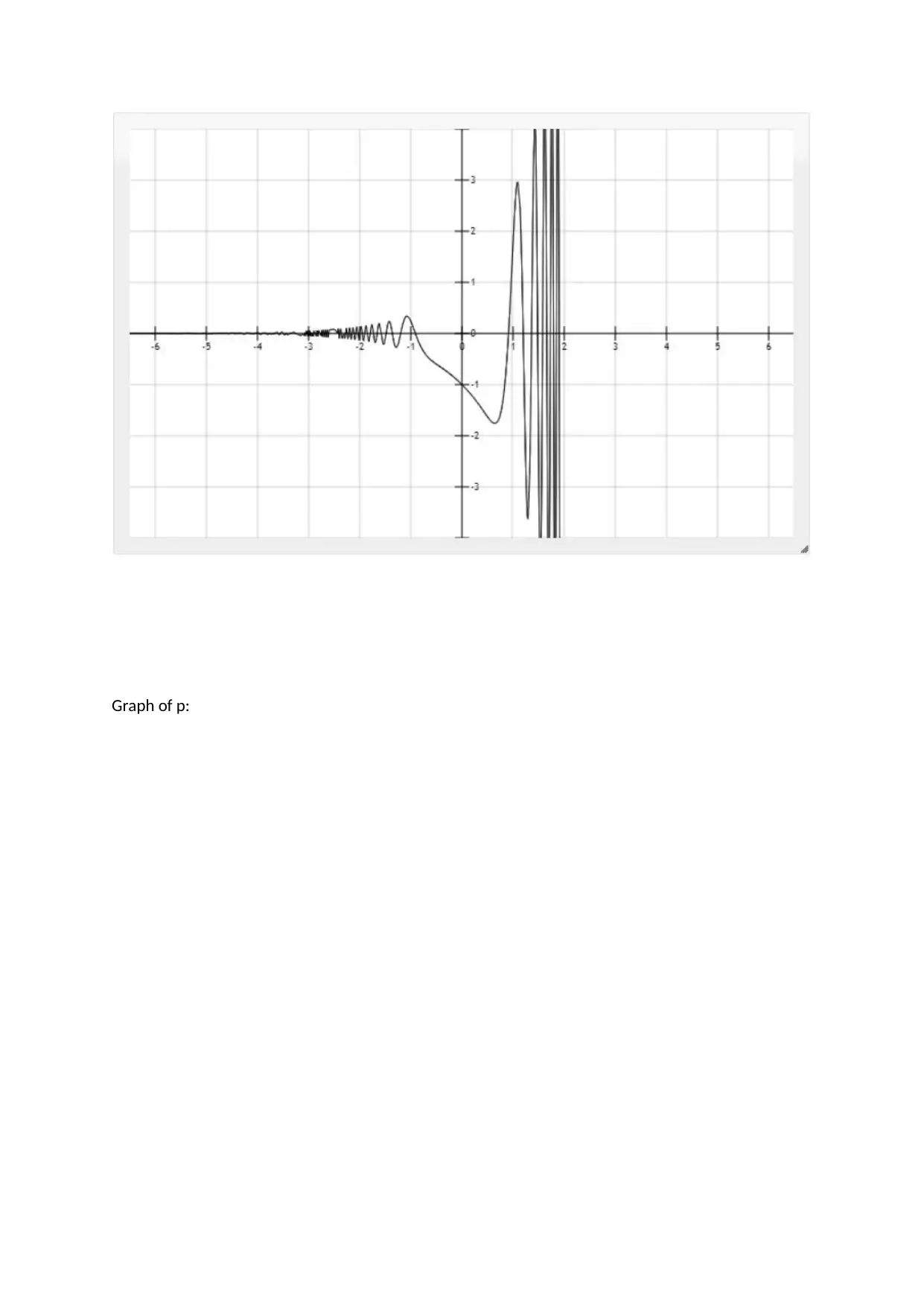
Graph of p:
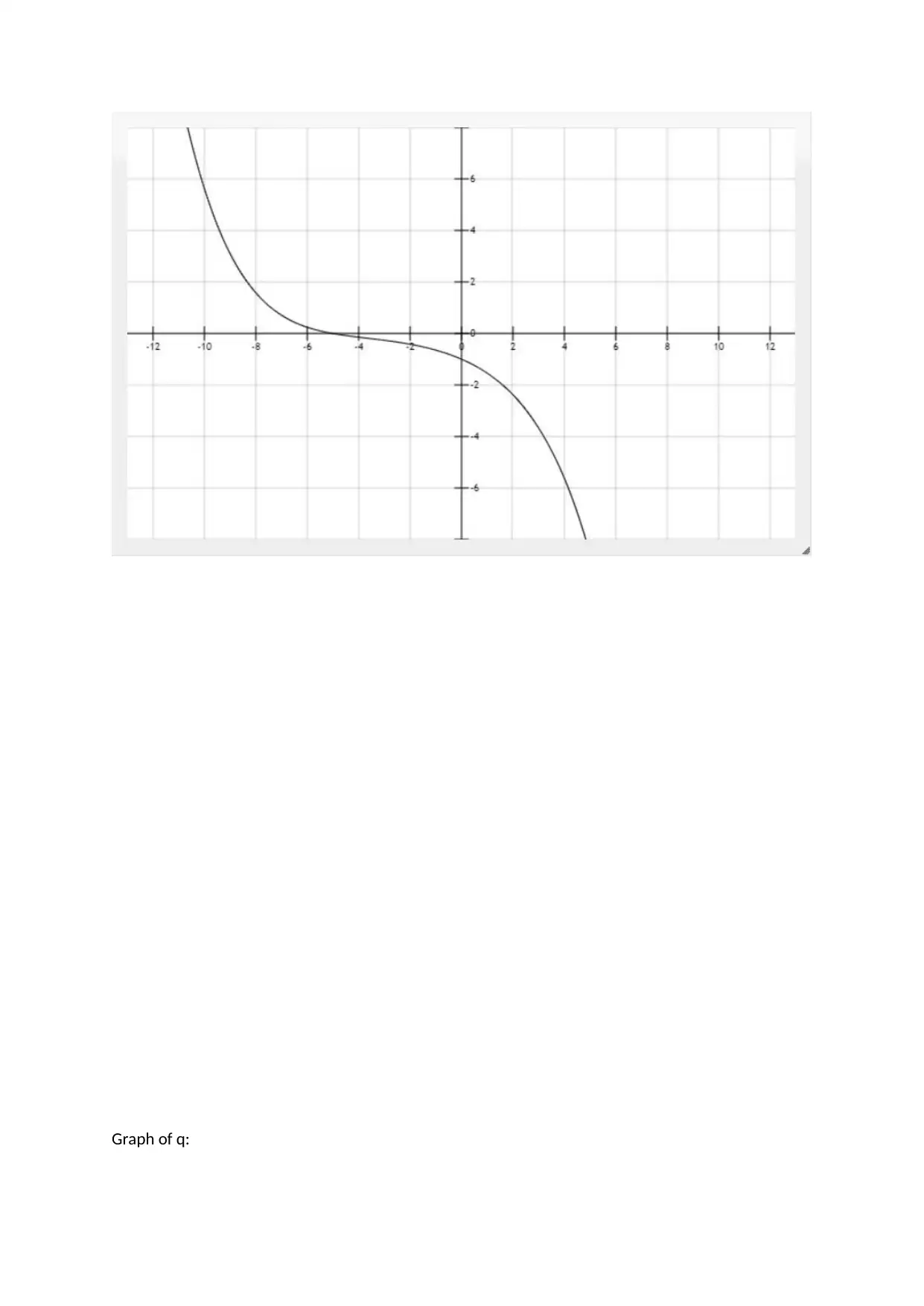
Graph of q:
⊘ This is a preview!⊘
Do you want full access?
Subscribe today to unlock all pages.

Trusted by 1+ million students worldwide
1 out of 10
Related Documents
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.




