Cost, Revenue, and Break-Even Analysis for Timber Toys and Insects

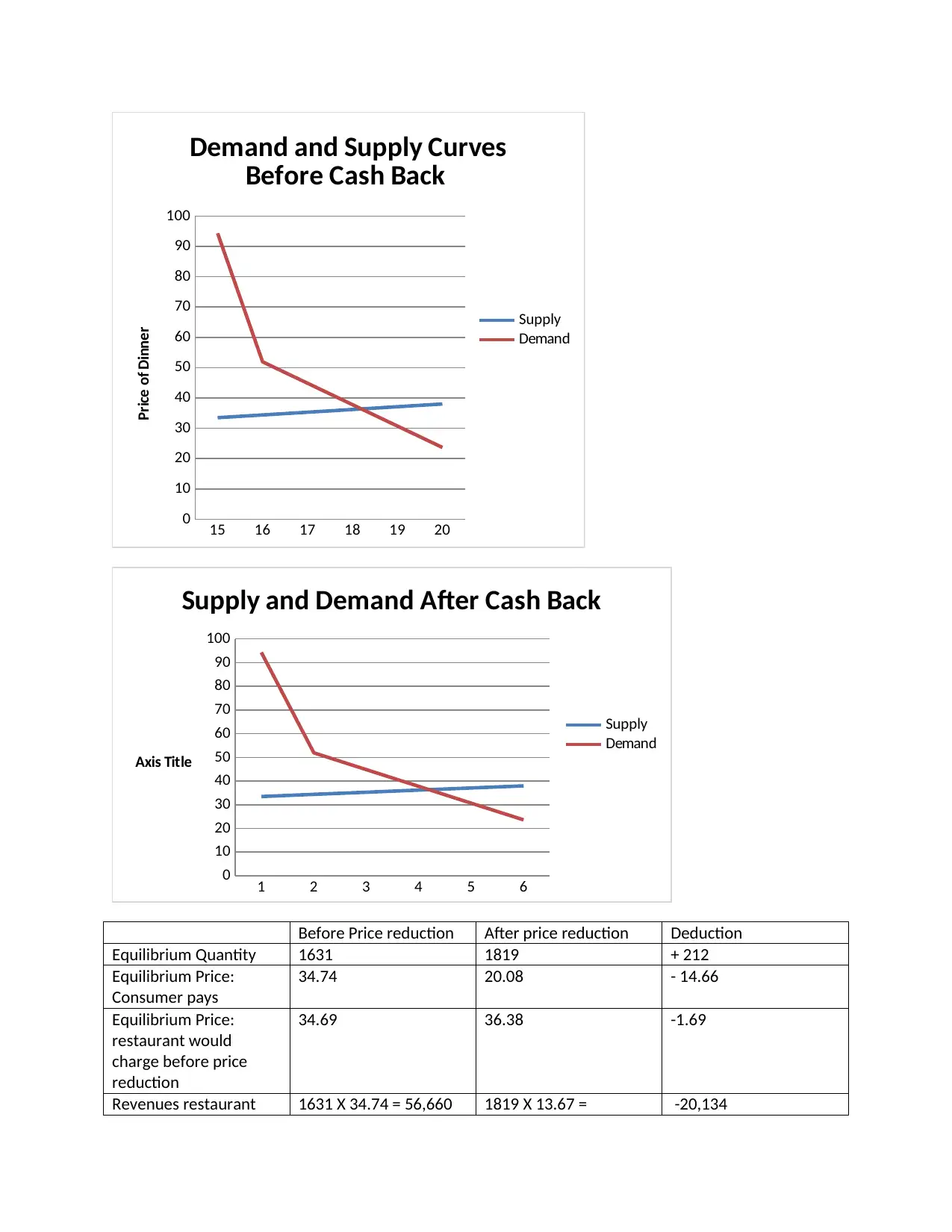
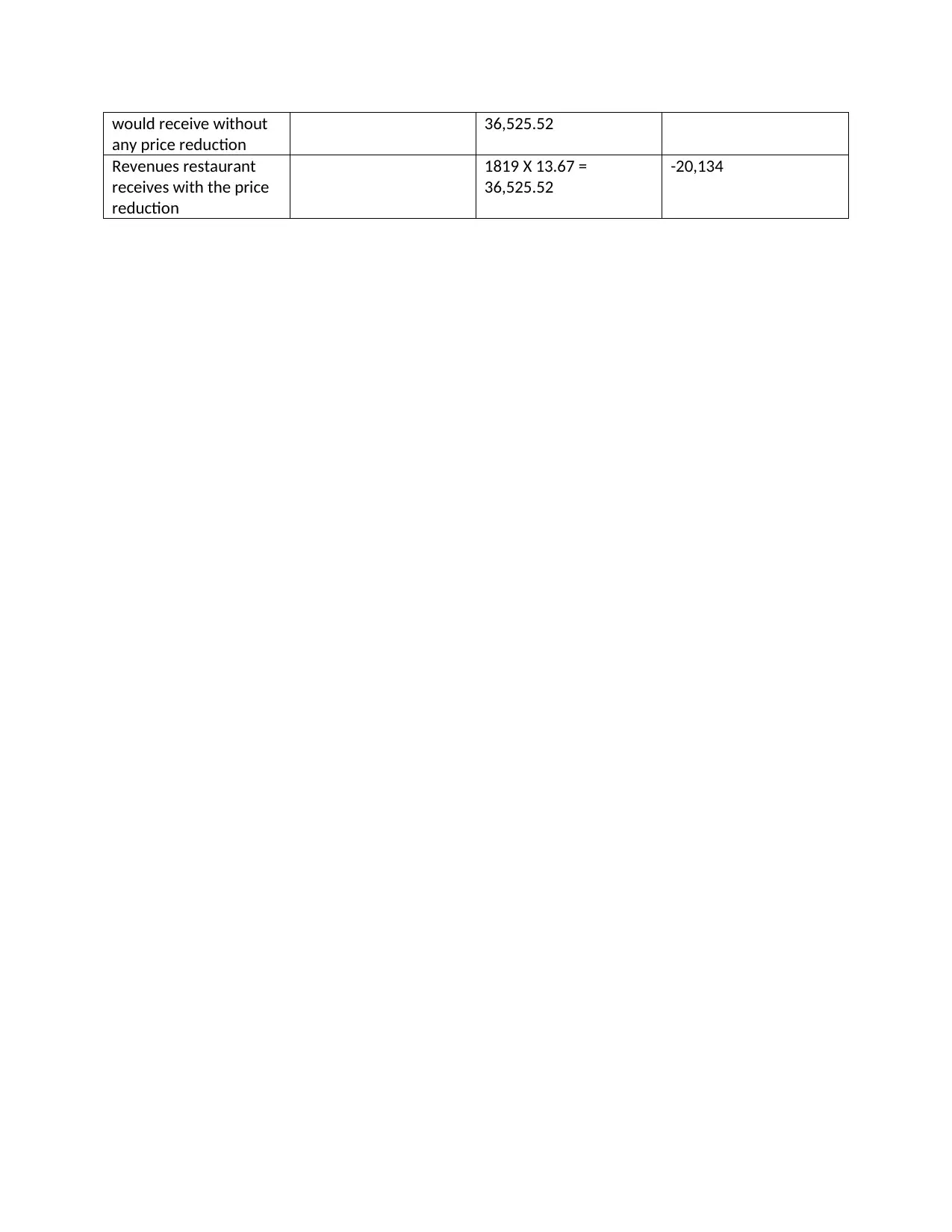
This assignment analyzes the cost and revenue functions for producing timber toys and insects, considering different materials like pine, myrtle, and sassafras. It calculates break-even points, where total revenue equals total cost, for various sale prices, highlighting how these points vary with price changes. The analysis includes fixed and variable costs, illustrating how profit margins are affected. Furthermore, the assignment explores demand and supply functions, determining equilibrium price and quantity before and after a price discount. The impact of the discount on equilibrium, consumer price, and restaurant revenue is evaluated, showcasing changes in customer numbers and profitability. The document uses Excel to generate random numbers for coefficients in the demand function and provides detailed calculations to determine the equilibrium, considering the impact of price reductions on both consumer and business outcomes.

Part 1
Information given below
Pine Myrtle Sassafras
Cost of Timber Parts $15.50 $17.50 $24.50
Cost of Wheels $3.00 $3.00 $3.00
Sale Price Range $20-25 $26-30 $32-40
Let the number of units produced are ‘x’
The total cost of producing ‘x’ goods = Number of Units Sold + Total Cost of Timber parts for x units +
Total Cost of Wheels for x units
 Total Revenue = Number of Units Sold (x) X Price (p)
 Total Revenue = Total Cost + Total Profits
 Total Profits = Profit per Unit X Number of Units Sold (n)
1.1)For Pine Timber Toys
Sale Price Variable Costs of Making in $) Profits Per Unit
20 18.5 1.5
21 18.5 2.5
22 18.5 3.5
23 18.5 4.5
24 18.5 5.5
25 18.5 6.5
Total Costs = 300 + 15.50 x + 3x
Total Cost = 300 +18.5 x - Cost Function
Let us assume the sale price of $20. Therefore, total revenues are 21x.
The revenue function, therefore, is
20x = 300 +18.5 x + 1.5 x -> Revenue Function

1 2 3 4 5 6
0
5
10
15
20
25
30
Costs and Profit Per Unit of Pine
Timber Toys
Sale Price
Price Per Unit
Axis Title
1.b)Similarly for Insects made out of Myrtle Timber
Sale
Price
Variable Costs of Making in
$) Profits Per Unit
26 20.5 5.5
27 20.5 6.5
28 20.5 7.5
29 20.5 8.5
30 20.5 9.5
1 2 3 4 5
0
5
10
15
20
25
30
35
Costs and Profit Per Unit of Myrtle Timber
Toys
Sale Price
Price Per Unit
Total Cost = 300 +20.5 y - Cost Function
Let us assume sale price of 27.
Total Revenues ($)= 27y
Total Revenue ($)= 300 + 20.5 y + 6.5y - Revenue Function

= 300 +26.5x
1.c) Similarly, for insects made out of Sassafras
Sale
Price
Variable Costs of Making in
$) Profits Per Unit
32 27.5 4.5
33 27.5 5.5
34 27.5 6.5
35 27.5 7.5
36 27.5 8.5
37 27.5 9.5
38 27.5 10.5
39 27.5 11.5
40 27.5 12.5
1 2 3 4 5 6 7 8 9
0
5
10
15
20
25
30
35
40
45
Costs and Profit Per Unit of Sassafras
Timber Toys
Sale Price
Price Per Unit
Total Cost Function = 300 +27.5 y - Cost Function
Let us assume sale price of 33.
Total Revenues ($)= 33z
Total Revenues ($)= 300 + 27.5 z + 5.5z  Revenue Function
= 300 +26.5z

Q2) Total revenue and Total Cost Curve Intersect when Total Revenue = Total Cost. This is known as
Break even point.
 In case of Pine Timber Toys, Total Revenues
20x = 300 +18.5 x
1.5 x = 300
X= 200
This is the break even point when price is $20 per unit.
Similarly,
 For Insects made out of Myrtle,
27y = 300 + 20.5y
Solving for y, we get 46.1
Y= 46
Hence, break even point is 46 units
 For Sassafras insects
Total Revenues = 300 + 24.5z +3z
= 300 +27.5z
At price $33 , break even point is
33x = 300 +27.5z
6z = 300
z= 50
Summary
As seen above the revenues increase as the price range increases. The variable costs are the cost of
timber while $300 are the overhead costs. The overhead costs of $300 and the cost of wheel $3 per unit
are known as fixed costs.
The break even points are those points where the Company makes neither profits nor loss. This is the
minimum number of unites that the Company must sell in order to ensure that they do not go out of
business As seen here, the break even point goes on decreasing as the price rises. This indicates that the

As seen above, the break even point is lowest for Myrtle Toys. This implies that the Company may be
more profitable from producing insects from Myrtle as the selling price is higher in range and break even
point low.
Some of the other assumptions made were:
 Taxes are included in the overall sales price.
 There are no other costs
Demand is stable. In reality, the market demand large influences the decision to produce. For example,
while he break even point for the Sassafras timber may be low, even the lower quantities might not be
demanded.

Part 11
Demand Equation :
 P = 150- 10aq
Using the random number function in excel, we obtained the Co-efficient
 Random Number a = 0.7067
 Random number b = 0.450267
Substituting this value in demand function we get:
P = 150 - 10 (0.7067) q ---- equation 1
 The supply function is given as
P=0.00001q2 +2bq+ 20
= 43.31
= 0.00001q2 + 2(0.450267) q +20 ---- equation 2
A) Equilibrium price and quantity occur when demand = supply
i.e when :
150 - 10 (0.7067) q = 0.00001q2 + 2(0.450267) q +20
150 – 7.067 q= 0.00001q2 + 0.900534 q +20
0.00001q2 + 7.967q - 130 = 0
Solving for ‘q’ we, get x as 16.31 or x as -796716.31,
Since, the number of customers cannot be negative
0.00266+ 14.68+20
Q = 16.31
It has been stated that the number of customers is in hundreds hence, total number of customers at
equilibrium = 16.31 X100 i.e. 1631.
Solving the equation for ‘p’ or dinner price, we get
P = 150- 10aq

= 150 - 10 (0.7067) (16.31)
= 150 – 115.26
p = 34.74
The equilibrium dinner price before discount for consumer is $34.74 while the equilibrium number of
customers is 1631.
The equilibrium dinner price before discount for the Restuara is $34.69 while the equilibrium number
of customers is 1631.
B)Further , we update the demand function to factor in the discount.
P - 15= 150- 10aq
Therefore, p = 165 – 10aq
The equilibrium price is
165 – 10aq = 0.00001q2 +2bq+ 20
Substituting Co-efficients, we get
165 - 10 (0.7067) q = 0.00001q2 + 2(0.450267) q +20
Therefore, 0.00001q2 + 7.967q - 145 = 0
q = 18.19 or q = -796718.199
Taking q as 18.19, we get the equilibrium number of customers at 18.19 or 1819.
We solve for p,
P = 150- 10aq +15
P = 20.08
The new equilibrium price for the consumer is $20.08 and new quantity is 1819.
The new equilibrium price for the consumer is $36.38and new quantity is 1819

15 16 17 18 19 20
0
10
20
30
40
50
60
70
80
90
100
Demand and Supply Curves
Before Cash Back
Supply
Demand
Price of Dinner
1 2 3 4 5 6
0
10
20
30
40
50
60
70
80
90
100
Supply and Demand After Cash Back
Supply
Demand
Axis Title
Before Price reduction After price reduction Deduction
Equilibrium Quantity 1631 1819 + 212
Equilibrium Price:
Consumer pays
34.74 20.08 - 14.66
Equilibrium Price:
restaurant would
charge before price
reduction
34.69 36.38 -1.69
Revenues restaurant 1631 X 34.74 = 56,660 1819 X 13.67 = -20,134

would receive without
any price reduction
36,525.52
Revenues restaurant
receives with the price
reduction
1819 X 13.67 =
36,525.52
-20,134