Derivatives Pricing, Hedging, and Arbitrage Homework Solution

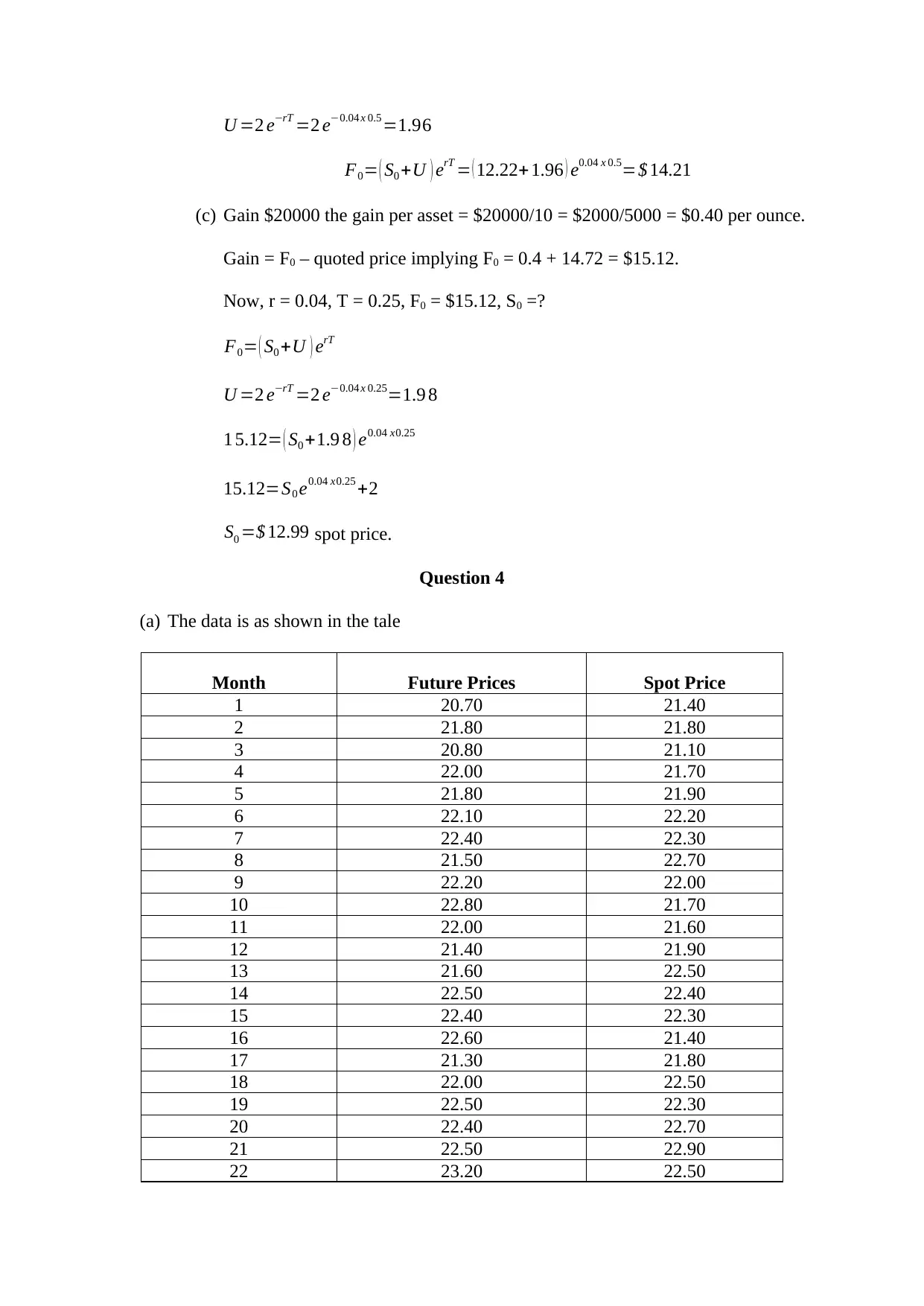
This document presents solutions to a finance assignment focusing on derivatives, hedging, and arbitrage strategies. The solutions cover various aspects, including calculating forward prices, determining the value of contracts, and understanding arbitrage opportunities. The assignment delves into concepts such as spot prices, future prices, and the impact of interest rates and coupon values. It also explores hedging strategies, including calculating the minimum variance hedge ratio and determining the optimal number of contracts. The solutions are detailed, providing step-by-step calculations and explanations to facilitate understanding of the underlying financial principles. The assignment covers key concepts related to the valuation of financial derivatives, risk management techniques, and the practical application of these concepts in real-world scenarios. The document is designed to assist students in grasping the intricacies of financial derivatives and their applications.

Question 1
(a) F0=¿ $928.17, r = 0.04, S0 = $980, and q =?
F0=S0 e (r−q ) T=980 e
( 0.01−q ) 1
928.17=970.25 e−q
q=ln ( 970.25
928.17 )=0.0443
coupon value = 0.0443 x $1,000 = $44.3.
(b) Given T = 6 months = 6
12=0.5, r = 0.04, S0 = $980, and q = 0.0443
F0=S0 e (r−q ) T=9 80 e
( 0.01−0.0443 ) x 0.5=$ 963.34
(c) The arbitrageur borrows $980 to purchase the bond and short a forward contract. To
compute the present value of the first coupon, we discount
44.3 e−0.0 4 x 3
12 =$ 43.86
The remaining $980 - $43.86 = $936.14 is borrowed at 4% annually for remaining 3
months
936.14 e−0.04 x 3
12 =$ 926.83.
The arbitrageur makes
$962.28 - $926.83 = $ 35.45 for 3 months. Therefore, the arbitrage strategy is action
now and borrow $980 for $43.86 for three months and $936.14 for 3 months. Buy one
unit of the asset and enter into forward contract to sell asset in 6 months for $962.28.
Next action in three months receive $43.86 of income on asset use $43.86 to repay
first loan with interest. Finally, action in 6 months. Sell asset for $962.28 use $926.83
to repay second loan with interest.

Question 2
(a) Here r = 0.01, S0 = 1458, T = 1, and q = 2ln (1+ 0.02
2 ) = 0.0199.
The future price F0 is therefore,
F0=S0 e (r−q ) T=1458 e( 0.01−0.0199 ) 1=1,443.64
(b) F0 = 1,432, r = 0.01, T = 1, q = 0.0199, and S0 =?
1,432=S0 e (0.01−0.0199 ) 1
S0 = 1,446.25 = K
The value of the contract is
f = ( F0−K ) e−rT = ( 1,432−1446 ) e−0.01=−0.010.
Therefore, this contract does not contain value its not worth investing in.
(c) F0 = 1,450, r = 0.01, T = 1, q = 0.0199, and S0 =?
1,4 50=S0 e( 0.01−0.0199 ) 1
S0 = 1,464 = K
The value of the contract is
f = ( F0−K ) e−rT = ( 1,4 50−146 4 ) e−0.01=−13.86.
Therefore, this contract does not contain value it is not worth investing in.
Question 3
(a) r = 0.04, T = 1, F0 = $14.72, S0 =?
F0= ( S0 +U ) erT
U =2 e−rT =2 e−0.04=1.922
14.72= ( S0+1.922 ) e0.04
14.72=S0 e0.04 +2
S0 =$ 12.22 spot price.
(b) The following are given r = 0.04, T = 0.5, S0 = $12.22 and

U =2 e−rT =2 e−0.04 x 0.5 =1.96
F0= ( S0 +U ) erT = ( 12.22+ 1.96 ) e0.04 x 0.5=$ 14.21
(c) Gain $20000 the gain per asset = $20000/10 = $2000/5000 = $0.40 per ounce.
Gain = F0 – quoted price implying F0 = 0.4 + 14.72 = $15.12.
Now, r = 0.04, T = 0.25, F0 = $15.12, S0 =?
F0= ( S0 +U ) erT
U =2 e−rT =2 e−0.04 x 0.25=1.9 8
1 5.12= ( S0 +1.9 8 ) e0.04 x0.25
15.12=S0 e0.04 x0.25 +2
S0 =$ 12.99 spot price.
Question 4
(a) The data is as shown in the tale
Month Future Prices Spot Price
1 20.70 21.40
2 21.80 21.80
3 20.80 21.10
4 22.00 21.70
5 21.80 21.90
6 22.10 22.20
7 22.40 22.30
8 21.50 22.70
9 22.20 22.00
10 22.80 21.70
11 22.00 21.60
12 21.40 21.90
13 21.60 22.50
14 22.50 22.40
15 22.40 22.30
16 22.60 21.40
17 21.30 21.80
18 22.00 22.50
19 22.50 22.30
20 22.40 22.70
21 22.50 22.90
22 23.20 22.50

23 22.50 22.70
24 23.20 22.70
The minimum variance hedge ratio is given as follows:
h=ρ . δs
δF
Where,
ρ – correlation between future price and spot price
δsand δF – standard deviation of the spot price and future price respectively.
From excel, ρ = 0.520259, δs=0. 492774 and δF =0. 648689.
h=0.520259 x 0. 492774
0.648689 =0.395213
(b) The company should take long contracts.
Optimal number of contracts = ¿ desired underlying portfolio
contract ¿ one futurecontract x h
Optimal contract = 125,000
1,000 x 0.395213=49 contracts.
(c) The effective price paid by the company is calculated as
Price = 21.4 + (23.2 – 20.9) = $23.7