Financial Math Assignment: Calculations for Personal Finance

This document provides complete solutions to a series of financial math problems. The problems cover a range of personal finance topics, including calculating monthly expenses from annual and semi-annual costs, determining the future value of investments with compound interest, calculati...Read More

Solve the following problems completely. Show your work.
1. Mike has an annual expense of $846 for life insurance, a semi-annual car insurance
premium of $750,
and monthly charges of $90 for health insurance. Prorate the given expenses to find the
monthly cost.
Annual life insurance expense $ 846
This gives ¿ 846
12 =$ 70.5
Semi-annual car insurance expense $ 750
Gives 750
6 =$ 125
Monthly health insurance charges $ 90
The total monthly cost
¿ $ 70.5+$ 125+$ 90=$ 285.5
2. Suppose that you invest $500 in an account that earns interest at an APR of 2.75%,
compounded
quarterly. Determine the accumulated balance after 5 years.
Accumulated balance= p ( 1+ i
n )
nt
Where p is the invested amount, r the APR and n the compounding times per year
¿ 500∗(1+ 0.0275
4 )4∗5
=$ 573.43
3. Your savings account pays an APR of 5.2%, compounded annually. If you deposit $600
at the end of
each year for 8 years, what will be the accumulated balance in the account?
Since the $ 600 is payable at the end of every year the payment steam is treated as an
ordinary annuity.

To calculate the accumulated values, we use the formula
FV =C∗( ( 1+ i ) n−1)/i ¿
Where c is the periodic cashflow, I the APR and n the number of payments
FV =600∗( (1+0.052 )8−1)/0.052
¿ $ 5770.61
4. Calculate the monthly payments for a home mortgage of $189,000 with a fixed APR of
5.875% for 15
years.
The future value is $ 189,000 and the interest rate is 0.05875 with a payment period of 15
years
Using the formula
FV =C∗( ( 1+ i ) n−1)/i ¿
We find that
FV /¿( ( 1+i ) n−1)/i¿=C
Hence
C= 189000
( 1+0.05875 )15−1
0.05875
=$ 8,197.59
5. Find the net monthly cash flow. (1 month = 4 weeks)
Income Expenses
Job $800 per week Rent $650 per month

Loan $3000 per semester Groceries $60 per week
Tuition and fees $2500 per semester
Miscellaneous $150 per week
Cash inflow
Job: 800∗4=$ 3200
Loan: 3000
3 =$ 1000
Total $ 4200
Cash outflow
Rent; $ 650
Grocery: 60∗4=240
Tuition: 2500
3 =$ 833.33
Miscellaneous; 150∗4=$ 600
Total; $ 2323.33
Net monthly cashflow is; 4200−2323.33=$ 1876.67
6. Prorate the following expenses and find the corresponding monthly expense. Lan pays a
semiannual premium of $450 for automobile insurance, a monthly premium of $120 for
health insurance, and an annual premium of $500 for life insurance. Round to the nearest
cent as needed.
Semiannual premium $ 450

Monthly; 450
6 =225
Monthly health insurance premium: $ 120
Annual life insurance premium; $ 500
Monthly we have 500
12 =$ 41.67
Total monthly expense
¿ 225+120+41.67=$ 386.67
Which is equivalent to $ 386.70
7. Comparing Investment Plans: Rosa invests $3000 in an account with an APR of 4%
and annual compounding. Julian invests $2500 in an account with an APR of 5% and
annual compounding. Compute the balance in each account after 5 and 20 years.
Rosa
Accumulated amount:
After 5yrs ¿ 3000∗( 1+ 0.04 ) 5=$ 3649.96
After 20yrs ¿ 3000∗( 1+ 0.04 )20=$ 6573.37
Julian
Accumulated amount
After 5yrs; 2500∗( 1+ 0.05 ) 5=$ 3190.70
After 5yrs; 2500∗( 1+ 0.05 ) 20=$ 6633.24
8. Choosing a Personal Loan: You need to borrow $12,000 to buy a car and you determine
that you can afford monthly payments of $250. The bank offers three choices: a 3-year
loan at 7% APR, a 4-year loan at 7.5% APR, or a 5-year loan at 8% APR. Which loan
best fits your needs? Explain your reasoning.
Evaluating the bank choices
3- year loan
Present value; PV =R∗( 1− ( 1−i ) )−n ¿ /i
Where R is the monthly payments and n the compounding period

Rate is 0.07
12 =0.005833
PV = 250∗1− ( 1+0.005833 )−36
0.005833 =$ 8096.66
4- year loan
PV = 250∗1− ( 1+0.00625 )−48
0.00 625 =$ 10 , 339.59
5-year loan
PV = 250∗1− ( 1+0.006 67 )−60
0.0 0667 =$ 12328.44
Using the present value for each of the given loan options, the 5-year loan option is the
recommended loan. This loan has a present value of $ 12, 328.44 which is adequate to
cater for the $ 12,000 car that is to be purchased.
9. Suppose your after-tax annual income is $38,000. Your annual expenses are $12,000 for
rent, $6000 for food and household expenses, $1200 for interest on credit cards, and
$8500 for entertainment, travel, and others. Do you have a surplus or a deficit? Explain
and show all work below.
Cash inflow
Income $ 38000
Outflow
Rent $ 12000
Food $ 6000
interest expenses $1200
Entertainment $ 8500
Total $ $ 27,700
Net cashflow is $ 10300.
Since the net income is a positive value, I have a surplus income of $ 10,300
10. 1. Mike has an annual expense of $846 for life insurance, a semi-annual car insurance
premium of $750,

11. and monthly charges of $90 for health insurance. Prorate the given expenses to find the
monthly cost.
12.
13.
14.
15.
16.
17.
18.
19.
20.
21.
22.
23.
24.
25. 2. Suppose that you invest $500 in an account that earns interest at an APR of 2.75%,
compounded
26. quarterlyHow Much House Can You Afford? You can afford monthly payments of
$500. If current mortgage rates are 3.75% for a 30-year fixed rate loan, how much can
you afford to borrow? (Hint: You will need to solve for the loan payment formula for P.)
To solve this, we calculate the present value of the loan
Interest will be 0.003135 compounded monthly
PV =50 0∗1− ( 1+ 0.003125 )−3 60
0.003125 =$ 107 ,964.41
At a monthly payment of $ 500 and using the details of the available loan, I can afford to
borrow up to a maximum of $ 107 , 964.41.
27. A student loan of $50,000 at a fixed APR of 6% for 20 years.
a. Calculate the monthly payment

The interest compounded monthly is 0.005
The monthly payment is thus
¿ 50000
1− ( 1+ 0.005 )−24 0
0.00 5
=$ 358.22 per months
This is equivalent to 358 months
b. Determine the accumulated balance after 5 years.
c.
d.
e.
f.
g.
h.
i.
j.
k.
l.
m.
n.
o.
p.
q. 3. Your savings account pays an APR of 5.2%, compounded annually. If you deposit
$600 attotal amount paid over the endterm of
r. each year for 8 years, what will be the accumulated balance in the account?loan.
Total amount paid is 358.33∗240=$ 85,971.73
28. Fred pays $6200 for each of two semesters to attend a local college. He pays an
additional $560 for books each semester; summer school tuition is $1240 and books for
summer school are $110. Prorate the given expenses to find the monthly cost.
Each of the two semesters; 6200∗2=$ 12400
Monthly; $ 12400
6 =$ 2066.67

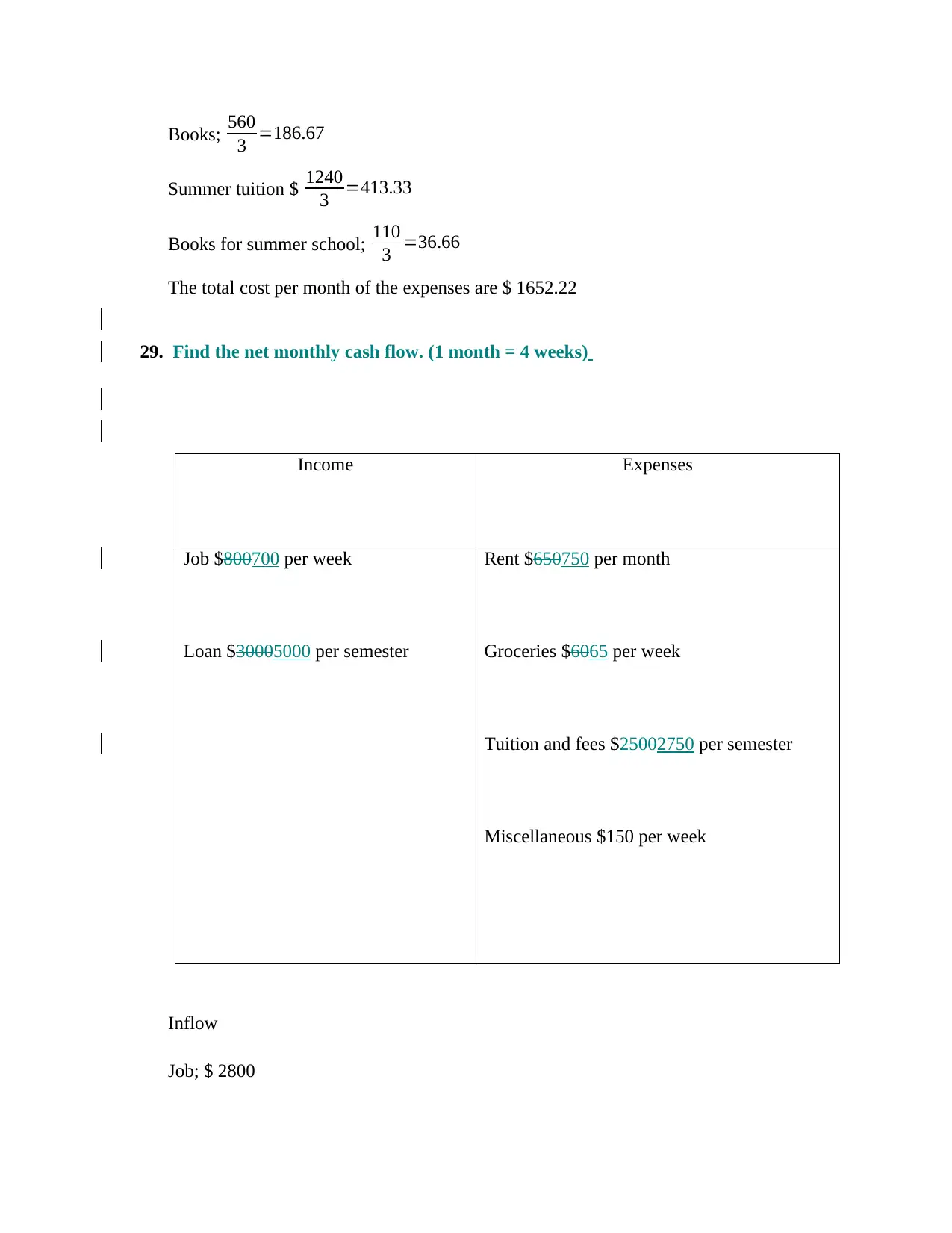
Books; 560
3 =186.67
Summer tuition $ 1240
3 =413.33
Books for summer school; 110
3 =36.66
The total cost per month of the expenses are $ 1652.22
29. Find the net monthly cash flow. (1 month = 4 weeks)
Income Expenses
Job $800700 per week
Loan $30005000 per semester
Rent $650750 per month
Groceries $6065 per week
Tuition and fees $25002750 per semester
Miscellaneous $150 per week
Inflow
Job; $ 2800

Loan $ 1666.67
Total; $ 4466.67
Outflows
Rent 750
Groceries $ 260
Tuition $ 916.67
Miscellaneous $ 600
Total; 2526.67
The net monthly cashflow is $ 1940