Geometry Homework: Area, Volume, and Coordinate Geometry Solutions
VerifiedAdded on 2023/01/20
|9
|716
|51
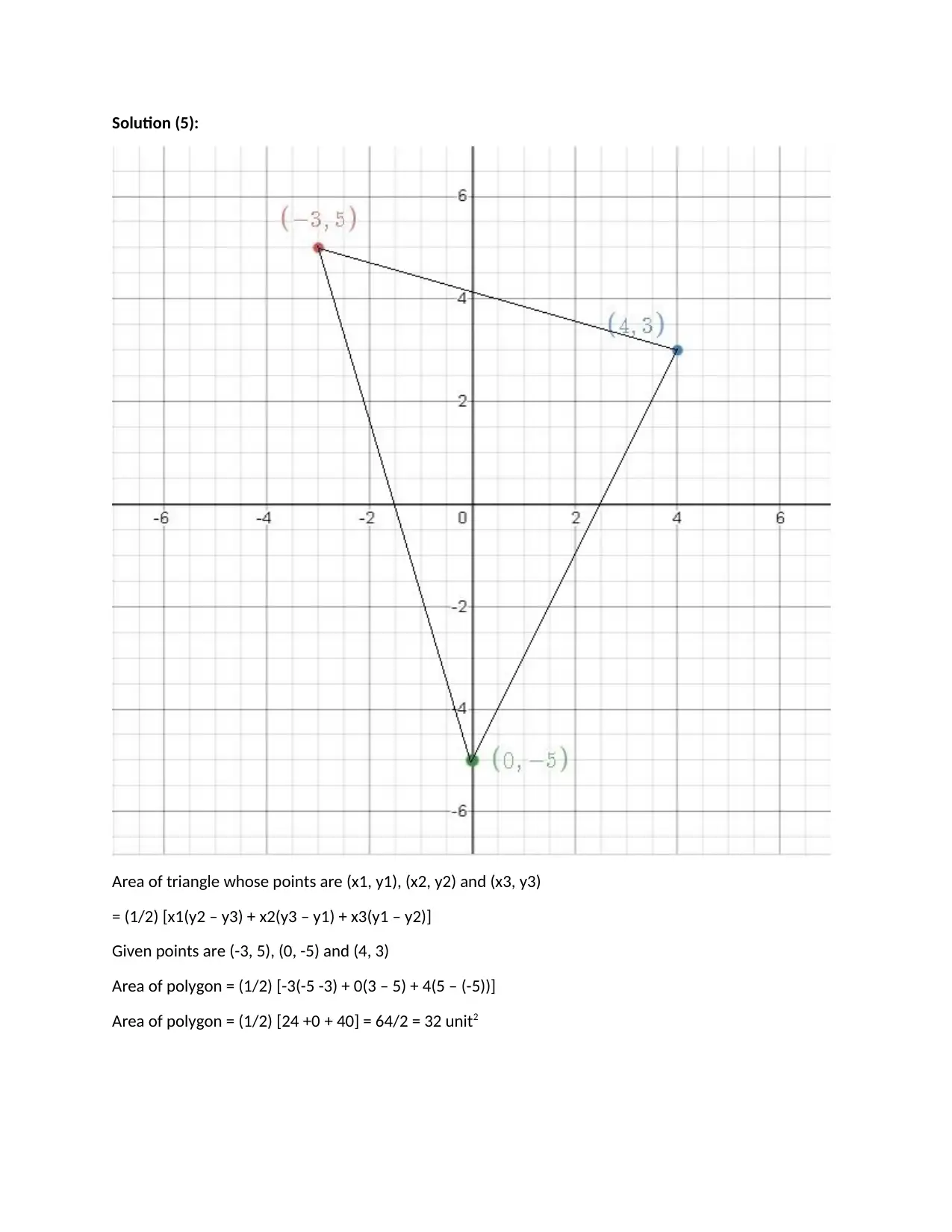
Homework Assignment
AI Summary
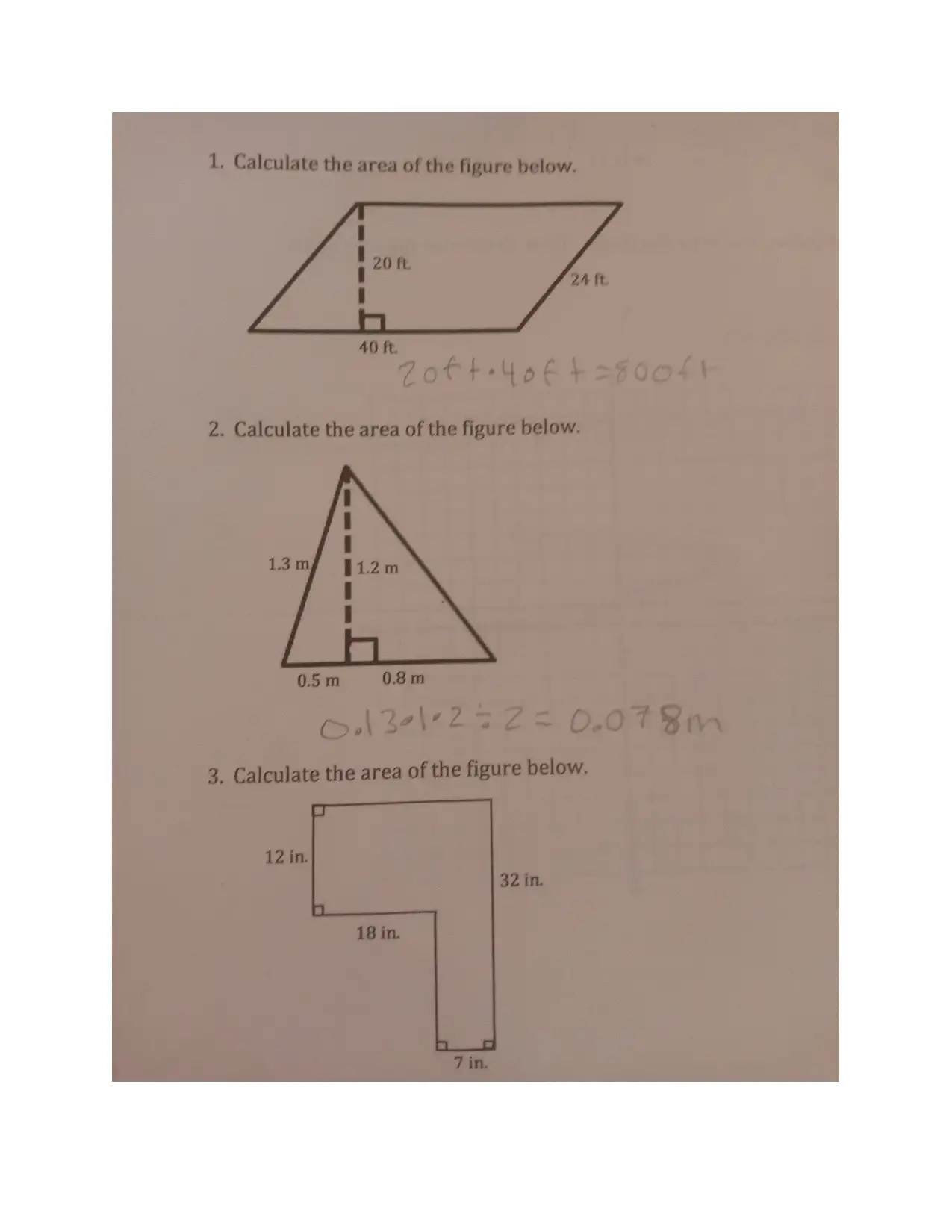
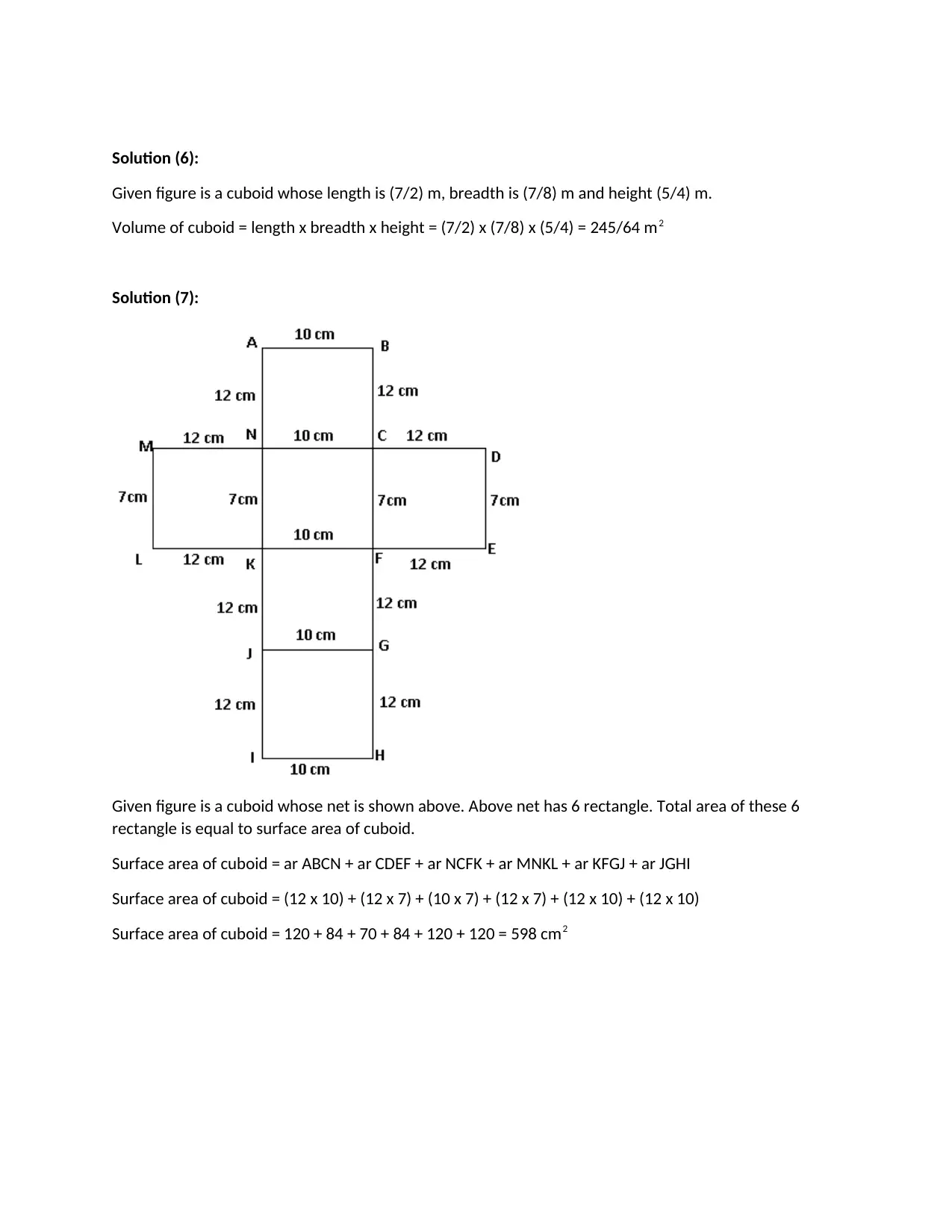
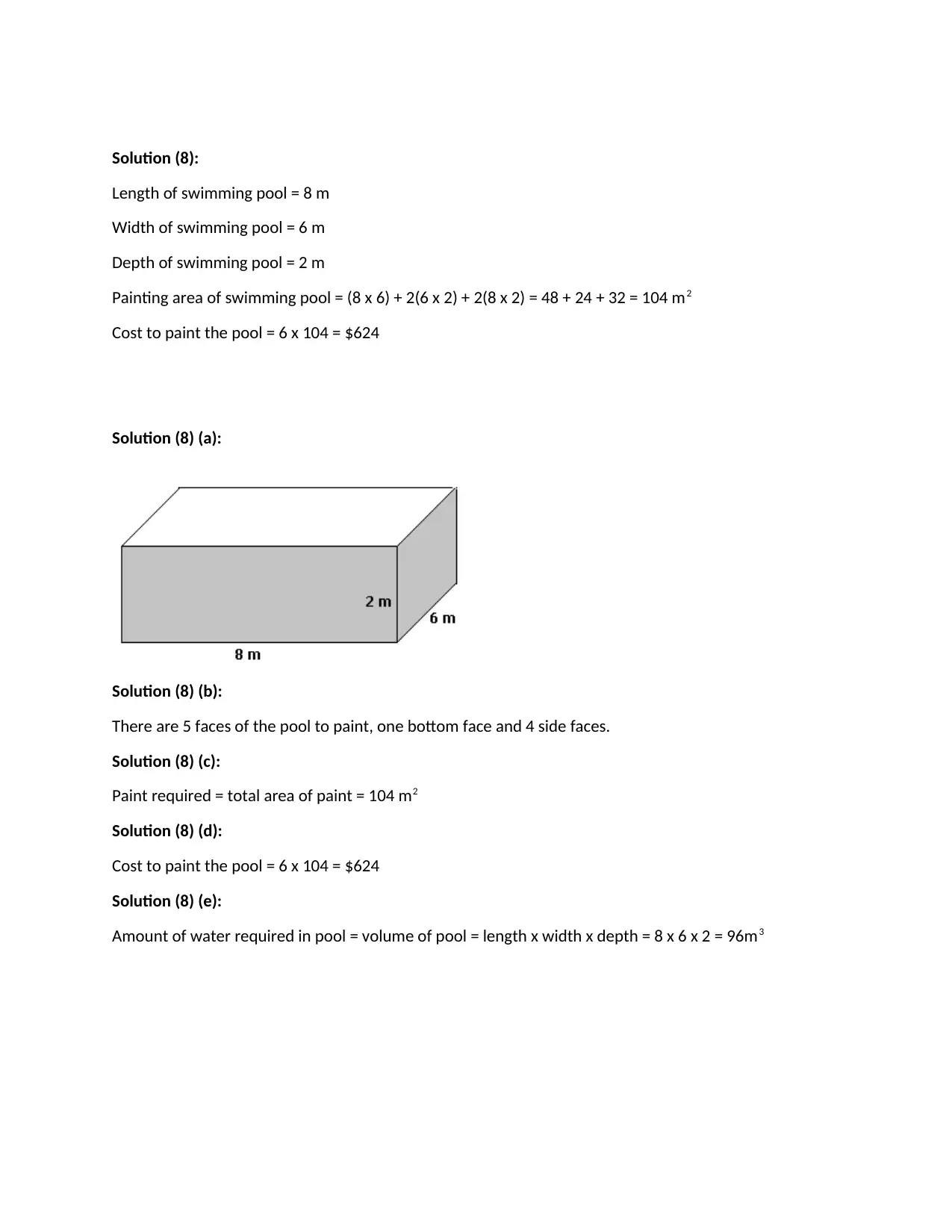
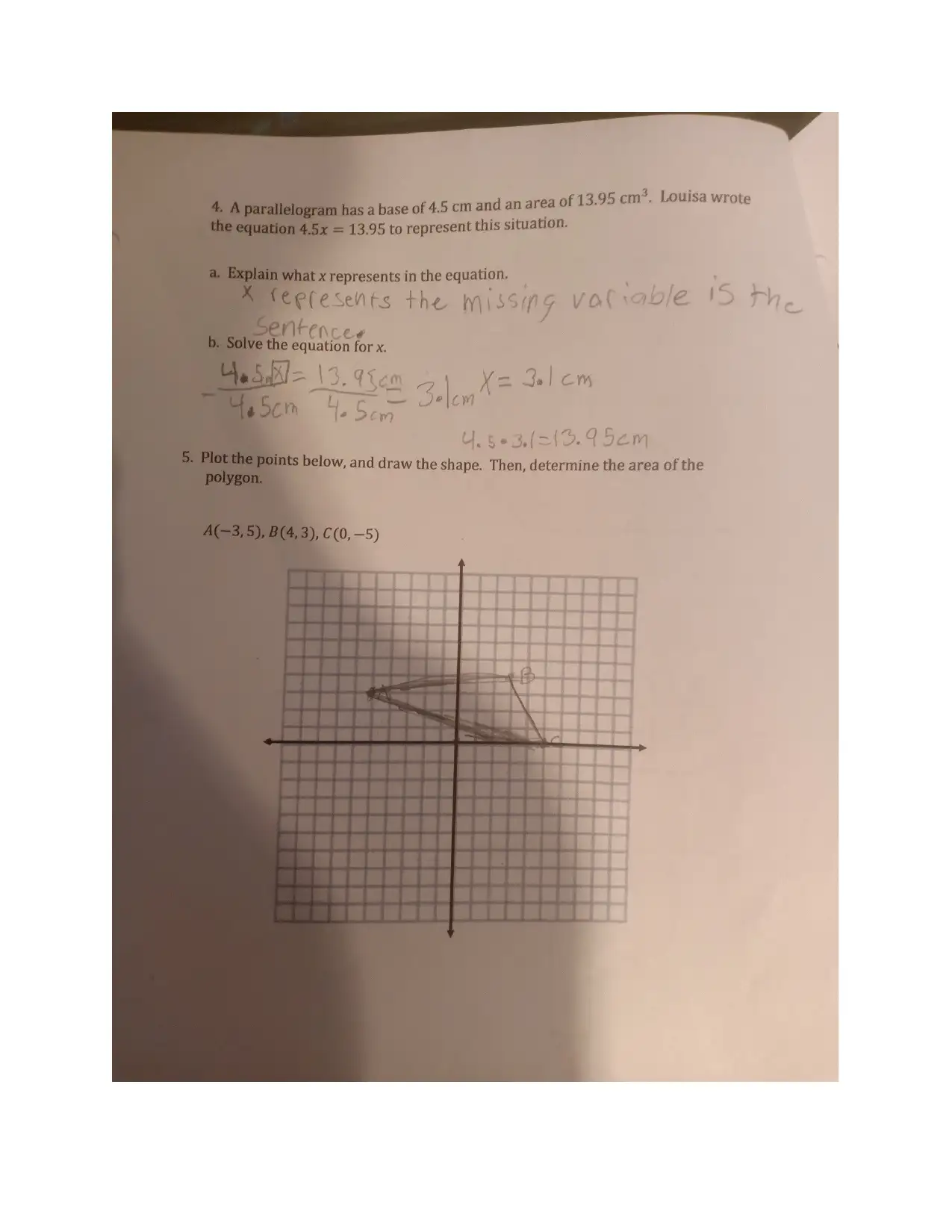
This document presents solutions to a variety of geometry problems. It includes calculations for the area of parallelograms and triangles, the area of composite figures, and the volume and surface area of cuboids. The solutions are step-by-step, providing clear explanations for each calculation. Furthermore, the assignment covers a problem involving coordinate geometry, calculating the area of a polygon given its vertices. Additional examples include real-world applications, like calculating the painting area and water volume of a swimming pool. This resource is designed to aid students in understanding and solving geometry problems related to area, volume, and coordinate geometry.
1 out of 9




![[object Object]](/_next/static/media/star-bottom.7253800d.svg)