Montgomery County Community College MAT 142 Exam 2 Solution
VerifiedAdded on 2021/04/22
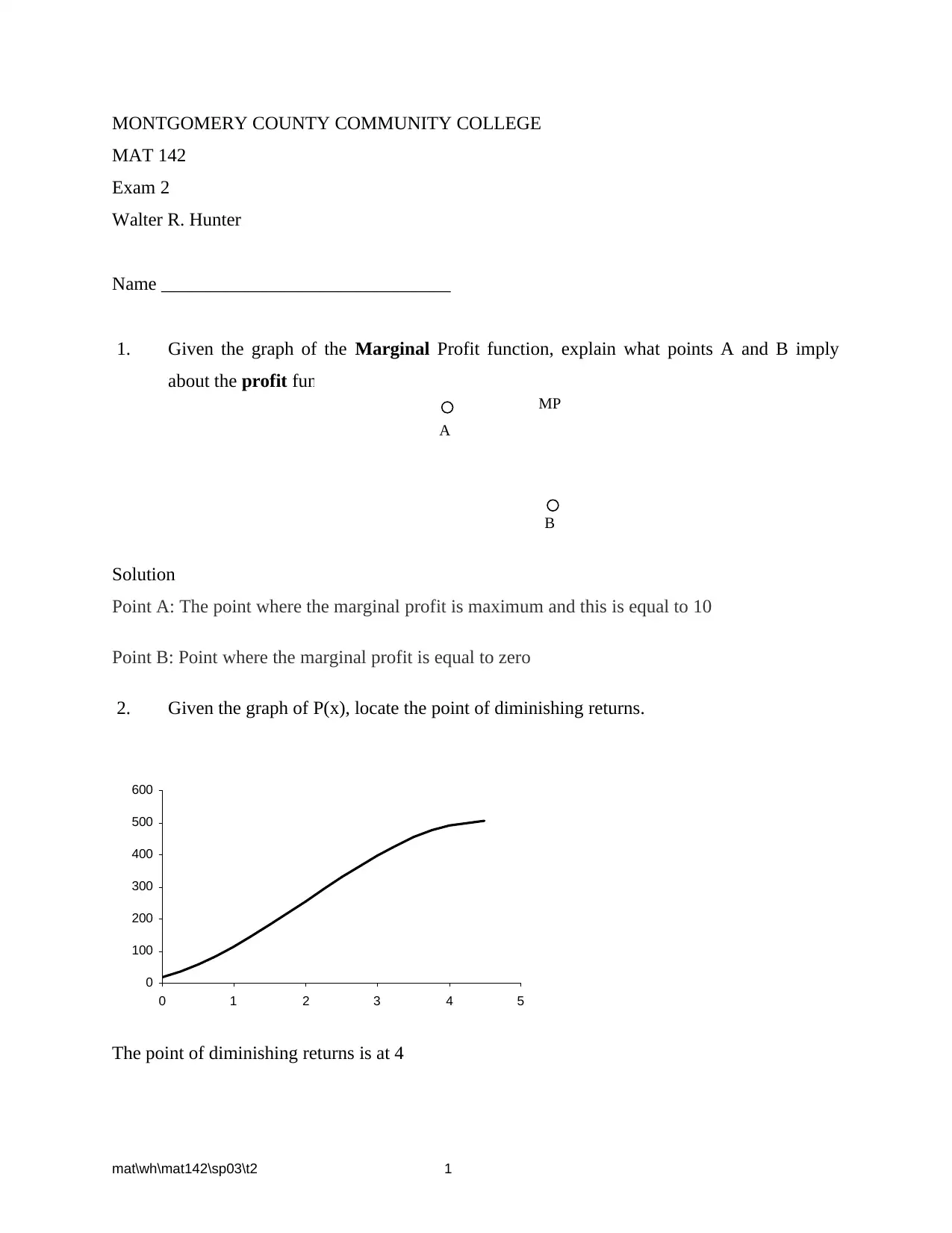
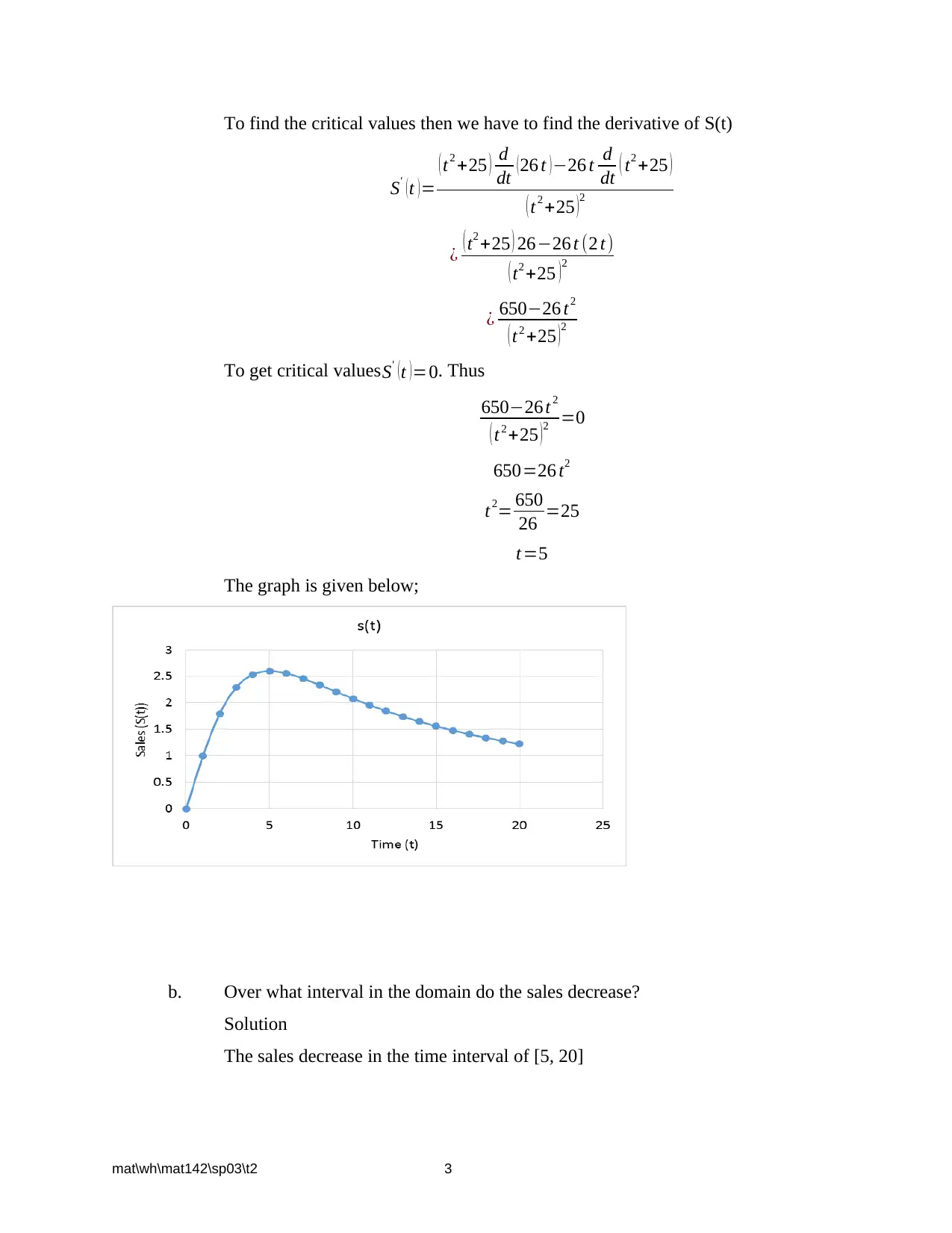
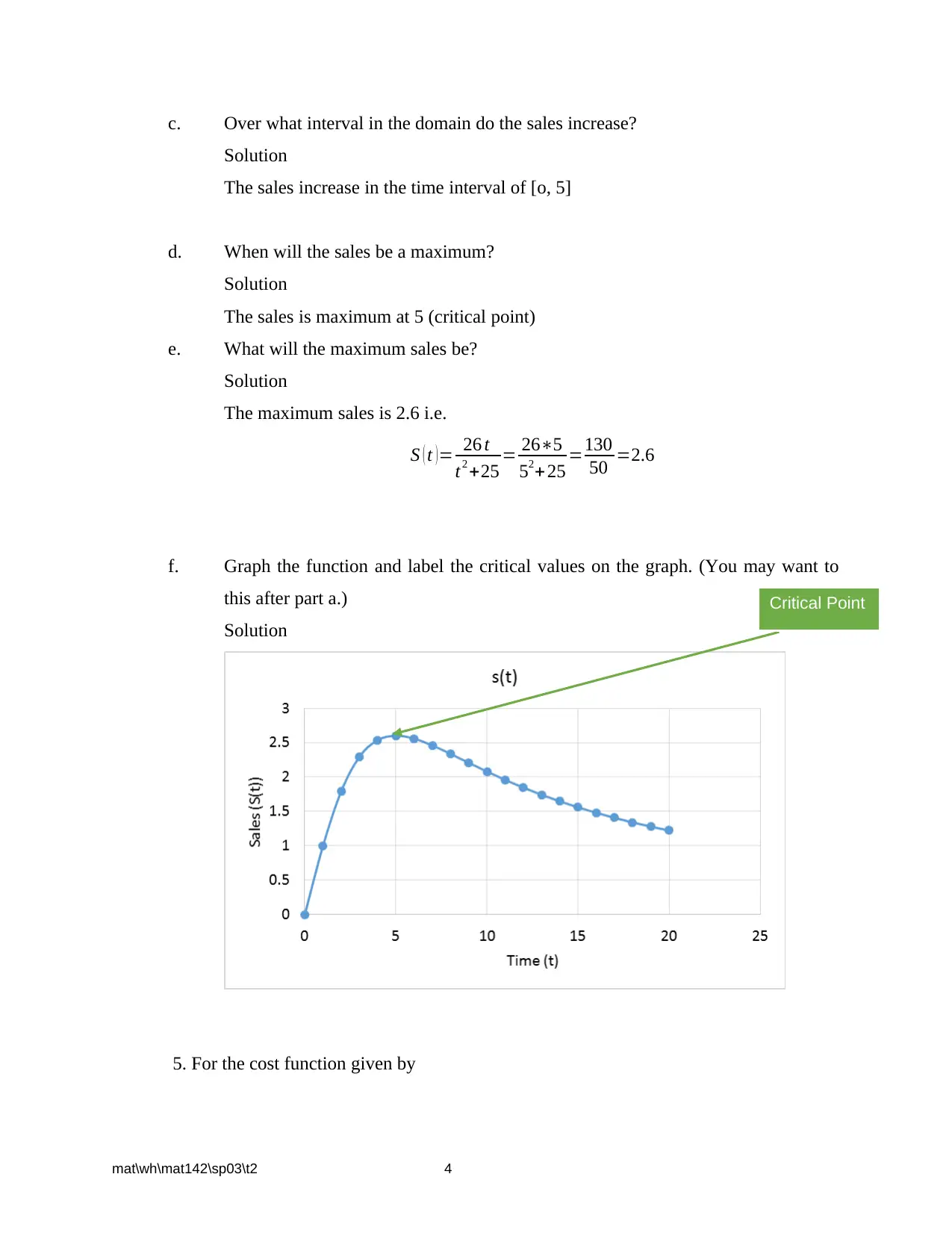
|8
|812
|49
Homework Assignment
AI Summary
This document presents a comprehensive solution set for the MAT 142 Exam 2, covering a range of calculus concepts and problem types. The solutions address questions related to marginal profit analysis, identifying points of diminishing returns from graphs and equations, and determining the implications of these points. The assignment includes problems involving cost and revenue functions, requiring the calculation of critical values, intervals of increase and decrease, and the maximization of sales and profit. Furthermore, the solutions demonstrate the application of calculus to optimize production and minimize costs, including problems related to production runs and inventory management. Detailed step-by-step solutions are provided for each problem, including graphical representations where applicable, ensuring a thorough understanding of the concepts and problem-solving techniques. This resource is designed to help students grasp the core principles of calculus and prepare for similar exams.
1 out of 8











![[object Object]](/_next/static/media/star-bottom.7253800d.svg)