MAT9004 Assignment 1 Solution - Mathematics, University

This document presents the complete solution to MAT9004 Assignment 1, a mathematics assignment. The solution includes answers to a quiz section and detailed workings with explanations for problem-solving questions. The assignment covers topics such as finding the inverse of a function, concavity, derivatives, integrals, logarithmic functions, and growth rates. Furthermore, it involves applying Gaussian elimination, calculating eigenvalues and eigenvectors, and analyzing the behavior of systems over time. The solution provides step-by-step calculations and justifications for each step, making it a valuable resource for students studying mathematics.

MAT9004 Assignment 1
Solution 1
(a)
The largest possible interval containing 0 such that function, f ( x ) =( x−1)2 has an inverse is,
[−1 , ∞ ).
(b)
Option - A
Second derivative of the function, f ( x )=x3 + x2 + x+1, is positive for x >−1
3 =¿ concave up
¿> function will be concave up ∈interval ¿(Ans.)
(c)
a=1
(d)
Option – A
(e)
( i )⃗ v1∧⃗ v3 , ( ii )⃗ v2∧⃗ v3 , ( iii )⃗ v4 ∧⃗ v5
Solution 2
(a)
Rate of growth of algae is r -----------(1)
Let concentration of algae on 30th November be C
¿> concentratinnext day=C1=r C
Concentration on 2nd day ¿ C1=r ( r C )=r2 C
Concentration on 7th day (7th December)= ¿ C7=r7 C-------(2)
Given, C=10 mg/L and C7=700 mg/ L
¿>C7=700=r7 ×10
Taking log of both sides,
log 700=7 log r +log 10=¿ 2+ log 7=7 log r +1=¿ 7 log r=log 70

¿> r7=70=¿ r=7
√ 70=1.8348 (Ans.)
Percentage increase= C2−C1
C1
×100= ( r−1 ) ×100=83.48 %(Ans.)
(b)
Cf =Ci rt-----------------------(3)
Where, Ci, is the initial concentration,
Cf , is the final concentration,
t, is the time in days
r, is the growth rate
¿> logCf =¿ log Ci+t log r=¿ t=¿ ¿ ¿
¿>t=
log Cf
Ci
logr =
log 10Ci
Ci
logr = log 10
log r = 1
log r = 1
log 70
1
7
= 7
log70
t=3.793 days (Ans.)
(c)
Cf = 6 ×1024 ×103
30 ×103 ×106 =1
5 ×1018 gm /l
Ci=10 ×10−3 gm/l
t=¿
¿>t=73.225 days(Ans.)
(d)
Cf =( 1
10 ) 6 ×1024 ×103
30 × 103 × 106 = 1
50 ×1018 gm/ l
t=¿
¿>t=69.43 days (Ans.)
(e)
Cf =r12 ×700=(1.8348)12 × 700=1018 ×103 mg=1018 gm ≅ 1 kg(Ans)

Solution 3
(a)
Given, f ( x ) =−( 1
4 ) x4 +a x3+b x2 + cx+ 644------------(1)
Also, f(12)=f(-12)----------------------------------------------------(2)
f ( 12 ) =−5184+1728 a+144 b+12 c+ 64 4=1728 a+ 144 b+12 c−4540-------------(3)
f (−12 )=−1728 a+144 b−12 c−4540--------------------(4)
Putting values of f ( 12 ) and f ( −12 ) from equations (3) and (4) in equation (2), we get,
1728 a+12 c=−1728 a−12 c=¿ 2 ( 12c +11728 a ) =0
¿> c+144 a=0--------------------------------------------------(5)(ans-i)
Given f(8)=2500----------------------------------------------------(6)
¿>−1024+512 a+64 b +8 c +644=2500=¿ 8 c+64 b+512 a=2880
¿> c+ 8 b+64 a=360-----------------------------------------(7)(ans-ii)
Given x=8 is the stationary point of f(x)
¿> ´f (8)=0-------------------------------------------------------(8)
´f (x)=−x3 +3 a x2 +2 bx+ c
¿> ´f (8)=−512+ 192a+ 16 b+c=0
¿> c+16 b +192 a=512------------------------------------(9)(ans-iii)
(b)
Applying Gaussian elimination method and using equations 5, 7 and 9, we get,
[ 1 0 144
1 8 64
1 16 192 ] [ c b a ] =
[ 0
360
512 ]
R2−R1 → R2 ; R3 −R1 → R3
[1 0 144
0 8 −80
0 16 48 ] [c b a ] = [ 0
360
512 ]
R3−2 R1 → R3

[ 1 0 144
0 8 −80
0 0 208 ] [ c b a ] = [ 0
360
−208 ]
( 1
8 ) R2
→ R2 ;( 1
208 ) R3 → R3
[1 0 144
0 1 −10
0 0 1 ] [ c b a ] = [ 0
45
−1 ]---------------------(10)
From equation 10, we get,
a=−1----------------------------------------------------(11)
b−10 a=45=¿ b=45+10 a
Putting the value of a from equation 11, we get
b=45+10× (−1 )=35----------------------------(12)
c +144 a=0
Putting here the value of a from equation 11, we get
c=−144 × ( −1 ) =144----------------------------(13)
(c)
Given ´f (x)= ( x−8 ) p (x)----------------------------(14)
From equations 1, 11, 12 and 13 we get,
f ( x )= (−1
4 ) x4−x3 +35 x2 +144 x +644
¿> ´f (x )=−x3−3 x2+ 70 x +144= ( x−8 ) p(x )
Dividing ´f (x) by ( x −8 ) , we get
p ( x )=−( x2 +11 x+18)----------------------(15)
p ( x )=− ( x +9 ) ( x+ 2 )
¿> ´
f ( x ) =− ( x−8 ) ( x +9 ) ( x+ 2 )
¿> stat ionary point of f ( x ) are x=8 , x=−2∧x =−9-------------(16)
f '' ( x )=−3 x2−6 x+70-----------------------(16)
f '' ( 8 )=−170 which isnegative , hence local maxima--------------------(17)

f '' (−2 )=70 which is positive, hence local minima------------------------(18)
f '' (−9 ) =−119 which is negative , hence local maxima------------------(19)
(d)
Given f ( 8 )=2500--------------------------------(20)
Putting x=-9 in equation 1, we get
f ( −9 ) = ( −1
4 ) (−9 ) 4 − ( −9 ) 3 +35 ( −9 ) 2+144 ( −9 ) +644=1271.75------(21)
As we can see that f ( 8 ) >f ( −9 ) =¿ x=8 is the global maxim
Putting x=-2 in equation 1, we get minimum rate of consumption is 500 units.
(e)
Total energy consumed in a day = ∫
−12
12
f ( x ) dx
Using value of f ( x ) from equation 1, we get,
∫
−12
12
f ( x ) dx=(−x5
20 − x4
4 + 35 x3
3 + 144 x2
2 +644 x ) ]−12
12
∫
−12
12
f ( x ) dx= ( 20630.4− (−10262.4 ) ) =30892.8 units------------------(22)
Average energy consumed in a day is 30892.8
24 =1287.2units per hour(Ans.)
Solution 4
(a)
Year Well-Behaved Naughty
1 a1K b1K
2
1/2(a1K+(b1K/2))
+b1K/2
=1/2a1K+3/4b1K
1/2(a1K+b1K/
2)
n+1 anK/2+3bnK/4 anK/2+bnK/4
[an +1
bn +1 ]= [K /2 3 K /4
K /2 K /4 ] [an
bn ]=K [1/2 3 / 4
1/2 1 / 4 ] [an
bn ] for n ≥ 0

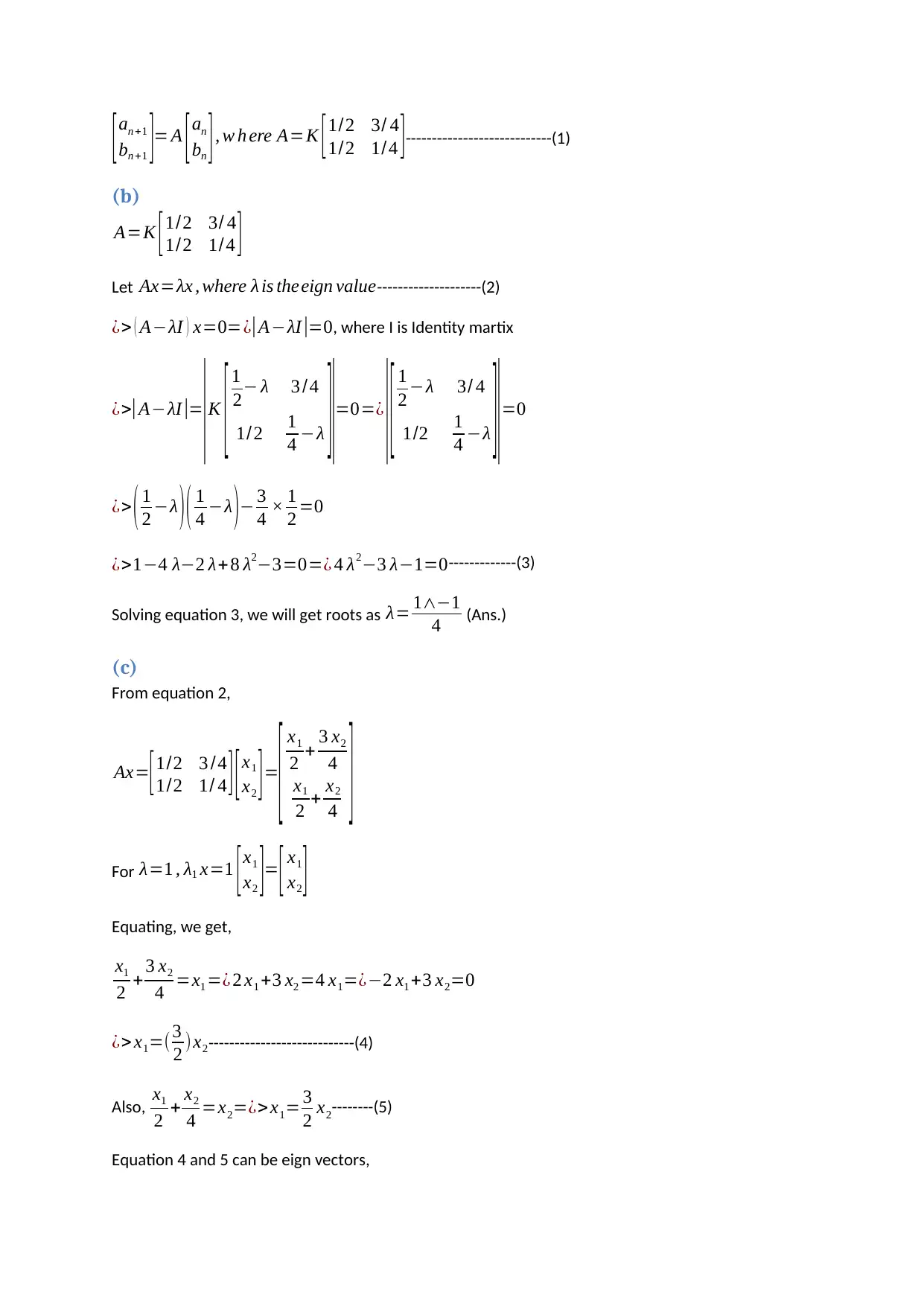
[an +1
bn +1 ]= A [an
bn ], w h ere A=K [1/2 3/ 4
1/2 1/4 ]----------------------------(1)
(b)
A=K [ 1/2 3/ 4
1/2 1/4 ]
Let Ax=λx , where λ is theeign value--------------------(2)
¿> ( A−λI ) x=0=¿|A−λI |=0, where I is Identity martix
¿>|A−λI |=
|K
[ 1
2− λ 3 /4
1/2 1
4 −λ ]|=0=¿
|[ 1
2 −λ 3/ 4
1/2 1
4 −λ ]|=0
¿> ( 1
2 −λ )( 1
4 −λ )− 3
4 × 1
2 =0
¿>1−4 λ−2 λ+ 8 λ2−3=0=¿ 4 λ2−3 λ−1=0-------------(3)
Solving equation 3, we will get roots as λ= 1∧−1
4 (Ans.)
(c)
From equation 2,
Ax=[ 1/2 3 /4
1/2 1/ 4 ] [ x1
x2 ] =
[ x1
2 + 3 x2
4
x1
2 + x2
4 ]
For λ=1 , λ1 x=1 [x1
x2 ]= [ x1
x2 ]
Equating, we get,
x1
2 + 3 x2
4 =x1 =¿ 2 x1 +3 x2 =4 x1=¿−2 x1 +3 x2=0
¿> x1=(3
2 )x2----------------------------(4)
Also, x1
2 + x2
4 =x2=¿>x1= 3
2 x2--------(5)
Equation 4 and 5 can be eign vectors,

[ ( 3
2 ) t
t ]=t [ 3
2 ] , one such eignvector isu1=
[ 3
2 ](Ans.)
Similarly for λ=−1/4, we will get equations,
x1
2 +( 3
4 ) x2=−x1
4 =¿ x1=−x2---------(6)
And, x1
2 + x2
4 =−x2
4 =¿ x1=−x2--------(7)
¿> eignvectors will be t [−1
1 ]∧one such eignvector will be u2= [ −1
1 ](Ans.)
(d)
If A=PD P−1, where D is the diagonal matrix, then,
P= [3 −1
2 1 ]∧D= [1 0
0 −1/4 ]
P−1= 1
3− (−2 ) [ 1 1
−2 3 ]=1
5 [ 1 1
−2 3 ]= [ 0.2 0.2
−0.4 0.6 ]
(e)
An =P Dn P−1
A=K
[ 1
2
3
4
1
2
1
4 ] =¿ An =Kn
[ 1
2
3
4
1
2
1
4 ] n
=P Dn P−1
An =1
5 [3 −1
2 1 ] [1 0
0 −1
4 ]n
[ 1 1
−2 3 ]= 1
5 [3 −1
2 1 ] [1 0
0 (−1
4 )n
] [ 1 1
−2 3 ]
¿> An= 1
5 [3+ 2(−1
4 )
n
3−3(−1
4 )
n
2−2(−1
4 )
n
2+3 (−1
4 )
n
]
For very large n,
An = K
5 [ 3 3
2 2 ]

[an
bn ]=An
[a1
b1 ]= K
5 [3 3
2 2 ] [a1
b1 ]
¿> an= K
5 (3 a1 +3 b1 )
And bn= K
5 (2 a1+2 b1 )(Ans.)
(f)
Let, number of well-behaved kids be a1 ,and number of naughty kids be b1
¿> Santa gave gifts¿ 0.9 a1+ 0.4 b1
Next year 0.1a1kids who were well-behaved but did not receive the gift, turned into naughty kids. In
addition to the number of naughty kids next year according to old case, that is, 50% of gift receiving
kids also turn as naughty kids. So, proportion of naughty kids next year is,
b2=0.1 a1 + 0.9 a1+ 0.4 b1
2
50% of gift receiving kids remain well-behaved and 60% of naughty kids who did not receive gifts
turned well-behaved. Therefore, proportion of well-behaved kids next year will be,
a2= 0.9 a1+ 0.4 b1
2 +0.6 b1
a2∧b2 can be simplified as under,
a2=0.45 a1 +0.8 b1
b2=0.55 a1 +0.2 b1
¿> [an +1
bn +1 ]= [0.45 0.8
0.55 0.2 ] [an
bn ]
A=
[ 0.45 0.8
0.55 0.2 ]
( A−λI ) x=0=¿ (0.45− λ 0.8
0.55 0.2−λ )=0
¿> ( 0.45−λ ) ( 0.2−λ ) −0.8 ×0.55=0
Solving for λ, we get eign-values as 1 and -0.35
For λ=1 , we willeign-vector, u1= [ 16
11 ]

And for λ=−0.35 , u2= [ −1
1 ]
Therefore, P= [ 16 −1
11 1 ] ∧P−1= [ −1 1
−11 16 ]
We know An =P Dn P−1= [ 16 −1
11 1 ] [ 1n 0
0 (−0.35)n ] [ −1 1
−11 16 ]
¿> An= [ −16+(−0.35)n 16(1− ( −0.35 ) n )
−11( 1+ ( −0.35 ) n ) 11+16(−0.35)n ]
For very large n
An ≅ [−16 16
−11 11 ]
an+1=−16 ( a1 +b1 )
an+1=−11 ( a1+ b1 )
Since 11<16, the proportion of naughty kids becomes smaller when n is very large (Ans.)