Mathematics Assignment Solution: Calculus Units 2-4, 2020

This document presents a comprehensive solution to a mathematics assignment focusing on calculus, specifically covering units 2-4. The assignment encompasses a wide range of calculus concepts, including trigonometric functions, domains of functions, quadratic equations, stationary points, limits, continuity, derivatives (chain rule, quotient rule, product rule), and asymptotes. Solutions are provided for each question, with detailed explanations and steps to derive the answers. The assignment explores various aspects of function analysis, such as finding roots, sketching graphs, determining continuity, and calculating limits using various techniques. The document is a valuable resource for students studying calculus, offering insights into problem-solving approaches and a deeper understanding of the subject matter.

Mathematics Assignment:
Student Name:
Instructor Name:
Course Number:
8th January 2020

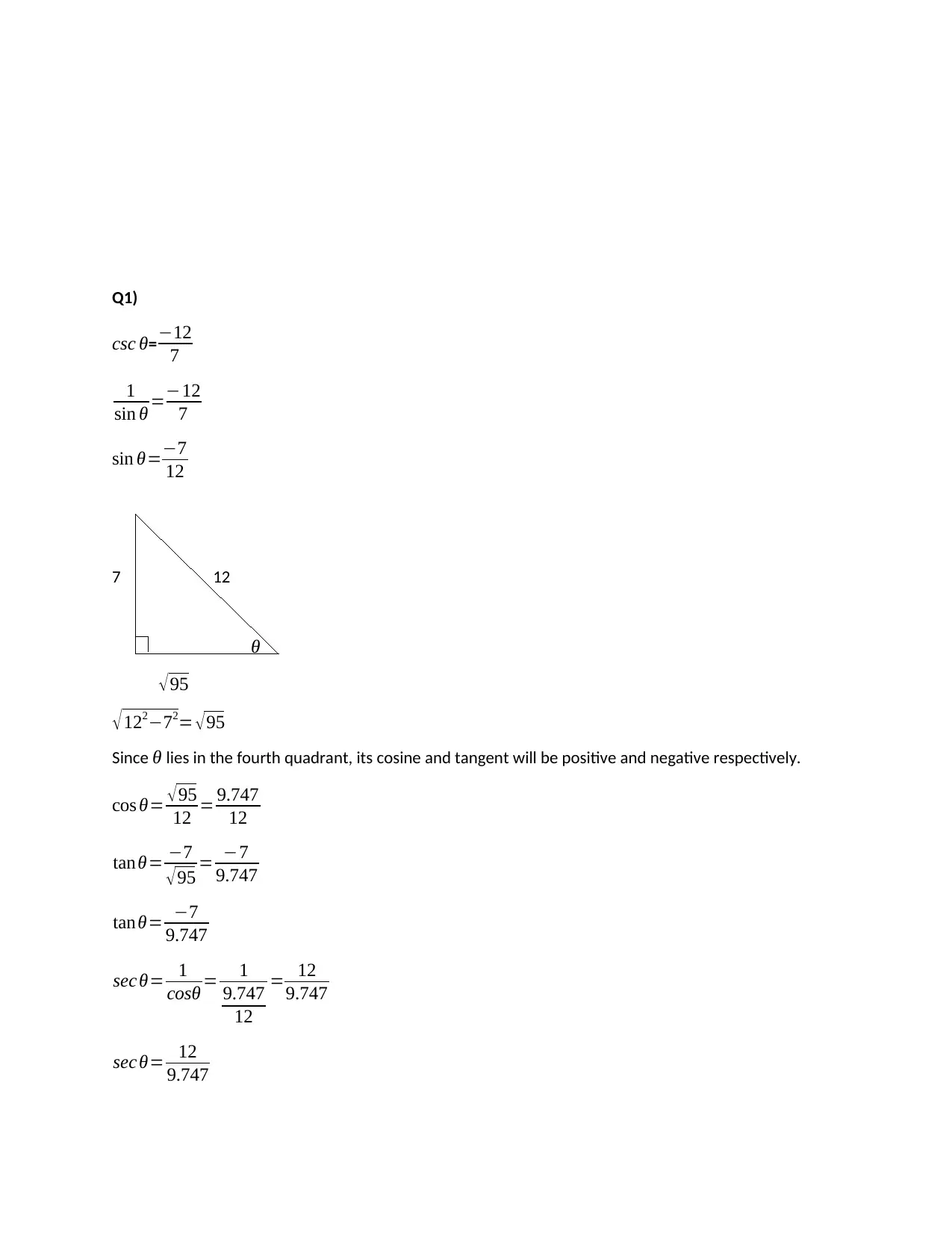
Q1)
csc θ= −12
7
1
sin θ =−12
7
sin θ=−7
12
7 12
θ
√ 95
√122−72= √95
Since θ lies in the fourth quadrant, its cosine and tangent will be positive and negative respectively.
cos θ= √95
12 = 9.747
12
tanθ= −7
√ 95 = −7
9.747
tanθ= −7
9.747
sec θ= 1
cosθ = 1
9.747
12
= 12
9.747
sec θ= 12
9.747

cot θ= 1
tanθ = 1
−7
9.747
=−9.747
7
cot θ=−9.747
7
Q2a)
C ( x )=0.3456 x
C ( 35 )=0.3456 ( 35 )=12.096
C ( 35 )=12.096
b)
Domain of C ( x ) is from x=0 to x <75
Q3a)
The function g touches the x axis twice at points (-1, 0) and (5, 0). We may therefore say that it
is a quadratic equation in x with roots x=−1∧x=5.Therefore we have
( x +1 ) ( x−5 )=0
¿ x2−4 x−5=0
Assuming that g takes the form g( x )=a x2 +bx+ c we get
g ( x )=x2−4 x−5
The function f is a curve with stationary point at (3 , 0).
Assuming that f takes the form f ( x)=a x2 +bx +c.
At the stationary point f ' ( x )=2 ax +b=0
f ' ( 3 ) =2 a(3)+b=0
6 a+b=0 ………………………………………..i
At (3, 0)
f ( 3 )=a (3)2 +3 b+ c
0=9 a+3 b+c ……………………………ii

f Also passes through (4 ,−1)
f ( 4 ) =a(4)2 +4 b+c
−1=16 a+4 b+c ……………………………iii
iii minus ii gives 7 a+ b=−1
7 a+ b=−1 … …… … …… . iv
Solving i and iv simultaneously by taking iv minus i we have
a=−1
7 a+ b=−1
7(−1)+b=−1
b=−1+7=6
−1=16 (−1)+ 4 (6)+ c
−1+16−24=c
−9=c
f ( x ) =a x2 +bx +c=−x2 +6 x−9
f ( x )=−x2 +6 x−9
f ( x )=−x2 +6 x−9
g ( x )=x2−4 x−5
g ( x )=x2−4 x−5
g ( 1 )=12−4 ( 1 )−5=−8
g ( 1 )=−8
f ( x )=−x2 +6 x−9
f ( 1 ) =−12+ 6 ( 1 )−9=−4
f ( 1 ) =−4
( f ° g ) ( 1 )=−8 ×−4=32
b)
g ( x )=x2−4 x−5

g ( 4 ) =42−4 ( 4 ) −5=−5
g ( 4 ) =−5
f ( x )=−x2 +6 x−9
f ( 4 )=−42 +6 ( 4 )−9=−1
f ( 4 )=−1
( g ° f ) ( 4 )=−5 ×−1=5
c)
g ( x )=x2−4 x−5
g (−2 )=(−2)2−4 (−2 )−5=7
g (−2 )=7
f ( x )=−x2 +6 x−9
f (−2 )=−(−2)2 +6 (−2 ) −9=−25
f ( −2 ) =−25
( f ° g ° g ) ( −2 ) =−25× 7 ×7=−1225
Q4)
y=(x2−1)
1
3
Let u=x2−1
du
dx =2 x
y=(u)
1
3
dy
du = 1
3 u
−2
3
dy
dx = dy
du . du
dx =(2 x) 1
3 u
−2
3

dy
dx = 2
3 xu
−2
3 = 2
3 x (x2−1)
−2
3
dy
dx = 2
3 (x2−1)
2
3
Let 3( x2−1)
2
3 =0
x2−1=0
x2=1
x=1∨−1
The largest set is (−1,1)
Q5)
3
√ 12=(12)
1
3
Let y=x
1
3
Where x=8 and ∆ x=4
dy
dx =1
3 x
−2
3 = 1
3 x
2
3
We also that ∆ y = dy
dx ∆ x
∆ y = 1
3 x
2
3
∆ x
∆ y = 1
3 ( 8 )
2
3
( 4)
∆ y = 1
3 (2)3 × 2
3
(4)
∆ y = 1
3 (2)2 (4 )
∆ y = 1
3 ( 4 ) ( 4 ) = 4
12 =0.3333

We have
∆ y =f ( x+ ∆ x)
1
3 −x
1
3
0.3333=(8+4)
1
3 −8
1
3
0.3333=(12)
1
3 −8
1
3
(12)
1
3 =0.3333+8
1
3
(12)
1
3 =0.3333+( 2)3 × 1
3
(12)
1
3 =0.3333+2=2.3333
3
√12=2.3333
Q6)
a)
The graph of function h has the following roots;
x=−3 ,−1,3,1
The equation of h can be obtained as shown below
h=(x +3)( x +1)( x−3)( x −1)
( x +3 ) ( x+1 ) =x2 + 4 x+ 3
( x−3 ) ( x−1 ) =x2−4 x +3
h=(x ¿¿ 2+4 x+3)(x ¿¿ 2−4 x +3)¿ ¿
h=x4−10 x2+ 9
F (x)=h ( x
2 )+ 1
F (x)=(x ¿¿ 4−10 x2+9)( x
2 )+1 ¿
F ( x )= 1
2 x5−5 x3+ 9
2 x+1
x -3 -2 -1 0 1 2 3

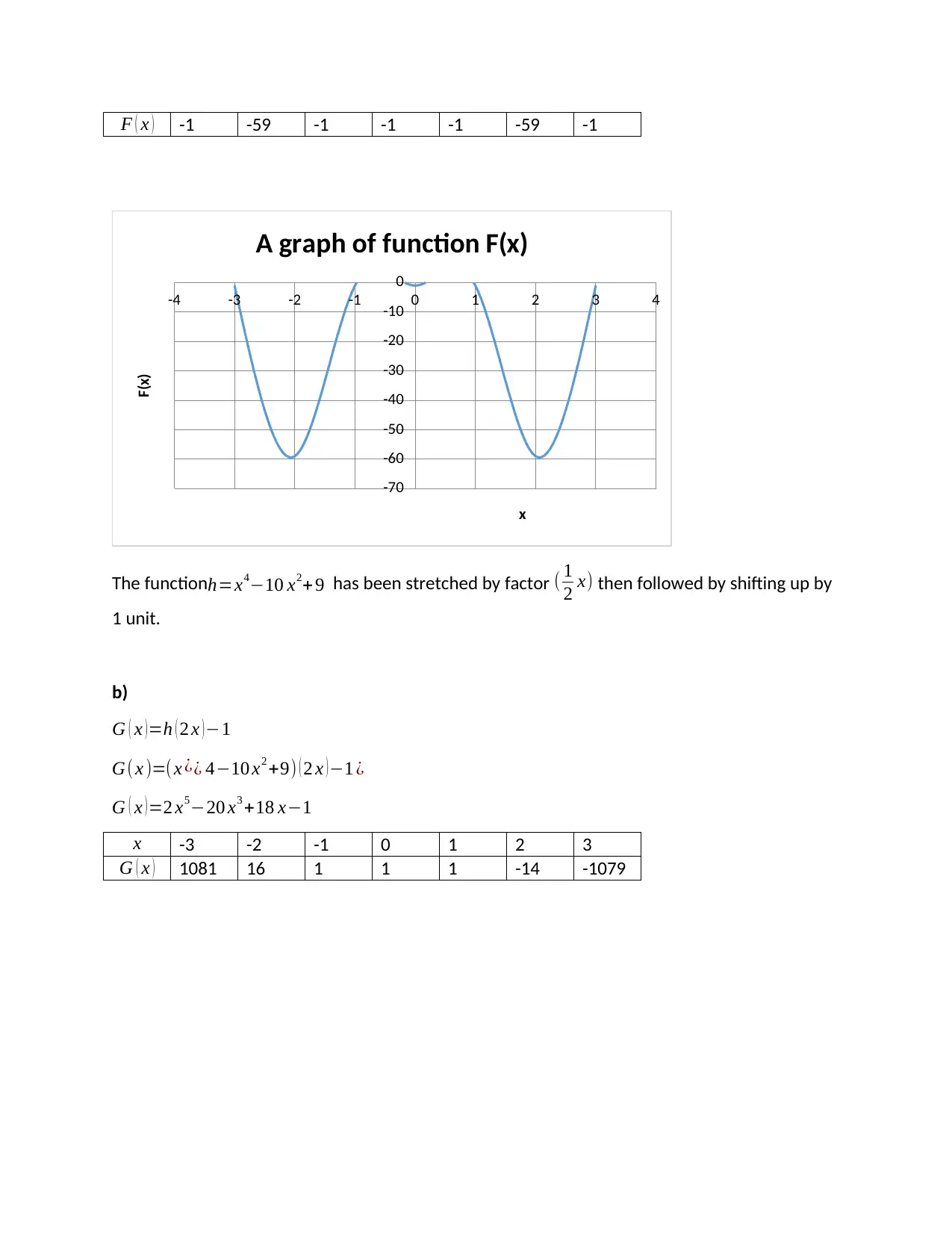
F ( x ) -1 -59 -1 -1 -1 -59 -1
-4 -3 -2 -1 0 1 2 3 4
-70
-60
-50
-40
-30
-20
-10
0
A graph of function F(x)
x
F(x)
The functionh=x4−10 x2+ 9 has been stretched by factor ( 1
2 x) then followed by shifting up by
1 unit.
b)
G ( x )=h ( 2 x )−1
G( x )=( x ¿¿ 4−10 x2 +9) ( 2 x ) −1 ¿
G ( x ) =2 x5−20 x3 +18 x−1
x -3 -2 -1 0 1 2 3
G ( x ) 1081 16 1 1 1 -14 -1079

-4 -3 -2 -1 0 1 2 3 4
-1500
-1000
-500
0
500
1000
1500
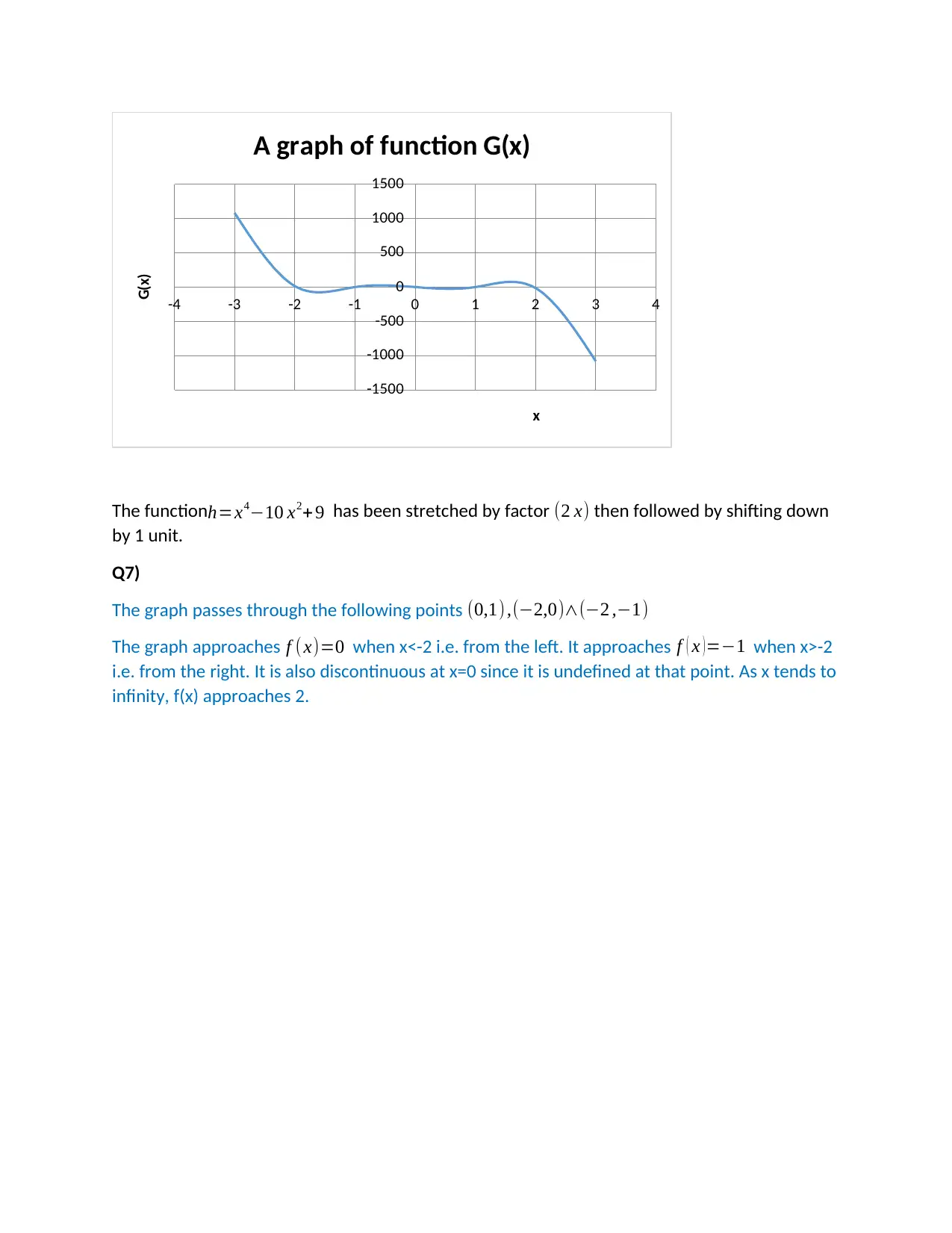
A graph of function G(x)
x
G(x)
The functionh=x4−10 x2+ 9 has been stretched by factor (2 x) then followed by shifting down
by 1 unit.
Q7)
The graph passes through the following points (0,1) ,(−2,0)∧(−2 ,−1)
The graph approaches f ( x)=0 when x<-2 i.e. from the left. It approaches f ( x )=−1 when x>-2
i.e. from the right. It is also discontinuous at x=0 since it is undefined at that point. As x tends to
infinity, f(x) approaches 2.

-3 -2 -1 0 1 2 3
-3
-2
-1
0
1
2
3
4
5
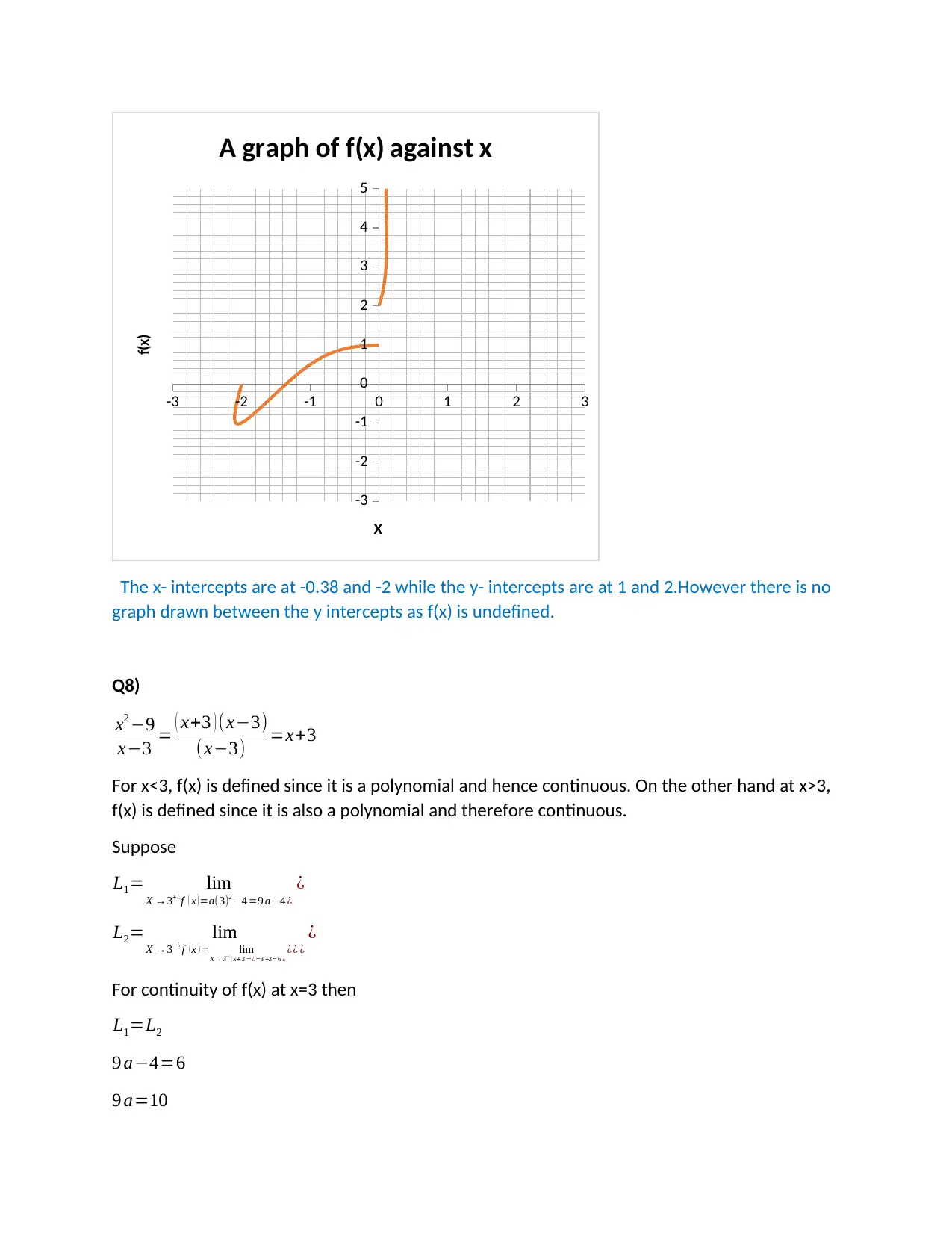
A graph of f(x) against x
X
f(x)
The x- intercepts are at -0.38 and -2 while the y- intercepts are at 1 and 2.However there is no
graph drawn between the y intercepts as f(x) is undefined.
Q8)
x2 −9
x−3 = ( x+3 ) (x−3)
(x−3) =x+3
For x<3, f(x) is defined since it is a polynomial and hence continuous. On the other hand at x>3,
f(x) is defined since it is also a polynomial and therefore continuous.
Suppose
L1= lim
X →3+ ¿f ( x ) =a(3)2−4 =9 a−4 ¿
¿
L2= lim
X →3−¿ f ( x ) = lim
X→ 3−¿ ( x+ 3 )=¿=3 +3=6 ¿
¿¿ ¿
¿
For continuity of f(x) at x=3 then
L1=L2
9 a−4=6
9 a=10

a= 10
9 =1 1
9
At x=3
f ( x)=b2
f ( 3 ) =b2 =0
b=0
Thus we have
f ( x )=
{ x2−9
x−3 , x <3
10
9 x2 , x >3
0 x=3
Q9)
a)
Using quotient law of limit we have
lim
x→ a ( f
g )=¿
lim
x→ a
f
lim
x→ a
f ¿
lim
x→ 0−¿
( sinx
g(x) )=¿ lim
x →0−¿ sinx
¿ lim
x→ 0−¿ g(x)
¿ sin 0
∞ = 0
∞ =0 ¿¿ ¿¿
¿
lim
x→ 0−¿
( sinx
g(x) )=0 ¿
¿
b)
lim
x→ 0+¿ g ( x ) tan (2 x)
tan(6 x) =¿¿ ¿
¿
= 4 × 0
0 =∞
There is no limit since we have 0 in the denominator hence making the function undefined.
c)

lim
x→ ∞ ( 1
g (x) )=
lim
x →∞
1
lim
x→ ∞
g(x )= 1
0 =∞
There is no limit since we have 0 in the denominator hence making the function undefined.
d)
lim
x →0 ( x−4
g(x ) ) =
lim
x→ ∞
x−4
lim
x →∞
g (x)= 0−4
4 =−4
4 =−1
Q10)
g( x )= x3−8
( x−2 ) (x2−x−12)
Let x=2
As x approaches 2, the denominator and the numerator both approach 0.
If x>2 , g(x )>0 Thus lim
x→ 2+¿ g ( x ) =∞ ¿
¿
If x<2 , g(x )<0 Thus lim
x→ 2−¿ g ( x ) =−∞ ¿
¿
Therefore x=2 is a vertical asymptote.
Let x=4
As x approaches 4, the denominator approaches 0.
If x>4 , g(x )>0 Thus lim
x→ 4+ ¿ g ( x ) =∞ ¿
¿
If x<4 , g(x )<0 Thus lim
x→ 4−¿ g ( x )=−∞ ¿
¿
Therefore x=4 is a vertical asymptote.
Let x=-3
As x approaches -3, the denominator approaches 0.
If x>−3 , g ( x)>0 Thus lim
x→−3+ ¿ g (x )=∞ ¿
¿
If x ←3 , g( x )>0 Thus lim
x→−3−¿ g ( x )=∞ ¿
¿

Therefore x=-3 is a vertical asymptote.
Q11)
The lines that are parallel have equal gradient.
y− √2 x=3
y= √2 x+ 3
The gradient of the parallel line is √2 and hence the tangent line will have the same gradient.
When x=a=−π
2
cos (2 x )=cos (2 a)=cos (-π ¿=−1
The tangent passes through the point ( −π
2 ,−1 ¿
((a , f (a) ¿=(a , cos (2 a))
Using the points ( −π
2 ,−1 ¿ and (a , cos (2 a)¿ having a gradient of √2
cos(2a)+1
a+ π
2
= √ 2
cos (2 a)+ 1= √2 (a+ π
2 )
cos (2 a)+1= √ 2 a+ √ 2
2 π
cos (2 a)= √2 a+ √ 2
2 π−1 ……………………………….i
When x=a= π
3
cos (2 x )=cos (2 a)=cos ( 2
3 π ¿=−1
2
The tangent passes through the point ( π
3 ,− 1
2 ¿
Using the points ( π
3 ,− 1
2 ) and (a , cos (2 a)¿ having a gradient of √ 2

cos( 2a)+ 1
2
a− π
3
= √ 2
cos (2 a)+ 1
2 = √2 (a− π
3 )
cos ( 2 a )+ ¿ 1
2 = √2 a− √ 2
3 π ¿
cos ( 2 a )= √2 a− √ 2
3 π −1
2 ……………………………….ii
Comparing i and ii we have
cos (2 a)= √ 2 a+ √ 2
2 π−1= √ 2 a− √ 2
3 π −1
2
cos (2 a)= √ 2
2 π −1=−√ 2
3 π −1
2
cos (2 a)= √ 2
2 π + √ 2
3 π −1+1
2 =0
cos (2 a)= √ 2
2 π + √ 2
3 π −1+1
2 =0
cos (2 a)= 5 √ 2
6 π− 1
2 =0
cos (2 a)= 5 √ 2
6 π− 1
2
cos (2 a)=0
Ignoring cos( 2a)=5 √ 2
6 π − 1
2 and using
cos (2 a)=0
cos (2 a)=cos ( π
2 ,− π
2 )
2 a= π
2 ,− π
2
a= π
4 ,− π
4

a= π
4 Or
a=−π
4
Q12)
a)
d
dx √sec ( 2 x )
We shall apply the chain rule
df (u)
dx = df
du . du
dx
Let f =√u and u=sec (2 x)
d
dx √sec ( 2 x )= d
dx ( √ u ) d
dx ( sec(2 x) )
¿ 1
2 √ u ( sec ( 2 x ) tan ( 2 x ) .2 )
¿ 1
2 √ sec (2 x ) ( sec ( 2 x ) tan ( 2 x ) .2 )
b)
d
dx ( sin x
x−3 x
2
3 )
We shall apply the Quotient rule
( f
g )'
= f ' . g−g' . f
g2
d
dx ( sin x
x−3 x
2
3 )=
d
dx ( sinx ¿ ) ( x−3 x
2
3 )− d
dx ( x−3 x
2
3 ) ( sinx ¿ )
( x−3 x
2
3 )2
=
( cosx¿ ) ( x−3 x
2
3 )−
(1− 2
x
1
3 ) ( sinx ¿ )
( x−3 x
2
3 )2

c)
d2
d x2 ( x3 tan x )
We shall apply the product rule
d
dx ( x3 tan x ) =3 x2 tan (x)+ sec2 ( x) x3
d
dx (3 x2 tan ( x ) + sec2 ( x ) x3 )
=2 x3 sec2 ( x ) tan ( x )+6 x2 sec2 ( x )+6 xtan( x )
Q13)
v h
45°
x
Let v ¿ represent speed of aircraft ∈the direction shown above while x∧h
horizontal∧vertical component of the speed of the aircraft respectively .
sin 45 °= h
v
h=v (sin 45 °)
v=500 mi /h
h=500(sin 45 ° )
√ 2 1

45°
1
( sin 45 ° ) = 1
√2
h=500(sin 45 ° )
h=500 ( 1
√ 2 )= 500
√2
h=500 ( 1
√ 2 )= 500
√2
Rationalizing the denominator we get
h=500
√2 × √2
√2 = 500 √ 2
2 =250 √2
The aircraft is gaining altitude at 250 √2mi /h