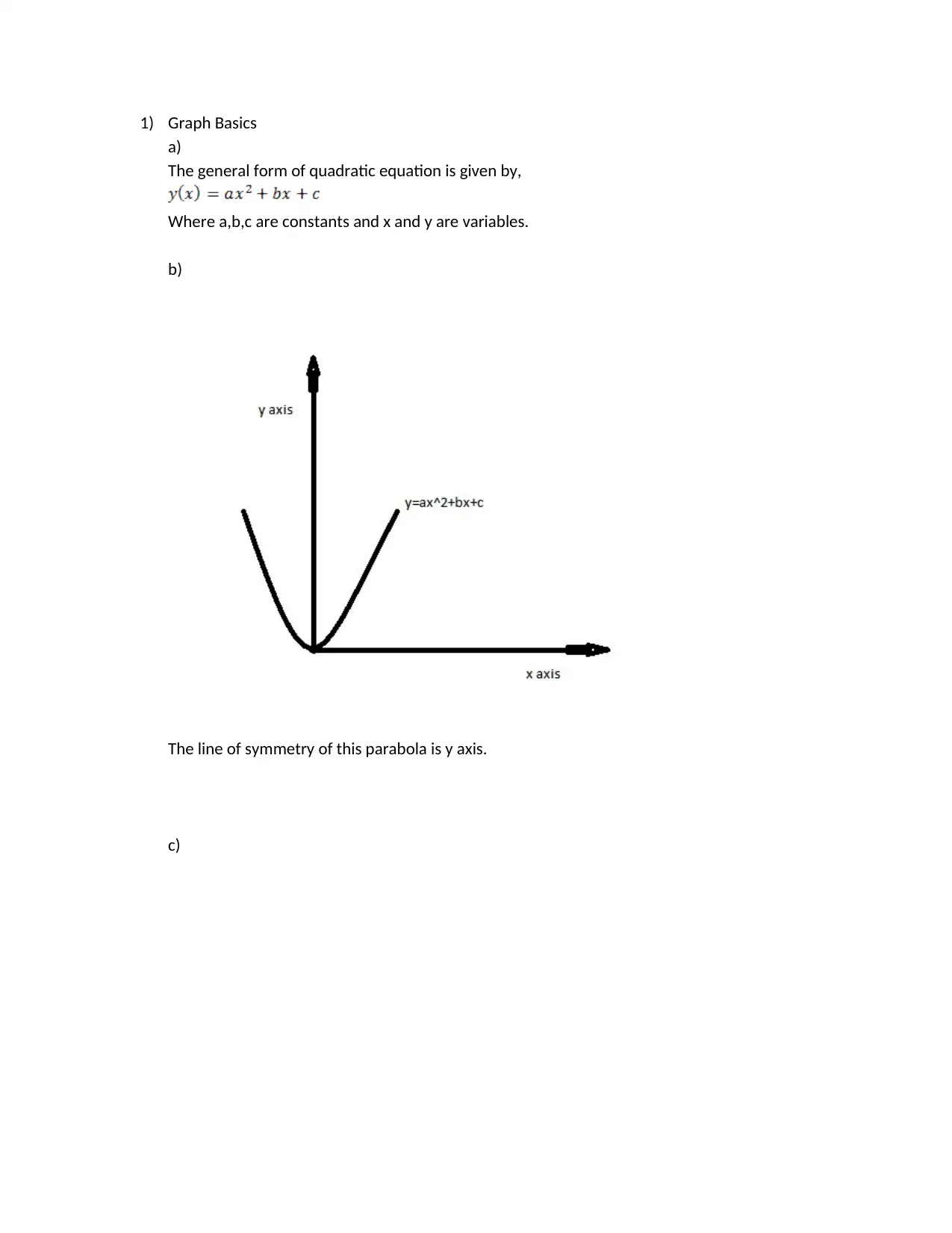
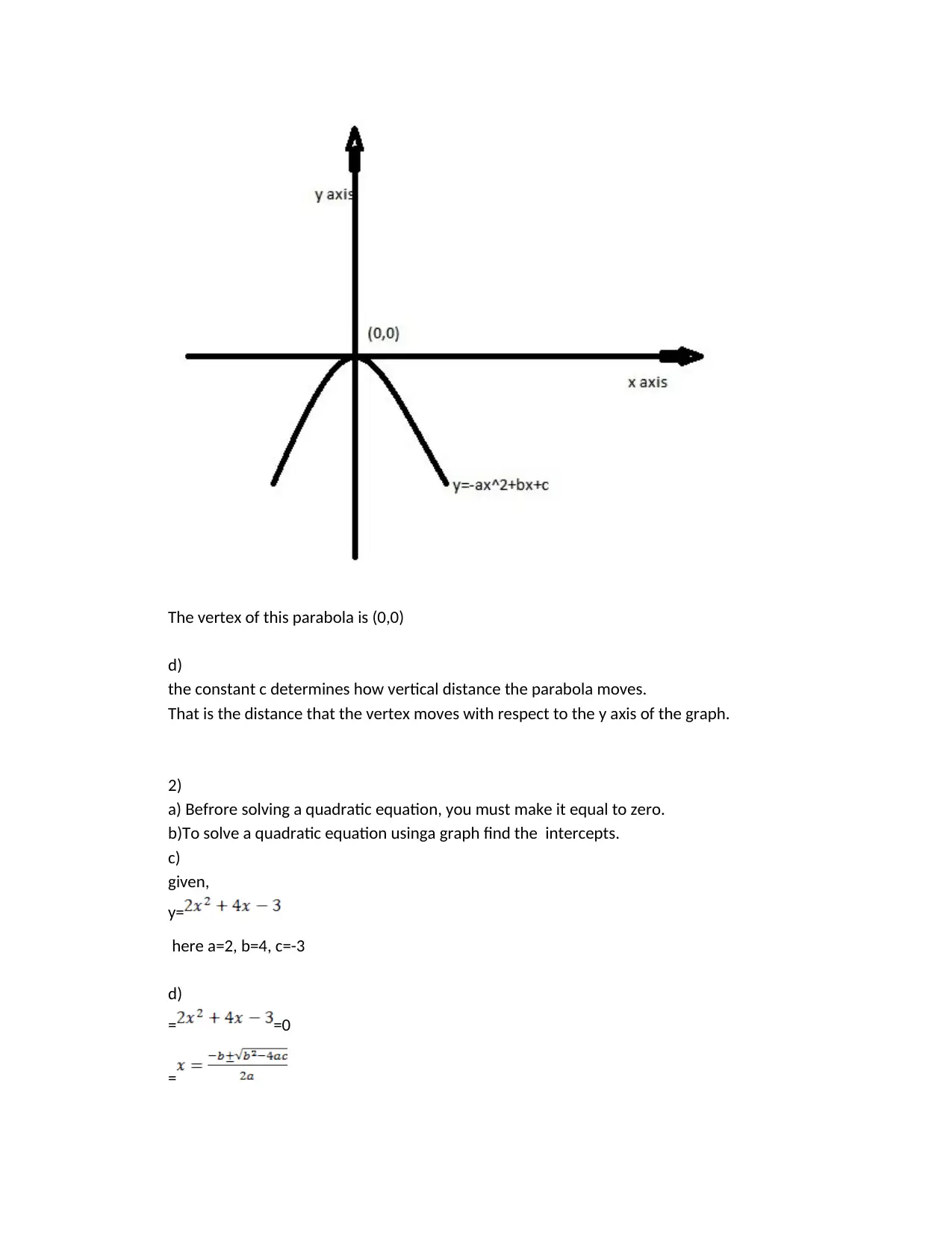
Quadratic Equations: Graphing, Solving, and Discriminant Analysis

This assignment solution addresses key concepts related to quadratic equations. It begins by defining the general form of a quadratic equation and identifying its components, including variables and constants. The solution then explores the graphical representation of quadratic equations, focusing on parabolas, the line of symmetry, and the vertex, as well as how the constant 'c' affects the graph's vertical position. The assignment progresses to solving quadratic equations, including setting the equation to zero and using the quadratic formula. The solution provides examples and calculations, including the discriminant and its role in determining the nature of the roots (real or complex). Finally, the assignment covers the relationship between the quadratic equation's coefficients and the graph's characteristics, such as the axis of symmetry and the vertex coordinates.

1) Graph Basics
a)
The general form of quadratic equation is given by,
Where a,b,c are constants and x and y are variables.
b)
The line of symmetry of this parabola is y axis.
c)

The vertex of this parabola is (0,0)
d)
the constant c determines how vertical distance the parabola moves.
That is the distance that the vertex moves with respect to the y axis of the graph.
2)
a) Befrore solving a quadratic equation, you must make it equal to zero.
b)To solve a quadratic equation usinga graph find the intercepts.
c)
given,
y=
here a=2, b=4, c=-3
d)
= =0
=

=
=x= , -1-
That is x=0.58,-2.58
e)
discriminant, D=
=
i)
if the discriminant is positive, there will be 2 values for x and there fore two separate roots
ii)
if the discriminant is zero, there is only one value for x, but there are in fact two roots which are
equal.
iii)
if the discriminant is negative, there is no real value for x. The value for the roots is a complex
number.
3.
a)
For a quadratic equation in standard for, y= .
the axis of symmetry is a vertical line
b)the y cordinate of the vertex is found out by substituting the above value of x the general
quadratic equation.
That is,
=
=
Therefore the cordinates = (
c)
i)does the graph turn up or down
ii)find the vertex
iii)find the intercepts
iv)graph the parabola