Reaction Rates and Equilibrium: A Comprehensive Chemistry Homework

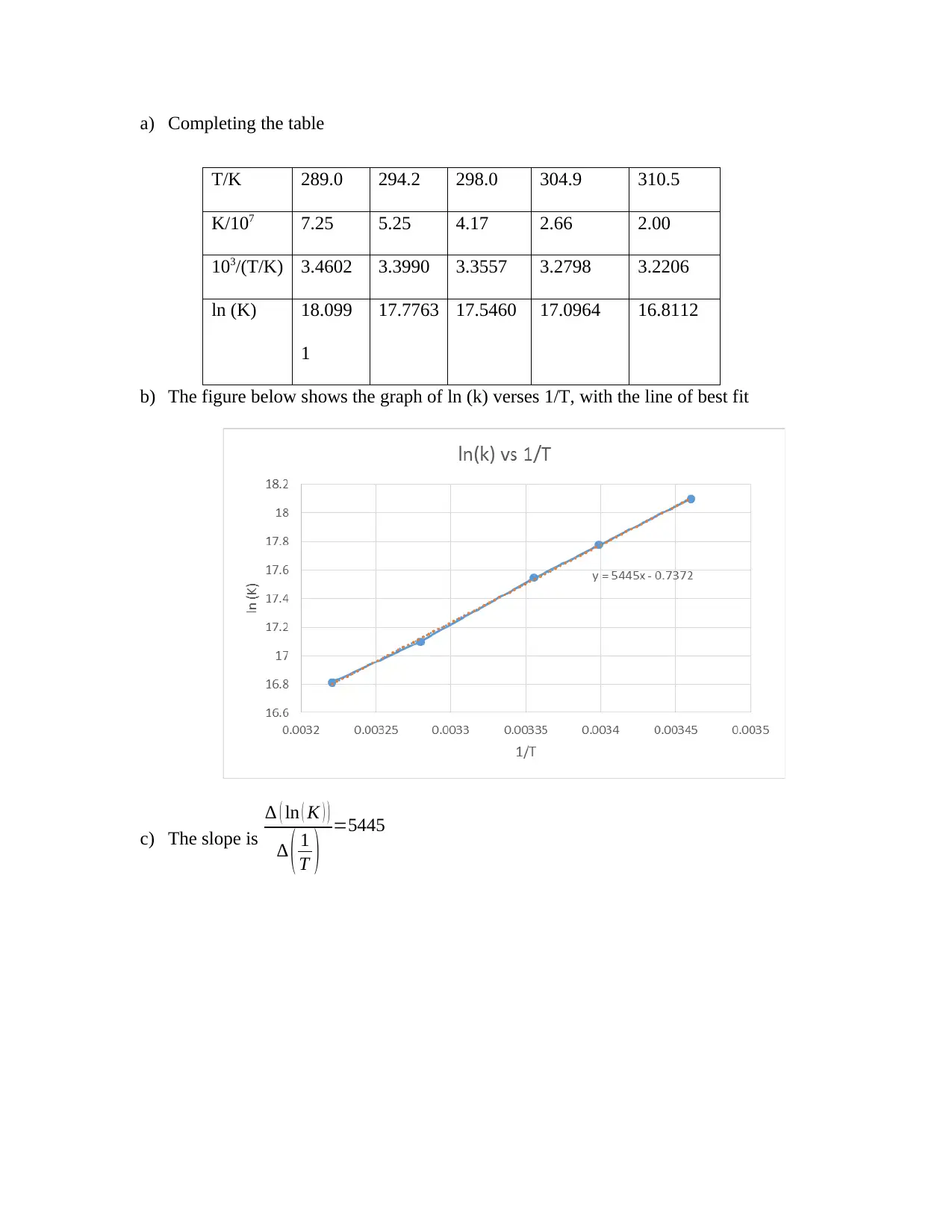
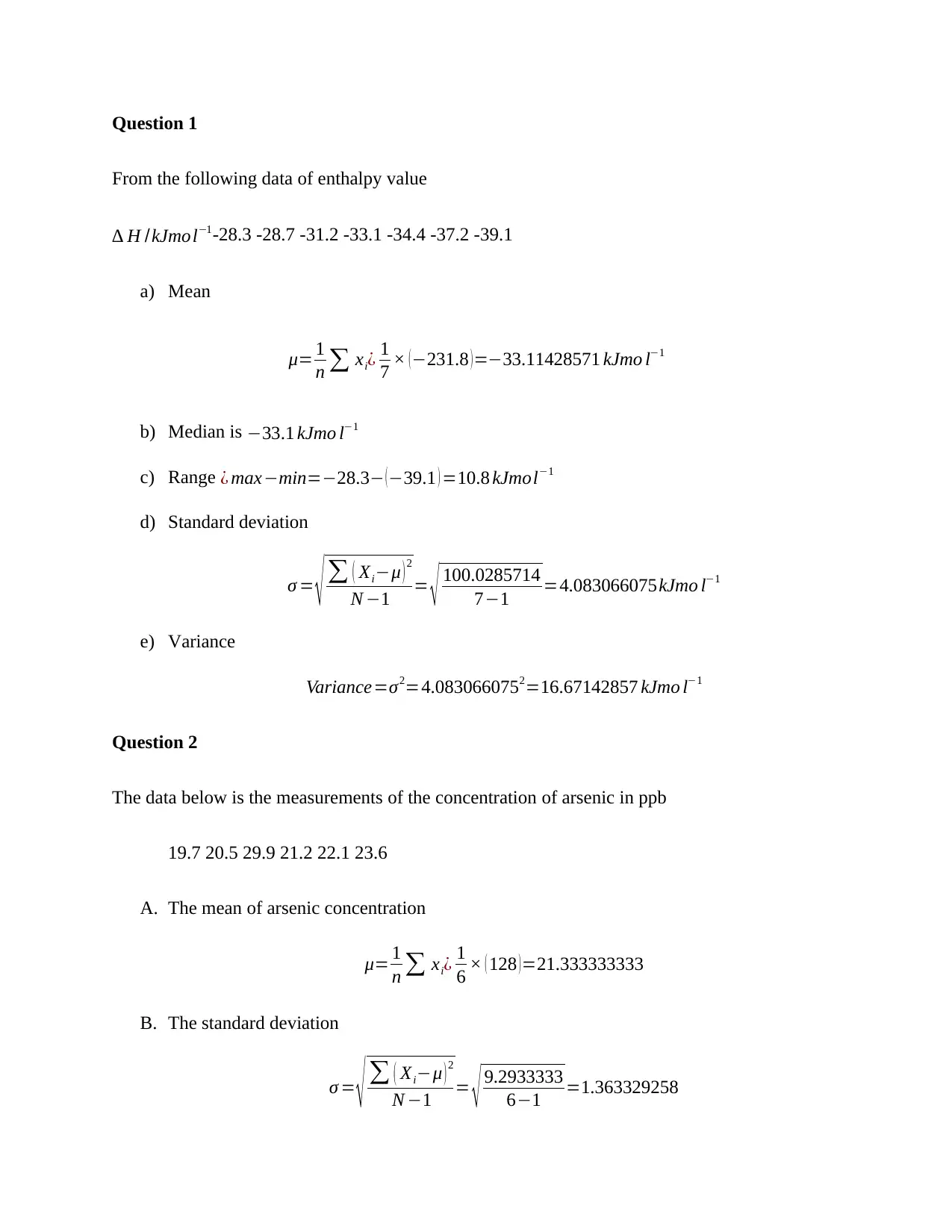
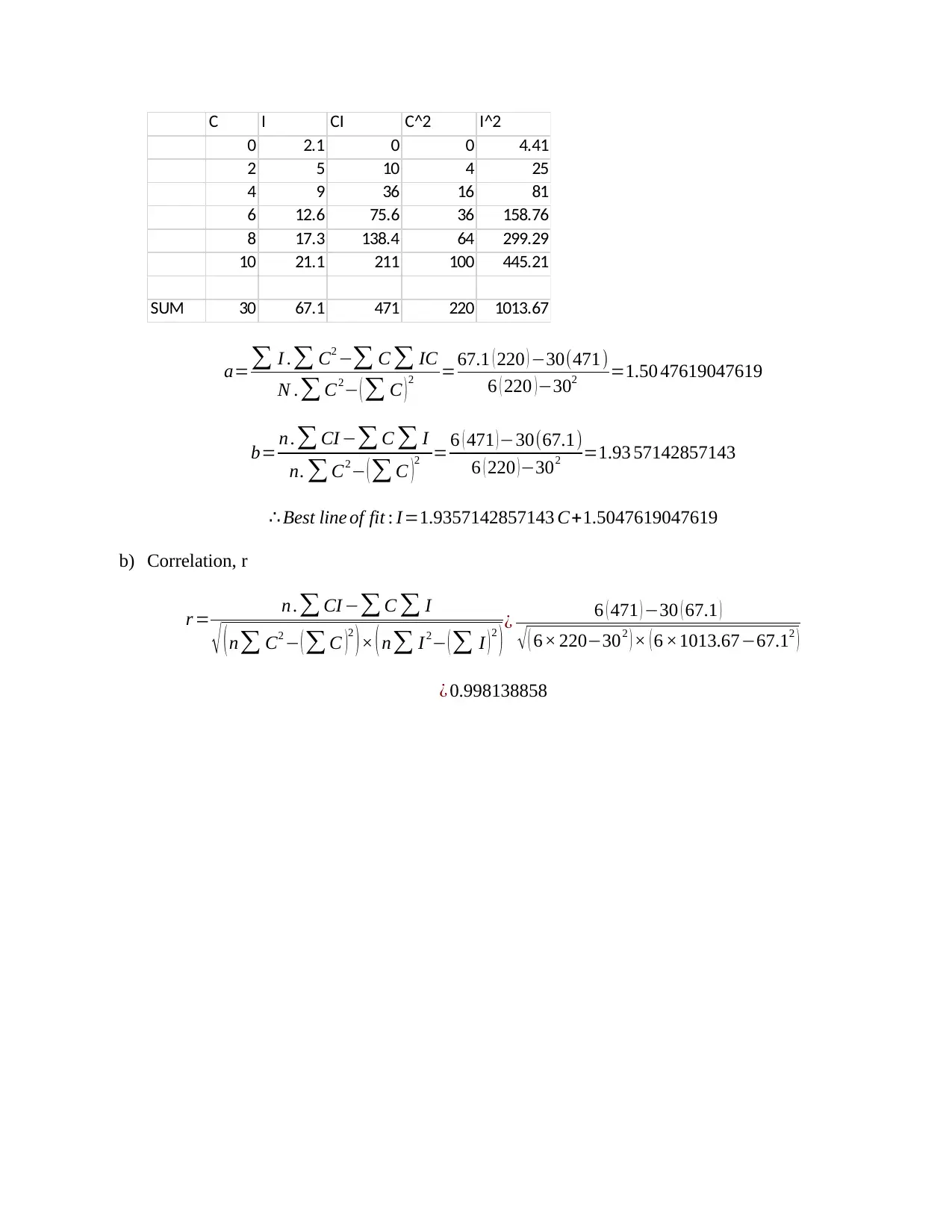
This chemistry assignment solution covers various aspects of chemical kinetics, equilibrium, and data analysis. It includes calculations related to first-order reactions, such as determining the equation for ln(c) and relating it to the form y=mx+c. The solution also demonstrates how to calculate the mass required to prepare a specific molarity solution of MgCl2 and how to determine the final concentration after diluting a NaOH solution. Furthermore, the assignment addresses the variation of the equilibrium constant with temperature, providing a table with temperature and equilibrium constant data, a graph of ln(K) versus 1/T, and the calculation of the slope. Additionally, the solution includes statistical analysis of enthalpy data, calculating the mean, median, range, standard deviation, and variance. It also covers the statistical analysis of arsenic concentration measurements, including the calculation of the mean, standard deviation, standard error of the mean, and the 95% confidence interval. Finally, the assignment includes problems on error propagation, such as calculating the total volume and average speed with associated uncertainties, and finding the best line of fit for fluorescence spectrometry data using linear regression and correlation analysis.

MATH
Question 2:
The rate order for the first order chemical reaction is given by:c=c0 e−kt , where c is the
concentration at time t, c0is the concentration at time t=0
a) Equation for ln(c)
ln ( c )=ln (c0 e−kt )¿ ln ( c0 ) + ln ( e−kt )¿ ln ( c0 ) −kt
b) The equation ln ( c ) =−kt+ ln ( c0 ), is in the form of y=mx+c with slope of −kt and y-
intercept is ln ( c0 ),
Question 3:
Mass required to make 250 cm3, of 0.100 M MgCl2 solution: Molar mass of MgCl2 =95.2
Molarity= moles
Volume 0.100= n
0.25 n=0.1× 0.25=0.025 moles of MgC l2
Mass=Molar mass ×number of moles ¿ 95.2 ×0.025¿ 2.38 g
Question 4
Diluting 25cm3 of 5.00 NaOH with 125 cm3of water. The final volume will be 25+125 =150cm3.
c2 V 2=c1 V 1s c2= c1 V 1
V 2
= 5.00× 25
150 ¿ 0.8333 M NaOH
Question 5
The variation of the equilibrium constant, K with temperature, T for an enzyme reactor

a) Completing the table
T/K 289.0 294.2 298.0 304.9 310.5
K/107 7.25 5.25 4.17 2.66 2.00
103/(T/K) 3.4602 3.3990 3.3557 3.2798 3.2206
ln (K) 18.099
1
17.7763 17.5460 17.0964 16.8112
b) The figure below shows the graph of ln (k) verses 1/T, with the line of best fit
c) The slope is
∆ ( ln ( K ) )
∆ ( 1
T ) =5445

Question 1
From the following data of enthalpy value
∆ H /kJmol−1-28.3 -28.7 -31.2 -33.1 -34.4 -37.2 -39.1
a) Mean
μ= 1
n ∑ xi¿ 1
7 × ( −231.8 ) =−33.11428571 kJmo l−1
b) Median is −33.1 kJmo l−1
c) Range ¿ max−min=−28.3− (−39.1 ) =10.8 kJmol−1
d) Standard deviation
σ = √ ∑ ( Xi−μ ) 2
N −1 = √ 100.0285714
7−1 =4.083066075kJmo l−1
e) Variance
Variance=σ2=4.0830660752=16.67142857 kJmo l−1
Question 2
The data below is the measurements of the concentration of arsenic in ppb
19.7 20.5 29.9 21.2 22.1 23.6
A. The mean of arsenic concentration
μ= 1
n ∑ xi¿ 1
6 × ( 128 ) =21.333333333
B. The standard deviation
σ = √ ∑ ( Xi−μ ) 2
N −1 = √ 9.2933333
6−1 =1.363329258

C. Standard error of the mean
σ M = σ
√n = 1.363329258
√6 =0.556576839
D. 95 confident interval of the mean
μ−t0.95 σ M ≤ X ≤ μ−t0.95 σM
21.333333333−2.57 ( 0.556576839 ) ≤ X ≤21.333333333−2.57 ( 0.556576839 )
19.90293086 ≤ X ≤ 22.76373581
Question 3
V total=V final−V initial ¿ [ 43.20 ± 0.05 ] − [ 25.60 ± 0.05 ] ¿ ( 43.20−25.60 ) ± ( 0.05+0.05 )¿ 17.6 ± 0.10
UV =± 0.10
Question 4
Average speed= Distance
Time ¿ 100± 0.1
10.20± 0.02
m
s ¿ 100
10.20 ± ( 0.1+0.02 ) ¿ 9.80 ± 0.12U speed=± 0.12
Question 5
The result of a fluorescence spectrometry experiment with intensity, I as a function of
concentration
I 2.1 5.0 9.0 12.6 17.3 21.1
C 0.00 2.00 4.00 6.00 8.00 10.00
a) To find the best line of fit is given by I=a+bC, where a is the slope and b is the y- intercept

C I CI C^2 I^2
0 2.1 0 0 4.41
2 5 10 4 25
4 9 36 16 81
6 12.6 75.6 36 158.76
8 17.3 138.4 64 299.29
10 21.1 211 100 445.21
SUM 30 67.1 471 220 1013.67
a=∑ I .∑ C2 −∑ C ∑ IC
N .∑ C2− (∑ C )2 =67.1 ( 220 ) −30(471)
6 ( 220 )−302 =1.50 47619047619
b= n .∑ CI −∑ C ∑ I
n. ∑ C2− ( ∑ C )
2 = 6 ( 471 ) −30(67.1)
6 ( 220 ) −302 =1.93 57142857143
∴ Best line of fit : I=1.9357142857143 C+1.5047619047619
b) Correlation, r
r = n .∑ CI −∑ C ∑ I
√ (n∑ C2 − (∑ C )2
)× ( n∑ I2− (∑ I )2
) ¿ 6 ( 471 ) −30 ( 67.1 )
√ ( 6× 220−302 ) × ( 6 ×1013.67−67.12 )
¿ 0.998138858