Physics Investigation: Analyzing Simple Pendulum Motion & Behavior

This report provides a comprehensive analysis of the simple pendulum, examining its motion, period, and the forces acting upon it. It begins by defining the components of a simple pendulum and deriving the equation for its period, highlighting the influence of length and gravitational acceleration while noting the independence from mass. The report discusses the conditions under which the pendulum approximates simple harmonic motion, particularly at small angles, and explores the restoring force and its relationship to displacement. It also addresses the complexities introduced by larger angles, leading to a non-linear differential equation. The report includes solutions to example problems, demonstrating the application of the derived principles, and interprets the results in terms of the pendulum's behavior. Finally, it references relevant studies to support the analysis and discussion.

PHYSICS
By Name
Course
Instructor
Institution
Location
Date

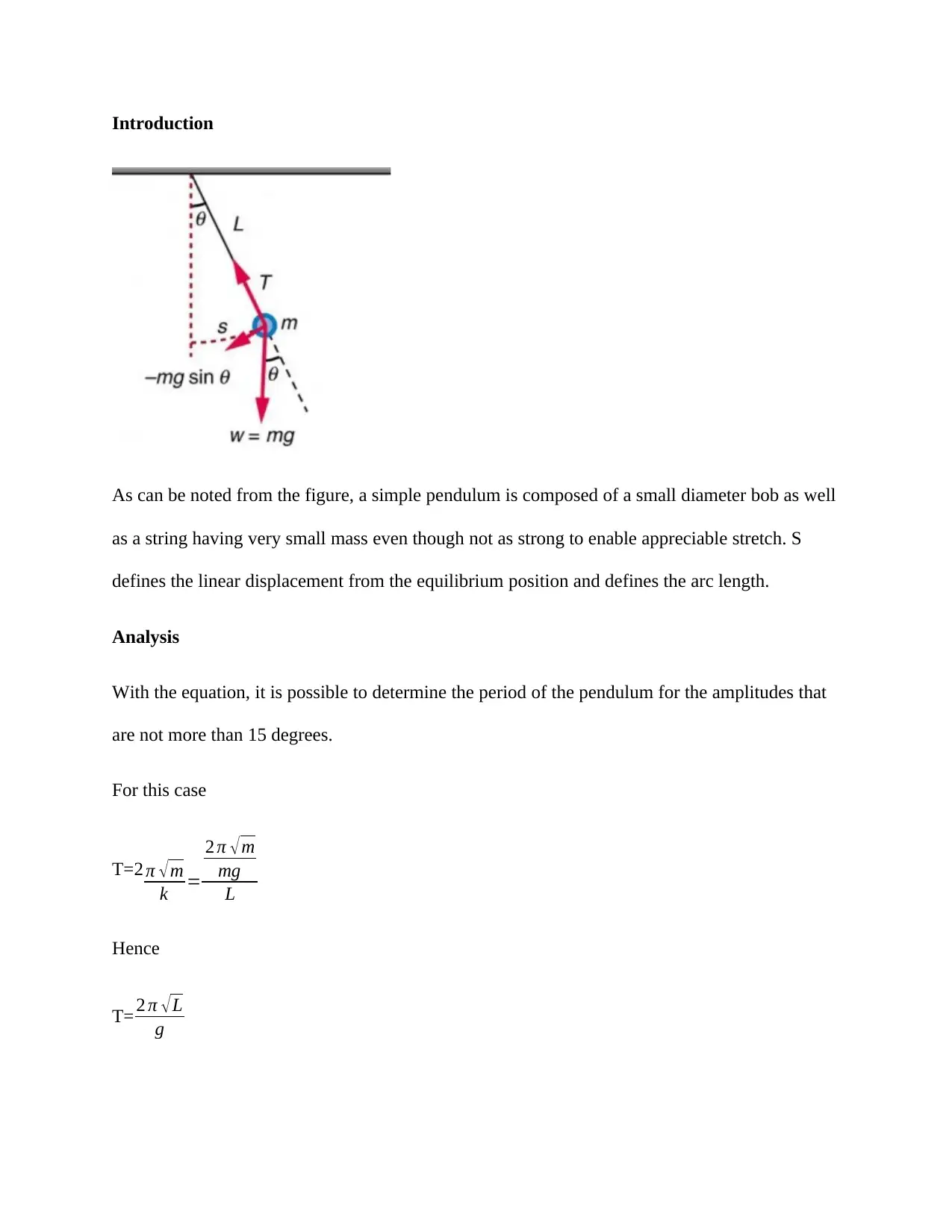
Introduction
As can be noted from the figure, a simple pendulum is composed of a small diameter bob as well
as a string having very small mass even though not as strong to enable appreciable stretch. S
defines the linear displacement from the equilibrium position and defines the arc length.
Analysis
With the equation, it is possible to determine the period of the pendulum for the amplitudes that
are not more than 15 degrees.
For this case
T=2 π √ m
k =
2 π √ m
mg
L
Hence
T= 2 π √L
g

For the period, T, of the provided pendulum, still, in this equation, only the acceleration and
length have an effect on the period of the pendulum as a result of gravity. The period does not in
any way depending on any other factors for example mass [3]. For the case of the simple
harmonic oscillators, the period of the pendulum is almost independent of amplitude mostly in
case Ɵ is below 15 degrees. The periodic time depends on the gravitational acceleration of the
pendulum. Suppose the length is defined, it may be used in the determination of the acceleration
as a result of the gravity.
Is a second order linear differential equation with the term second order insinuating the equation
encompasses a second derivative as well as a linear hence it is composed of no non-linear x
terms including x2 or even sin(x). The equation can be solved as:
In which A defines the amplitude of the motion as the x position rotates between –A and +A
position as φ defines the phase contact. A as well as φ rely on the initul condtions including the
velcouty as well as the time when t=0 while there is a relationship between T, the period, and ω
as given by
Two forces act on a mass on a pendulum: the force due to gravity defined by mg as well as the
tension that is found in the string, Fstring. Fstring does not have a component along the curved x-axis
even as gravity does not have a component along the x-axis that is equivalent to –mg sin Ɵ with

the negative sign showing the force direction is to the origin, normally opposite the direction of
Ɵ and x.
Suppose Ɵ is very small and written in form of radians, then Ɵ is almost equal to sign Ɵ hence
almost is obtained and by the use of F=ma
In such a case of small x or Ɵ values, there is proportionality between the restoring force and x,
the value of displacement resulting in a simple harmonic oscillator having the equation of motion
same as above with the exception of having the constant k/m being replaced with another g/L.
Solution of T provides the first equation and emphasis is given to that the expression holds in the
small amplitude motion limit
In case an assumption is not made of a small value of Ɵ being small, the simplifying assumption
cannot be madesin θ ≡ϑ , the restoration force will then be given by:
With the equation of motion reactions being

Under such scenario, a non-linear differential equation is obtained that proved challenging to
solve. In such a relatively more sophisticated scenario, the period T is not a factor of the mass as
the mass does not appear in the motion equation; it is not possible to have it appear on the values
of m. Nevertheless the period is not a factor of the amplitude of motion. The precise solution
may be expressed in infinite power series alongside the first three terms of solution as shown:
The terms beyond the first three terms tend to be having very small values and as such may often
be infinite. The expression of the angle has to be provided in radians as opposed to degrees.
Solutions
Problem 1

Problem 2
When the friction is reduced by 100 as a result of oiling
Hence the clock ticks by about 50 times after oiling of the clock
Interpretations
The displacement of the pendulum, s is directly proportional to the angle of displacement which
is often expressed in radians
s=LƟ
Such that
Ɵ=s/L
For angles of small sizes, the expression that is used for restoring force is
F= (mg/L) s
The expression can be made in the form
F=-kx
In which the force constant is provided as k=mg/L while the displacement is provided by x=s.
For the angles that are less than 15 degrees as provided in this case, there is a direct
proportionality between the displacement and the restoring force and the simple pendulum acts
as a simple harmonic oscillator [3].

The period of pendulum is not factor of the mass but rather the length of the string hence two
pendula having varied masses but same length will exhibit the same period while on the other
hand, two pendula that are of varied lengths with varied periods will see the pendulum having a
longer string have a longer period. The frequency and period of a pendulum do not depend on the
initial angular displacement amplitude for the case of very small angles as given in the problem.
Regardless of the initial angle, there pendulum will exhibit the same period [1].
When the displacement from the equilibrium position is small, then it is established that sinƟ
Ɵ in radian
Hence F=-mg Ɵ=-(mg/L)
The force is thus obeying Hooke’s law, F=-ks where k=mg/L
For small displacement like the one demonstrated in the problem, the pendulum executes simple
harmonic motion
s (t)=smaxcos (wt+Ɵ), where w2=g/L
For the case of small oscillations, the period of the simple pendulum hence is provided by T=2
π
ω =2 π √(L /g)
It does not depend on the mass of the bob and instead only a factor of the strength of the
acceleration of gravity, g, as well as the length of the string used, L. By taking measurement of
the period of a simple pendulum, the gravitational acceleration can be obtained.
There is a direct proportionality between the restoring force and the displacement hence a simple
harmonic oscillator. In an attempt to determine if it is a simple harmonic oscillator, the angles the

small angels are such thatsin θ ≈ θ. Hence for angles that are below 15 degree, the restoring force
F is given by [2].
F≈−mgθ
Discussion
The period of pendulum is not factor of the mass but rather the length of the string hence two
pendula having varied masses but same length will exhibit the same period while on the other
hand, two pendula that are of varied lengths with varied periods will see the pendulum having a
longer string have a longer period

References
[1] Déda, T., Fujiwara, E. and Carneiro, E., 2018. Modular approach for control design of an
autonomous two-wheeled inverted pendulum. Journal of the Brazilian Society of
Mechanical Sciences and Engineering, 40(11), p.536
[2] Najdecka, A., Kapitaniak, T. and Wiercigroch, M., 2015. Synchronous rotational motion
of parametric pendulums. International Journal of Non-Linear Mechanics, 70, pp.84-94
[3] Muraleedharan, N., Cohen, D.S. and Isenberg, D.R., 2016, March. Omnidirectional
locomotion control of a pendulum driven spherical robot. In SoutheastCon 2016 (pp. 1-
6). IEEE