STA101 Statistics for Business: Assignment on Data Analysis, 2017
VerifiedAdded on 2020/03/16
|15
|2206
|36
Homework Assignment
AI Summary
This document presents a comprehensive solution to an STA101 Statistics for Business assignment. The assignment covers various aspects of data analysis, including data types (categorical, discrete, and continuous), measurement scales (nominal, ordinal, interval, and ratio), and descriptive statistics such as mean, median, and mode. It delves into the calculation and interpretation of standard deviation, the identification of outliers using Z-scores, and the application of the Empirical Rule to assess data normality. Furthermore, the solution addresses probability calculations, including the probability of alternator failures, and provides a detailed analysis of student performance on quizzes, comparing measures of central tendency and assessing data symmetry. The document also includes a confidence interval calculation and a sample size determination, along with a visual representation of the data through a line chart and concludes with a list of references.

1
Individual Assignment
Unit: STA101 – Statistics for Business
Total Marks: This assignment is worth 30% of the total marks in the unit.
Due Date: 13th October 2017
Individual Assignment
Unit: STA101 – Statistics for Business
Total Marks: This assignment is worth 30% of the total marks in the unit.
Due Date: 13th October 2017
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

Table of Contents
Answer:1................................................................................................................................................3
Answer 2.................................................................................................................................................4
ANSWER 3...............................................................................................................................................8
ANSWER 4...............................................................................................................................................9
ANSWER 5.............................................................................................................................................10
References............................................................................................................................................13
Answer:1................................................................................................................................................3
Answer 2.................................................................................................................................................4
ANSWER 3...............................................................................................................................................8
ANSWER 4...............................................................................................................................................9
ANSWER 5.............................................................................................................................................10
References............................................................................................................................................13

Answer:1
Data is the small fragments of raw information collected for study and analysis for making it
useful in form an informed conclusion. Data is of three types namely, Categorical, discrete
numerical and continuous numerical. Categorical data is also understood as the qualitative
data which represents various characteristics like gender, marital status, city, etc. Such data
may have a numerical value but which don’t have any mathematical meaning.
On the other hand, numerical data, as the name itself suggest, is with quantitative
characteristic of measurement, like height, weight, etc. Further dividing into two as Discrete
data which can be counted and have possible values which is either fixed or in a range going
on to infinity; and Continuous data represent measurements that can be described using
intervals.
As per the views of Cressie (2015), mmeasurement scales are of three types namely, Nominal,
ordinal, interval and ratio scale. The nominal scale measures variable with a descriptive
category, but have no natural numerical value. The ordinal scale has both identity and
magnitude property. The interval scale has identity, magnitude and equal intervals as its
properties (Willer and Lernoud, 2016). The ratio scale has all four properties of measurement
namely, identity, magnitude, equal intervals and minimum value of zero.
What is your gender? (Male = 0, Female = 1)
Data Type: Categorical data with qualitative characteristic of a gender.
Measurement Level: Nominal scale of measurement as satisfies only identity property.
What is your approximate undergraduate college GPA? (1.0 to 4.0)
Data Type: Discrete data with finite possible values.
Measurement Level: Ordinal scale of measurement satisfying both identity and magnitude as
property.
About how many hours per week do you expect to work at an outside job this semester?
Data Type: Discrete date with infinite possible values.
Measurement Level: Ordinal scale of measurement satisfying both identity and magnitude as
property.
What do you think is the ideal number of children for a married couple?
Data Type: Discrete data with finite possible values.
Measurement Level: Interval scale of measurement with identity, magnitude and equal
Data is the small fragments of raw information collected for study and analysis for making it
useful in form an informed conclusion. Data is of three types namely, Categorical, discrete
numerical and continuous numerical. Categorical data is also understood as the qualitative
data which represents various characteristics like gender, marital status, city, etc. Such data
may have a numerical value but which don’t have any mathematical meaning.
On the other hand, numerical data, as the name itself suggest, is with quantitative
characteristic of measurement, like height, weight, etc. Further dividing into two as Discrete
data which can be counted and have possible values which is either fixed or in a range going
on to infinity; and Continuous data represent measurements that can be described using
intervals.
As per the views of Cressie (2015), mmeasurement scales are of three types namely, Nominal,
ordinal, interval and ratio scale. The nominal scale measures variable with a descriptive
category, but have no natural numerical value. The ordinal scale has both identity and
magnitude property. The interval scale has identity, magnitude and equal intervals as its
properties (Willer and Lernoud, 2016). The ratio scale has all four properties of measurement
namely, identity, magnitude, equal intervals and minimum value of zero.
What is your gender? (Male = 0, Female = 1)
Data Type: Categorical data with qualitative characteristic of a gender.
Measurement Level: Nominal scale of measurement as satisfies only identity property.
What is your approximate undergraduate college GPA? (1.0 to 4.0)
Data Type: Discrete data with finite possible values.
Measurement Level: Ordinal scale of measurement satisfying both identity and magnitude as
property.
About how many hours per week do you expect to work at an outside job this semester?
Data Type: Discrete date with infinite possible values.
Measurement Level: Ordinal scale of measurement satisfying both identity and magnitude as
property.
What do you think is the ideal number of children for a married couple?
Data Type: Discrete data with finite possible values.
Measurement Level: Interval scale of measurement with identity, magnitude and equal
⊘ This is a preview!⊘
Do you want full access?
Subscribe today to unlock all pages.

Trusted by 1+ million students worldwide
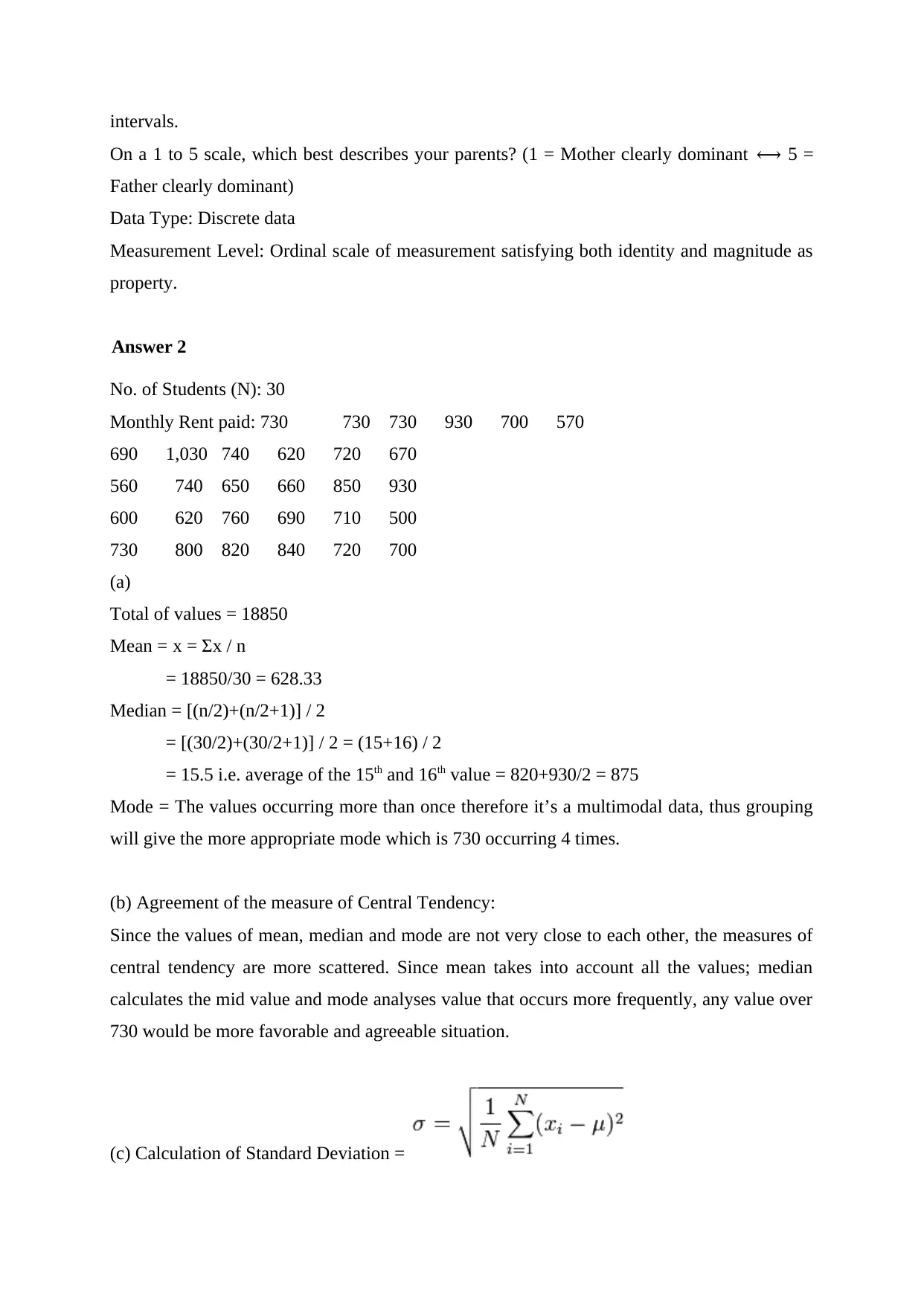
intervals.
On a 1 to 5 scale, which best describes your parents? (1 = Mother clearly dominant ⟷ 5 =
Father clearly dominant)
Data Type: Discrete data
Measurement Level: Ordinal scale of measurement satisfying both identity and magnitude as
property.
Answer 2
No. of Students (N): 30
Monthly Rent paid: 730 730 730 930 700 570
690 1,030 740 620 720 670
560 740 650 660 850 930
600 620 760 690 710 500
730 800 820 840 720 700
(a)
Total of values = 18850
Mean = x = Σx / n
= 18850/30 = 628.33
Median = [(n/2)+(n/2+1)] / 2
= [(30/2)+(30/2+1)] / 2 = (15+16) / 2
= 15.5 i.e. average of the 15th and 16th value = 820+930/2 = 875
Mode = The values occurring more than once therefore it’s a multimodal data, thus grouping
will give the more appropriate mode which is 730 occurring 4 times.
(b) Agreement of the measure of Central Tendency:
Since the values of mean, median and mode are not very close to each other, the measures of
central tendency are more scattered. Since mean takes into account all the values; median
calculates the mid value and mode analyses value that occurs more frequently, any value over
730 would be more favorable and agreeable situation.
(c) Calculation of Standard Deviation =
On a 1 to 5 scale, which best describes your parents? (1 = Mother clearly dominant ⟷ 5 =
Father clearly dominant)
Data Type: Discrete data
Measurement Level: Ordinal scale of measurement satisfying both identity and magnitude as
property.
Answer 2
No. of Students (N): 30
Monthly Rent paid: 730 730 730 930 700 570
690 1,030 740 620 720 670
560 740 650 660 850 930
600 620 760 690 710 500
730 800 820 840 720 700
(a)
Total of values = 18850
Mean = x = Σx / n
= 18850/30 = 628.33
Median = [(n/2)+(n/2+1)] / 2
= [(30/2)+(30/2+1)] / 2 = (15+16) / 2
= 15.5 i.e. average of the 15th and 16th value = 820+930/2 = 875
Mode = The values occurring more than once therefore it’s a multimodal data, thus grouping
will give the more appropriate mode which is 730 occurring 4 times.
(b) Agreement of the measure of Central Tendency:
Since the values of mean, median and mode are not very close to each other, the measures of
central tendency are more scattered. Since mean takes into account all the values; median
calculates the mid value and mode analyses value that occurs more frequently, any value over
730 would be more favorable and agreeable situation.
(c) Calculation of Standard Deviation =
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

Value Mean A-B Square
(A-B)
(A-B)
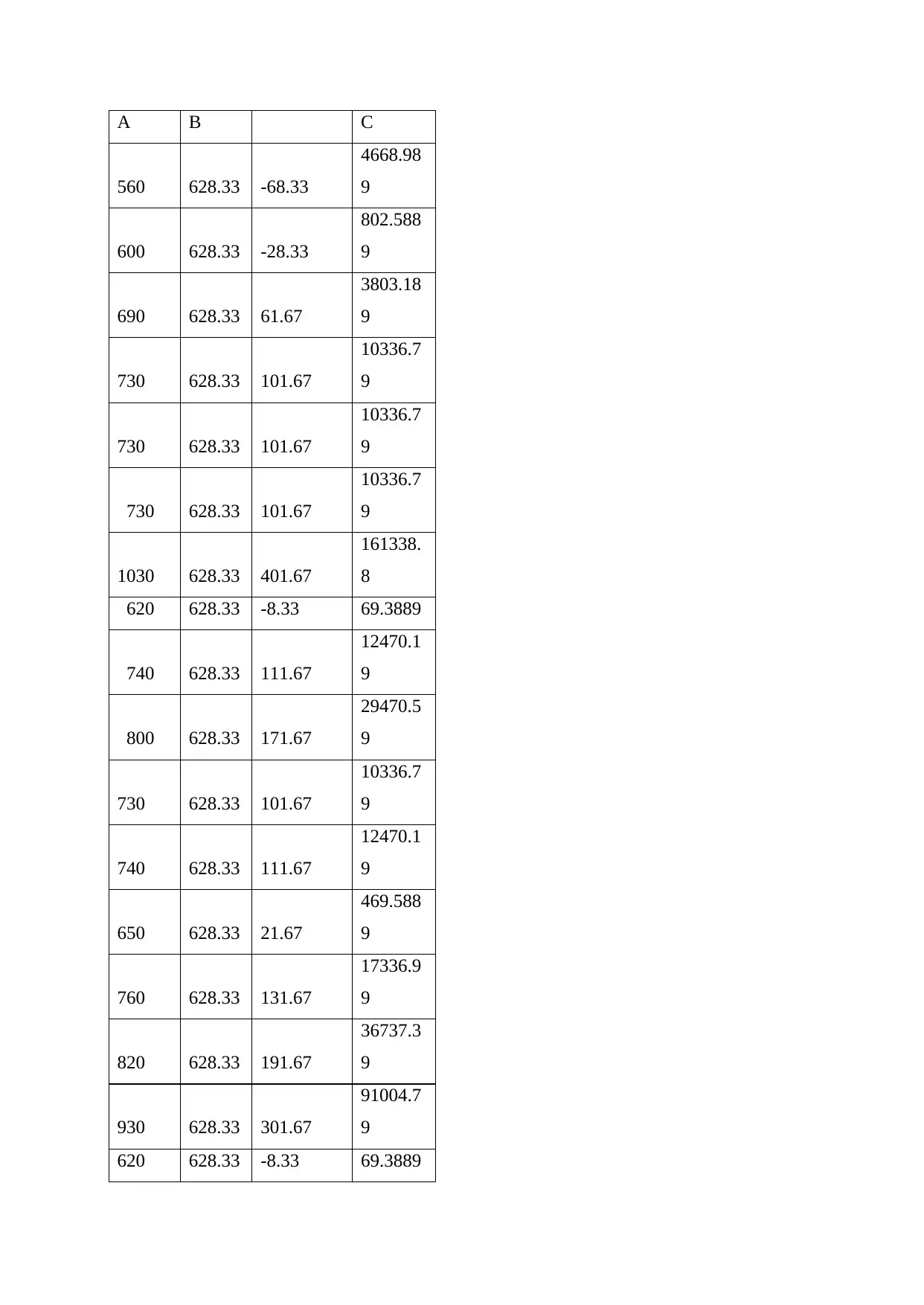
A B C
560 628.33 -68.33
4668.98
9
600 628.33 -28.33
802.588
9
690 628.33 61.67
3803.18
9
730 628.33 101.67
10336.7
9
730 628.33 101.67
10336.7
9
730 628.33 101.67
10336.7
9
1030 628.33 401.67
161338.
8
620 628.33 -8.33 69.3889
740 628.33 111.67
12470.1
9
800 628.33 171.67
29470.5
9
730 628.33 101.67
10336.7
9
740 628.33 111.67
12470.1
9
650 628.33 21.67
469.588
9
760 628.33 131.67
17336.9
9
820 628.33 191.67
36737.3
9
930 628.33 301.67
91004.7
9
620 628.33 -8.33 69.3889
560 628.33 -68.33
4668.98
9
600 628.33 -28.33
802.588
9
690 628.33 61.67
3803.18
9
730 628.33 101.67
10336.7
9
730 628.33 101.67
10336.7
9
730 628.33 101.67
10336.7
9
1030 628.33 401.67
161338.
8
620 628.33 -8.33 69.3889
740 628.33 111.67
12470.1
9
800 628.33 171.67
29470.5
9
730 628.33 101.67
10336.7
9
740 628.33 111.67
12470.1
9
650 628.33 21.67
469.588
9
760 628.33 131.67
17336.9
9
820 628.33 191.67
36737.3
9
930 628.33 301.67
91004.7
9
620 628.33 -8.33 69.3889
⊘ This is a preview!⊘
Do you want full access?
Subscribe today to unlock all pages.

Trusted by 1+ million students worldwide
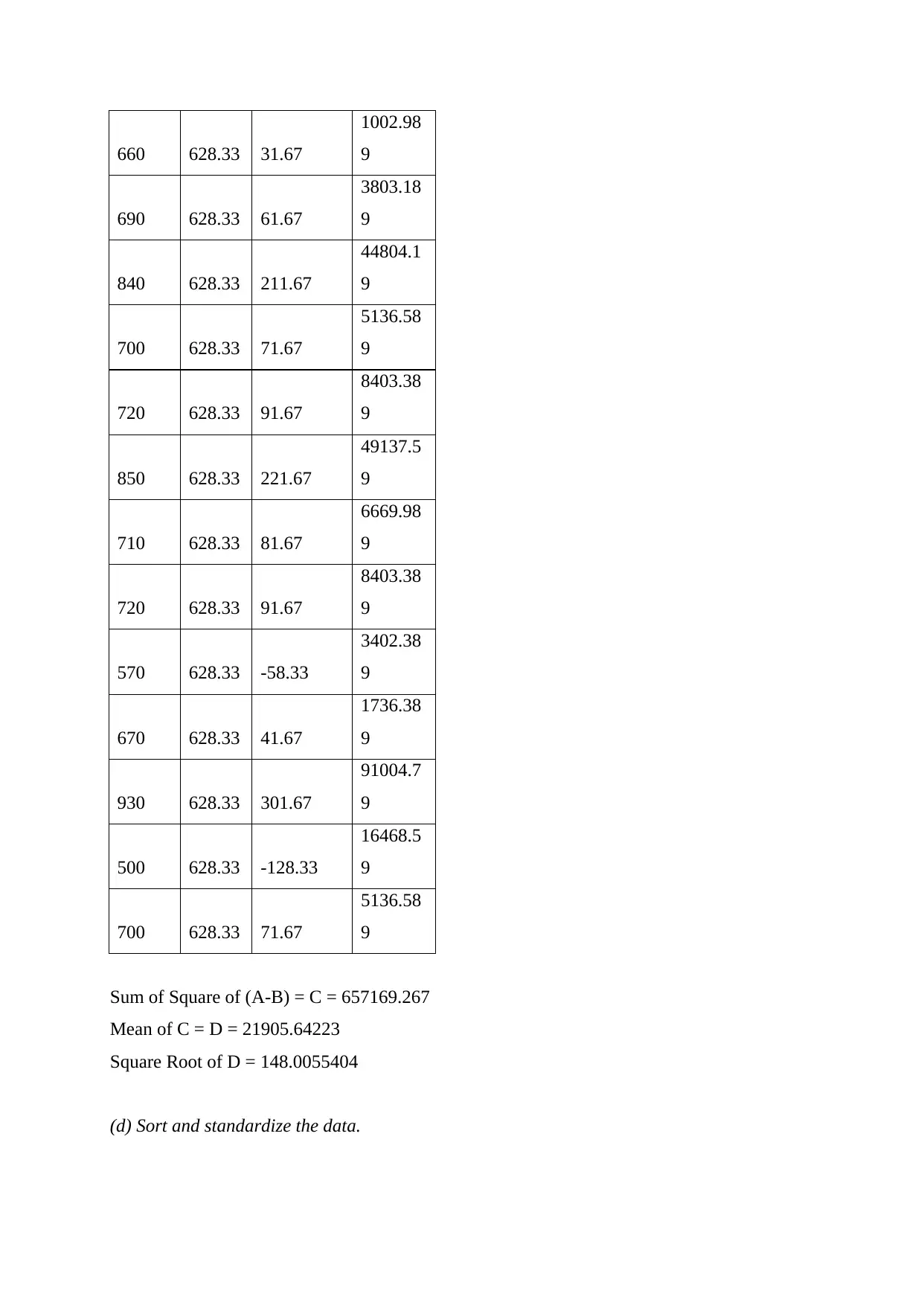
660 628.33 31.67
1002.98
9
690 628.33 61.67
3803.18
9
840 628.33 211.67
44804.1
9
700 628.33 71.67
5136.58
9
720 628.33 91.67
8403.38
9
850 628.33 221.67
49137.5
9
710 628.33 81.67
6669.98
9
720 628.33 91.67
8403.38
9
570 628.33 -58.33
3402.38
9
670 628.33 41.67
1736.38
9
930 628.33 301.67
91004.7
9
500 628.33 -128.33
16468.5
9
700 628.33 71.67
5136.58
9
Sum of Square of (A-B) = C = 657169.267
Mean of C = D = 21905.64223
Square Root of D = 148.0055404
(d) Sort and standardize the data.
1002.98
9
690 628.33 61.67
3803.18
9
840 628.33 211.67
44804.1
9
700 628.33 71.67
5136.58
9
720 628.33 91.67
8403.38
9
850 628.33 221.67
49137.5
9
710 628.33 81.67
6669.98
9
720 628.33 91.67
8403.38
9
570 628.33 -58.33
3402.38
9
670 628.33 41.67
1736.38
9
930 628.33 301.67
91004.7
9
500 628.33 -128.33
16468.5
9
700 628.33 71.67
5136.58
9
Sum of Square of (A-B) = C = 657169.267
Mean of C = D = 21905.64223
Square Root of D = 148.0055404
(d) Sort and standardize the data.
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
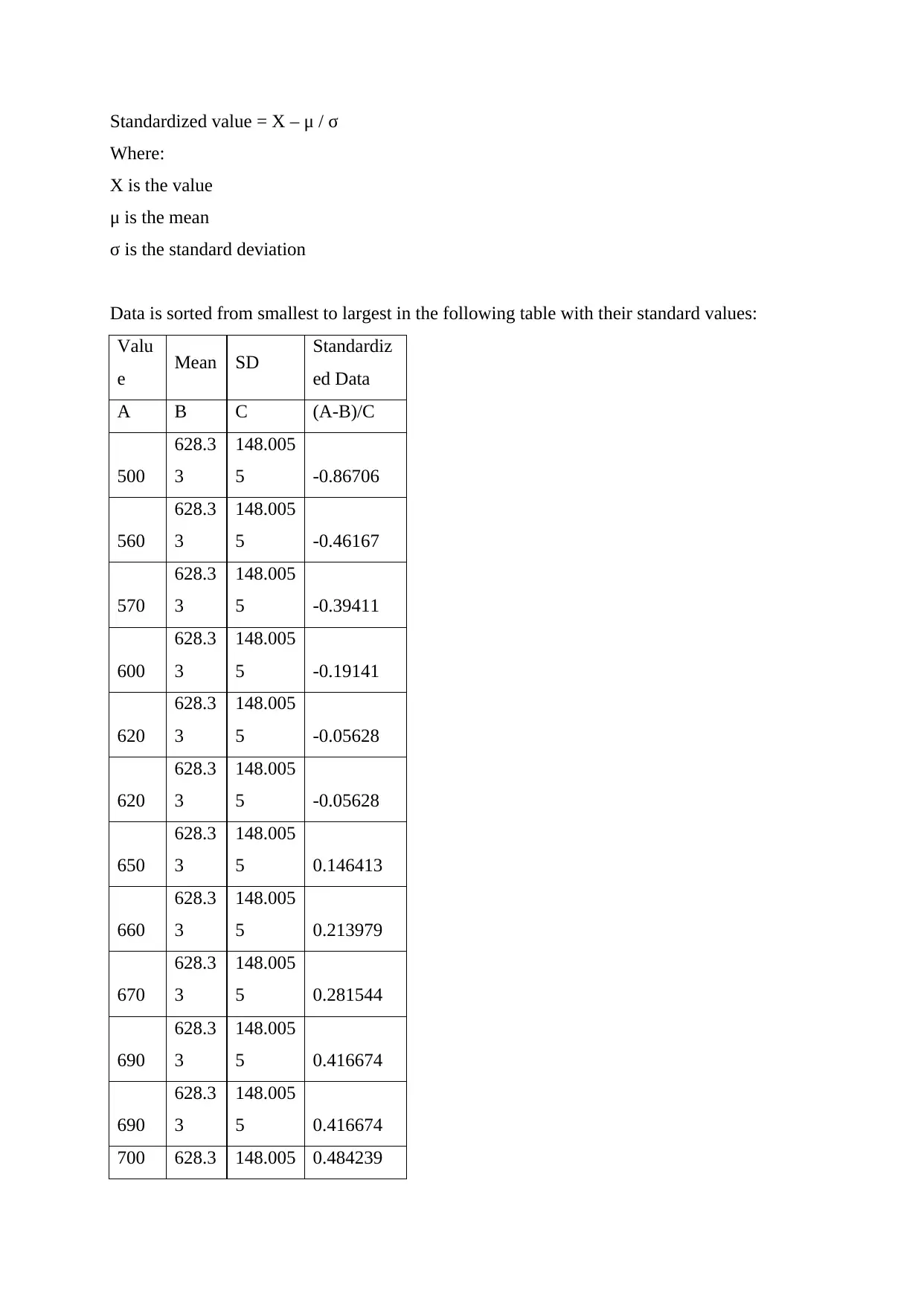
Standardized value = X – μ / σ
Where:
X is the value
μ is the mean
σ is the standard deviation
Data is sorted from smallest to largest in the following table with their standard values:
Valu
e Mean SD Standardiz
ed Data
A B C (A-B)/C
500
628.3
3
148.005
5 -0.86706
560
628.3
3
148.005
5 -0.46167
570
628.3
3
148.005
5 -0.39411
600
628.3
3
148.005
5 -0.19141
620
628.3
3
148.005
5 -0.05628
620
628.3
3
148.005
5 -0.05628
650
628.3
3
148.005
5 0.146413
660
628.3
3
148.005
5 0.213979
670
628.3
3
148.005
5 0.281544
690
628.3
3
148.005
5 0.416674
690
628.3
3
148.005
5 0.416674
700 628.3 148.005 0.484239
Where:
X is the value
μ is the mean
σ is the standard deviation
Data is sorted from smallest to largest in the following table with their standard values:
Valu
e Mean SD Standardiz
ed Data
A B C (A-B)/C
500
628.3
3
148.005
5 -0.86706
560
628.3
3
148.005
5 -0.46167
570
628.3
3
148.005
5 -0.39411
600
628.3
3
148.005
5 -0.19141
620
628.3
3
148.005
5 -0.05628
620
628.3
3
148.005
5 -0.05628
650
628.3
3
148.005
5 0.146413
660
628.3
3
148.005
5 0.213979
670
628.3
3
148.005
5 0.281544
690
628.3
3
148.005
5 0.416674
690
628.3
3
148.005
5 0.416674
700 628.3 148.005 0.484239
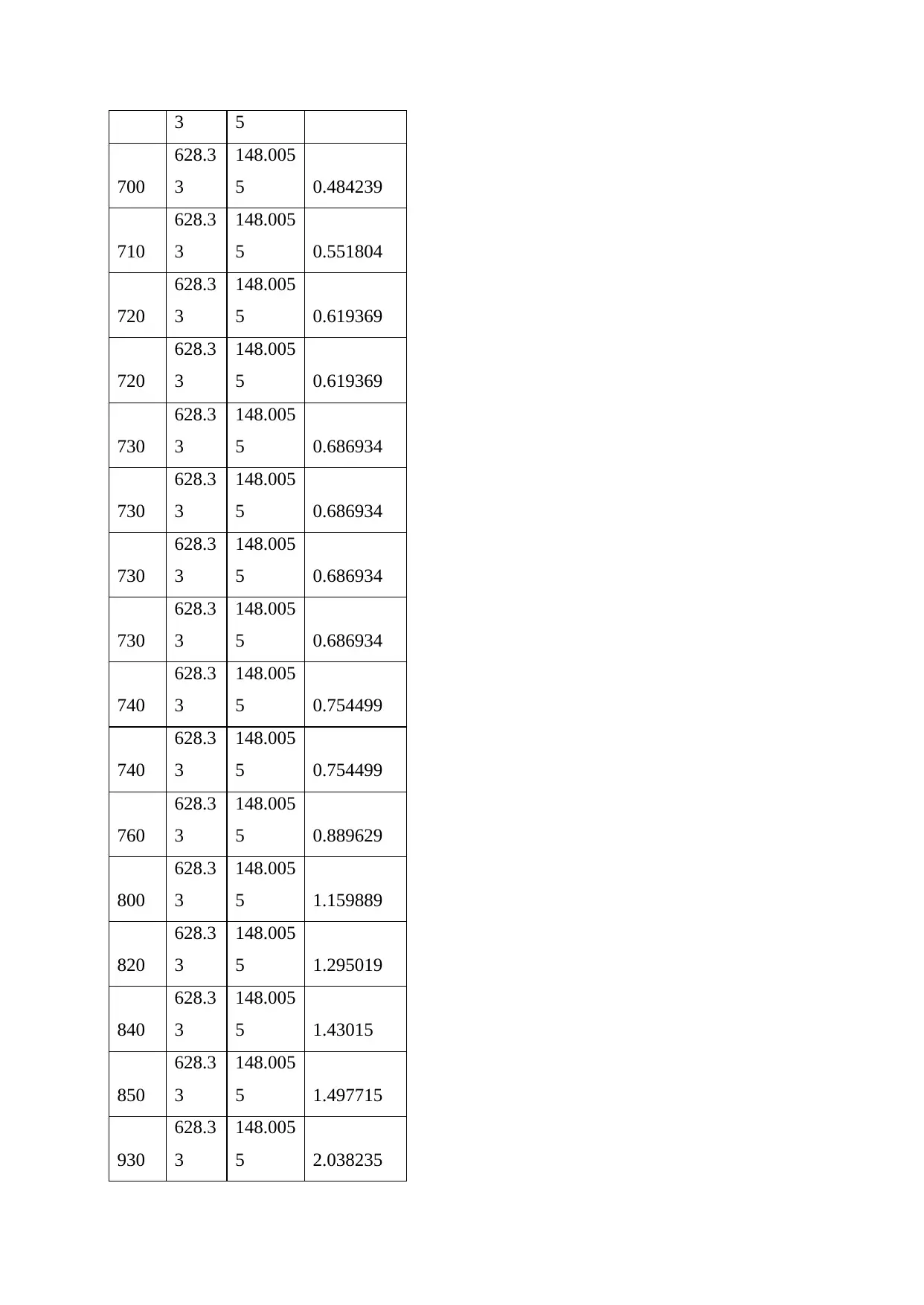
3 5
700
628.3
3
148.005
5 0.484239
710
628.3
3
148.005
5 0.551804
720
628.3
3
148.005
5 0.619369
720
628.3
3
148.005
5 0.619369
730
628.3
3
148.005
5 0.686934
730
628.3
3
148.005
5 0.686934
730
628.3
3
148.005
5 0.686934
730
628.3
3
148.005
5 0.686934
740
628.3
3
148.005
5 0.754499
740
628.3
3
148.005
5 0.754499
760
628.3
3
148.005
5 0.889629
800
628.3
3
148.005
5 1.159889
820
628.3
3
148.005
5 1.295019
840
628.3
3
148.005
5 1.43015
850
628.3
3
148.005
5 1.497715
930
628.3
3
148.005
5 2.038235
700
628.3
3
148.005
5 0.484239
710
628.3
3
148.005
5 0.551804
720
628.3
3
148.005
5 0.619369
720
628.3
3
148.005
5 0.619369
730
628.3
3
148.005
5 0.686934
730
628.3
3
148.005
5 0.686934
730
628.3
3
148.005
5 0.686934
730
628.3
3
148.005
5 0.686934
740
628.3
3
148.005
5 0.754499
740
628.3
3
148.005
5 0.754499
760
628.3
3
148.005
5 0.889629
800
628.3
3
148.005
5 1.159889
820
628.3
3
148.005
5 1.295019
840
628.3
3
148.005
5 1.43015
850
628.3
3
148.005
5 1.497715
930
628.3
3
148.005
5 2.038235
⊘ This is a preview!⊘
Do you want full access?
Subscribe today to unlock all pages.

Trusted by 1+ million students worldwide
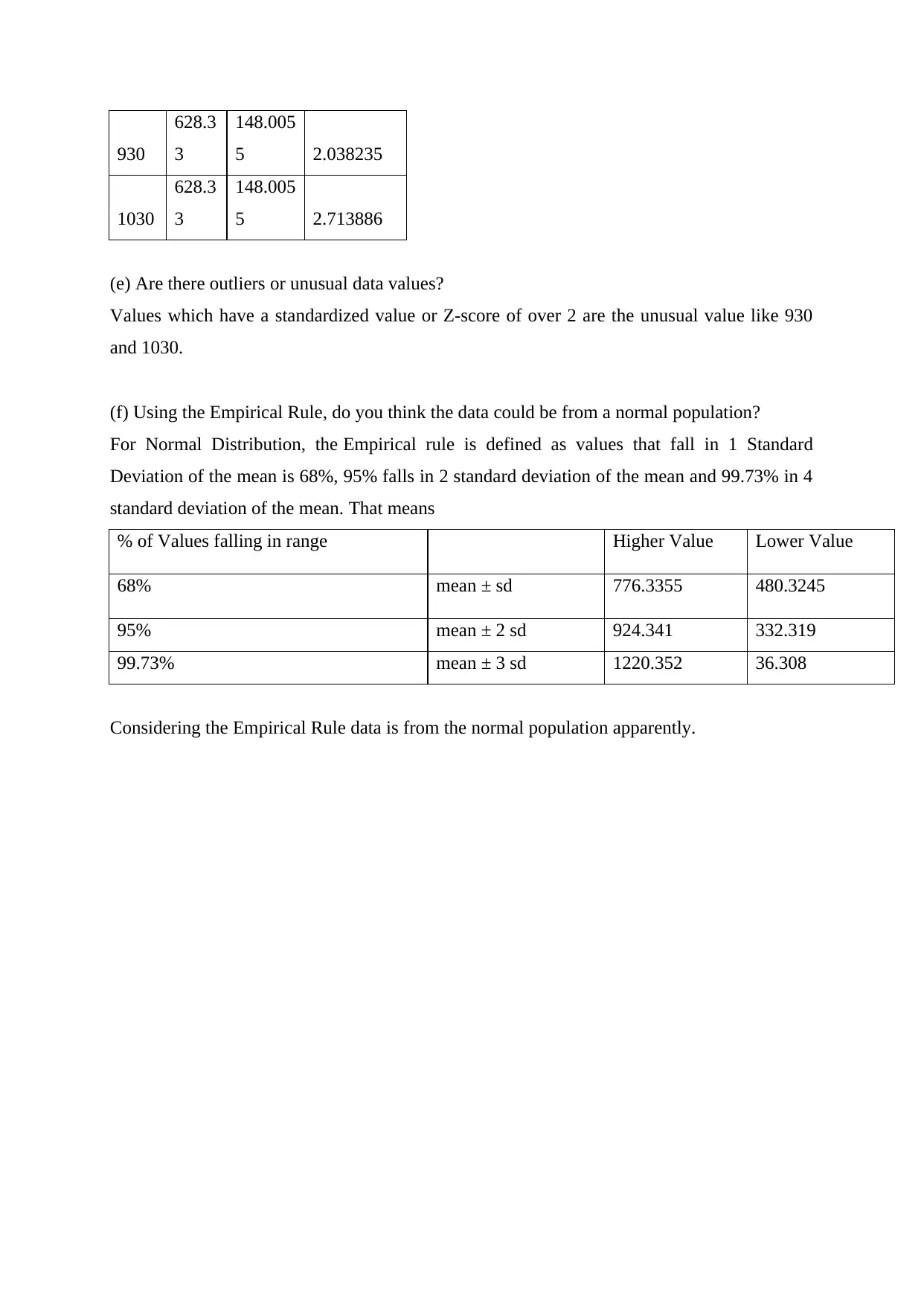
930
628.3
3
148.005
5 2.038235
1030
628.3
3
148.005
5 2.713886
(e) Are there outliers or unusual data values?
Values which have a standardized value or Z-score of over 2 are the unusual value like 930
and 1030.
(f) Using the Empirical Rule, do you think the data could be from a normal population?
For Normal Distribution, the Empirical rule is defined as values that fall in 1 Standard
Deviation of the mean is 68%, 95% falls in 2 standard deviation of the mean and 99.73% in 4
standard deviation of the mean. That means
% of Values falling in range Higher Value Lower Value
68% mean ± sd 776.3355 480.3245
95% mean ± 2 sd 924.341 332.319
99.73% mean ± 3 sd 1220.352 36.308
Considering the Empirical Rule data is from the normal population apparently.
628.3
3
148.005
5 2.038235
1030
628.3
3
148.005
5 2.713886
(e) Are there outliers or unusual data values?
Values which have a standardized value or Z-score of over 2 are the unusual value like 930
and 1030.
(f) Using the Empirical Rule, do you think the data could be from a normal population?
For Normal Distribution, the Empirical rule is defined as values that fall in 1 Standard
Deviation of the mean is 68%, 95% falls in 2 standard deviation of the mean and 99.73% in 4
standard deviation of the mean. That means
% of Values falling in range Higher Value Lower Value
68% mean ± sd 776.3355 480.3245
95% mean ± 2 sd 924.341 332.319
99.73% mean ± 3 sd 1220.352 36.308
Considering the Empirical Rule data is from the normal population apparently.
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
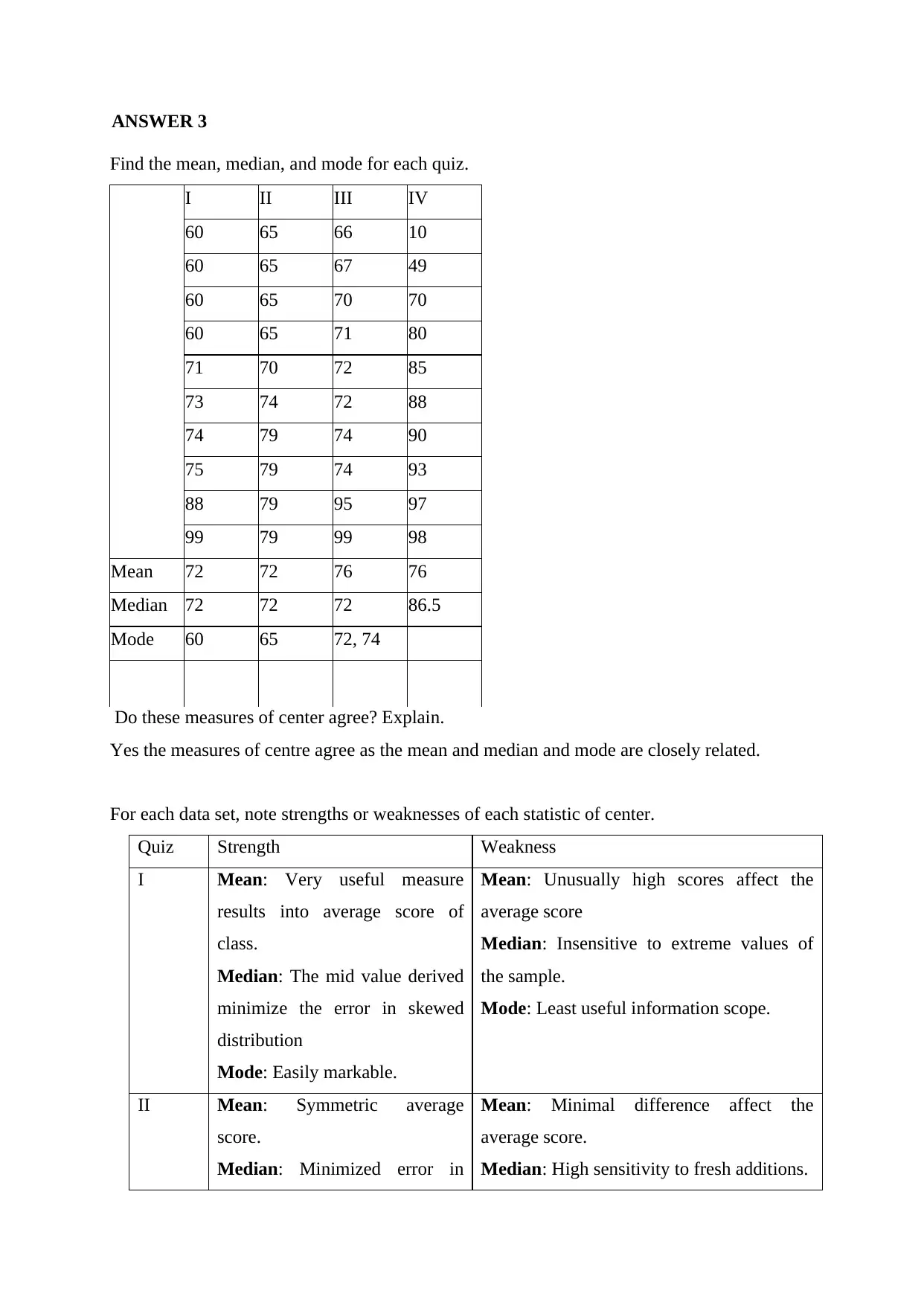
ANSWER 3
Find the mean, median, and mode for each quiz.
I II III IV
60 65 66 10
60 65 67 49
60 65 70 70
60 65 71 80
71 70 72 85
73 74 72 88
74 79 74 90
75 79 74 93
88 79 95 97
99 79 99 98
Mean 72 72 76 76
Median 72 72 72 86.5
Mode 60 65 72, 74
Do these measures of center agree? Explain.
Yes the measures of centre agree as the mean and median and mode are closely related.
For each data set, note strengths or weaknesses of each statistic of center.
Quiz Strength Weakness
I Mean: Very useful measure
results into average score of
class.
Median: The mid value derived
minimize the error in skewed
distribution
Mode: Easily markable.
Mean: Unusually high scores affect the
average score
Median: Insensitive to extreme values of
the sample.
Mode: Least useful information scope.
II Mean: Symmetric average
score.
Median: Minimized error in
Mean: Minimal difference affect the
average score.
Median: High sensitivity to fresh additions.
Find the mean, median, and mode for each quiz.
I II III IV
60 65 66 10
60 65 67 49
60 65 70 70
60 65 71 80
71 70 72 85
73 74 72 88
74 79 74 90
75 79 74 93
88 79 95 97
99 79 99 98
Mean 72 72 76 76
Median 72 72 72 86.5
Mode 60 65 72, 74
Do these measures of center agree? Explain.
Yes the measures of centre agree as the mean and median and mode are closely related.
For each data set, note strengths or weaknesses of each statistic of center.
Quiz Strength Weakness
I Mean: Very useful measure
results into average score of
class.
Median: The mid value derived
minimize the error in skewed
distribution
Mode: Easily markable.
Mean: Unusually high scores affect the
average score
Median: Insensitive to extreme values of
the sample.
Mode: Least useful information scope.
II Mean: Symmetric average
score.
Median: Minimized error in
Mean: Minimal difference affect the
average score.
Median: High sensitivity to fresh additions.
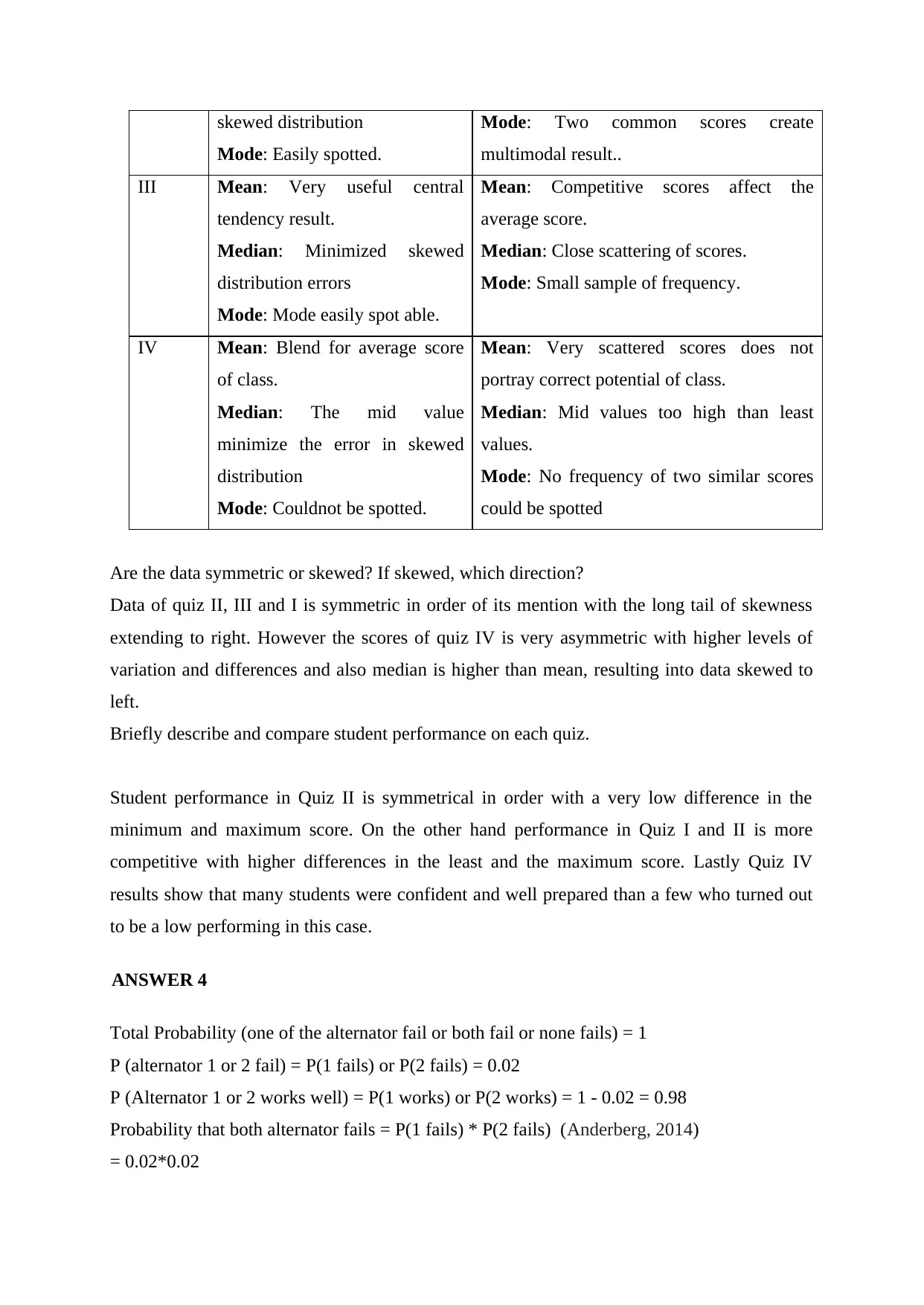
skewed distribution
Mode: Easily spotted.
Mode: Two common scores create
multimodal result..
III Mean: Very useful central
tendency result.
Median: Minimized skewed
distribution errors
Mode: Mode easily spot able.
Mean: Competitive scores affect the
average score.
Median: Close scattering of scores.
Mode: Small sample of frequency.
IV Mean: Blend for average score
of class.
Median: The mid value
minimize the error in skewed
distribution
Mode: Couldnot be spotted.
Mean: Very scattered scores does not
portray correct potential of class.
Median: Mid values too high than least
values.
Mode: No frequency of two similar scores
could be spotted
Are the data symmetric or skewed? If skewed, which direction?
Data of quiz II, III and I is symmetric in order of its mention with the long tail of skewness
extending to right. However the scores of quiz IV is very asymmetric with higher levels of
variation and differences and also median is higher than mean, resulting into data skewed to
left.
Briefly describe and compare student performance on each quiz.
Student performance in Quiz II is symmetrical in order with a very low difference in the
minimum and maximum score. On the other hand performance in Quiz I and II is more
competitive with higher differences in the least and the maximum score. Lastly Quiz IV
results show that many students were confident and well prepared than a few who turned out
to be a low performing in this case.
ANSWER 4
Total Probability (one of the alternator fail or both fail or none fails) = 1
P (alternator 1 or 2 fail) = P(1 fails) or P(2 fails) = 0.02
P (Alternator 1 or 2 works well) = P(1 works) or P(2 works) = 1 - 0.02 = 0.98
Probability that both alternator fails = P(1 fails) * P(2 fails) (Anderberg, 2014)
= 0.02*0.02
Mode: Easily spotted.
Mode: Two common scores create
multimodal result..
III Mean: Very useful central
tendency result.
Median: Minimized skewed
distribution errors
Mode: Mode easily spot able.
Mean: Competitive scores affect the
average score.
Median: Close scattering of scores.
Mode: Small sample of frequency.
IV Mean: Blend for average score
of class.
Median: The mid value
minimize the error in skewed
distribution
Mode: Couldnot be spotted.
Mean: Very scattered scores does not
portray correct potential of class.
Median: Mid values too high than least
values.
Mode: No frequency of two similar scores
could be spotted
Are the data symmetric or skewed? If skewed, which direction?
Data of quiz II, III and I is symmetric in order of its mention with the long tail of skewness
extending to right. However the scores of quiz IV is very asymmetric with higher levels of
variation and differences and also median is higher than mean, resulting into data skewed to
left.
Briefly describe and compare student performance on each quiz.
Student performance in Quiz II is symmetrical in order with a very low difference in the
minimum and maximum score. On the other hand performance in Quiz I and II is more
competitive with higher differences in the least and the maximum score. Lastly Quiz IV
results show that many students were confident and well prepared than a few who turned out
to be a low performing in this case.
ANSWER 4
Total Probability (one of the alternator fail or both fail or none fails) = 1
P (alternator 1 or 2 fail) = P(1 fails) or P(2 fails) = 0.02
P (Alternator 1 or 2 works well) = P(1 works) or P(2 works) = 1 - 0.02 = 0.98
Probability that both alternator fails = P(1 fails) * P(2 fails) (Anderberg, 2014)
= 0.02*0.02
⊘ This is a preview!⊘
Do you want full access?
Subscribe today to unlock all pages.

Trusted by 1+ million students worldwide
1 out of 15
Related Documents
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
Copyright © 2020–2025 A2Z Services. All Rights Reserved. Developed and managed by ZUCOL.



