Mechanical Engineering: Thermodynamics and Engine Analysis Homework

This document presents a detailed solution to a mechanical engineering homework assignment focusing on thermodynamics and engine analysis. The solution encompasses a variety of problems, including calculations related to adiabatic processes, internal energy changes, and work done in th...Read More

4) i)
γ= C p
Cv
γ= 1
0.72 =1.389
Pbottom
1
γ V bottom=Ptop
1
γ V top
V bottom
V top
= 10
1
V bottom
V top
= Ptop
Pbottom
1
γ =¿ Ptop
Pbottom
1
γ =10
Ptop
Pbottom
=101.389=24.49
Pbottom=0.286 MPa
Similarly,
T bottom
1
γ−1 V bottom=T top
1
γ −1 V top
Ttop
Tbottom
1
γ −1 =10
Ttop
Tbottom
=100.389=2.45
T bottom=723.673 K =450.67℃
iii)
∆ U =Cv ∆T
∆ T = ( 450.67−1500 ) ℃=−1049.33 K
Cv=0.72 KJ /kgK
∆ U =0.72 × (−1049.33 ) =−755.515 KJ /kg
ii)
For adiabatic process,
∆ W =−∆U
∆ W =755.515 KJ /kg
iv)
Since it is an adiabatic process heat flow is 0.

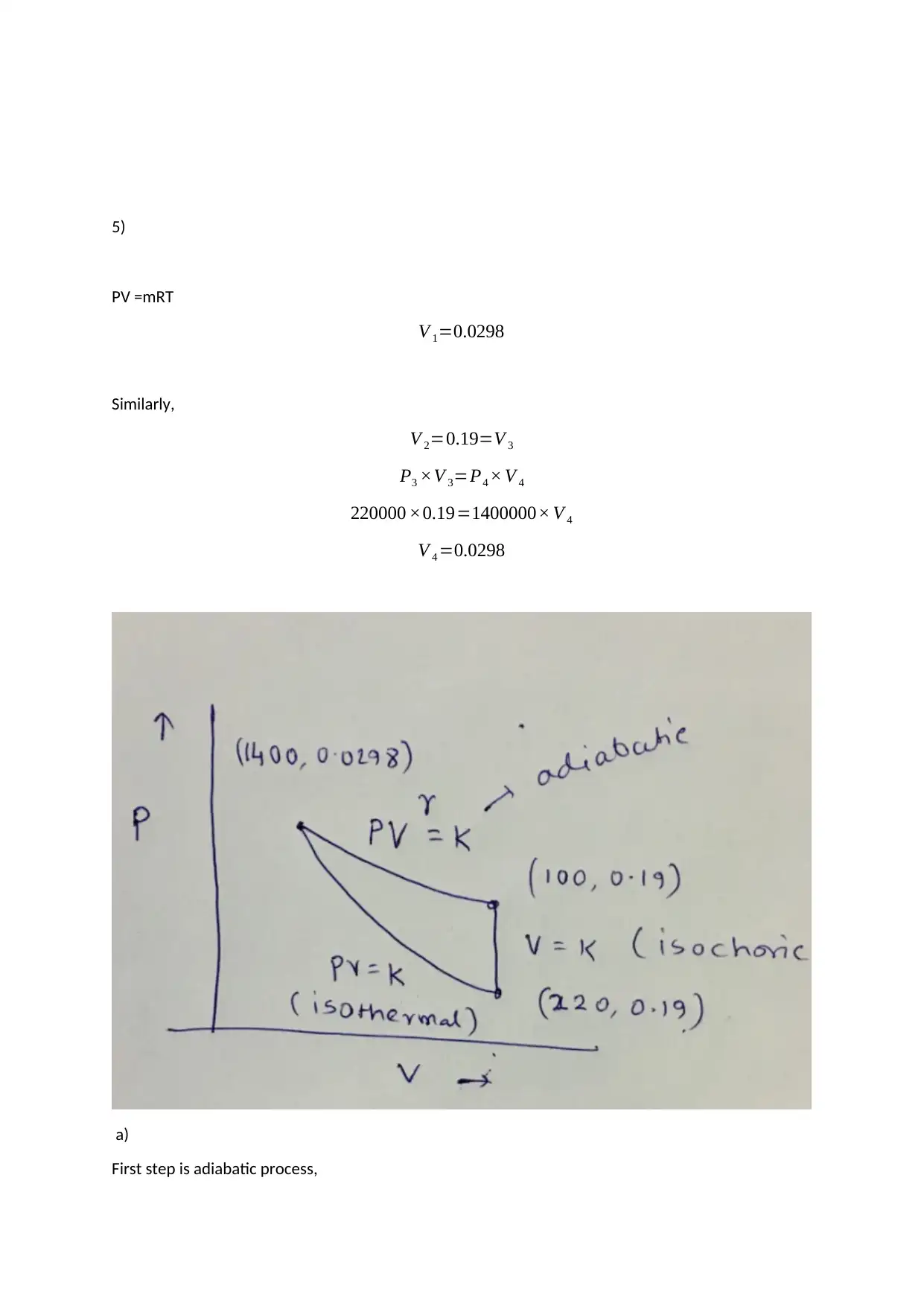
5)
PV =mRT
V 1=0.0298
Similarly,
V 2=0.19=V 3
P3 ×V 3=P4 × V 4
220000 ×0.19=1400000× V 4
V 4 =0.0298
a)
First step is adiabatic process,

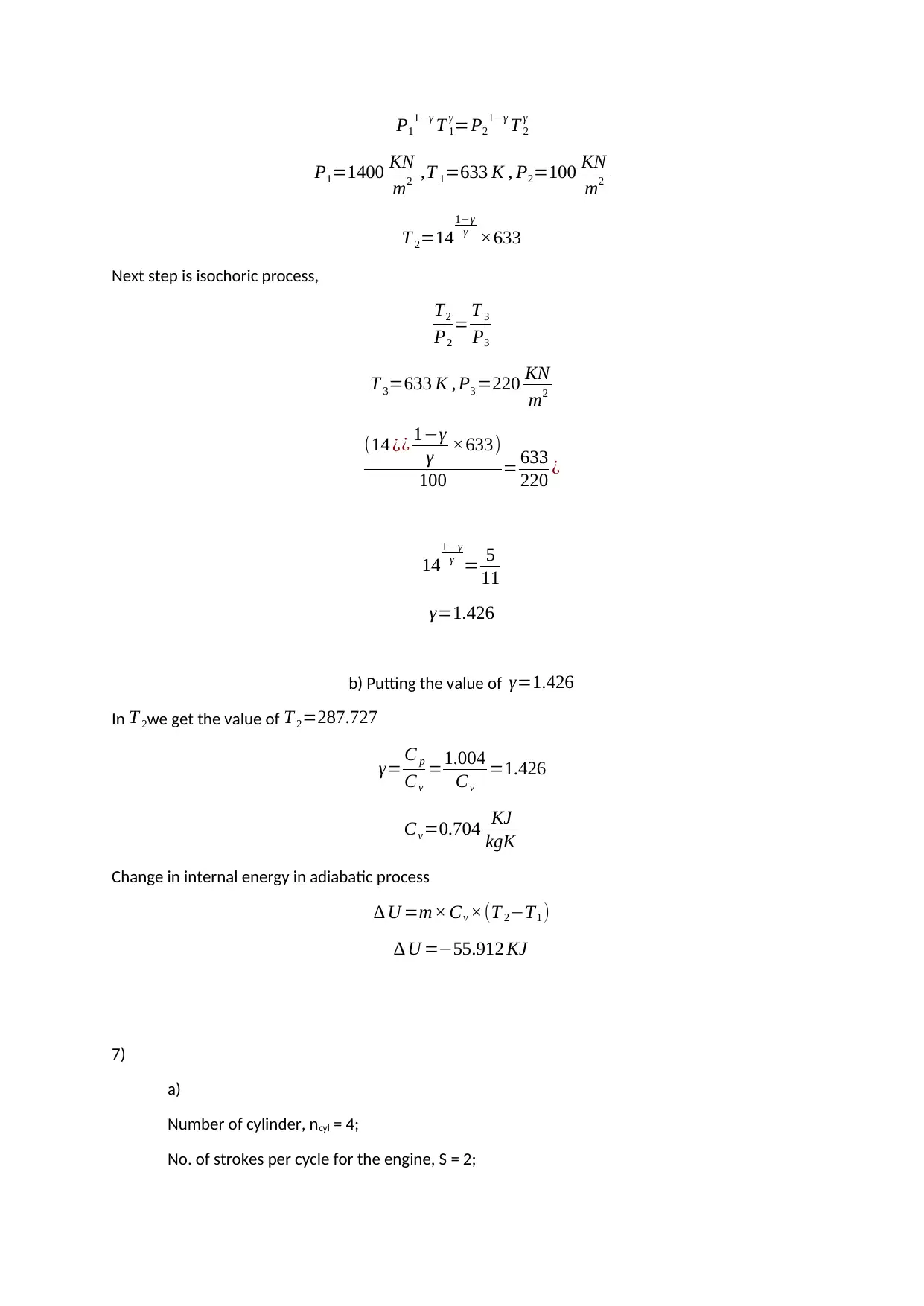
P1
1−γ T 1
γ =P2
1−γ T 2
γ
P1=1400 KN
m2 ,T 1=633 K , P2=100 KN
m2
T 2=14
1−γ
γ ×633
Next step is isochoric process,
T2
P2
= T 3
P3
T 3=633 K , P3 =220 KN
m2
(14 ¿¿ 1−γ
γ ×633)
100 = 633
220 ¿
14
1− γ
γ = 5
11
γ=1.426
b) Putting the value of γ=1.426
In T 2we get the value of T 2=287.727
γ= C p
Cv
=1.004
Cv
=1.426
Cv=0.704 KJ
kgK
Change in internal energy in adiabatic process
∆ U =m× Cv ×(T 2−T1 )
∆ U =−55.912 KJ
7)
a)
Number of cylinder, ncyl = 4;
No. of strokes per cycle for the engine, S = 2;

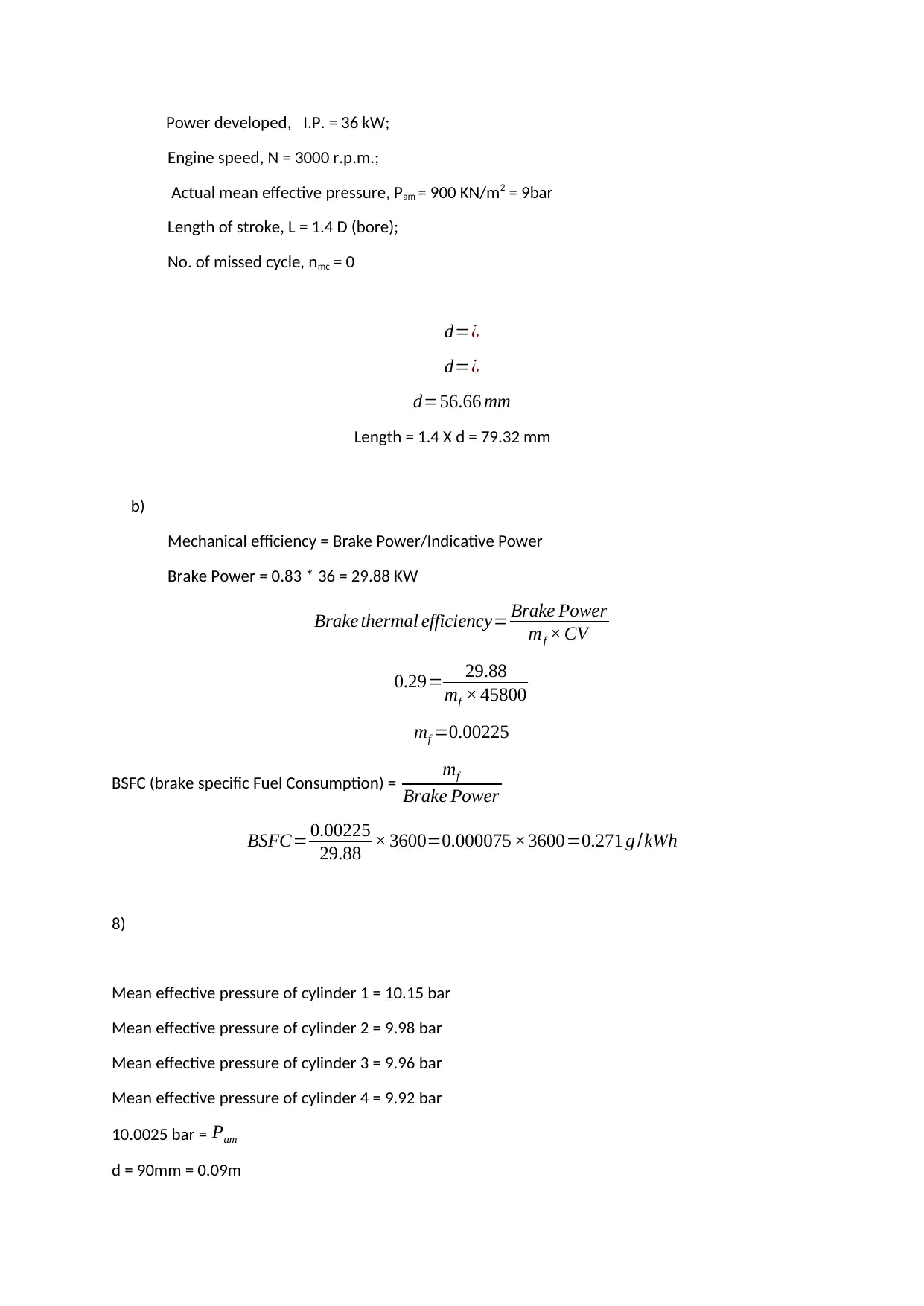
Power developed, I.P. = 36 kW;
Engine speed, N = 3000 r.p.m.;
Actual mean effective pressure, Pam = 900 KN/m2 = 9bar
Length of stroke, L = 1.4 D (bore);
No. of missed cycle, nmc = 0
d=¿
d=¿
d=56.66 mm
Length = 1.4 X d = 79.32 mm
b)
Mechanical efficiency = Brake Power/Indicative Power
Brake Power = 0.83 * 36 = 29.88 KW
Brake thermal efficiency= Brake Power
mf × CV
0.29= 29.88
mf × 45800
mf =0.00225
BSFC (brake specific Fuel Consumption) = mf
Brake Power
BSFC= 0.00225
29.88 × 3600=0.000075 ×3600=0.271 g /kWh
8)
Mean effective pressure of cylinder 1 = 10.15 bar
Mean effective pressure of cylinder 2 = 9.98 bar
Mean effective pressure of cylinder 3 = 9.96 bar
Mean effective pressure of cylinder 4 = 9.92 bar
10.0025 bar = Pam
d = 90mm = 0.09m

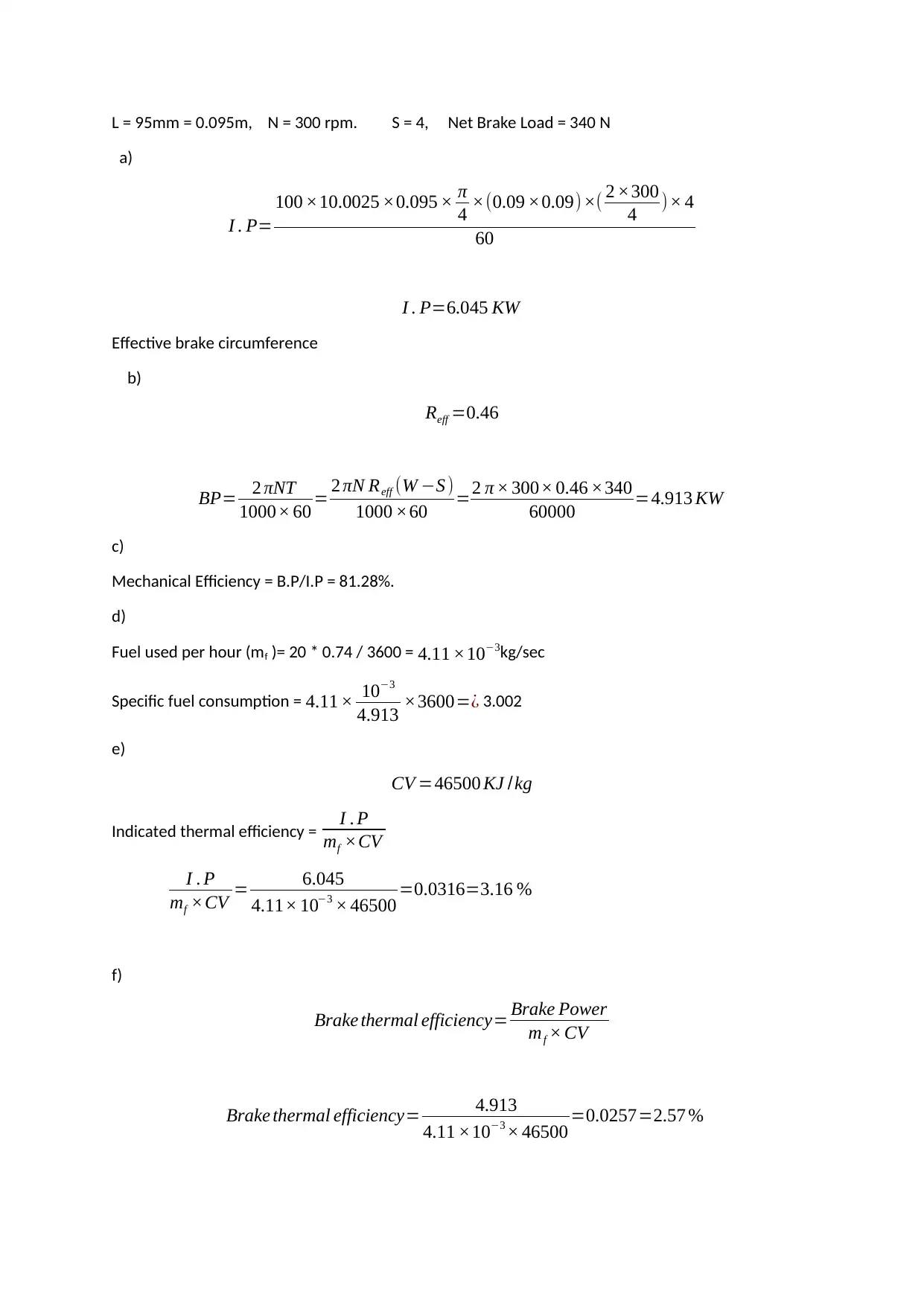
L = 95mm = 0.095m, N = 300 rpm. S = 4, Net Brake Load = 340 N
a)
I . P=
100 ×10.0025 ×0.095 × π
4 ×(0.09 ×0.09)×( 2 ×300
4 )× 4
60
I . P=6.045 KW
Effective brake circumference
b)
Reff =0.46
BP= 2 πNT
1000× 60 = 2 πN Reff (W −S )
1000 ×60 =2 π × 300× 0.46 ×340
60000 =4.913 KW
c)
Mechanical Efficiency = B.P/I.P = 81.28%.
d)
Fuel used per hour (mf )= 20 * 0.74 / 3600 = 4.11 ×10−3kg/sec
Specific fuel consumption = 4.11 × 10−3
4.913 ×3600=¿ 3.002
e)
CV =46500 KJ /kg
Indicated thermal efficiency = I . P
mf ×CV
I . P
mf ×CV = 6.045
4.11× 10−3 × 46500 =0.0316=3.16 %
f)
Brake thermal efficiency= Brake Power
mf × CV
Brake thermal efficiency= 4.913
4.11 ×10−3 × 46500 =0.0257=2.57 %