Bending Moment and Shear Force with Beam Design Consideration
VerifiedAdded on 2023/06/03
|31
|4112
|413
AI Summary
This text explains the calculation of bending moment and shear force for different beam arrangements. It also discusses the statutory requirements for structural design, dead load, live load, and factor of safety. Additionally, it calculates the maximum deflection for a simply supported beam. The output is in JSON format.
Contribute Materials
Your contribution can guide someone’s learning journey. Share your
documents today.

BENDING MOMENT
&
SHEAR FORCE
WITH
BEAM DESIGN CONSIDERATION
Page | 1
&
SHEAR FORCE
WITH
BEAM DESIGN CONSIDERATION
Page | 1
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

TASK 1
TASK 1(A) P(1)
a) A simply supported beam of length is 14 meters and carries 150 kN load at its center.
The beam arrangement is shown below:-
Figure 1.1 Beam arrangement
Calculation for reaction at each support .
ΣMA = 0
The moments about point A is shown below:
- P1*7 + RB*14 = 0
ΣMB = 0:
The moments about point B is shown below:
- RA*14 + P1*7 = 0
Reaction at support B
RB = ( P1*7) / 14 = ( 150*7) / 14
RB = 75.00 (kN)
Reaction at support A
RA = ( P1*7) / 14 = ( 150*7) / 14 = 75.00 (kN)
RA = 75.00 (kN)
Page | 2
TASK 1(A) P(1)
a) A simply supported beam of length is 14 meters and carries 150 kN load at its center.
The beam arrangement is shown below:-
Figure 1.1 Beam arrangement
Calculation for reaction at each support .
ΣMA = 0
The moments about point A is shown below:
- P1*7 + RB*14 = 0
ΣMB = 0:
The moments about point B is shown below:
- RA*14 + P1*7 = 0
Reaction at support B
RB = ( P1*7) / 14 = ( 150*7) / 14
RB = 75.00 (kN)
Reaction at support A
RA = ( P1*7) / 14 = ( 150*7) / 14 = 75.00 (kN)
RA = 75.00 (kN)
Page | 2

As load is acting at centre of beam therefore, both support share equal reaction.
Calculations for shear force and bending moment diagram (Hibbeler, 2008)
For left half of beam
Equation for Shear force (Q)
Q(x1) = + RA
Shear force values at the edges of beam
Q1(0) = + 75 = 75 (kN)
Q1(7) = + 75 = 75 (kN)
Equation for bending moment (M)
M(x1) = + RA*(x1)
Bending moment values at the edges of beam
M1(0) = + 75*(0) = 0 (kN*m)
M1(7) = + 75*(7) = 525 (kN*m)
For right half of beam
Equation for Shear force (Q)
Q(x2) = + RA - P1
Q2(7) = + 75 - 150 = -75 (kN)
Q2(14) = + 75 - 150 = -75 (kN)
Equation for bending moment (M)
M(x2) = + RA*(x2) - P1*(x2 - 7)
Bending moment values at the edges of beam
M2(7) = + 75*(7) - 150*(7 - 7) = 525 (kN*m)
M2(14) = + 75*(14) - 150*(14 - 7) = 0 (kN*m)
From the shear force and bending moment values calculated, shear force and bending moment
diagram shown below :-
Page | 3
Calculations for shear force and bending moment diagram (Hibbeler, 2008)
For left half of beam
Equation for Shear force (Q)
Q(x1) = + RA
Shear force values at the edges of beam
Q1(0) = + 75 = 75 (kN)
Q1(7) = + 75 = 75 (kN)
Equation for bending moment (M)
M(x1) = + RA*(x1)
Bending moment values at the edges of beam
M1(0) = + 75*(0) = 0 (kN*m)
M1(7) = + 75*(7) = 525 (kN*m)
For right half of beam
Equation for Shear force (Q)
Q(x2) = + RA - P1
Q2(7) = + 75 - 150 = -75 (kN)
Q2(14) = + 75 - 150 = -75 (kN)
Equation for bending moment (M)
M(x2) = + RA*(x2) - P1*(x2 - 7)
Bending moment values at the edges of beam
M2(7) = + 75*(7) - 150*(7 - 7) = 525 (kN*m)
M2(14) = + 75*(14) - 150*(14 - 7) = 0 (kN*m)
From the shear force and bending moment values calculated, shear force and bending moment
diagram shown below :-
Page | 3

Figure 1.2 SF & BM Diagram (Robert ,2012)
Page | 4
Page | 4
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

b) A simply supported beam of length is 8 meters and carries 65 kN load at 2 m from the left of
support.
The beam arrangement is shown below :-
Figure 1.3 Beam arrangement
Calculation for reaction at each support .
ΣMA = 0
The moments about point A is shown below:
- P1*2 + RB*8 = 0
ΣMB = 0:
The moments about point B is shown below:
- RA*8 + P1*6 = 0
Reaction at support B
RB = ( P1*2) / 8 = ( 65*2) / 8 = 16.25 (kN)
RB = 16.25 (kN)
Reaction at support A
RA = ( P1*6) / 8 = ( 65*6) / 8 = 48.75 (kN)
RA = 48.75 (kN)
As load is acting at left side of beam therefore, left support having more reaction.
Page | 5
support.
The beam arrangement is shown below :-
Figure 1.3 Beam arrangement
Calculation for reaction at each support .
ΣMA = 0
The moments about point A is shown below:
- P1*2 + RB*8 = 0
ΣMB = 0:
The moments about point B is shown below:
- RA*8 + P1*6 = 0
Reaction at support B
RB = ( P1*2) / 8 = ( 65*2) / 8 = 16.25 (kN)
RB = 16.25 (kN)
Reaction at support A
RA = ( P1*6) / 8 = ( 65*6) / 8 = 48.75 (kN)
RA = 48.75 (kN)
As load is acting at left side of beam therefore, left support having more reaction.
Page | 5

Calculations for shear force and bending moment diagram
For left half of beam
Equation for Shear force (Q)
Q(x1) = + RA
Shear force values at the edges of beam
Q1(0) = + 48.75 = 48.75 (kN)
Q1(2) = + 48.75 = 48.75 (kN)
Equation for bending moment (M)
M(x1) = + RA*(x1)
Bending moment values at the edges of beam
M1(0) = + 48.75*(0) = 0 (kN*m)
M1(2) = + 48.75*(2) = 97.50 (kN*m)
For right half of beam
Equation for Shear force (Q)
Q(x2) = + RA - P1
Q2(2) = + 48.75 - 65 = -16.25 (kN)
Q2(8) = + 48.75 - 65 = -16.25 (kN)
Equation for bending moment (M)
M(x2) = + RA*(x2) - P1*(x2 - 2)
Bending moment values at the edges of beam
M2(2) = + 48.75*(2) - 65*(2 - 2) = 97.50 (kN*m)
M2(8) = + 48.75*(8) - 65*(8 - 2) = 0 (kN*m)
From the shear force and bending moment values calculated, shear force and bending moment
diagram shown below :-
Page | 6
For left half of beam
Equation for Shear force (Q)
Q(x1) = + RA
Shear force values at the edges of beam
Q1(0) = + 48.75 = 48.75 (kN)
Q1(2) = + 48.75 = 48.75 (kN)
Equation for bending moment (M)
M(x1) = + RA*(x1)
Bending moment values at the edges of beam
M1(0) = + 48.75*(0) = 0 (kN*m)
M1(2) = + 48.75*(2) = 97.50 (kN*m)
For right half of beam
Equation for Shear force (Q)
Q(x2) = + RA - P1
Q2(2) = + 48.75 - 65 = -16.25 (kN)
Q2(8) = + 48.75 - 65 = -16.25 (kN)
Equation for bending moment (M)
M(x2) = + RA*(x2) - P1*(x2 - 2)
Bending moment values at the edges of beam
M2(2) = + 48.75*(2) - 65*(2 - 2) = 97.50 (kN*m)
M2(8) = + 48.75*(8) - 65*(8 - 2) = 0 (kN*m)
From the shear force and bending moment values calculated, shear force and bending moment
diagram shown below :-
Page | 6

Figure 1.4 SF & BM Diagram
Page | 7
Page | 7
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

c) A simply supported beam of length is 25 meters and carries uniform distributed load of 25
kN/m load .
The beam arrangement is shown below :-
Figure 1.5 Beam arrangement
Calculation for reaction at each support .
ΣMA = 0
The moments about point A is shown below:
- q1*25*(25/2) + RB*25 = 0
ΣMB = 0:
The moments about point B is shown below:
- RA*25 + q1*25*(25 - 25/2) = 0
Reaction at support B
RB = ( q1*25*(25/2)) / 25 = ( 25*25*(25/2)) / 25 = 312.50 (kN)
RB = 312.5 (kN)
Reaction at support A
RA = ( q1*25*(25 - 25/2)) / 25 = ( 25*25*(25 - 25/2)) / 25 = 312.50 (kN)
RA = 312.5 (kN)
Page | 8
kN/m load .
The beam arrangement is shown below :-
Figure 1.5 Beam arrangement
Calculation for reaction at each support .
ΣMA = 0
The moments about point A is shown below:
- q1*25*(25/2) + RB*25 = 0
ΣMB = 0:
The moments about point B is shown below:
- RA*25 + q1*25*(25 - 25/2) = 0
Reaction at support B
RB = ( q1*25*(25/2)) / 25 = ( 25*25*(25/2)) / 25 = 312.50 (kN)
RB = 312.5 (kN)
Reaction at support A
RA = ( q1*25*(25 - 25/2)) / 25 = ( 25*25*(25 - 25/2)) / 25 = 312.50 (kN)
RA = 312.5 (kN)
Page | 8

As load is acting uniform on the beam therefore, both support share equal reaction.
Calculations for shear force and bending moment diagram
For full beam
Equation for Shear force (Q)
Q(x1) = + RA - q1*(x1 - 0)
Shear force values at the edges of beam
Q1(0) = + 312.50 - 25*(0 - 0) = 312.50 (kN)
Q1(25) = + 312.50 - 25*(25 - 0) = -312.50 (kN)
Equation for bending moment (M)
M(x1) = + RA*(x1) - q1*(x1)2/2
Bending moment values at the edges of beam
M1(0) = + 312.50*(0) - 25*(0 - 0)2/2 = 0 (kN*m)
M1(25) = + 312.50*(25) - 25*(25 - 0)2/2 = 0 (kN*m)
Point at which shear force is zero having bending moment maximum is point of contraflexure
x = 12.50
Point of contraflexure point x = 12.50:
Maximum bending moment
M1(12.50) = + 312.50*(12.50) - 25*(12.50 - 0)2/2 = 1953.13 (kN*m)
From the shear force and bending moment values calculated, shear force and bending moment
diagram shown below :-
Page | 9
Calculations for shear force and bending moment diagram
For full beam
Equation for Shear force (Q)
Q(x1) = + RA - q1*(x1 - 0)
Shear force values at the edges of beam
Q1(0) = + 312.50 - 25*(0 - 0) = 312.50 (kN)
Q1(25) = + 312.50 - 25*(25 - 0) = -312.50 (kN)
Equation for bending moment (M)
M(x1) = + RA*(x1) - q1*(x1)2/2
Bending moment values at the edges of beam
M1(0) = + 312.50*(0) - 25*(0 - 0)2/2 = 0 (kN*m)
M1(25) = + 312.50*(25) - 25*(25 - 0)2/2 = 0 (kN*m)
Point at which shear force is zero having bending moment maximum is point of contraflexure
x = 12.50
Point of contraflexure point x = 12.50:
Maximum bending moment
M1(12.50) = + 312.50*(12.50) - 25*(12.50 - 0)2/2 = 1953.13 (kN*m)
From the shear force and bending moment values calculated, shear force and bending moment
diagram shown below :-
Page | 9

Figure 1.6 SF & BM Diagram
Page | 10
Page | 10
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

d) A simply supported beam of length is 14 meters and carries point load of 150 kN and also
uniform distributed load of 25 kN/m load .
Shear force and bending moment diagram shown below :-
Figure 1.7 SF & BM Diagram
Page | 11
uniform distributed load of 25 kN/m load .
Shear force and bending moment diagram shown below :-
Figure 1.7 SF & BM Diagram
Page | 11

e) A simply supported beam of length is 25 meters and carries uniform distributed load of 25
kN/m load and 50kN at 10 m from right side side .
Shear force and bending moment diagram shown below :-
Figure 1.8 SF & BM Diagram
Page | 12
kN/m load and 50kN at 10 m from right side side .
Shear force and bending moment diagram shown below :-
Figure 1.8 SF & BM Diagram
Page | 12

TASK 1 (B)
Statutory requirements to ensure safety in structural design (R.C ,2009).
Structural safety- This includes design safety of structures .While designing the
structure ,designer need to ensure it safety by considering all necessary parameters like
dead loads , live loads , seismic loads ,wind loads and snow loads .
Fire safety –Location of structures need to be fire proof and fire extinguisher need to
kept around buildings /structures.
Air ventilation –As wind loading is one of most important factor for structure design ,so
proper ventilation channelize the air flow .
Electricity safety –It is to ensure that structures and building should be shock proof .
Sound resistant safety –Buildings and structures near porsche areas need to take care
of sound safety .
Some of other requirements are:-
Environmental impacts
Sanitation
Drainage system
Building access
TASK 1 (C)
Dead load –This is the permanent load having constant magnitude and its position also fixed ,
It includes weight of the parts of structure .
Live load - This is the temporary load which may be either constant or variable in magnitude
and its position also fixed or may vary with time , It generates when the system is in
operation/working .
Factor of safety- It is the safety factor considered while designing the structure so that any
fluctuation in load do not harm the structure (Johnston, 2010) .
According to standard codes
Dead load (final) = 1.4 x actual dead load
Live load (final) = 1.7 x actual live load
Page | 13
Statutory requirements to ensure safety in structural design (R.C ,2009).
Structural safety- This includes design safety of structures .While designing the
structure ,designer need to ensure it safety by considering all necessary parameters like
dead loads , live loads , seismic loads ,wind loads and snow loads .
Fire safety –Location of structures need to be fire proof and fire extinguisher need to
kept around buildings /structures.
Air ventilation –As wind loading is one of most important factor for structure design ,so
proper ventilation channelize the air flow .
Electricity safety –It is to ensure that structures and building should be shock proof .
Sound resistant safety –Buildings and structures near porsche areas need to take care
of sound safety .
Some of other requirements are:-
Environmental impacts
Sanitation
Drainage system
Building access
TASK 1 (C)
Dead load –This is the permanent load having constant magnitude and its position also fixed ,
It includes weight of the parts of structure .
Live load - This is the temporary load which may be either constant or variable in magnitude
and its position also fixed or may vary with time , It generates when the system is in
operation/working .
Factor of safety- It is the safety factor considered while designing the structure so that any
fluctuation in load do not harm the structure (Johnston, 2010) .
According to standard codes
Dead load (final) = 1.4 x actual dead load
Live load (final) = 1.7 x actual live load
Page | 13
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
For example
A simply supported beam of length is 14 meters and carries 150 kN load at its center.
According to standard codes
Dead load (final) = 1.4 x actual dead load
Dead load (final) = 1.4 *150 = 210 kN
Live load (final) = 1.7 x actual live load
Live load (final) = 1.7 x 150 = 255 kN
For designing the above beam , its dead load is considered to be 210 kN and live load will be
255kN .
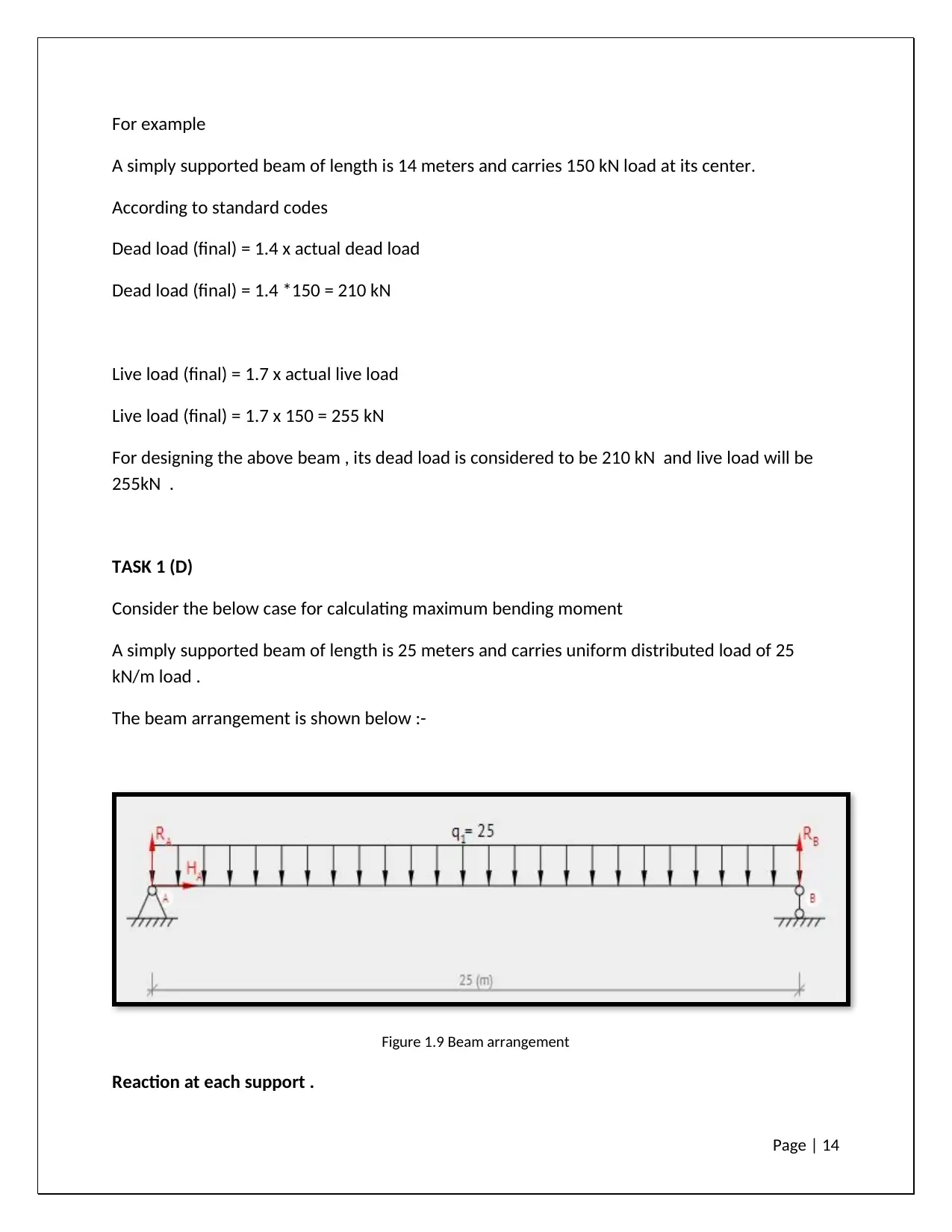
TASK 1 (D)
Consider the below case for calculating maximum bending moment
A simply supported beam of length is 25 meters and carries uniform distributed load of 25
kN/m load .
The beam arrangement is shown below :-
Figure 1.9 Beam arrangement
Reaction at each support .
Page | 14
A simply supported beam of length is 14 meters and carries 150 kN load at its center.
According to standard codes
Dead load (final) = 1.4 x actual dead load
Dead load (final) = 1.4 *150 = 210 kN
Live load (final) = 1.7 x actual live load
Live load (final) = 1.7 x 150 = 255 kN
For designing the above beam , its dead load is considered to be 210 kN and live load will be
255kN .
TASK 1 (D)
Consider the below case for calculating maximum bending moment
A simply supported beam of length is 25 meters and carries uniform distributed load of 25
kN/m load .
The beam arrangement is shown below :-
Figure 1.9 Beam arrangement
Reaction at each support .
Page | 14

RB = 312.5 (kN)
RA = 312.5 (kN)
As load is acting uniform on the beam therefore, both support share equal reaction.
Calculations for shear force and bending moment diagram
Shear force
Q1(0) = + 312.50 - 25*(0 - 0) = 312.50 (kN)
Q1(25) = + 312.50 - 25*(25 - 0) = -312.50 (kN)
Point of contraflexure point x = 12.50:
Maximum bending moment
M1 = 1953.13 (kN*m)
Considering current codes using bending moment equation
M/ I = S / y = E / r
M/ I = S / y
M = Maximum bending moment
I = Moment of inertia of beam section
S = Allowable stress
Y =Distance from neural axis
The above figure shows the variation of bending stress in rectangular profile
S =M X y /I
Page | 15
S max
S min
S max
y
RA = 312.5 (kN)
As load is acting uniform on the beam therefore, both support share equal reaction.
Calculations for shear force and bending moment diagram
Shear force
Q1(0) = + 312.50 - 25*(0 - 0) = 312.50 (kN)
Q1(25) = + 312.50 - 25*(25 - 0) = -312.50 (kN)
Point of contraflexure point x = 12.50:
Maximum bending moment
M1 = 1953.13 (kN*m)
Considering current codes using bending moment equation
M/ I = S / y = E / r
M/ I = S / y
M = Maximum bending moment
I = Moment of inertia of beam section
S = Allowable stress
Y =Distance from neural axis
The above figure shows the variation of bending stress in rectangular profile
S =M X y /I
Page | 15
S max
S min
S max
y
According to above formula
Maximum bending stress is directly proportional to bending moment and y value and inversely
proportional moment of inertia .
For steel, bending stress is maximum at extreme fibers as shown in diagram above .More be
the moment of inertia of steel section less be the bending stress .
So generally rectangular , I section , t section , L sections are preferred over circular or square
section .
TASK 2
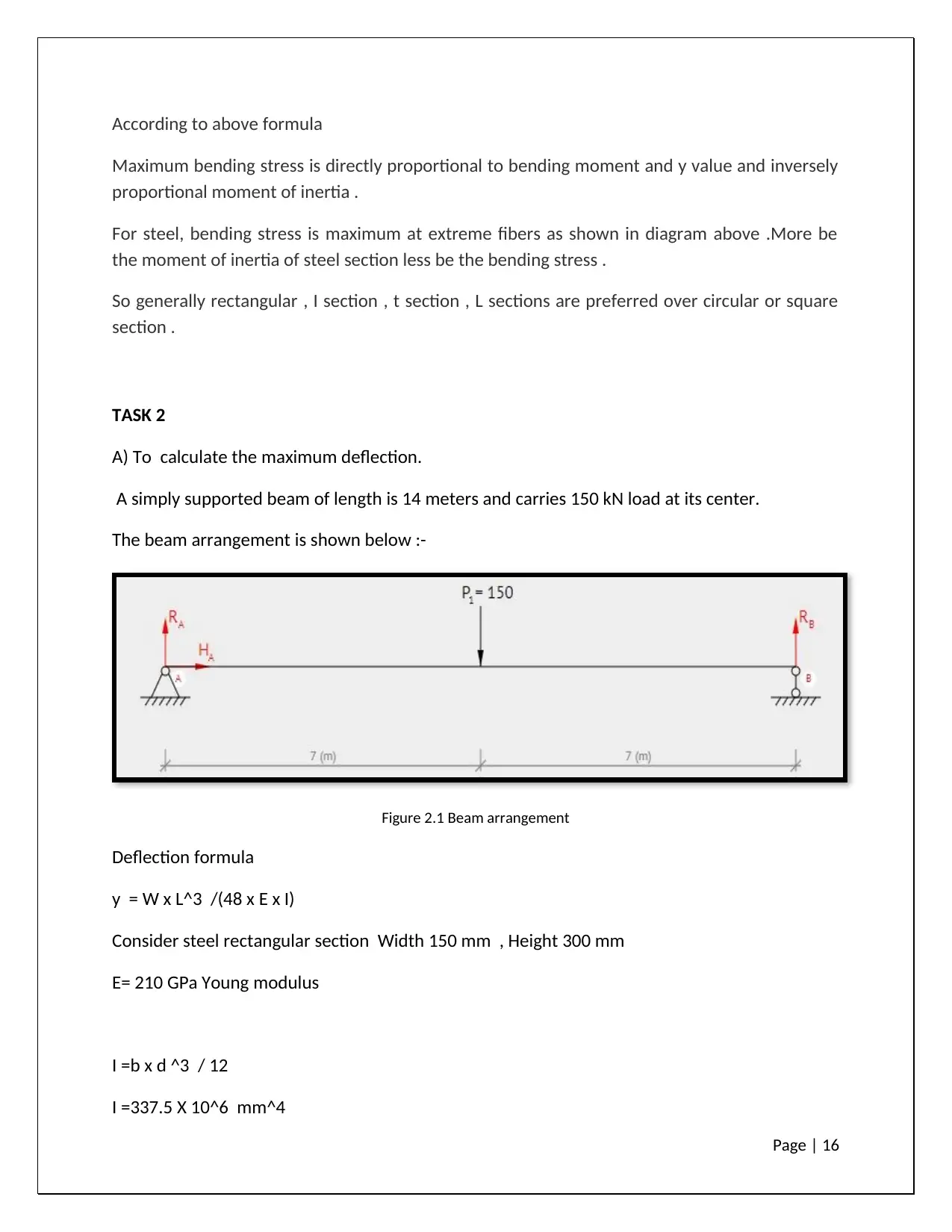
A) To calculate the maximum deflection.
A simply supported beam of length is 14 meters and carries 150 kN load at its center.
The beam arrangement is shown below :-
Figure 2.1 Beam arrangement
Deflection formula
y = W x L^3 /(48 x E x I)
Consider steel rectangular section Width 150 mm , Height 300 mm
E= 210 GPa Young modulus
I =b x d ^3 / 12
I =337.5 X 10^6 mm^4
Page | 16
Maximum bending stress is directly proportional to bending moment and y value and inversely
proportional moment of inertia .
For steel, bending stress is maximum at extreme fibers as shown in diagram above .More be
the moment of inertia of steel section less be the bending stress .
So generally rectangular , I section , t section , L sections are preferred over circular or square
section .
TASK 2
A) To calculate the maximum deflection.
A simply supported beam of length is 14 meters and carries 150 kN load at its center.
The beam arrangement is shown below :-
Figure 2.1 Beam arrangement
Deflection formula
y = W x L^3 /(48 x E x I)
Consider steel rectangular section Width 150 mm , Height 300 mm
E= 210 GPa Young modulus
I =b x d ^3 / 12
I =337.5 X 10^6 mm^4
Page | 16
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

y = W x L^3 /(48 x E x I)
y = 150 x 1000 x 14000^3 /(48 x 210 x 1000 x 337.5X 10^6 )
y = 0.1 mm
A simply supported beam of length is 25 meters and carries uniform distributed load of 25
kN/m load .
The beam arrangement is shown below :-
Figure 2.2 Beam arrangement
Deflection formula
y = 5W x L^4 /(384 x E x I)
Consider steel rectangular section Width 150 mm , Height 300 mm
E= 210 GPa Young modulus
I =b x d ^3 / 12
I =337.5 X 10^6 mm^4
Page | 17
y = 150 x 1000 x 14000^3 /(48 x 210 x 1000 x 337.5X 10^6 )
y = 0.1 mm
A simply supported beam of length is 25 meters and carries uniform distributed load of 25
kN/m load .
The beam arrangement is shown below :-
Figure 2.2 Beam arrangement
Deflection formula
y = 5W x L^4 /(384 x E x I)
Consider steel rectangular section Width 150 mm , Height 300 mm
E= 210 GPa Young modulus
I =b x d ^3 / 12
I =337.5 X 10^6 mm^4
Page | 17

y = 5W x L^4 /(384 x E x I)
y = 5 x (25 x 1000) x 25000^4 /(384 x 210 x 1000 x 337.5X 10^6 )
y = 0.45 mm
B) Deflection is the deviation of beam from neutral axis in y direction due to application of
transverse load .
Deflection formula for simply supported beam at point loading .
y = W x L^3 /(48 x E x I)
From the above formula , deflection is directly proportional to applied load and length of beam
but it is inversely proportional to flexural rigidity (E X I) .
E is young modulus and depend on material , I is moment of inertia and depend on beam
profile .More be the product of E & I therefore less be the deflection .So for structural designing
, generally steel beams with I , T sections used as these combination have high flexural rigidity
(E X I) .
(C) Methods of supports.
Figure 2.3 Supports
As the load act on the beam then supports externally provided on beam to carry that
load .There are different types of support used for structural design .
Page | 18
y = 5 x (25 x 1000) x 25000^4 /(384 x 210 x 1000 x 337.5X 10^6 )
y = 0.45 mm
B) Deflection is the deviation of beam from neutral axis in y direction due to application of
transverse load .
Deflection formula for simply supported beam at point loading .
y = W x L^3 /(48 x E x I)
From the above formula , deflection is directly proportional to applied load and length of beam
but it is inversely proportional to flexural rigidity (E X I) .
E is young modulus and depend on material , I is moment of inertia and depend on beam
profile .More be the product of E & I therefore less be the deflection .So for structural designing
, generally steel beams with I , T sections used as these combination have high flexural rigidity
(E X I) .
(C) Methods of supports.
Figure 2.3 Supports
As the load act on the beam then supports externally provided on beam to carry that
load .There are different types of support used for structural design .
Page | 18

Fixed support (cantilever) – Under this support , beam is fixed from one side and free
from other side as shown in figure above .When the motion in any direction of beam is
restricted then that restriction provide opposite reaction ,The fixed beam provide 3
reaction . One is in horizontal direction, one in vertical direction and other as moment .
Deflection for cantilever beam is less as compared to simply supported (Baumeister,
2011)
y = W x L^3 /(3 x E x I)
Roller support – Under this support , beam is supported vertically and free to move in
horizontal direction as shown in figure above .When the motion in any direction of
beam is restricted then that restriction provide opposite reaction ,Roller support
provide 1 reaction only in vertical direction.
Deflection for roller support beam is more as compared to cantilever beam .This is
because the reaction occurs in one direction.
Pinned support – Under this support , beam is supported vertically as well as in
horizontal direction as shown in figure above .When the motion in any direction of
beam is restricted then that restriction provide opposite reaction ,Roller support
provide 2 reaction one in vertical direction and other in horizontal direction
Deflection for pinned support beam is more as compared to cantilever beam .This is
because the reaction occurs in two direction.
(D) From the all mentioned supports , Fixed supports are best to use as it absorb all the forces
at the end of beam and provide least deflection .
Example
Generally fixed supports are used in structure as the foundation. In foundation , steel beam is
drilled deep inside the ground and concrete is put around the steel so that concrete kept the
steel fixed in the ground .All the load act on that steel beam transfer it to ground as the main
function of foundation is to transfer all the load to ground so that its effect on the beam
reduces .So by comparing all types of support it is preferred to used fixed beam for more safer
design .
TASK 3
Page | 19
from other side as shown in figure above .When the motion in any direction of beam is
restricted then that restriction provide opposite reaction ,The fixed beam provide 3
reaction . One is in horizontal direction, one in vertical direction and other as moment .
Deflection for cantilever beam is less as compared to simply supported (Baumeister,
2011)
y = W x L^3 /(3 x E x I)
Roller support – Under this support , beam is supported vertically and free to move in
horizontal direction as shown in figure above .When the motion in any direction of
beam is restricted then that restriction provide opposite reaction ,Roller support
provide 1 reaction only in vertical direction.
Deflection for roller support beam is more as compared to cantilever beam .This is
because the reaction occurs in one direction.
Pinned support – Under this support , beam is supported vertically as well as in
horizontal direction as shown in figure above .When the motion in any direction of
beam is restricted then that restriction provide opposite reaction ,Roller support
provide 2 reaction one in vertical direction and other in horizontal direction
Deflection for pinned support beam is more as compared to cantilever beam .This is
because the reaction occurs in two direction.
(D) From the all mentioned supports , Fixed supports are best to use as it absorb all the forces
at the end of beam and provide least deflection .
Example
Generally fixed supports are used in structure as the foundation. In foundation , steel beam is
drilled deep inside the ground and concrete is put around the steel so that concrete kept the
steel fixed in the ground .All the load act on that steel beam transfer it to ground as the main
function of foundation is to transfer all the load to ground so that its effect on the beam
reduces .So by comparing all types of support it is preferred to used fixed beam for more safer
design .
TASK 3
Page | 19
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

a) Calculate axial load carrying capacity of concrete beam
For short column
L / d < 8
3.75 /25 <8
Therfore it is short column
As strain in 2 material is same
f st/ f cr = E st / E c
f cr =0.6∗0.85∗ √ f c
'
f cr =0.6∗0.85∗ √ 40
f cr =3.225 MPa
Total load = Load on steel +Load on concrete
Load on steel Ps = f ys∗As
Load on steel Ps = 550∗2950
Load on steel Ps =1.622 MN
Load on concrete Pc = f cr∗Ac
Load on concrete Pc = 3.225∗350
Load on concrete Pc = 1.128 KN
Total load carrying capacity
Total load = 1622.1+1.128 = 1623.28 KN
b) The steel is designed according to calculated total load
Page | 20
For short column
L / d < 8
3.75 /25 <8
Therfore it is short column
As strain in 2 material is same
f st/ f cr = E st / E c
f cr =0.6∗0.85∗ √ f c
'
f cr =0.6∗0.85∗ √ 40
f cr =3.225 MPa
Total load = Load on steel +Load on concrete
Load on steel Ps = f ys∗As
Load on steel Ps = 550∗2950
Load on steel Ps =1.622 MN
Load on concrete Pc = f cr∗Ac
Load on concrete Pc = 3.225∗350
Load on concrete Pc = 1.128 KN
Total load carrying capacity
Total load = 1622.1+1.128 = 1623.28 KN
b) The steel is designed according to calculated total load
Page | 20

As total load is 1623.28 KN
f c
' =40 MPa
f ys=550 Mpa
Es=200 GPa
Ec=4500∗√ f c
'
Ec=4500∗√ 40
Ec=28.46GPa
Modular ratio = Es
Ec
n= 200
28.46 =7
Calculate stress in steel using modular ratio to equivalent the load
f s
f c
' =7
f s
40 =7
f s=40∗7=280 MPa
Now for steel , permissible stress is 280 MPa
f s= Total load
Area
280=1623.28∗1000
Area
Area=5797.4 mm2
(A) Slenderness ratio is the ratio of equivalent length to the radius of gyration
S.R = Le /K
Page | 21
f c
' =40 MPa
f ys=550 Mpa
Es=200 GPa
Ec=4500∗√ f c
'
Ec=4500∗√ 40
Ec=28.46GPa
Modular ratio = Es
Ec
n= 200
28.46 =7
Calculate stress in steel using modular ratio to equivalent the load
f s
f c
' =7
f s
40 =7
f s=40∗7=280 MPa
Now for steel , permissible stress is 280 MPa
f s= Total load
Area
280=1623.28∗1000
Area
Area=5797.4 mm2
(A) Slenderness ratio is the ratio of equivalent length to the radius of gyration
S.R = Le /K
Page | 21

Figure 3.1 Effective length
Effective length is the length formed by the beam which different supports added on the beam .
The above figure shows the column having different bucking shape for steel and concrete and
their values of effective length and the symbol used in it (Barry ,2012).
(B) Actual load carrying capacity of beam .
Load capacity Pcr = 3.14 ^2 * E * I / (Le)^2
For steel E = 200 GPa
I = 3.14 *25 ^4 /64 = 19.17 *10^3 mm^4
Consider this as both hinge joint
Le =3.75 mm
Pcr = 3.14 ^2 * E * I / (Le)^2
Pcr = 3.14 ^2 * 200*1000 * 19.17 *10^3 / (3.75)^2
Pcr = 3.69 MN
Page | 22
Effective length is the length formed by the beam which different supports added on the beam .
The above figure shows the column having different bucking shape for steel and concrete and
their values of effective length and the symbol used in it (Barry ,2012).
(B) Actual load carrying capacity of beam .
Load capacity Pcr = 3.14 ^2 * E * I / (Le)^2
For steel E = 200 GPa
I = 3.14 *25 ^4 /64 = 19.17 *10^3 mm^4
Consider this as both hinge joint
Le =3.75 mm
Pcr = 3.14 ^2 * E * I / (Le)^2
Pcr = 3.14 ^2 * 200*1000 * 19.17 *10^3 / (3.75)^2
Pcr = 3.69 MN
Page | 22
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

(C) The load carry capacity is depend on the young modulus (E) of material which is calculated
from stress strain diagram shown below .
Figure 3.2 Stress strain diagram
Different material effects on atmosphere shows its different properties .
Figure 3.3 Material effects
Page | 23
from stress strain diagram shown below .
Figure 3.2 Stress strain diagram
Different material effects on atmosphere shows its different properties .
Figure 3.3 Material effects
Page | 23

Figure 3.4 Material effects
TASK 4
Steel design
(A) Considering the case of uniformly distributed load 55KN/m and 8 m span of beam.
The profile of beam selected is Channel ISMC
Using ISMC 250 beam , the design is considered to be safe .
Figure 4.1 Stress in beams
Page | 24
TASK 4
Steel design
(A) Considering the case of uniformly distributed load 55KN/m and 8 m span of beam.
The profile of beam selected is Channel ISMC
Using ISMC 250 beam , the design is considered to be safe .
Figure 4.1 Stress in beams
Page | 24
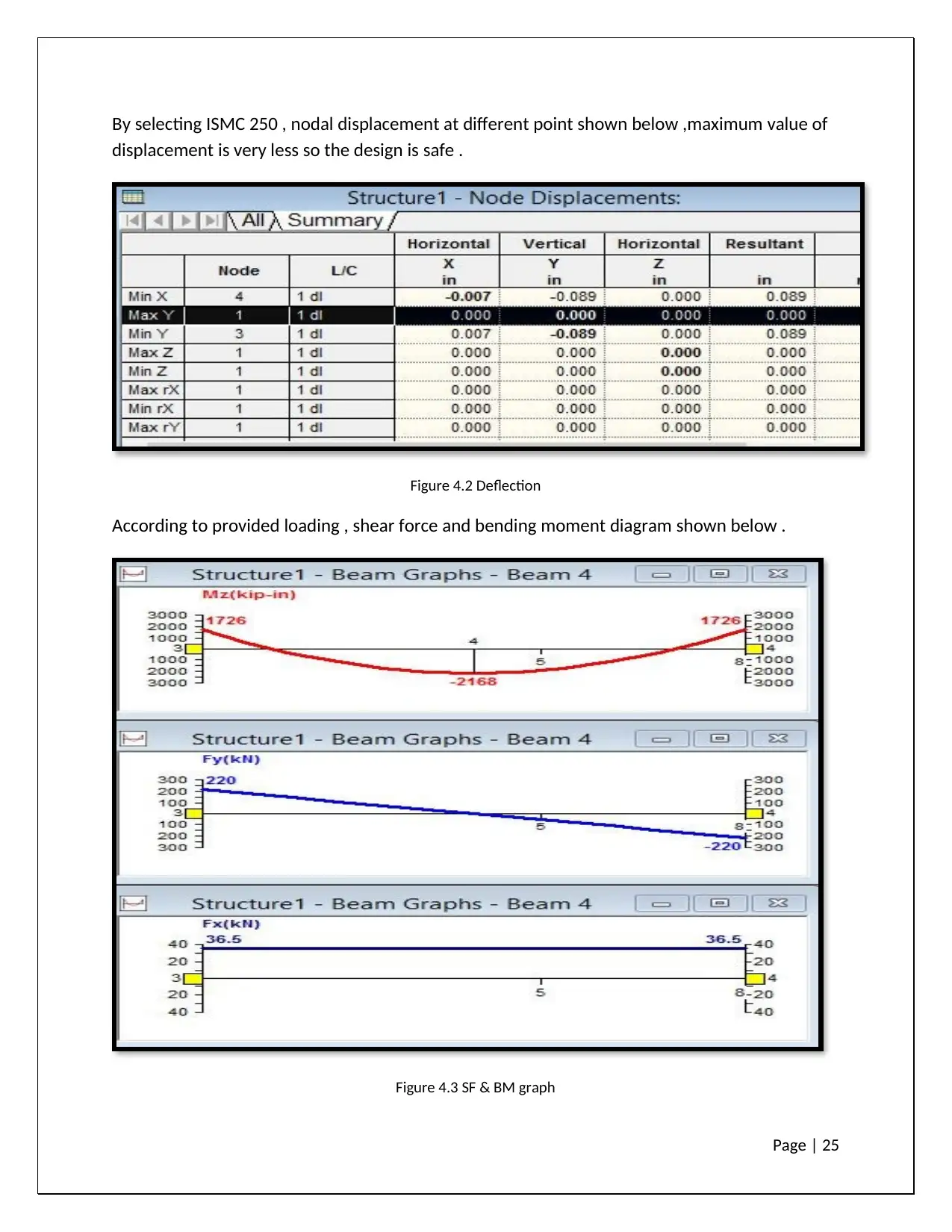
By selecting ISMC 250 , nodal displacement at different point shown below ,maximum value of
displacement is very less so the design is safe .
Figure 4.2 Deflection
According to provided loading , shear force and bending moment diagram shown below .
Figure 4.3 SF & BM graph
Page | 25
displacement is very less so the design is safe .
Figure 4.2 Deflection
According to provided loading , shear force and bending moment diagram shown below .
Figure 4.3 SF & BM graph
Page | 25
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

Cost calculation
Figure 4.4 Take off
For one side set of 3 beams
As Weight = 7.19 KN or733 Kg
Cost of beam per kg =1.2 $ per Kg
Total cost of steel beam = 733 x 1.2
Total cost of steel beam =880 $
(B) Drawing and beam profile shown below .
Figure 4.5 Profile
Page | 26
Figure 4.4 Take off
For one side set of 3 beams
As Weight = 7.19 KN or733 Kg
Cost of beam per kg =1.2 $ per Kg
Total cost of steel beam = 733 x 1.2
Total cost of steel beam =880 $
(B) Drawing and beam profile shown below .
Figure 4.5 Profile
Page | 26

Figure 4.6 Profile
Figure 4.7 Profile properties
Page | 27
Figure 4.7 Profile properties
Page | 27

(C) Except Steel and concrete some other materials can be used as structures are timber , brick
and glass .Properties of all the materials shown below .
Property of Timber
Table :4.1 (Material properties)
Property of Brick/Masonry
Table :4.2 (Material properties)
Page | 28
and glass .Properties of all the materials shown below .
Property of Timber
Table :4.1 (Material properties)
Property of Brick/Masonry
Table :4.2 (Material properties)
Page | 28
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

Property of Glass
Table :4.3 (Material properties)
After comparing the properties, glass having high tensile strength, high value of young
modulus , high density and fire resistant .so glass can be used as structure .The main drawback
of glass is its brittle property .Under brittleness , the material fail instantly after reaching its
ultimate load point .So for safety purpose glass can be used with reinforcing metal to increase
the ductility of composite material .
Page | 29
Table :4.3 (Material properties)
After comparing the properties, glass having high tensile strength, high value of young
modulus , high density and fire resistant .so glass can be used as structure .The main drawback
of glass is its brittle property .Under brittleness , the material fail instantly after reaching its
ultimate load point .So for safety purpose glass can be used with reinforcing metal to increase
the ductility of composite material .
Page | 29

(D) BUILDING INFORMATION MODELLING( BIM)
It is the process of preparing and managing the characteristics of places that are formed
and stored digitally (Kathleen ,2008).
From BIM, information can be easily extracted, used and exchanged for structural
design purpose.
There are special BIM softwares for specific task that are used by design
professionals ,organizations and government .
Planning of infrastructure can done comes under bim considering roads , railways ,
bridges ,electric supply , communication wires etc( Dobelis ,2013)
Figure 4.8 Building information modeling
Page | 30
It is the process of preparing and managing the characteristics of places that are formed
and stored digitally (Kathleen ,2008).
From BIM, information can be easily extracted, used and exchanged for structural
design purpose.
There are special BIM softwares for specific task that are used by design
professionals ,organizations and government .
Planning of infrastructure can done comes under bim considering roads , railways ,
bridges ,electric supply , communication wires etc( Dobelis ,2013)
Figure 4.8 Building information modeling
Page | 30

REFERENCES
Hibbeler, R.C.(2008) Statics and Mechanics of Materials, SI Edition. Prentice-Hall,. ISBN 0-13-
129011-8.
Kleppner, Daniel; Kolenkow, Robert (2012). An Introduction to Mechanics. McGraw-Hill.
pp. 267–68.
Beer, F.P., E.R. Johnston, et al.(2010) Mechanics of Materials, 3rd edition. McGraw-Hill,. ISBN 0-
07-248673-2
Tylecote, R. F. (2011) A history of metallurgy 2nd ed., Institute of Materials, London. pp. 95–99
and 102–105. ISBN 0901462888.
Gere, James M.; Goodno, Barry J(2012). Mechanics of Materials (Eighth ed.). pp. 1083–
1087. ISBN 978-1-111-57773-5.
Hartog Den, Jacob P.(2007) Strength of Materials. Dover Publications, Inc., ISBN 0-486-60755-0.
Hibbeler, R.C (2009). Structural Analysis. Macmillan. pp. 146–148.
Avallone A, E.A.; Baumeister, T. (2011.). Mark's Standard Handbook for Mechanical
Engineers (10th ed.). McGraw-Hill. pp. 11–42. ISBN 0-07-004997-1.
Hool C, George A.; Johnson, Nathan Clarke (2007). "Elements of Structural Theory -
Definitions". Handbook of Building Construction (Google Books). vol. 1 (1st ed.). New
York: McGraw-Hill. p. 2.
Luciani Cinti, Garagnani S., Mingucci R. (2012) "BIM tools and design intent. Limitations and
opportunities", in K. Kensek, J. Peng, Practical BIM 2012 - Management, Implementation,
Coordination and Evaluation, Los Angeles
Dobelis M. (2013), “Drawbacks of BIM concept adoption”, in the 12th International Conference
on Engineering Graphics, BALTGRAF 2013, June 5–7, 2013, Riga, Latvia
Page | 31
Hibbeler, R.C.(2008) Statics and Mechanics of Materials, SI Edition. Prentice-Hall,. ISBN 0-13-
129011-8.
Kleppner, Daniel; Kolenkow, Robert (2012). An Introduction to Mechanics. McGraw-Hill.
pp. 267–68.
Beer, F.P., E.R. Johnston, et al.(2010) Mechanics of Materials, 3rd edition. McGraw-Hill,. ISBN 0-
07-248673-2
Tylecote, R. F. (2011) A history of metallurgy 2nd ed., Institute of Materials, London. pp. 95–99
and 102–105. ISBN 0901462888.
Gere, James M.; Goodno, Barry J(2012). Mechanics of Materials (Eighth ed.). pp. 1083–
1087. ISBN 978-1-111-57773-5.
Hartog Den, Jacob P.(2007) Strength of Materials. Dover Publications, Inc., ISBN 0-486-60755-0.
Hibbeler, R.C (2009). Structural Analysis. Macmillan. pp. 146–148.
Avallone A, E.A.; Baumeister, T. (2011.). Mark's Standard Handbook for Mechanical
Engineers (10th ed.). McGraw-Hill. pp. 11–42. ISBN 0-07-004997-1.
Hool C, George A.; Johnson, Nathan Clarke (2007). "Elements of Structural Theory -
Definitions". Handbook of Building Construction (Google Books). vol. 1 (1st ed.). New
York: McGraw-Hill. p. 2.
Luciani Cinti, Garagnani S., Mingucci R. (2012) "BIM tools and design intent. Limitations and
opportunities", in K. Kensek, J. Peng, Practical BIM 2012 - Management, Implementation,
Coordination and Evaluation, Los Angeles
Dobelis M. (2013), “Drawbacks of BIM concept adoption”, in the 12th International Conference
on Engineering Graphics, BALTGRAF 2013, June 5–7, 2013, Riga, Latvia
Page | 31
1 out of 31
Related Documents
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.





