Hydrogenerator basic design calculation PDF

CHAPTER 2
HYDROGENERATOR BASIC DESIGN CALCULATION
As a basic case for the consideration of the hydrogenerator losses reduction
and improving its efficiency, hydrogenerators are designed in accordance with the
recommendations of the textbook “Design of Hydrogenerators and Synchronous
Compensators” by A. V. Ivanov-Smolensky, A. I. Abramov and ‘‘Electric Machines
Design ’’ by I. P. Kopilov, B. K. Klakov, V. P. Marozin.
In this thesis, will be calculate Yeywa data hydrogenerator design and
theoretical hydrogenerator design. The rated capacity 230 MVA.
The cost will not be considered in these design calculations.
2.1. Basic Dimensions
Active Power
(2.1)
Hijacking Speed
(2.2)
where, — multiplication factor of hijacking speed
for Pelton turbine
for Francis turbine
for Diagonal Kaplan turbine
for Axial Kaplan turbine
Rated Phase Voltage (Star connection)
(2.3)
Rated Phase Current
(2.4)
No. of Pole Pair

8
(2.5)
Leakage inductive reactance of stator winding (as a preliminary)
e.m.f at rated load
(2.6)
where, = coefficient
Rated Capacity
(2.7)
And then pole pitch , linear load and magnetic flux density
are previously selected by the value of .
Machine Constant
(2.8)
Moment of inertia of the rotor
(2.9)
where, = angular velocity of rotation
Minimum inner diameter of the stator core
(2.10)
where, = coefficient, depending on the rotor
construction and number of field period
Maximum inner diameter of the stator core
(2.11)
where, m/sec at m and .
m/sec at m and and alloy steel segment
rim.

9
m/sec at .
Optimum inner diameter of the stator core
(2.12)
where,
The inner diameter of the stator core must be . If
, take . In contrast, if , take . But
take , if .
Pole pitch at the selected inner diameter of the stator core
(2.13)
Hijacking circumferential velocity at the outer surface of the rotor must not
exceed the allowable values.
(2.14)
Length of the stator yoke
(2.15)
The relative length of the stator magnetic circuit
(2.16)
must be within the allowance value 1.5 to 4.0.
2.2. Winding Type and Number of Stator Slots
For machines with phase current A, especially at voltages
V should be applied double-layer bar type winding, having very reliable
coil insulation. Double-layer coil type lap windings are used in smaller phase currents.
If the number of slots per pole per phase q = 3 and a winding is usually performed
with integer number of slots per pole per phase; If a smaller q - with a fractional
number of slots per pole and phase.
Double-layer bar type wave winding (usually a fractional number of slots per
pole per phase) is mainly used in high-power multi-pole hydrogenerators (at ,
rpm), where they are more favorable to the lap windings by reducing the

10
number and length of connections. Lap winding with fractional or integer of slots per
pole per phase is applied in hydrogenerators (at and rpm) and
compensators.
Number of consecutive turns of phase (as a preliminary)
(2.17)
Effective conductors in the slot
for double-layer bar winding;
(even no:) for coil type lap winding;
The number of parallel branches
It must satisfy the inequality;
(2.18)
Stator slot current
(2.19)
The number of slots per pole per phase
(2.20)
If or is different from the nearest integer number of , take
. If , it must be selected fraction, i.e. .
The number of stator slots
(2.21)
The number of slots per segment
(2.22)
where, — the number of stator segments.
— integer number of segments laying in one layer of magnetic core.
The number of stator segments is dependent on the inner diameter of stator.
-----------
----------- 1 2, 4, 6 4, 6, 8
The outer diameter of the stator core (as a preliminary)

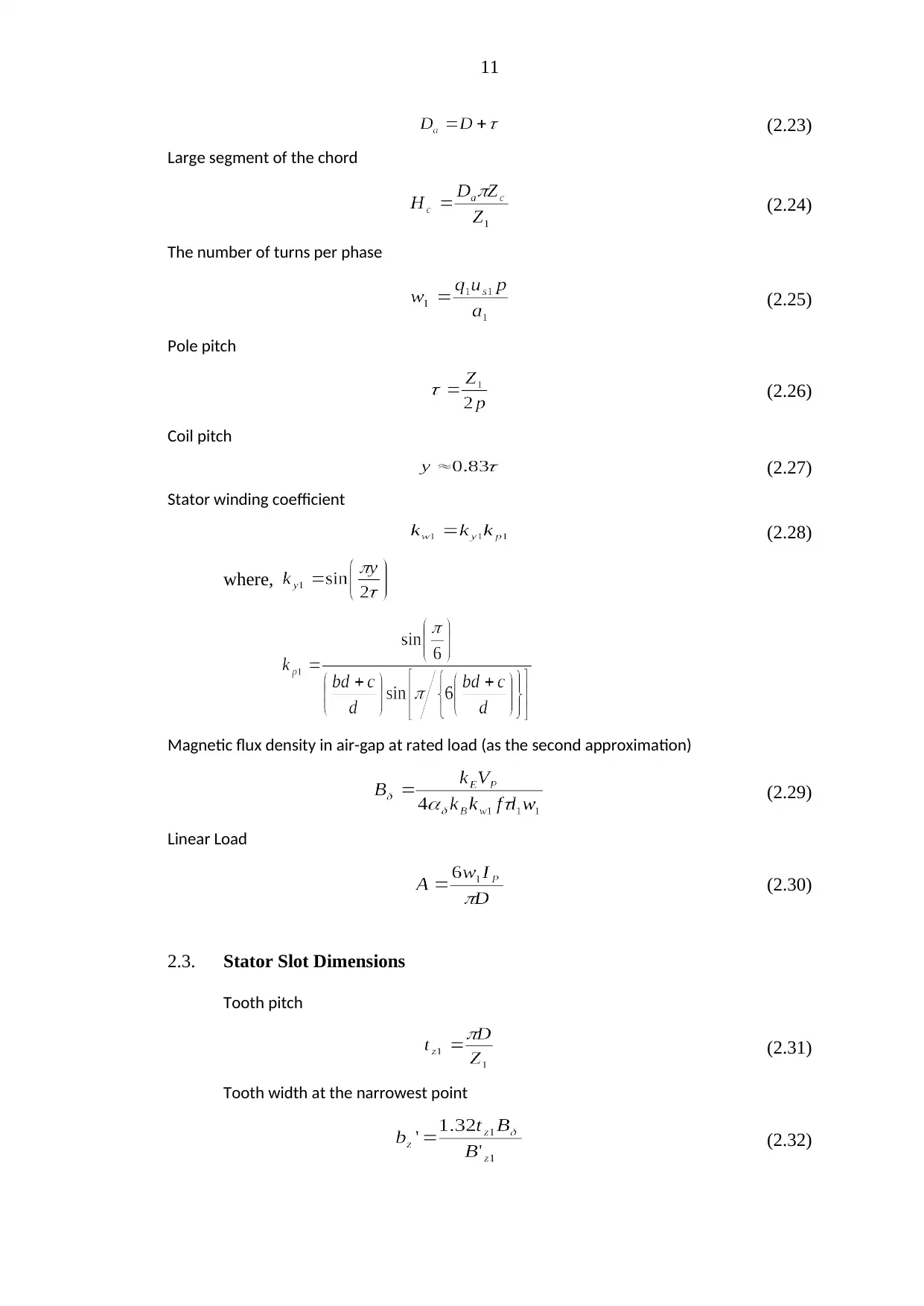
11
(2.23)
Large segment of the chord
(2.24)
The number of turns per phase
(2.25)
Pole pitch
(2.26)
Coil pitch
(2.27)
Stator winding coefficient
(2.28)
where,
Magnetic flux density in air-gap at rated load (as the second approximation)
(2.29)
Linear Load
(2.30)
2.3. Stator Slot Dimensions
Tooth pitch
(2.31)
Tooth width at the narrowest point
(2.32)

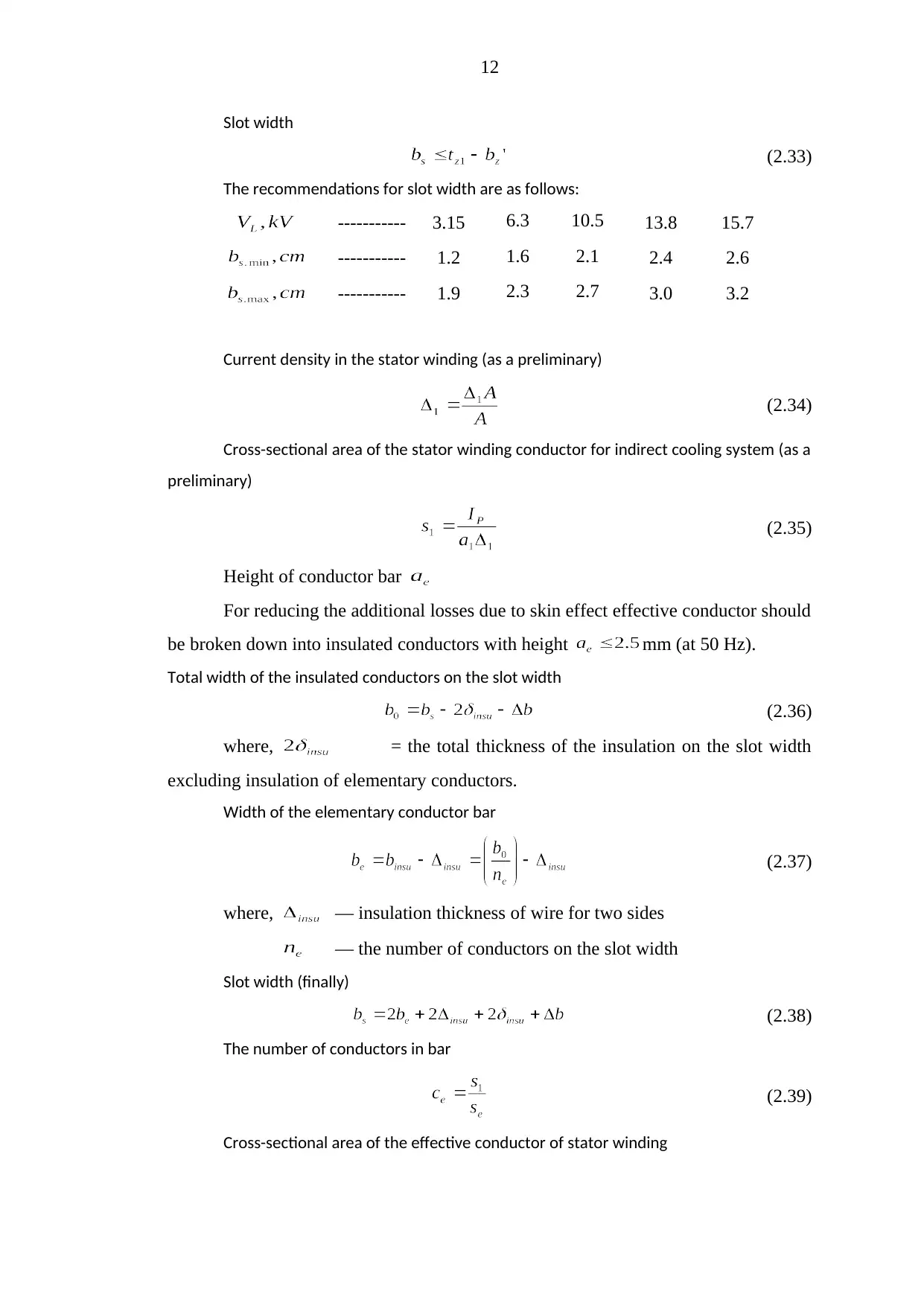
12
Slot width
(2.33)
The recommendations for slot width are as follows:
----------- 3.15 6.3 10.5 13.8 15.7
----------- 1.2 1.6 2.1 2.4 2.6
----------- 1.9 2.3 2.7 3.0 3.2
Current density in the stator winding (as a preliminary)
(2.34)
Cross-sectional area of the stator winding conductor for indirect cooling system (as a
preliminary)
(2.35)
Height of conductor bar
For reducing the additional losses due to skin effect effective conductor should
be broken down into insulated conductors with height mm (at 50 Hz).
Total width of the insulated conductors on the slot width
(2.36)
where, = the total thickness of the insulation on the slot width
excluding insulation of elementary conductors.
Width of the elementary conductor bar
(2.37)
where, — insulation thickness of wire for two sides
— the number of conductors on the slot width
Slot width (finally)
(2.38)
The number of conductors in bar
(2.39)
Cross-sectional area of the effective conductor of stator winding

13
(2.40)
Current density in the stator winding
(2.41)
Height of the elementary conductor bar with insulation
(2.42)
Width of the elementary conductor with insulation
(2.43)
Total height of insulated elementary conductors of one bar
(2.44)
Slot height or tooth height of stator
(2.45)
where, — wedge height
— insulation thickness according to slot height, taking into
account the swelling
— distance from the wedge to gap
At not only bar winding, but also coil winding slot height must be exist within
the allowance value .
Average temperature drop in slot insulation
(2.46)
where, — winding resistivity for copper winding at
— losses magnification factor due to skin effect in the winding
— thermal conductivity of insulation
2.4. Air-gap and Stator Magnetic Core Dimensions
Maximum magnetic flux density in air-gap at no-load and rated voltage
(2.47)
Leakage inductive reactance (in p.u)

14
(2.48)
Minimum air-gap between the stator and the rotor
(2.49)
where, — air-gap coefficient
— Reaction coefficient along the direct axis
Maximum air-gap under the pole edge
(2.50)
Number of ventilation channels in the core
(2.51)
where, m — stator core package length (pre)
m — channel width in the core
Stator core package length (Final)
(2.52)
Total length of the magnetic core packages
(2.53)
Magnetic flux at rated load
(2.54)
where,
Height of the stator magnetic core yoke
(2.55)
where, T — Magnetic flux density of stator for hot-rolled
and cold-rolled steel
— steel package filling coefficient: Usually varnished
electro technical steel sheets with 0.5 mm thickness are used.
Outer diameter of the stator core

15
(2.56)
Large cord of stator core segment
(2.57)
2.5. Rotor Magnetic Core and Damper Winding Dimensions
Pole pitch width
(2.58)
where, — overlapping pole coefficient
Arc radius of pole pitch
(2.59)
Pole core length
(2.60)
Calculated pole core length
(2.61)
Damper winding slot pitch
When the slots per pole per phase is integer, and
, slot pitch of the damper winding is selected smaller than stator tooth
pitch .
(2.62)
When , damper winding pitch should be taken as equal size in stator
tooth pitch.
(2.63)
Number of bars per pole
(2.64)
where, (as a preliminary)
Cross-sectional area of copper bar of damper winding
(2.65)

16
Cross-sectional area of the damper winding short-circuiting segment
(2.66)
where, and .
Dimensions of slot groove damper winding
m (2.67)
Damper winding bar length
(2.68)
Pole pitch height
For the lack of damper winding and , use the following equation.
And this equation is suited for the massive poles.
(2.69)
For the laminated pole pitch with damper winding use the equation shown
in below.
(2.70)
Pole core height
(2.71)
It should be within the limit m.
Pole core width
It is determined by solving a nonlinear equation with sequential
approximations method. The following inputs are pre-calculated.
Electromotive force of self-induction at rated load
(2.72)
where,
Magnetomotive force of excitation at rated load
(2.73)
where,
Current at rated voltage and no-load condition

17
(2.74)
Estimated magnetic flux density at pole
(2.75)
Coefficient
(2.76)
The value of
(2.77)
The value of
(2.78)
where,
The value of
For , take . But for , the following
equation is used.
(2.79)
Then the iterative process for determining the pole core width is made
by the algorithm. The initial value at the first iteration (i=1) is calculated by
the following formula.
(2.80)
When it is carried out the first iteration (i=1), determining:
(a) magnetic conductivity for the leakage flux of the poles
(2.81)
where,

18
(b) Leakage factor of the poles
(2.82)
(c) Magnetic flux in the pole base
(2.83)
(d) Final value of pole width after the first iteration (i=1)
(2.84)
(e) Relative accuracy of the for the i-th (1st iteration)
(2.85)
Assuming the specified relative accuracy , the
required relative accuracy must be less than or equal to i.e .
Therefore it is necessary to carry out (i+1)th – 2nd iteration and other iterations.
Outer diameter of the rotor rim
(2.86)
Rotor rim length (as a preliminary)
(2.87)
where, m
— width of the excitation winding conductor
Rotor rim height
(2.88)
Inner diameter of the rotor rim
(2.89)
2.6. Magnetic Circuit at No-load Condition
Air-gap coefficient
(2.90)

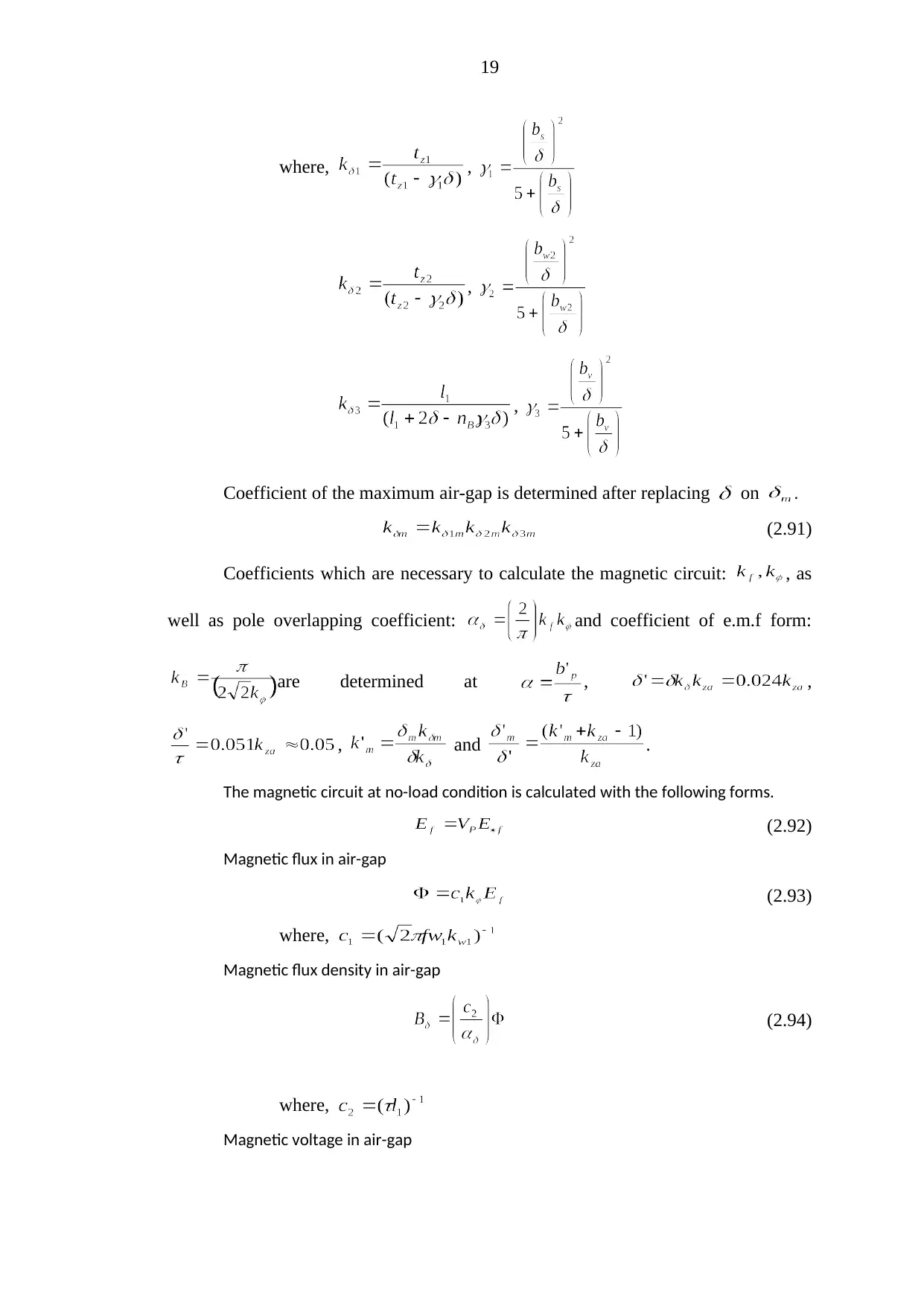
19
where, ,
,
,
Coefficient of the maximum air-gap is determined after replacing on .
(2.91)
Coefficients which are necessary to calculate the magnetic circuit: , as
well as pole overlapping coefficient: and coefficient of e.m.f form:
are determined at , ,
, and .
The magnetic circuit at no-load condition is calculated with the following forms.
(2.92)
Magnetic flux in air-gap
(2.93)
where,
Magnetic flux density in air-gap
(2.94)
where,
Magnetic voltage in air-gap

20
(2.95)
where,
Magnetic flux density in one-third height of tooth
(2.96)
where,
Magnetic voltage of stator tooth
(2.97)
Magnetic flux density in the stator yoke
(2.98)
where,
Magnetic voltage of the stator yoke
(2.99)
where, — the estimated length of the magnetic
lines in yoke
Magnetic flux density in the pole teeth
(2.100)
where, ;
Magnetic voltage of pole teeth
(2.101)
where, — height of the damper winding teeth
Magnetic voltage of the air-gap, the stator teeth and yoke, and the pole teeth
(2.102)

21
Saturation coefficient is . If , the
calculation must be repeated for the proximate , in which — selected
value in the previous iteration.
Leakage coefficient of the pole at no-load
(2.103)
where,
— magnetic conductance for the leakage flux
Magnetic flux in pole
(2.104)
Magnetic flux density in pole
(2.105)
where,
Magnetic voltage of the pole core
(2.106)
2.7. Inductive Reactance of the Windings
Shape factor of the field along the longitudinal axis
(2.107)
Shape factor of the field along the transverse axis
(2.108)
Inductive reactance of the mutual magnetic flux density of stator winding
along the longitudinal axis
(2.109)
Inductive reactance of the mutual magnetic flux density of stator winding
along the transverse axis
(2.110)
Leakage inductive reactance of the stator winding

22
(2.111)
Slot leakage conductivity coefficient
(2.112)
Frontal leakage conductivity coefficient
(2.113)
Damper leakage conductivity coefficient
(2.114)
Leakage inductive reactance of the excitation winding
(2.115)
where, — conductivity coefficient for the differential leakage
on one pole
— conductivity coefficient for the flux linkage of slot
leakage
— conductivity coefficient for the flux linkage of the
frontal parts
Leakage inductive reactance of the damper winding along the longitudinal axis
(in relative unit)
(a) Leakage inductive reactance of damper winding bar
(2.116)
where, — conductivity coefficient for the flux linkage of the
slot leakage and leakage along the tooth heads of the damper
winding (on the pole along the longitudinal axis)

23
— conductivity coefficient of the damper winding
differential leakage
(b) Leakage inductive reactance of the damper winding short-circuited
ring (frontal parts)
(2.117)
where, — conductivity coefficient for the flux linkage of short-
circuited ring
(c) Leakage inductive reactance of the damper winding
(2.118)
Leakage inductive reactance of the damper winding along the transversal axis
(in relative unit)
(a) Leakage inductive reactance of the damper winding bar
(2.119)
(b) Leakage inductive reactance of the damper winding short-circuited ring
(frontal parts)
(2.120)
(c) Leakage inductive reactance of the damper winding
(2.121)
Inductive reactance of zero-sequence
(2.122)
where, — conductivity coefficient for the flux linkage of the slot
leakage at zero-sequence currents

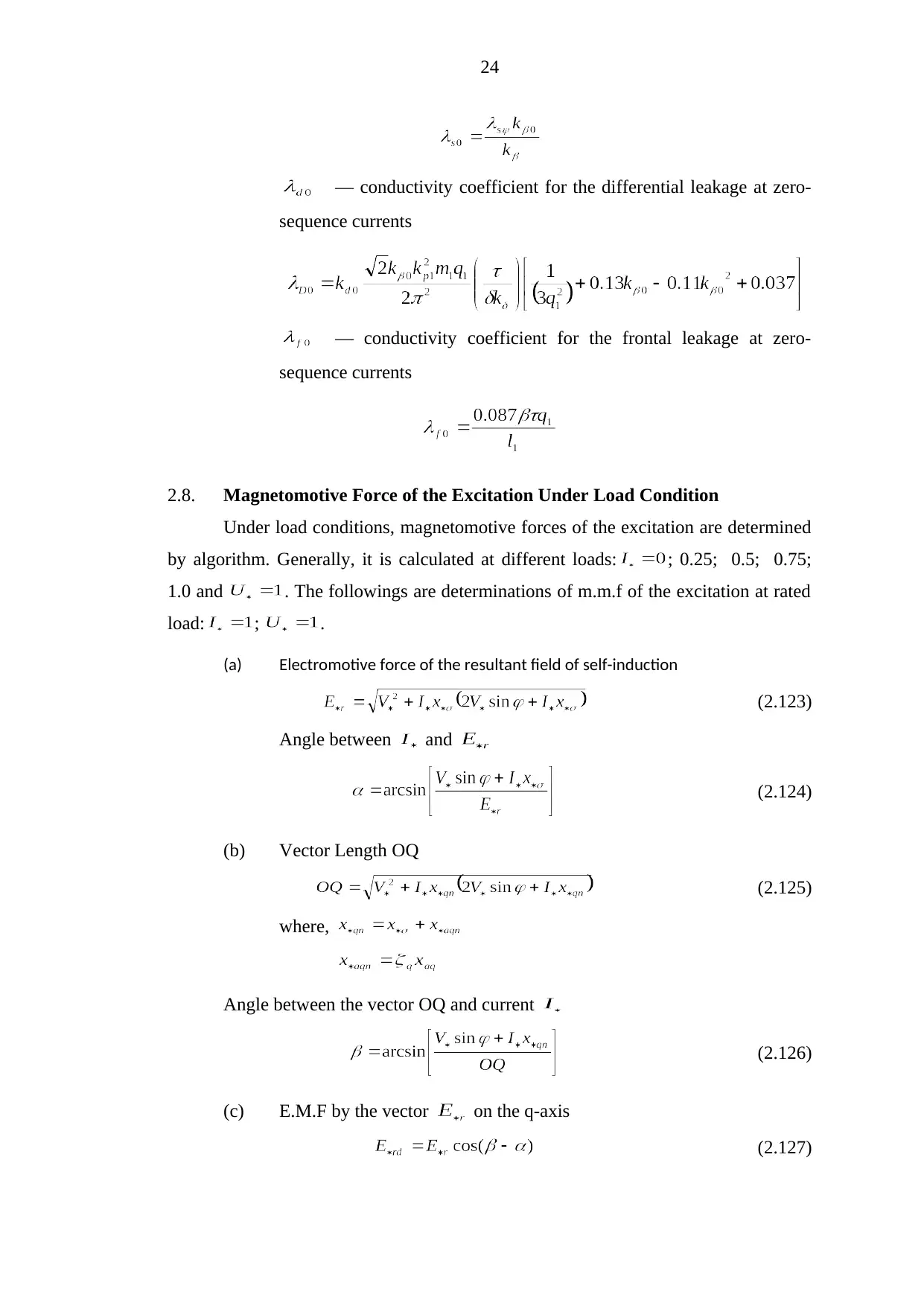
24
— conductivity coefficient for the differential leakage at zero-
sequence currents
— conductivity coefficient for the frontal leakage at zero-
sequence currents
2.8. Magnetomotive Force of the Excitation Under Load Condition
Under load conditions, magnetomotive forces of the excitation are determined
by algorithm. Generally, it is calculated at different loads: ; 0.25; 0.5; 0.75;
1.0 and . The followings are determinations of m.m.f of the excitation at rated
load: ; .
(a) Electromotive force of the resultant field of self-induction
(2.123)
Angle between and
(2.124)
(b) Vector Length OQ
(2.125)
where,
Angle between the vector OQ and current
(2.126)
(c) E.M.F by the vector on the q-axis
(2.127)

25
(d) According to the characteristic , m.m.f is
determined.
(e) According to the characteristic , the magnetic flux of mutual
induction is determined.
(f) m.m.f , equivalent with respect to the armature effect on the longitudinal
field
(2.128)
where,
(g) Magnetic voltage between the adjacent poles tips
(2.129)
(h) According to the characteristic , magnetic flux is
determined.
(i) Magnetic flux in the pole core
(2.130)
Magnetic voltage is determined by using the characteristic
.
(j) m.m.f of excitation at rated load
(2.131)
2.9. Dimensions and Basic Parameters of Excitation Winding
Average length of the half-turn excitation winding
(2.132)
The current density in the excitation winding
(2.133)
where, — heat transfer coefficient from the coil surface to cooling gas
(air cooling)

26
°С — exceeding temperature of the excitation winding
— coil height
— Resistivity of the copper winding at
exceeding temp: 75°С
Conductor width
(2.134)
The smallest distance between adjacent poles
(2.135)
where, — inner side of coil
Minimum permissible height of the wire
(2.136)
Maximum permissible height of the wire
(2.137)
The selected height of the wire must be between the minimum and maximum
permissible height of the wire: .
Cross-sectional area of the wire
(2.138)
Exceeding temperature at the selected sizes
(2.139)
Current density at the selected sizes
(2.140)
Rated excitation current (pre)
(2.141)
Number of turns in the excitation coil

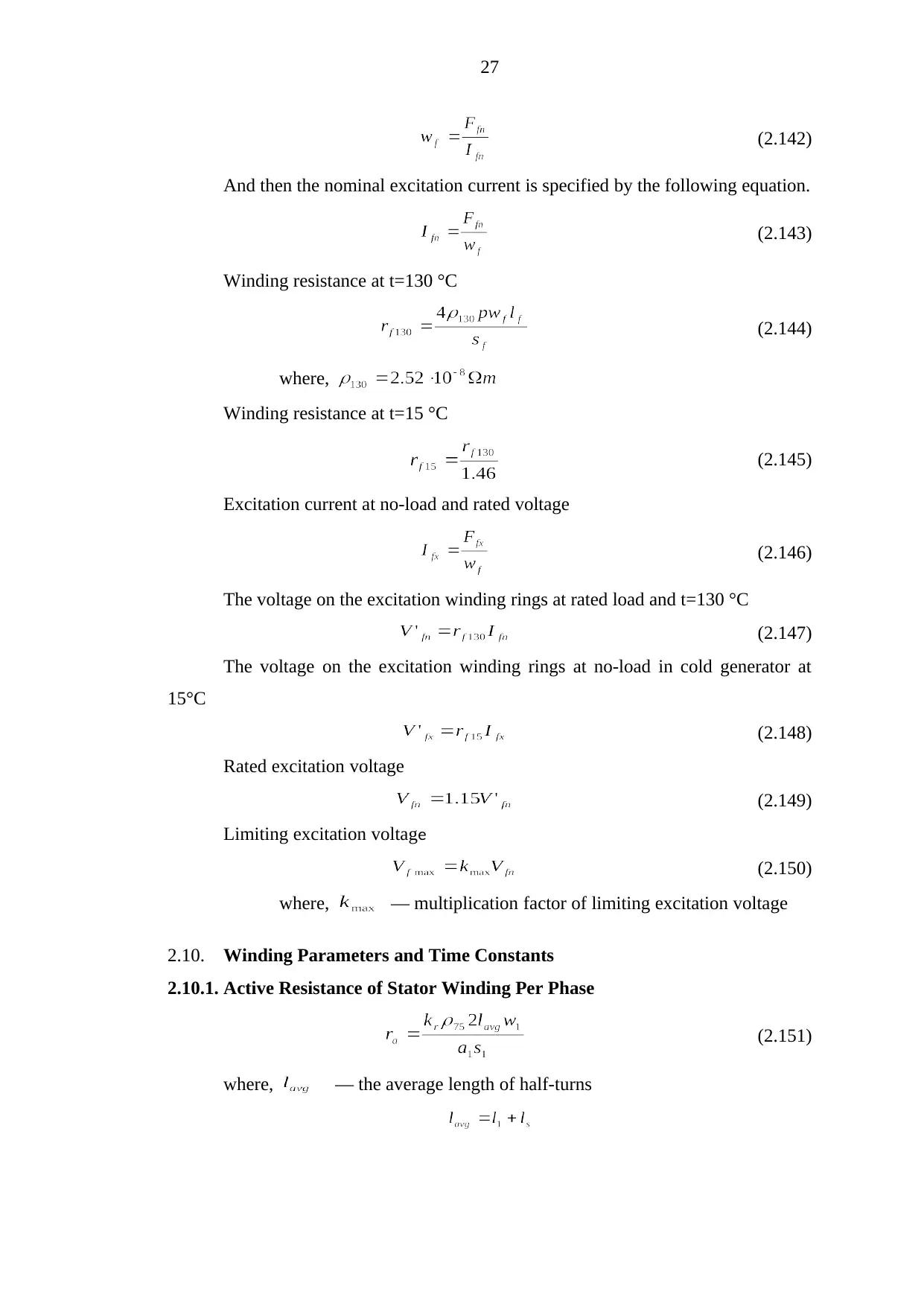
27
(2.142)
And then the nominal excitation current is specified by the following equation.
(2.143)
Winding resistance at t=130 °С
(2.144)
where,
Winding resistance at t=15 °С
(2.145)
Excitation current at no-load and rated voltage
(2.146)
The voltage on the excitation winding rings at rated load and t=130 °С
(2.147)
The voltage on the excitation winding rings at no-load in cold generator at
15°С
(2.148)
Rated excitation voltage
(2.149)
Limiting excitation voltage
(2.150)
where, — multiplication factor of limiting excitation voltage
2.10. Winding Parameters and Time Constants
2.10.1. Active Resistance of Stator Winding Per Phase
(2.151)
where, — the average length of half-turns

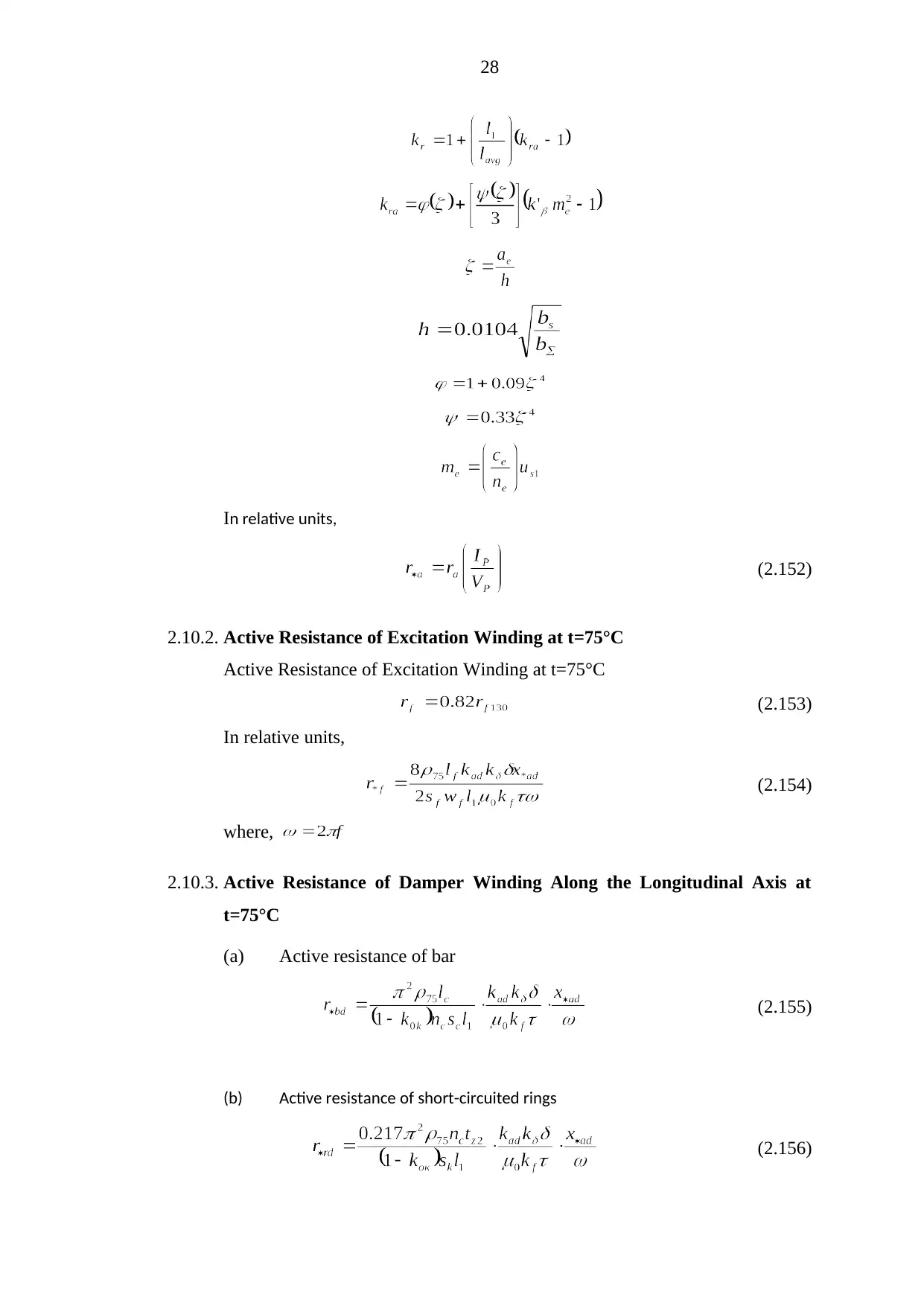
28
In relative units,
(2.152)
2.10.2. Active Resistance of Excitation Winding at t=75°С
Active Resistance of Excitation Winding at t=75°С
(2.153)
In relative units,
(2.154)
where,
2.10.3. Active Resistance of Damper Winding Along the Longitudinal Axis at
t=75°С
(a) Active resistance of bar
(2.155)
(b) Active resistance of short-circuited rings
(2.156)

29
(c) Active resistance damper winding
(2.157)
2.10.4. Active Resistance of Damper Winding Along the Transversal Axis at
t=75°С
(a) Active resistance of bar
(2.158)
(b) Active resistance of short-circuited rings
(2.159)
(c) Active resistance damper winding
(2.160)
2.10.5. Time Constants and Inductive Reactance of the Synchronous Machine
Winding at Other Open-circuited Windings
Inductive reactance of the armature winding along the longitudinal axis at the
open-circuited rotor windings,
(2.161)
Inductive reactance of the armature winding along the transversal axis at the
open-circuited rotor windings,
(2.162)
Inductive reactance and time constant of excitation winding at the other open-
circuited windings,
(2.163)
(2.164)
Inductive reactance and time constant of damper winding along the
longitudinal axis at the other open-circuited windings,
(2.165)
(2.166)
Inductive reactance and time constant of damper winding along the transversal
axis at the other open-circuited windings,

30
(2.167)
(2.168)
2.10.6. Parameters and Time Constants, Characterizing the Transient Process
Subtransient inductive reactance of the armature along the longitudinal axis,
(2.169)
Subtransient inductive reactance of the armature along the transversal axis,
(2.170)
Time constant decay of the periodic components of the armature current,
(2.171)
2.10.7. Asymmetric Operation Parameters of the Synchronous Machine
Inductive reactance for the zero-sequence currents
Inductive reactance for the reverse-sequence currents,
(2.172)
2.10.8. Mechanical Time Constant (or) Inertia Constant of Rotating Parts
(2.173)
2.11. Masses of Active and Constructive Parts, Load on the Thrust Bearing and
the Bearing
Stator core teeth mass
(2.174)
where,
Mass of the stator core yoke
(2.175)
Mass of the stator winding wires

31
(2.176)
Mass of the excitation winding wires
(2.177)
Mass of the damper winding bars
(2.178)
Short-circuited rings mass of the damper winding
(2.179)
Steel poles mass
(2.180)
Rotor rim mass
(2.181)
Rotor mass (including shaft and other rotating parts masses)
(2.182)
Load on the thrust bearing of Kaplan Turbine
(2.183)
where, m/seс2 ;
2.12. Power Losses and Efficiency
2.12.1. No-load Losses
(a) Magnetic Losses in the stator core
(2.184)
where, , W/kg;
, W/kg;
— specific losses in steel;
— empirical coefficient;
— empirical coefficient;
(b) Additional Losses on the Surfaces of Pole Pitch
(2.185)

32
where, ;
(c) Steel Losses
(2.186)
2.12.2. Short-circuited Losses
(a) Electrical Losses in the Stator Winding at rated current and
(2.187)
where, — winding resistance increasing coefficient due to the
skin effect;
(b) Additional Losses in the Stator Winding
(2.188)
(c) Additional Losses in the Stator Teeth due to the Third Harmonic
Components of Field
(2.189)
where, — magnetic flux density in the stator teeth from the t
hird harmonic component of the field, T:
(d) Additional Losses on the Pole Pitch Surface due to the Stator
Stair-case Effect
(2.190)
where, — losses on the surface of the poles at no-load condition;
(e) Additional Losses on the Pole Pitch Surface from Higher
Harmonic Components of Stator M.M.F
(2.191)
where, — coefficient in the function;
— relative winding pitch.
(f) Total additional losses at short-circuited condition
(2.192)

33
(g) Total losses at short-circuited condition and rated stator current
(2.193)
2.12.3. Losses in the Excitation
With direct electrical machine system of the excitation (build-in excitation)
losses are determined by the losses calculation in the excitation system at .
(2.194)
where, — efficiency of the excitation system
2.12.4. Mechanical Losses
(a) Ventilation Losses due to Circulation Gas in the Cooling System
(2.195)
where, — theoretical pressure, developed by the
rotating;
— the peripheral linear speed on the rotor outer
surface, ;
— coefficient, taking into account the losses on
the friction of the rotating parts due to cooling gas;
— gas density, ;
Gas consumption , involved in the heat transfer is determined by the
following equation;
(2.196)
where, — losses in the pole piece
— losses in the winding
— losses in the stator core
— volumetric heat capacity of the gas,
— heating gas
(b) Friction Losses in the Bearing (Thrust Bearings and Guide Bearings) of
Vertical Type Hydrogenerator
(2.197)

34
where, — load on the thrust bearing, N
— coefficient, depending on the average specific
pressure in the thrust bearing and hydrogenerator construction
where, — for suspension type
— for umbrella type
(c) Total Mechanical Losses of Vertical Type Hydrogenerator
(2.198)
2.12.5. Power Losses and Efficiency at Rated Load
(2.199)
(2.200)
2.13. Ventilation and Thermal State Calculation
2.13.1. Ventilation Calculation
Static rotor pressure
(2.201)
where, — the circumferential speed of the outer
rotor diameter
— the circumferential speed of the inner
rotor diameter
— the radial dimension of the channels
in the rim and the poles
g0=1.22 — air density, kg/m3
Cross-sectional area of the air-gap
(2.202)
Cross-sectional area of the stator ventilation channels
(2.203)
Cross-sectional area in the inter-poles space with the end sides
(2.204)

35
where, — average distance between the coils
and pole tips of the adjacent poles
— distance between the bases of adjacent coils
Cross-sectional area in the air-gap with the end sides
(2.205)
Cross-sectional area in the inter-poles space through the circular channels in
the rotor rim
(2.206)
Cross-sectional area in the inter-poles apace through the rectangular channels
between adjacent segments of the rotor rim
(2.207)
where,
Total cross-sectional area in the inter-pole space
(2.208)
Total cross-sectional area at the water coolers
(2.209)
where,
Q = 49.941 m3/seс
Hydraulic resistances of pipeline sections
(2.210)
(2.211)
(2.212)
Hydraulic resistance of the air duct