Statistical Problems Using Excel
VerifiedAdded on 2023/06/11
|4
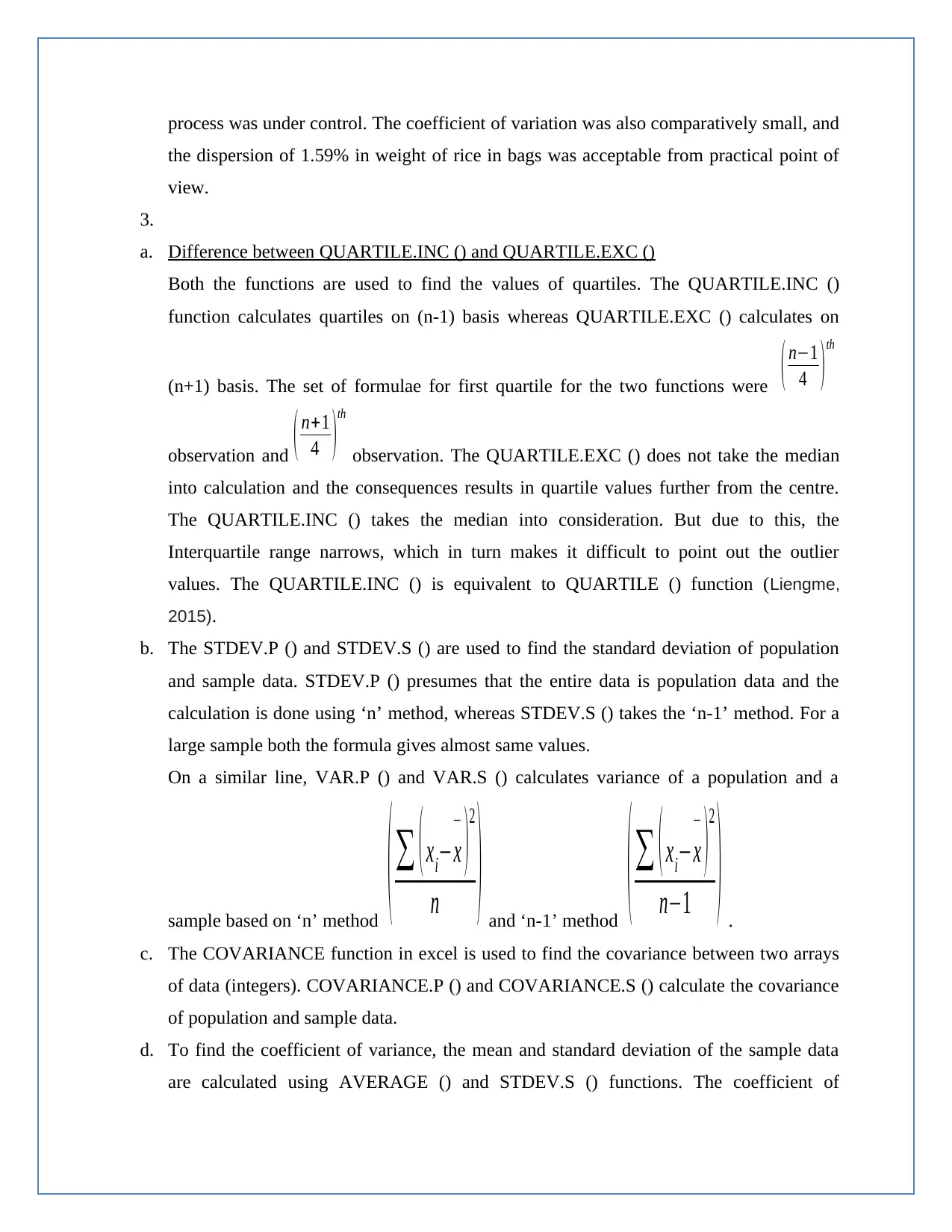
|836
|212
AI Summary
This article discusses how to solve statistical problems using Excel with solved examples and explanations. It covers topics like quartiles, standard deviation, coefficient of variation, and more. The article also explains the difference between QUARTILE.INC() and QUARTILE.EXC() functions, and STDEV.P() and STDEV.S() functions. The COVARIANCE function and coefficient of variance are also discussed. References are provided for further reading.
Contribute Materials
Your contribution can guide someone’s learning journey. Share your
documents today.
1 out of 4







![[object Object]](/_next/static/media/star-bottom.7253800d.svg)