First Year Physics Laboratory Preliminary Exercises Solution 2022
VerifiedAdded on 2022/10/04
|5
|946
|12
AI Summary
Contribute Materials
Your contribution can guide someone’s learning journey. Share your
documents today.
Page 1
FIRST YEAR PHYSICS LABORATORY PRELIMINARY EXERCISES
EXPERIMENT E2: ROTATION AND ROLLING
Complete the following questions on this sheet, either typed or handwritten, and hand in a hard
copy to a demonstrator at the beginning of your lab session. Show your reasoning and working.
Student’s Name : Number
Group Table Date of exp Mark /15
FIRST YEAR PHYSICS LABORATORY PRELIMINARY EXERCISES
EXPERIMENT E2: ROTATION AND ROLLING
Complete the following questions on this sheet, either typed or handwritten, and hand in a hard
copy to a demonstrator at the beginning of your lab session. Show your reasoning and working.
Student’s Name : Number
Group Table Date of exp Mark /15
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

Page 2
In these exercises use g = 9.8 m/s2
1. A wheel accelerates uniformly from rest to a rotational speed of 10 rad/s in 5 s. What
is its angular acceleration?
The angular acceleration of a rotating object is the change of its angular velocity with respect
with the time taken [2]. It is calculated by dividing the angular velocity with the change in time.
Angular acceleration = (change in angular velocity)/ (change in time)
= (final angular velocity- initial angular velocity)/ (final time – initial time)
Where:
α = angular acceleration, (radians/s2)
Δω = change in angular velocity (radians/s)
Δt = change in time (s)
ω1 = initial angular velocity (radians/s)
ω2= final angular velocity (radians/s)
t1 = initial time (s)
t2= final time (s)
from the question,
t1=0, t2=5s, initial angular velocity ω1 = 0 and ω2= 10 radians/sec
therefore, it will be; (10 - 0) / (5 - 0) = 10 ÷ 5 = 2
the angular acceleration α of the wheel is 2 rads / s2
2. A cylinder of diameter 100 mm rolls from rest down a 5 m long ramp and its Centre of
mass is moving with velocity 2 m/s at the bottom of the ramp.
2.1. What is its (constant) acceleration down the ramp?
For this exercise, we apply the kinematic equation. The kinematic equation gives a relationship
between velocities, distance and acceleration of a moving body.
Kinematic equation formula is vf² = vi²
+ 2a d
vi² = initial velocity
a = acceleration
d = displacement
the initial velocity = 0
final velocity Vf2 = 2 x 2 = 4
thus the equation will be vf² = 2a d
working out for a, 4 ÷ 2 x 5= 0.4 m/s2
2.2. What is its angular acceleration?
If it rolls without slipping, the angular acceleration is:
α = a / r
r = ½ (100mm) = 0.05 meters
0.4 / 0.05 = 8 rad/s2
In these exercises use g = 9.8 m/s2
1. A wheel accelerates uniformly from rest to a rotational speed of 10 rad/s in 5 s. What
is its angular acceleration?
The angular acceleration of a rotating object is the change of its angular velocity with respect
with the time taken [2]. It is calculated by dividing the angular velocity with the change in time.
Angular acceleration = (change in angular velocity)/ (change in time)
= (final angular velocity- initial angular velocity)/ (final time – initial time)
Where:
α = angular acceleration, (radians/s2)
Δω = change in angular velocity (radians/s)
Δt = change in time (s)
ω1 = initial angular velocity (radians/s)
ω2= final angular velocity (radians/s)
t1 = initial time (s)
t2= final time (s)
from the question,
t1=0, t2=5s, initial angular velocity ω1 = 0 and ω2= 10 radians/sec
therefore, it will be; (10 - 0) / (5 - 0) = 10 ÷ 5 = 2
the angular acceleration α of the wheel is 2 rads / s2
2. A cylinder of diameter 100 mm rolls from rest down a 5 m long ramp and its Centre of
mass is moving with velocity 2 m/s at the bottom of the ramp.
2.1. What is its (constant) acceleration down the ramp?
For this exercise, we apply the kinematic equation. The kinematic equation gives a relationship
between velocities, distance and acceleration of a moving body.
Kinematic equation formula is vf² = vi²
+ 2a d
vi² = initial velocity
a = acceleration
d = displacement
the initial velocity = 0
final velocity Vf2 = 2 x 2 = 4
thus the equation will be vf² = 2a d
working out for a, 4 ÷ 2 x 5= 0.4 m/s2
2.2. What is its angular acceleration?
If it rolls without slipping, the angular acceleration is:
α = a / r
r = ½ (100mm) = 0.05 meters
0.4 / 0.05 = 8 rad/s2
Page 3
3. For a solid cylinder, calculate the acceleration as it rolls down a ramp with a slope of
5° above the horizontal
For a rolling body down a ramp,
m a = m g sin (angle)
- I a / R2
(m + I / R2) a = m g sin(angle)
a = m g sin (angle) / (m + I / R2)
m = mass of the solid cylinder, g = acceleration due to gravity, I = moment of inertia and
R = radius
The moment of inertia of a solid cylinder about its central axes is 1/2 m R2
a = m g sin (angle) / (m + 1/2 m) = 2/3 x g sin (angle)
With angle = 50 and g = 9.8 m/s2
a = 2/3 x 9.8 m/s2 x sin (50) =
acceleration = 0.43 m/s2
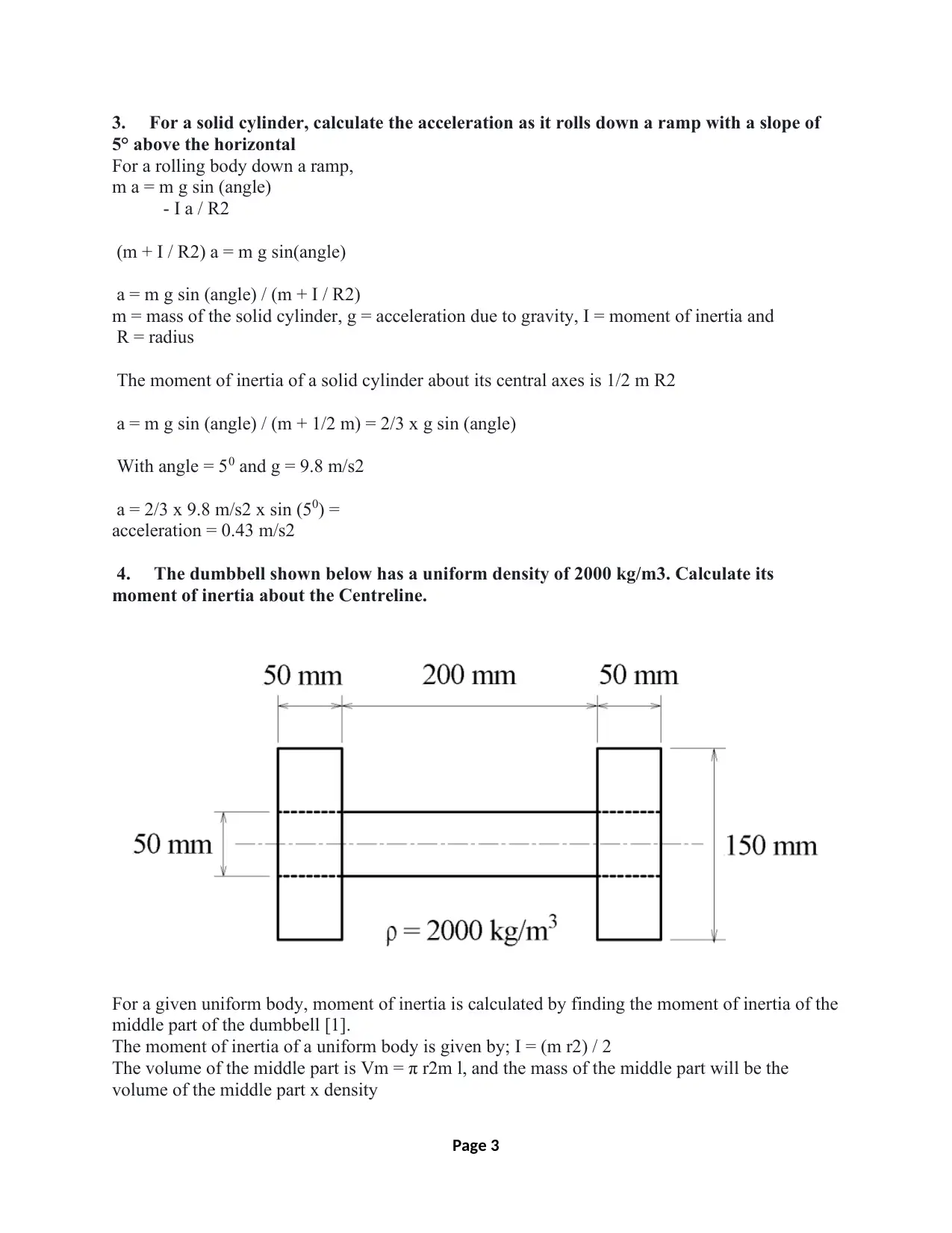
4. The dumbbell shown below has a uniform density of 2000 kg/m3. Calculate its
moment of inertia about the Centreline.
For a given uniform body, moment of inertia is calculated by finding the moment of inertia of the
middle part of the dumbbell [1].
The moment of inertia of a uniform body is given by; I = (m r2) / 2
The volume of the middle part is Vm = π r2m l, and the mass of the middle part will be the
volume of the middle part x density
3. For a solid cylinder, calculate the acceleration as it rolls down a ramp with a slope of
5° above the horizontal
For a rolling body down a ramp,
m a = m g sin (angle)
- I a / R2
(m + I / R2) a = m g sin(angle)
a = m g sin (angle) / (m + I / R2)
m = mass of the solid cylinder, g = acceleration due to gravity, I = moment of inertia and
R = radius
The moment of inertia of a solid cylinder about its central axes is 1/2 m R2
a = m g sin (angle) / (m + 1/2 m) = 2/3 x g sin (angle)
With angle = 50 and g = 9.8 m/s2
a = 2/3 x 9.8 m/s2 x sin (50) =
acceleration = 0.43 m/s2
4. The dumbbell shown below has a uniform density of 2000 kg/m3. Calculate its
moment of inertia about the Centreline.
For a given uniform body, moment of inertia is calculated by finding the moment of inertia of the
middle part of the dumbbell [1].
The moment of inertia of a uniform body is given by; I = (m r2) / 2
The volume of the middle part is Vm = π r2m l, and the mass of the middle part will be the
volume of the middle part x density

Page 4
Thus the moment of inertia of the middle part = ½π r4m l ρ
We also need to calculate the volume of the side part. Thus M= Is= π r2s d
The mass will be; πr2s d ρ while the MI will be ½ πr4d ρ
The total MI of the dumbbell is the moment of the middle part plus the moment of the side part;
Thus; ½π r4m l ρ + ½ π r4s d ρ where;
Ρ = 2000 kg/m3
Rm = 0.025 m
L = 0.2 m
Rs = 0.075 m
d = 0.05 m
replacing the values into the equation will be;
(½π x 0.0254 x 0.2 x 2000) + (½π x 0.0754 x 0.05 x 2000)
I = 0.0102 Kg/m2
Thus the moment of inertia of the middle part = ½π r4m l ρ
We also need to calculate the volume of the side part. Thus M= Is= π r2s d
The mass will be; πr2s d ρ while the MI will be ½ πr4d ρ
The total MI of the dumbbell is the moment of the middle part plus the moment of the side part;
Thus; ½π r4m l ρ + ½ π r4s d ρ where;
Ρ = 2000 kg/m3
Rm = 0.025 m
L = 0.2 m
Rs = 0.075 m
d = 0.05 m
replacing the values into the equation will be;
(½π x 0.0254 x 0.2 x 2000) + (½π x 0.0754 x 0.05 x 2000)
I = 0.0102 Kg/m2
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

Page 5
References
[1]X. Song, M. Lu and T. Qin, "The Graphics Section Moment of Inertia of an Area Rotation
Axis Calculation Diagram Method——Moment of Inertia of an Area Circle", Advanced
Materials Research, vol. 479-481, pp. 2086-2089, 2012. Available:
10.4028/www.scientific.net/amr.479-481.2086.
[2]"Calculation of angular velocity, angular acceleration and torque of two common point rigid
bodies using IMU", Journal of Applied and Physical Sciences, vol. 3, no. 2, 2017. Available:
10.20474/japs-3.2.3.
References
[1]X. Song, M. Lu and T. Qin, "The Graphics Section Moment of Inertia of an Area Rotation
Axis Calculation Diagram Method——Moment of Inertia of an Area Circle", Advanced
Materials Research, vol. 479-481, pp. 2086-2089, 2012. Available:
10.4028/www.scientific.net/amr.479-481.2086.
[2]"Calculation of angular velocity, angular acceleration and torque of two common point rigid
bodies using IMU", Journal of Applied and Physical Sciences, vol. 3, no. 2, 2017. Available:
10.20474/japs-3.2.3.
1 out of 5
Related Documents
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.





