Fluid Mechanics/ Fluid Flow 13

This assignment involves calculating various parameters of fluid mechanics/flow. This includes resultant force and overturning moment of a lock gate, upthrust on a body immersed in a liquid, various design characteristics of a tapering pipe, and identifying various methods used to determine density of solid objects and liquids.

Fluid Mechanics/ Fluid Flow 1
FLUID MECHANICS/ FLUID FLOW
Name
Course
Professor
University
City/state
Date

Fluid Mechanics/ Fluid Flow 2
Fluid Mechanics/ Fluid Flow
Introduction
The assignment involves calculating various parameters of fluid mechanics/flow. This includes
resultant force and overturning moment of a lock gate, upthrust on a body immersed in a liquid,
various design characteristics of a tapering pipe, and identifying various methods used to
determine density of solid objects and liquids.
Task 1
a) Resultant force on each gate
Looking at the geometry of the locks, the value of inclination of the lock gates in respect with the
walls, α, is determined as follows
α = 180 °−140 °
2 = 40°
2 =20 °
Based also on the geometry of the lock gates, the width of each gate can be determined using
trigonometric ratios as follows
cos α= 8 m
width of gate
Hence Width of each gate= 8 m
cos α = 8 m
cos 20 ° = 8 m
0.9397 =8.51m
Therefore wetted area of each gate, A = length/depth of water on the upstream x width of the
gate
A = 6 m x 8.51 m = 51.06 m2

Fluid Mechanics/ Fluid Flow 3
Force acting on each gate = H
2 x g x A; Where H = depth of water on the upstream, g =
gravitational acceleration and A = wetted area of each gate
= 6
2 x 9.81 x 51.06=1,502.7 KN
b) Resultant moment
The resultant moment that tends to overturn each gate at the base is calculated using the
following formula:
Resultant moment = resultant force x height at which the resultant force acts from the base (Hall,
2015)
Thus resultant moment = 1,502.7 KN x 6 m
3 =3,005.4 KNm
c) Size of the boat
The pressure on the lock gate is different depending on the size of the boat inside the lock mainly
because the amount of water displaced by the boat depends on the size of the boat. Bigger boats
displaces more water than smaller boats. As a result, the final height of the water acting on the
lock gate varies depending on the size of the boat. This height determines the height at which the
resultant force acts on the on the lock gate from the base. In other words, the amount of pressure
exerted on the lock gate by a bigger boat is relatively higher than that of a smaller boat because
the former displaces more water thus producing a greater height at which the water is acting on
the lock gate from its base.
Task 2

Fluid Mechanics/ Fluid Flow 4
Question 1
a) Calculating volume of the body
Weight of body in air = 18 N
Weight of body when completely immersed in water = 13.7 N
Density of water = 1,000 kg/m3
Gravitational acceleration = 9.81m/s2
The apparent loss of weight of a body immersed in water can be calculated using the following
formula
Apparent loss of the body’s weight = ρw x g x V (where ρw = density of water, g = gravitational
acceleration and V = volume of the body).
Therefore
(18N – 13.7N) = 1,000 kg/m3 x 9.81 m/s2 x V
4.3N = 9.81 x 1000 x V
V = 4.3
9.81 x 1000 =4.383 x 10− 4 m3
Thus volume of the body = 4.383 x 10-4 m3
b) Calculating density and relative density of the body
Density of the body, ρ, can be calculated using the following formula

Fluid Mechanics/ Fluid Flow 5
ρ= mass of thebody
volume of the body
Mass of body = 18N = 18N x 1
9.81 N /kg =1.835 kg
Therefore ρ= 1.835 kg
4.383 x 10−4 m3 =4,186.6 kg/m3
Relative density of the body, ρr, is calculated using the following formula
ρr= Density of the body
Density of water = 4,186,6 kg /m3
1,000 kg/m3 =4.1866
Question 2
a) Calculating submerged depth of the box
Apparent weight of floating box = 0 (this is because the weight of the box is equal to the weight
of displaced water).
Weight of the box = weight of displaced water = ρw x g x V (where ρw = density of water, g =
gravitational acceleration and V = volume of the body).
Therefore
223N = 1,030 kg/m3 x 9.81 m/s2 x V
V = 223 N
1030 kg /m3 x 9.81 m/s2 = 223 N
10,104.3 kg /m2 s2 =0.02207 m3
The above is the volume of submerged part of the box. This volume can also be calculated as
follows:

Fluid Mechanics/ Fluid Flow 6
Volume = l x w x d (where l = length of the box, w = width of the box, and d = submerged depth
of the box).
Therefore
0.02207m3 = 0.56m x 0.42m x d
d= 0.02207 m3
0.56 m x 0.42 m =0.02207 m3
0.2352m2 =0.09384 m=93.84 mm
b) Calculating force in the chain
The total volume of the box is equal to the volume of water displaced. The force in the chain is
the difference between the apparent loss of weight of the box (buoyancy or upthrust of the water)
and the weight of the box.
Total volume of the box = volume of water displaced = l x w x d
= 0.56m x 0.42m x 0.21m = 0.049392m3
Weight of water displaced = ρw x g x V
= 1,030kg/m3 x 9.81m/s2 x 0.049392m3
= 499.07 N
Therefore force in the chain = weight of water displaced – weight of the box (Bird, 2013)
= 499.01N – 223N
= 276.07N
Task 3

Fluid Mechanics/ Fluid Flow 7
a) Calculating the velocity
Discharge = 8 tonnes/hr. = 8,000 kg/hr.
Diameter of pipe, d = 50mm = 0.05m
Diameter of storage tank, D = 2.3m
Mass flow rate through the pipe, m = velocity of the oil through the pipe (V) x cross-sectional
area of the pipe (A) x density of oil through the pipe (ρ) (Hall, 2015).
m = V x A x ρ
Making V the subject gives: V = m
A x ρ
m = 8 tonnes/hr. = 8,000 kg/hr.
A= π d2
4 = π x ( 0.05 m )2
4 =0.0019635 m2
Density of oil, ρ = density of water x relative density = 1,000 kg/m3 x 0.9 = 900kg/m3
Therefore
V = 8,000 kg /hr .
0.0019635 m2 x 900 kg /m3 =4,527.06 m/hr =1.26 m/s
b) Calculating rate per hour
Equation of capacity is given as follows:
Q= m
ρ = 8,000 kg/hr .
900 kg /m3 =8.889 m3 /hr .
The rate at which the level of oil is increasing in the tank is given as

Fluid Mechanics/ Fluid Flow 8
Rate= Q
A ; Where Q = capacity, and A = cross sectional area of the storage tank.
A= π d2
4 = π x ( 2.3 m )2
4 =4.155 m2
Rate= 8.889 m3 /hr
4.155 m2 =2.14 m/hr
c) Calculating time taken to fill 5m depth
The time taken to fill a certain depth of the storage tank is determined using the rate equation
below
t= h
Rate ; Where t =time taken to fill a certain depth of the tank, h = depth of the tank, and R =
rate at which the level of the tank is rising.
Therefore
t= 5 m
2.14 m/hr =2.34 hr
Task 4
a) Experiment to determine density of a solid object
Density of a solid object can be determined by dividing the mass of the object by its volume. In
this experiment, the mass of the object is measured using an electronic balance. The mass is
measured directly by lacing the solid object on the balance and reading the value displayed on
the balance and recording it as M grams (g). On the other hand, the volume of the object is
measured by displacement method. The basic principle of this method is that the volume of an

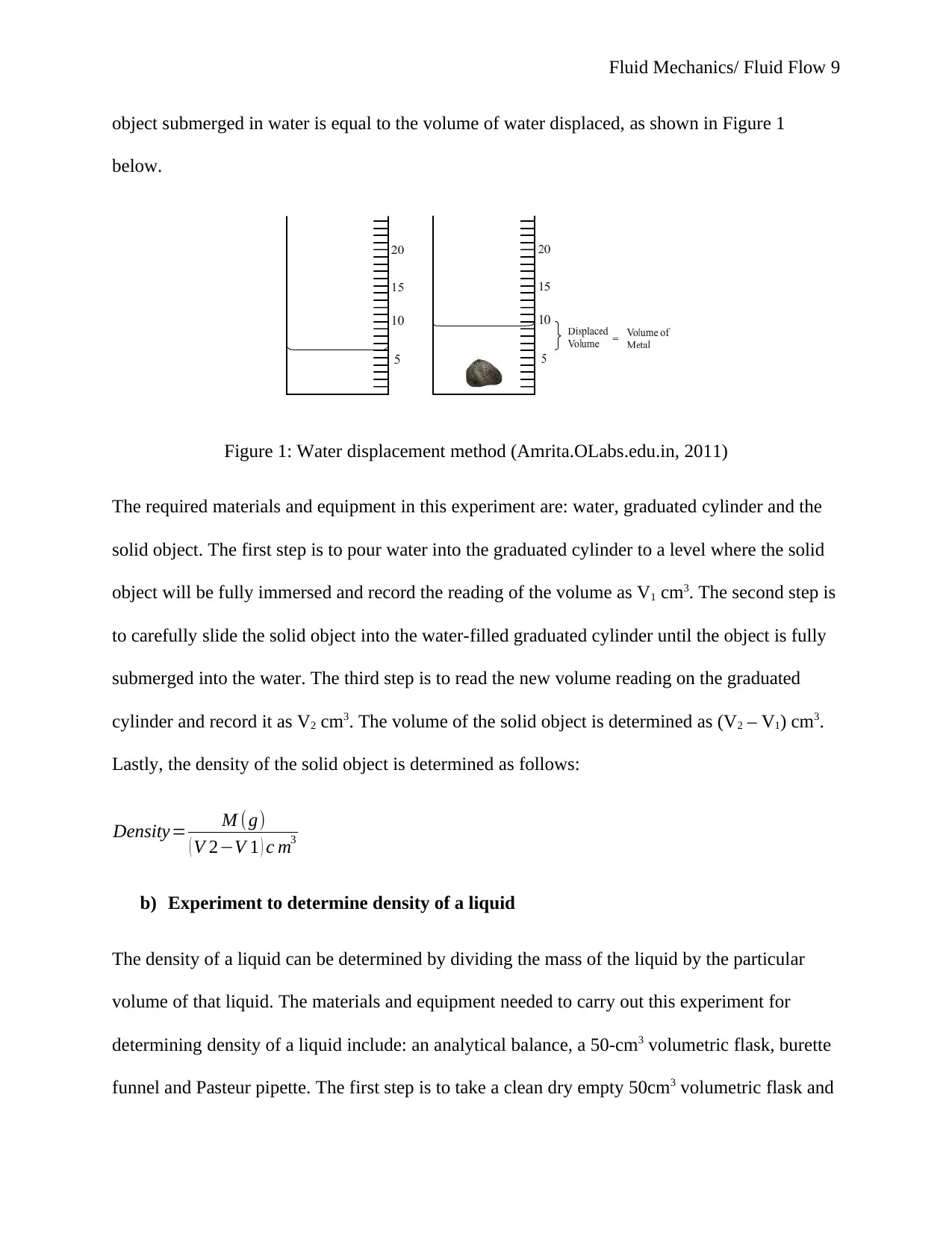
Fluid Mechanics/ Fluid Flow 9
object submerged in water is equal to the volume of water displaced, as shown in Figure 1
below.
Figure 1: Water displacement method (Amrita.OLabs.edu.in, 2011)
The required materials and equipment in this experiment are: water, graduated cylinder and the
solid object. The first step is to pour water into the graduated cylinder to a level where the solid
object will be fully immersed and record the reading of the volume as V1 cm3. The second step is
to carefully slide the solid object into the water-filled graduated cylinder until the object is fully
submerged into the water. The third step is to read the new volume reading on the graduated
cylinder and record it as V2 cm3. The volume of the solid object is determined as (V2 – V1) cm3.
Lastly, the density of the solid object is determined as follows:
Density= M (g)
( V 2−V 1 ) c m3
b) Experiment to determine density of a liquid
The density of a liquid can be determined by dividing the mass of the liquid by the particular
volume of that liquid. The materials and equipment needed to carry out this experiment for
determining density of a liquid include: an analytical balance, a 50-cm3 volumetric flask, burette
funnel and Pasteur pipette. The first step is to take a clean dry empty 50cm3 volumetric flask and

Fluid Mechanics/ Fluid Flow 10
place it on the analytical balance. The second step is to zero or tare the balance to ensure that it
reads 0.000g. The third step is to use a burette funnel and transfer 45cm3 of the subject liquid
into the empty volumetric flask. The fourth step is to use a Pasteur pipette and add 5cm3 of the
liquid to the flask until the bottom part of the meniscus of the liquid touches the flask’s 50cm3
marking. The fifth step is to read the weight of the volumetric flask filled with the liquid as
displayed on the balance and record the mass as M grams (g). The density of the liquid can then
be determined as follows:
Density= M g
50 c m3
Methods for measuring density of water
i) Measure mass and volume
First is to place a dry, empty graduated cylinder on a zeroed electronic balance and record its
mass as M1 g. Second is to fill the graduated cylinder with any amount of water and record the
volume of the added water as V cm3. Third is to weigh the water-filled graduated cylinder on a
zeroed electronic balance and record its mass as M2 g. Fourth is to subtract M1 g from M2 g (M2 –
M1)g to get ∆Mg. Fifth is to determine the density of water by dividing ∆Mg by V cm3.
ii) Hydrometer
In this method, a hydrometer is immersed in water (as shown in Figure 2 below) and the density
of the water is read directly from the hydrometer’s scale.

Fluid Mechanics/ Fluid Flow 11
Figure 2: Hydrometer (Paar, 2019)
Advantages of this method include: the hydrometer is small in size and easy to carry; it is less
expensive; and it takes less time. Disadvantages include: it is less accurate; the accuracy of the
hydrometer is affected by temperature changes; and it required large volume of water sample.
iii) Digital density meter
This method involves filling water sample into the digital density meter, which gets excited and
beginnings oscillating at a particular frequency. This frequency calculates the density of the
water sample. Figure 3 below is an example of a digital density meter.
Figure 3: Digital density meter (Paar, 2019)
Advantages of this method include: it is easy to operate; requires small amount of water sample;
it is not affected by temperature or pressure; and it has high precision. Disadvantages include: it
is fragile and expensive.

Fluid Mechanics/ Fluid Flow 12
iv) Gravity bottle
This is a glass flask with a cap or topper, as shown in Figure 4 below. It is also known as
pycnometer. The procedure is as follows: first is to clean the 50cm3 gravity bottle, dry it in an
oven and measure it weight using an electronic balance and record the weight as M1. Second is to
fill the bottle with water sample to the top and then return the cap, allowing the excess water to
flow out. Third is to dry the bottle, weigh it and record the weight as M2. Fourth is to determine
the difference in weight of the empty and water-filled gravity bottle (M2 – M1). The density of
water is determined by dividing (M2 – M1) by the volume of the bottle (50cm3). Advantages of
this method include: it is affordable, and can provide accurate and reliable results if temperature
is controlled during the experiment. Disadvantages include: it is slow and time consuming, the
bottle can break easily, it requires the skills of a skilled operator, and requires a large volume of
water sample.
Figure 4: Gravity bottle (Paar, 2019)
The best method for this situation is digital density meter. This is because the method is fast,
accurate, reliable, easy to perform, requires small amount of water sample, and it is not affected
by temperature or pressure.

Fluid Mechanics/ Fluid Flow 13
References
Amrita.OLabs.edu.in, 2011. Determination of density of solid. [Online]
Available at: https://amrita.olabs.edu.in/?sub=1&brch=1&sim=2&cnt=21
[Accessed 24 July 2019].
Bird, J., 2013. Science for Engineering. 4th ed. Abingdon, UK: Routledge.
Hall, F., 2015. Building Services and Equipment, Volume 3. 3rd ed. Abingdon, UK: Routledge.
Hall, N., 2015. Mass Flow Rate. [Online]
Available at: https://www.grc.nasa.gov/www/k-12/airplane/mflow.html
[Accessed 24 July 2019].
Paar, A., 2019. Density and density measurement. [Online]
Available at: https://wiki.anton-paar.com/en/density-and-density-measurement/
[Accessed 24 July 2019].