Calculation of Head Losses and Flow Rate in Pipes

This document provides a step-by-step solution to calculate head losses and flow rate in pipes. It includes the given parameters, solutions, and final results. The document also covers major and minor head losses in pipes, sudden contraction, sudden enlargement, and pipe bend. The subject is not mentioned.

[Type the document title]
[Type the abstract of the document here. The abstract is typically a short summary of the
contents of the document. Type the abstract of the document here. The abstract is typically
a short summary of the contents of the document.]
[Year]
[Type the company
name]
Windows User

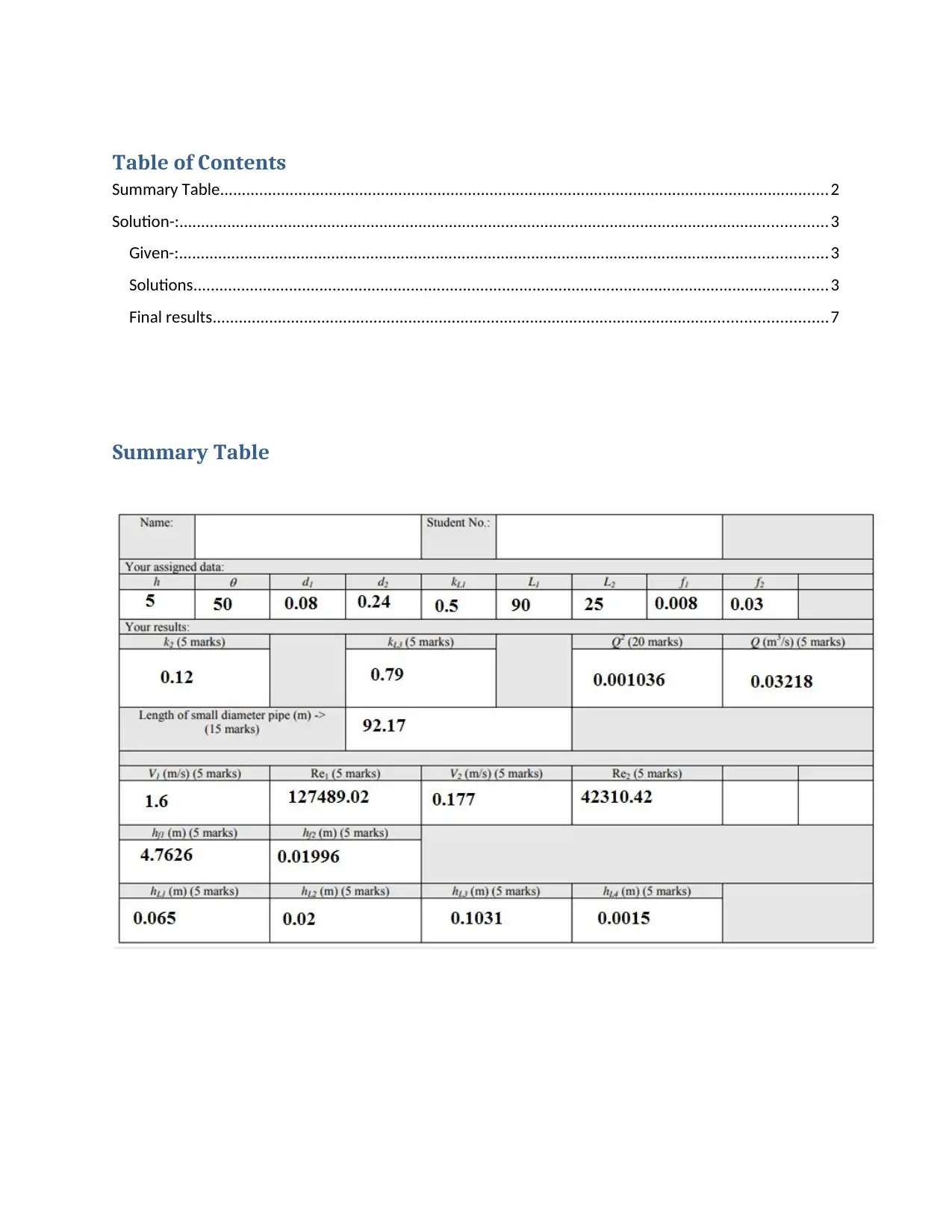
Table of Contents
Summary Table............................................................................................................................................2
Solution-:.....................................................................................................................................................3
Given-:.....................................................................................................................................................3
Solutions..................................................................................................................................................3
Final results.............................................................................................................................................7
Summary Table

Solution-:
Given-:
 Available head = 5m
 Horizontal length of d1 diameter pipe, L = 90m
 Horizontal length of d2 diameter pipe, L = 25m
 Diameter of small pipe, d1 = 0.08m
 Diameter of big pipe, d2 = 0.24m
 Friction factor of d1 diameter pipe, f =0.008
 Friction factor of d2 diameter pipe, f =0.03
 Coefficient of loss due to sudden contraction, k = 0.5
 Coefficient of loss due to sudden enlargement open to tank 2, k =1
 Inclination of d1 diameter pipe, = 50
 Centerline radius of bend = 300mm =0.3m
Solutions
Coefficient of bend
Ratio = centerline radius of bend
internaldiameter of pipe ¿ 0.3
0.08 = 3.75
Form given graph of coefficient of bend loss and ratio of centerline radius of bend and
internal diameter of pipe-
Coefficient of bend, kL2 = 0.112 (approx)
Coefficient of sudden enlargement from small to bigger diameter pipe
kL3 =
[ 1−( a1
a2 )
2
] 2
=
[ 1−( d 1
d 2 )
2
]
2
=
[1−( 0.08
0.24 )2
]2
kL3= 0.79
By continuity equation

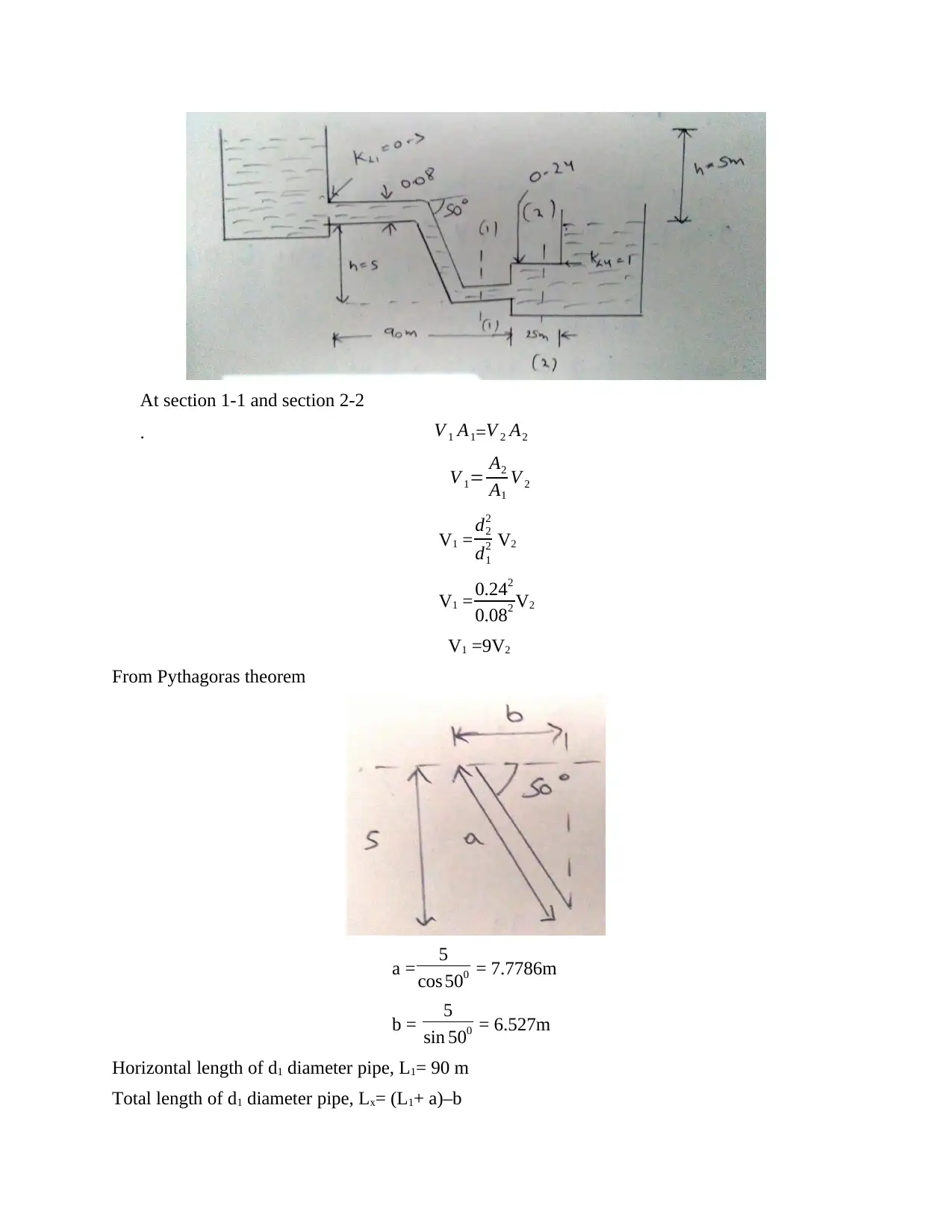
At section 1-1 and section 2-2
. V 1 A1=V 2 A2
V 1= A2
A1
V 2
V1 = d2
2
d1
2 V2
V1 = 0.242
0.082 V2
V1 =9V2
From Pythagoras theorem
a = 5
cos 500 = 7.7786m
b = 5
sin 500 = 6.527m
Horizontal length of d1 diameter pipe, L1= 90 m
Total length of d1 diameter pipe, Lx= (L1+ a)–b

Lx= (90 + 7.7786) -6.527
Lx = 91.2516m
Applying Bernoulli’s equation to the free surface of water in the two tanks, we have :–
H = hL1 + 2 * hL2 + hf1 + hL3 + hf1 + hL4
H =kL1* V 1
2
2 g + 2 * KL2 * V 1
2
2 g + 4 f 1 Lx
d1
* V 1
2
2 g + k L3∗V 1
2
2 g + 4 f 2 L2
d2
* V 2
2
2 g +KL4¿ V 2
2
2 g
From equation (i), V1 =9V2
By putting above value in equation (ii)
H = KL1 * V 1
2
2 g + 2 KL2 * V 1
2
2 g + 4 f 1 Lx
d1
* V 1
2
2 g +KL3 * V 1
2
2 g + 4 f 2 L2
d2
( 9 V 1 )2
2 g +KL4 *
( 9 V 1 )2
2 g
5 = V 1
2
2 X 9.81 [ 0.5+ 0.24+36.5+0.79+ 0.154+0.0123 ]
V 1
2=2.5683
V 1=1.6m/sec
Velocities in both pipes
V1=1.6 m/sec
From equation (i)
V1=9V2
V2= V 1
9 = 1.6
9
V2= 0.177 m/sec
Discharge, Q
Q = V1A1=V2A2
Q=V1* π
4 d1
2 = 1.6 * π
4 * (0.08)2
Q=0.03218 m3 /sec
Reynolds number value according to velocities
In small pipe diameter, d1 :-
Re1= V1 * d1 * ρ
μ
Re1= 1.6 * 0.08 * 998
1.002 X 10−3

Re1= 127489.02
In big pipe diameter pipe, d2 :-
Re2= V2* d2* ρ
μ
Re2= 0.177 * 0.24 * 998
1.002 X 10−3
Re2= 42310.42
Major Head losses in pipes
In small pipe diameter, d1 :-
hf1 = 4 f 1 lxV 1
2
2 g d1
hf1 = 4∗0.008∗91.2516∗1.62
2∗9.81∗0.08
hf1 = 4.7626m
In big pipe diameter pipe, d2 :-
hf2 = 4 f 2 V 2
2 lx
2 g d2
hf2 =
4∗0.03∗25∗0.1772
2∗9.81∗0.24
hf2 =0.01996m
Minor head losses in pipe
At sudden contraction
HL1 = kL1
V 1
2
2 g
HL1= 0.5 * 1.62
2∗9.81
HL1 = 0.065m
At pipe bend
HL2 = kL2 ¿ V 1
2
2 g
HL2 = 0.12 ¿ 1.62
2 g
HL2 = 0.02 m
At sudden enlargement from small diameter pipe to big diameter pipe

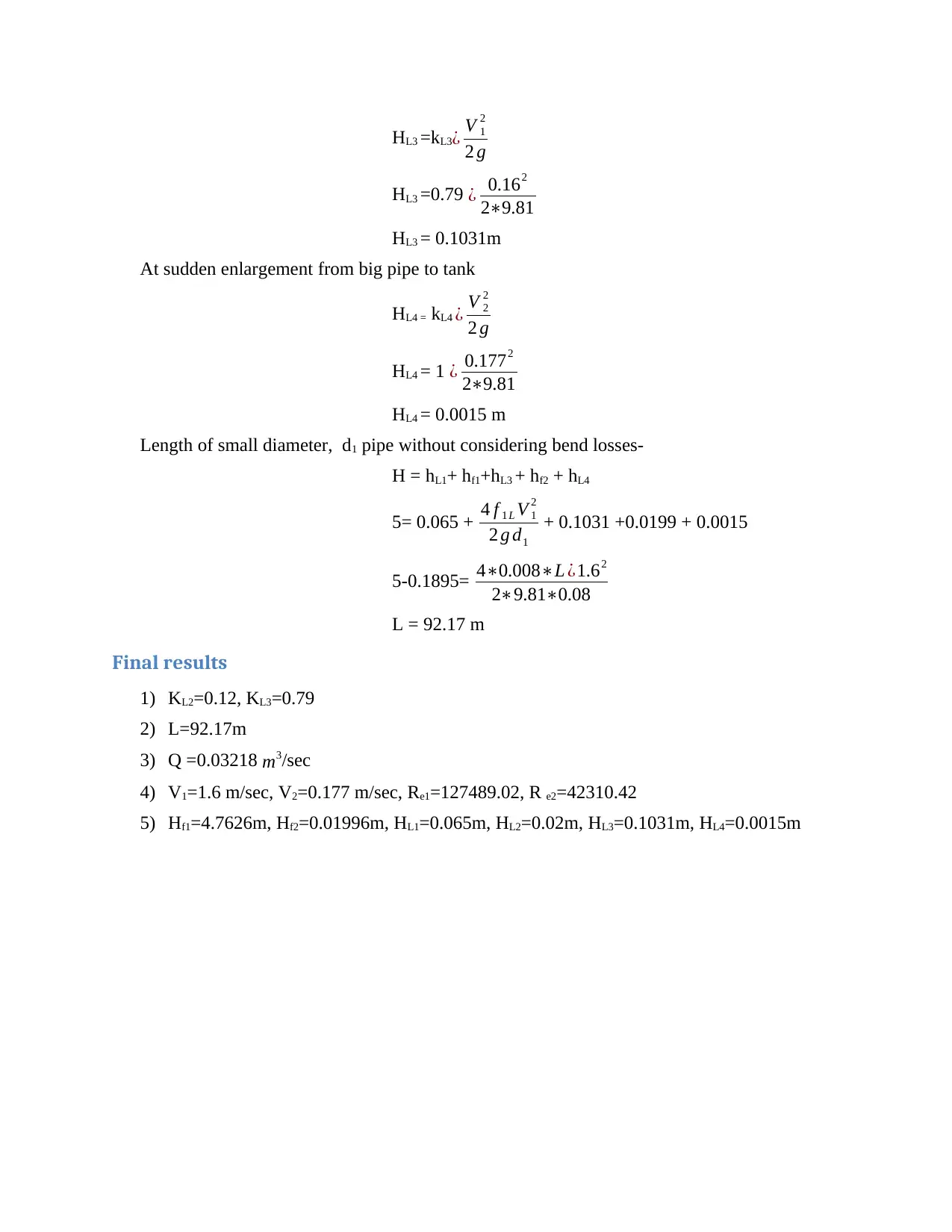
HL3 =kL3¿ V 1
2
2 g
HL3 =0.79 ¿ 0.162
2∗9.81
HL3 = 0.1031m
At sudden enlargement from big pipe to tank
HL4 = kL4 ¿ V 2
2
2 g
HL4 = 1 ¿ 0.1772
2∗9.81
HL4 = 0.0015 m
Length of small diameter, d1 pipe without considering bend losses-
H = hL1+ hf1+hL3 + hf2 + hL4
5= 0.065 + 4 f 1 L V 1
2
2 g d1
+ 0.1031 +0.0199 + 0.0015
5-0.1895= 4∗0.008∗L ¿1.62
2∗9.81∗0.08
L = 92.17 m
Final results
1) KL2=0.12, KL3=0.79
2) L=92.17m
3) Q =0.03218 m3/sec
4) V1=1.6 m/sec, V2=0.177 m/sec, Re1=127489.02, R e2=42310.42
5) Hf1=4.7626m, Hf2=0.01996m, HL1=0.065m, HL2=0.02m, HL3=0.1031m, HL4=0.0015m