Applied Quantitative Methods: Analysis of Relationship between Flights and Baggage Sales, Contingency Table Probability
VerifiedAdded on 2023/06/03
|9
|1746
|197
AI Summary
This article analyzes the relationship between flights and baggage sales using standard deviation, interquartile range, and coefficient of correlation. It also explores probability using a contingency table for a cricket team's recruitment and training modes.
Contribute Materials
Your contribution can guide someone’s learning journey. Share your
documents today.

University
Applied Quantitative Methods
By
Your Name
Date
Page 1 of 9
<Your Name> 2018
Applied Quantitative Methods
By
Your Name
Date
Page 1 of 9
<Your Name> 2018
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

Question 2
Information about the number of flights at a given airport and the number of baggage’s sold is
provided to a manager. The manager needs to use the information to examine whether there
exists a relationship between the flights in the airport and the baggage’s sold, so that he keeps
more baggage’s in stock during busy hours of the airport in the upcoming holiday. A series of
questions is to be answered using the data as below:
Solutions
a. The data provided for the flights and the baggage’s is a sample. It is different from a
population in that, it uses old airport flight schedule to only indicate portion of the flights
recorded in the airport during a period of 7 weeks, and the number of baggage’s that were
sold during this period and when only the recorded flights were within the airport unlike
a population which would represent all the flights in the airport and baggage’s sold
during the period.
b. Since it is a sample the formula for standard deviation for a sample is applied as follows:
σ = √ ∑ (x−μ)2
n−1
The x represents the value of flights, n is the sample size and μ represents the mean [1].
To simplify the process of, the data required for calculation of the standard deviation is
first simplified in table form as follows.
Page 2 of 9
<Your Name> 2018
Information about the number of flights at a given airport and the number of baggage’s sold is
provided to a manager. The manager needs to use the information to examine whether there
exists a relationship between the flights in the airport and the baggage’s sold, so that he keeps
more baggage’s in stock during busy hours of the airport in the upcoming holiday. A series of
questions is to be answered using the data as below:
Solutions
a. The data provided for the flights and the baggage’s is a sample. It is different from a
population in that, it uses old airport flight schedule to only indicate portion of the flights
recorded in the airport during a period of 7 weeks, and the number of baggage’s that were
sold during this period and when only the recorded flights were within the airport unlike
a population which would represent all the flights in the airport and baggage’s sold
during the period.
b. Since it is a sample the formula for standard deviation for a sample is applied as follows:
σ = √ ∑ (x−μ)2
n−1
The x represents the value of flights, n is the sample size and μ represents the mean [1].
To simplify the process of, the data required for calculation of the standard deviation is
first simplified in table form as follows.
Page 2 of 9
<Your Name> 2018

The standard deviation will be given by
¿ √ 151.43
6 =5.02
c. The interquartile range is the range the difference between the third quartile and the first
quartile [2]. To determine the interquartile range, the data is first arranged in ascending
order and the first (Q1), second (Q2) and third (Q3) quartiles determined. The data in
ascending order is as shown below:
Inter quartilerange=Third quartile−First Quartile
Interquartile range=40−33=7
The interquartile range is more useful than standard deviation where the data is not
perfectly normally distributed because of outliers or because of being skewed [2]. For
example, where the baggage’s sold is 43 indicates that this is an outlier and therefore,
interquartile range would be more useful for analysis.
d. Coefficient of correlation is a value that indicates the strength and direction of linear
relationship between variables [3]. The relationship is strong positive if it approaches
positive one and strong negative if tit approaches the negative one. The calculation of the
coefficient of correlation “r” is calculated using the equation below:
Page 3 of 9
<Your Name> 2018
¿ √ 151.43
6 =5.02
c. The interquartile range is the range the difference between the third quartile and the first
quartile [2]. To determine the interquartile range, the data is first arranged in ascending
order and the first (Q1), second (Q2) and third (Q3) quartiles determined. The data in
ascending order is as shown below:
Inter quartilerange=Third quartile−First Quartile
Interquartile range=40−33=7
The interquartile range is more useful than standard deviation where the data is not
perfectly normally distributed because of outliers or because of being skewed [2]. For
example, where the baggage’s sold is 43 indicates that this is an outlier and therefore,
interquartile range would be more useful for analysis.
d. Coefficient of correlation is a value that indicates the strength and direction of linear
relationship between variables [3]. The relationship is strong positive if it approaches
positive one and strong negative if tit approaches the negative one. The calculation of the
coefficient of correlation “r” is calculated using the equation below:
Page 3 of 9
<Your Name> 2018
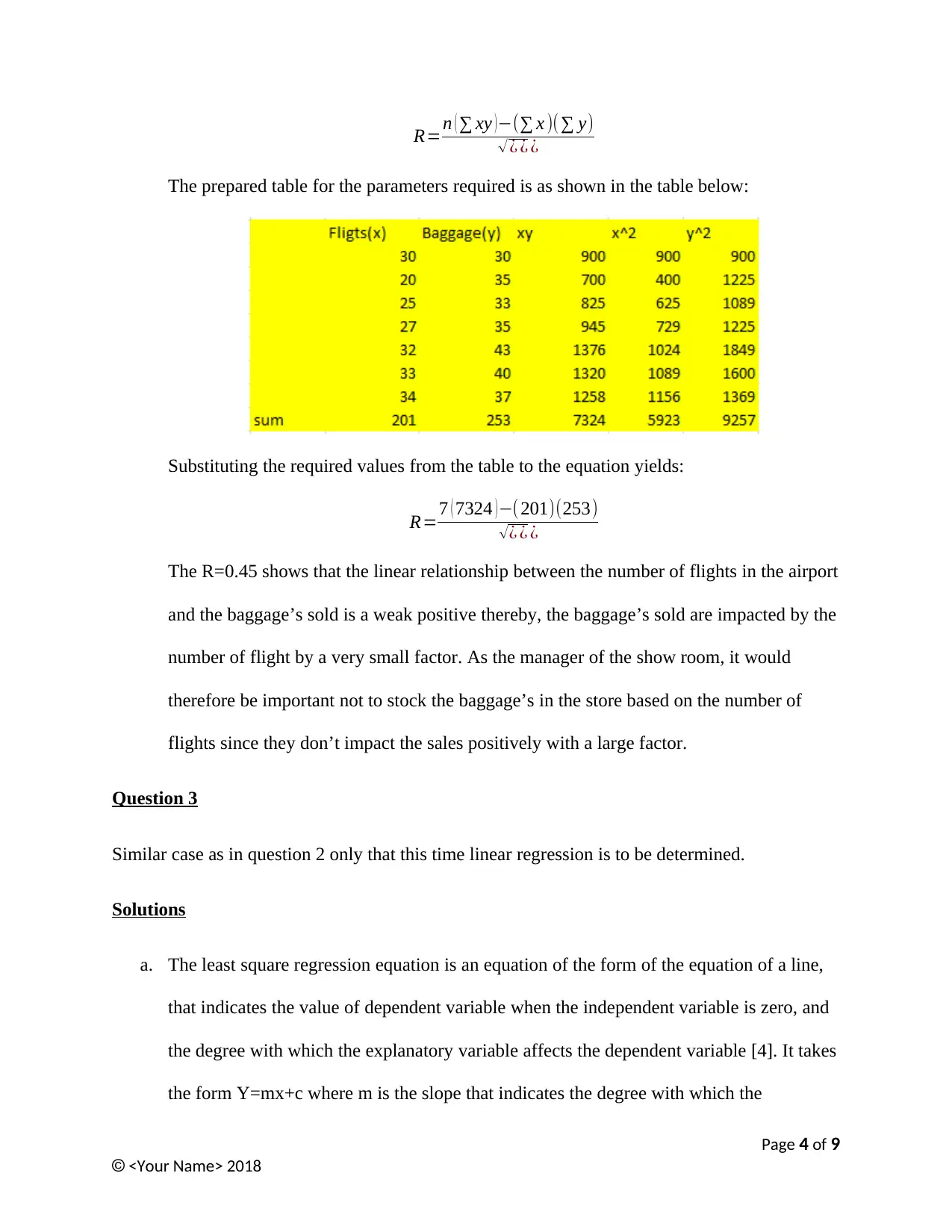
R=n ( ∑ xy ) −(∑ x )( ∑ y)
√ ¿ ¿ ¿
The prepared table for the parameters required is as shown in the table below:
Substituting the required values from the table to the equation yields:
R=7 ( 7324 ) −(201)(253)
√ ¿ ¿ ¿
The R=0.45 shows that the linear relationship between the number of flights in the airport
and the baggage’s sold is a weak positive thereby, the baggage’s sold are impacted by the
number of flight by a very small factor. As the manager of the show room, it would
therefore be important not to stock the baggage’s in the store based on the number of
flights since they don’t impact the sales positively with a large factor.
Question 3
Similar case as in question 2 only that this time linear regression is to be determined.
Solutions
a. The least square regression equation is an equation of the form of the equation of a line,
that indicates the value of dependent variable when the independent variable is zero, and
the degree with which the explanatory variable affects the dependent variable [4]. It takes
the form Y=mx+c where m is the slope that indicates the degree with which the
Page 4 of 9
<Your Name> 2018
√ ¿ ¿ ¿
The prepared table for the parameters required is as shown in the table below:
Substituting the required values from the table to the equation yields:
R=7 ( 7324 ) −(201)(253)
√ ¿ ¿ ¿
The R=0.45 shows that the linear relationship between the number of flights in the airport
and the baggage’s sold is a weak positive thereby, the baggage’s sold are impacted by the
number of flight by a very small factor. As the manager of the show room, it would
therefore be important not to stock the baggage’s in the store based on the number of
flights since they don’t impact the sales positively with a large factor.
Question 3
Similar case as in question 2 only that this time linear regression is to be determined.
Solutions
a. The least square regression equation is an equation of the form of the equation of a line,
that indicates the value of dependent variable when the independent variable is zero, and
the degree with which the explanatory variable affects the dependent variable [4]. It takes
the form Y=mx+c where m is the slope that indicates the degree with which the
Page 4 of 9
<Your Name> 2018
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

independent variable affects the dependent variable, c is the intercept that shows the
value of the dependent variable when the independent variable is zero, y is the dependent
variable and x is the independent variable.
The slope is determined as follows:
m= n ( ∑ xy )−(∑ x)(∑ y )
n(∑ x2 )−¿ ¿
Now substituting the required parameters from the table above:
m= 7 ( 7324 ) −(201)(203)
7 ( 5923 )− ( 201 )2 = 405
1060 =0.39
The intercept “c” is determined as follows:
c= ( ∑ y ) ( ∑ x2 )−(∑ x) ( ∑ xy )
n(∑ x2)−¿ ¿
Substituting the required parameters from the table above:
c= ( 253 ) ( 5923 ) −201(7324)
7 ( 5923 ) − ( 201 ) 2 = 26395
1060 =24.9
The regression equation is thus:
Y =0.39 x +24.9
Y is the number of baggage’s sold (Dependent Variable) while x is the number of flights
in the airport (Independent Variable). When there are ten extra flights in the airport, the
manager would be required to stock approximately 29 more baggage’s in the store as per
the equation below.
Y =0.39 (10 )+ 24.9=28.8
b. The coefficient of determination indicates the probability of future outcomes being within
the outcome predicted [5]. It is the multiple r square and is given by:
Page 5 of 9
<Your Name> 2018
value of the dependent variable when the independent variable is zero, y is the dependent
variable and x is the independent variable.
The slope is determined as follows:
m= n ( ∑ xy )−(∑ x)(∑ y )
n(∑ x2 )−¿ ¿
Now substituting the required parameters from the table above:
m= 7 ( 7324 ) −(201)(203)
7 ( 5923 )− ( 201 )2 = 405
1060 =0.39
The intercept “c” is determined as follows:
c= ( ∑ y ) ( ∑ x2 )−(∑ x) ( ∑ xy )
n(∑ x2)−¿ ¿
Substituting the required parameters from the table above:
c= ( 253 ) ( 5923 ) −201(7324)
7 ( 5923 ) − ( 201 ) 2 = 26395
1060 =24.9
The regression equation is thus:
Y =0.39 x +24.9
Y is the number of baggage’s sold (Dependent Variable) while x is the number of flights
in the airport (Independent Variable). When there are ten extra flights in the airport, the
manager would be required to stock approximately 29 more baggage’s in the store as per
the equation below.
Y =0.39 (10 )+ 24.9=28.8
b. The coefficient of determination indicates the probability of future outcomes being within
the outcome predicted [5]. It is the multiple r square and is given by:
Page 5 of 9
<Your Name> 2018
coefficient of determination=R2=0.452=0.2025
If a new sample of flights and baggage’s was taken, there would be a 0.2025 chance that
the relationship between the two would fall within the predicted outcome.
Question 4
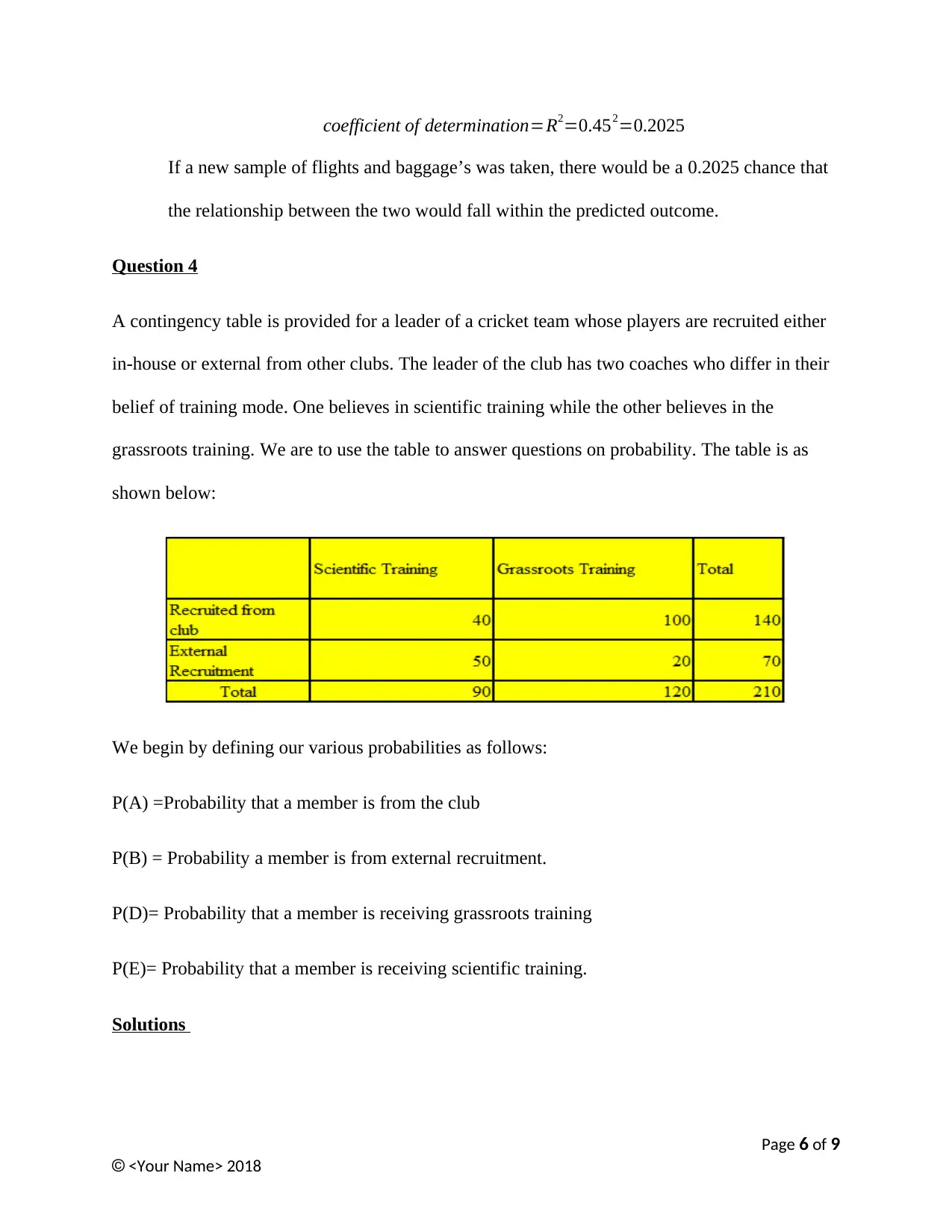
A contingency table is provided for a leader of a cricket team whose players are recruited either
in-house or external from other clubs. The leader of the club has two coaches who differ in their
belief of training mode. One believes in scientific training while the other believes in the
grassroots training. We are to use the table to answer questions on probability. The table is as
shown below:
We begin by defining our various probabilities as follows:
P(A) =Probability that a member is from the club
P(B) = Probability a member is from external recruitment.
P(D)= Probability that a member is receiving grassroots training
P(E)= Probability that a member is receiving scientific training.
Solutions
Page 6 of 9
<Your Name> 2018
If a new sample of flights and baggage’s was taken, there would be a 0.2025 chance that
the relationship between the two would fall within the predicted outcome.
Question 4
A contingency table is provided for a leader of a cricket team whose players are recruited either
in-house or external from other clubs. The leader of the club has two coaches who differ in their
belief of training mode. One believes in scientific training while the other believes in the
grassroots training. We are to use the table to answer questions on probability. The table is as
shown below:
We begin by defining our various probabilities as follows:
P(A) =Probability that a member is from the club
P(B) = Probability a member is from external recruitment.
P(D)= Probability that a member is receiving grassroots training
P(E)= Probability that a member is receiving scientific training.
Solutions
Page 6 of 9
<Your Name> 2018

a. The probability that a randomly chosen player will be from the club OR receiving grass
root training.
P ( A∨D ) =P ( A )+ P ( D ) −P ( A∧D )
P ( A )= 140
210
Probability Grassroot P ( D )= 120
210
ProbabilityP( A∧D)= 100
210
Therefore,
P ( A∨D ) =P ( A )+ P ( D ) −P ( A∧D )
140
210 + 120
210 +100
210 = 16
21 =0.7619
b. Probability that a randomly selected player will be external AND be in scientific training,
P ( B∧E ) = 50
210 =0.2381
c. Probability that a player is scientific given that the player is from club members:
P ( E|A ) = 40
140 =0.2857
d. Whether training is independent from recruitment proof.
If the training is independent of recruitment then,
P ( T ∧S ) =P ( T |S )∗P ( S ) =P ( T )∗P( S)
Where P(T) is the probability of training and P(S) the probability of recruitment.
Using part B above for P (B and E), we have
P ( B∧E ) =P ( B|E ) ∗P ( E ) =P ( B ) XP( E)
Page 7 of 9
<Your Name> 2018
root training.
P ( A∨D ) =P ( A )+ P ( D ) −P ( A∧D )
P ( A )= 140
210
Probability Grassroot P ( D )= 120
210
ProbabilityP( A∧D)= 100
210
Therefore,
P ( A∨D ) =P ( A )+ P ( D ) −P ( A∧D )
140
210 + 120
210 +100
210 = 16
21 =0.7619
b. Probability that a randomly selected player will be external AND be in scientific training,
P ( B∧E ) = 50
210 =0.2381
c. Probability that a player is scientific given that the player is from club members:
P ( E|A ) = 40
140 =0.2857
d. Whether training is independent from recruitment proof.
If the training is independent of recruitment then,
P ( T ∧S ) =P ( T |S )∗P ( S ) =P ( T )∗P( S)
Where P(T) is the probability of training and P(S) the probability of recruitment.
Using part B above for P (B and E), we have
P ( B∧E ) =P ( B|E ) ∗P ( E ) =P ( B ) XP( E)
Page 7 of 9
<Your Name> 2018
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

P ( B∧E ) = 50
210
P ( B ) = 70
210
P ( E )= 90
210
P ( B|E )= 50
90
Now,
P ( B∧E ) =P ( B|E ) ∗P ( E ) =P ( B ) XP( E)
50
210 =
50
90∗90
210 = 70
210 X 90
210
0.238=0.238=0.761
Because they are not the same training is not independent of recruitment [6].
References
[1] A. J. John, W. David and G. J. Davidson, "Statistical Thinking in Business," 2nd ed., Boca
Page 8 of 9
<Your Name> 2018
210
P ( B ) = 70
210
P ( E )= 90
210
P ( B|E )= 50
90
Now,
P ( B∧E ) =P ( B|E ) ∗P ( E ) =P ( B ) XP( E)
50
210 =
50
90∗90
210 = 70
210 X 90
210
0.238=0.238=0.761
Because they are not the same training is not independent of recruitment [6].
References
[1] A. J. John, W. David and G. J. Davidson, "Statistical Thinking in Business," 2nd ed., Boca
Page 8 of 9
<Your Name> 2018

Raton: Chapman & Hall/CRC, 2006.
[2] A. Graham, "Statistics," 2nd ed., London: Hodder Education, 2011.
[3] B. S. Daniel, "Statistics: An Introoduction to Quantitative Economic Research," 4th ed.,
Chicago: Rand McNally, 2006.
[4] C. Thomas, "Explanatory Data Analysis in Business and Economics : An Introduction Using
SPSS, Stata, and Excel," Cham : Springer, 2014.
[5] R. A. David, J. S. Dennis, A. W. Thomas, D. C. Jeffrey and J. C. James, "Statistics for
Business & Economics," 13th ed., Boston: Cengage Learning, 2018.
[6] S. C. John, "Introductory Mathematics & Statistics," 6th ed., North Ryde: N.S.W. McGraw-
Hill Education, 2016.
Page 9 of 9
<Your Name> 2018
[2] A. Graham, "Statistics," 2nd ed., London: Hodder Education, 2011.
[3] B. S. Daniel, "Statistics: An Introoduction to Quantitative Economic Research," 4th ed.,
Chicago: Rand McNally, 2006.
[4] C. Thomas, "Explanatory Data Analysis in Business and Economics : An Introduction Using
SPSS, Stata, and Excel," Cham : Springer, 2014.
[5] R. A. David, J. S. Dennis, A. W. Thomas, D. C. Jeffrey and J. C. James, "Statistics for
Business & Economics," 13th ed., Boston: Cengage Learning, 2018.
[6] S. C. John, "Introductory Mathematics & Statistics," 6th ed., North Ryde: N.S.W. McGraw-
Hill Education, 2016.
Page 9 of 9
<Your Name> 2018
1 out of 9
Related Documents
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.


