Statistics for Economists

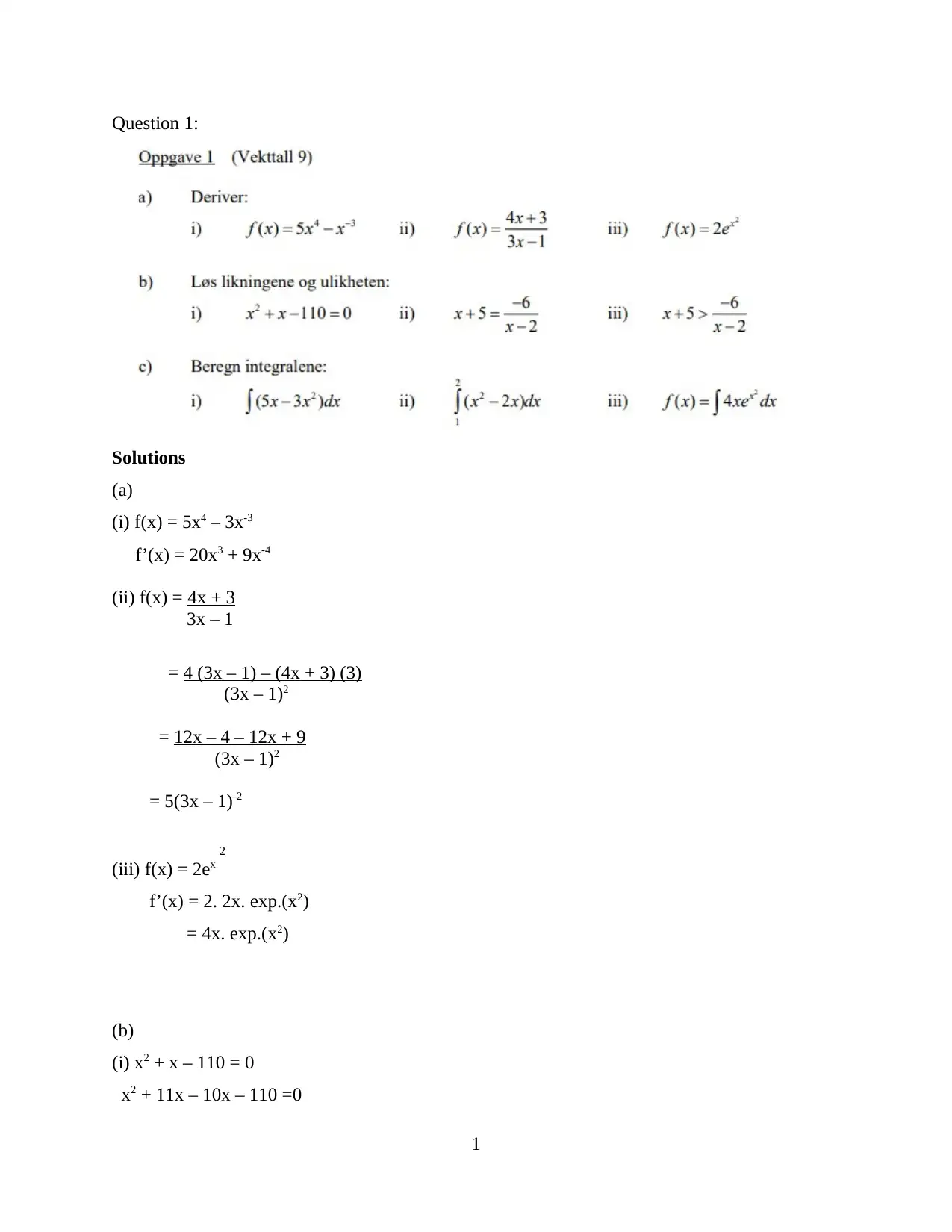
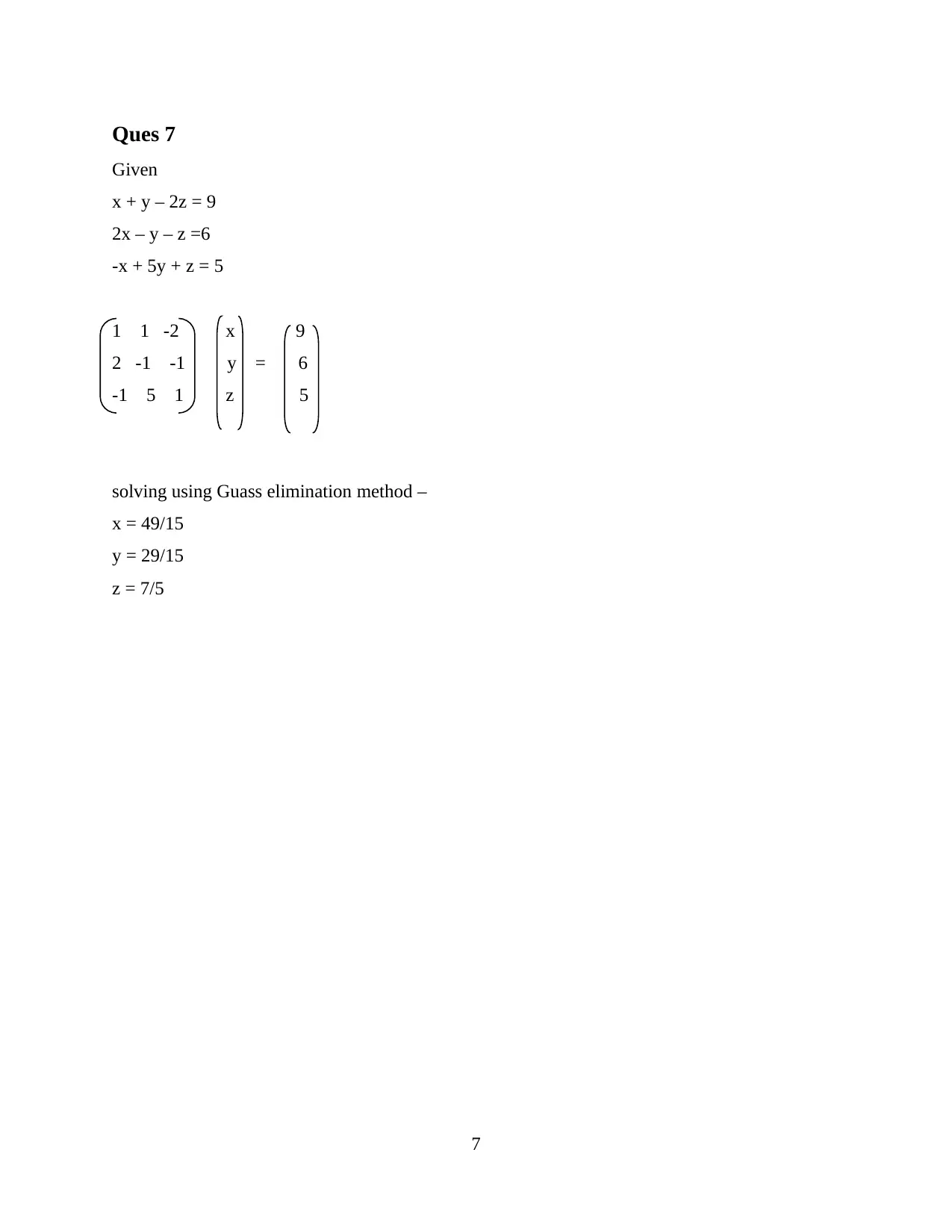
This document provides solutions for various statistics problems for economists. It includes topics such as differentiation, integration, cost and profit maximization, and solving linear equations. The solutions are explained step by step.

Statistics for Economists

Question 1:
Solutions
(a)
(i) f(x) = 5x4 – 3x-3
f’(x) = 20x3 + 9x-4
(ii) f(x) = 4x + 3
3x – 1
= 4 (3x – 1) – (4x + 3) (3)
(3x – 1)2
= 12x – 4 – 12x + 9
(3x – 1)2
= 5(3x – 1)-2
2
(iii) f(x) = 2ex
f’(x) = 2. 2x. exp.(x2)
= 4x. exp.(x2)
(b)
(i) x2 + x – 110 = 0
x2 + 11x – 10x – 110 =0
1

x (x + 11) – 10 (x + 11) = 0
(x + 11) (x – 10) = 0
so, x = 10, -11
(ii) x + 5 = -6 .
x – 2
(x + 5) (x – 2) = -6
(x2 + 3x – 10) = -6
x2 + 3x – 4 = 0
x2 + 4x – 1x – 4 = 0
x (x + 4) – 1(x + 4) =0
(x – 1) (x + 4) = 0
x = 1, -4
(iii) x + 5 > -6 .
x – 2
(x + 5) (x – 2) > -6
(x2 + 3x – 10) > -6
(x2 + 3x – 10) + 6 > -6 + 6
x2 + 3x – 4 > 0
x2 + 4x – 1x – 4 > 0
x (x + 4) – 1(x + 4) > 0
(x – 1) (x + 4) > 0
-4 < x < 1
2

(c)
(i) ∫(5x – 3x2) dx
= 5x2 – 3 x3
2 3
= 5x2 – x3
2
2
(ii) ∫1 (x2 – 2x) dx
x3 – x2 2
3 1
8 – 1 - 1 + 22
3 3
7 – 3 = -2/3
3
(iii) ∫ 4x exp.(x2) .dx
Put exp.(x2) = u
2x. exp(x2) dx = du
Then, ∫2.u. du
= u2
= [exp.(x2)]2
3

Ques 2.
Solution
Given, f(x) = x3 – 3x2 – x + 3
(a) If given function can divide with x – 3 then, f(3) will equal to zero
f(3) = (3)3 – 3(3)2 – 3 + 3
= 27 – 27 – 3 + 3
= 0
Hence, f(x) is divisible by x-3
(b)
x3 – 3x2 – x + 3
(x – 3) (x2 – 1)
(x + 1) (x – 3) (x – 1)
So,
at x = 1, -1 and 3 given f(x) = 0
while, -1 ≤ x ≤ 3
(c)
f(x) = x3 – 3x2 – x + 3
f’(x) = 3x2 – 6x – 1
f’(2) = 3(2)2 – 6 (2) – 1
= 12 – 12 – 1 = -1
So, function is decreasing at x = 2
4

Ques. 4
Solution:
Given, Total cost of producing x units of an item as –
K (x) = -0.25x2 + 75x + 1000
where, 0 ≤ x ≤ 100
(a) Expression of boundary cost -
-0.25x2 + 75x + 1000 = 0
(b) How many units are produced when the limit cost is NOK 60?
When limit cost is 60 then,
K(60) = -0.25(60)2 + 75(60) + 1000
= -0.25 x 3600 + 75 x 60 + 1000
= -900 + 4500 + 1000
= 4600 units
(c) Expression of average cost –
Average cost = Total production cost
Quantity of unit produced
= -0.25x2 + 75x + 1000
x
= -0.25x + 75 + 1000/x
(d) What is the average profit per unit sold when producing 50 units and each unit in this case
sold for 100 kroner?
Average profit per unit at x = 50
Average cost = -0.25(50) + 75 + 1000/50
= 107.5 kroner per unit
Then, average profit per unit will be 7.5 kroner.
5

Ques. 5
Solution –
P = 210 – 3x – y
Q = 180 – x – 2y
Total cost of production
c (x.y) = 1200 + 100x + 80y
Total profit = Total selling cost – Total production cost
f(x,y) = Px + Qy – c(x.y)
= x (210 – 3x – y) + y (180 – x – 2y) – (1200 + 100x + 80y)
= 210 x – 3x2 – xy + 180y – xy – 2y2 – 1200 – 100x – 80y
= -3x2 – 2xy – 2y2 + 110x +100y – 1200
(b) For maximise the profit –
f(x) = -3x2 – 2xy – 2y2 + 110x +100y – 1200
By differentiating with respect to x and y individually
f(x) = -6x -2y + 110
f(y) = -2x – 4y + 100
y = 24 and x = 3 (approx.)
(c) Proof –
Use D-test for (3,24) to check maximum value of given function
D = fxx (3,24) fyy (3,24) – [fxy(3,24)]2
= (-6) (-4) – 1200
= 24 – 1200 = -1176
so, (3,24) gives maximum value of given function
6

Ques 7
Given
x + y – 2z = 9
2x – y – z =6
-x + 5y + z = 5
1 1 -2 x 9
2 -1 -1 y = 6
-1 5 1 z 5
solving using Guass elimination method –
x = 49/15
y = 29/15
z = 7/5
7

8