Design and Analysis of Water Supply Network Distribution and Sewer System
VerifiedAdded on 2023/06/08
|24
|3532
|220
AI Summary
This report analyzes the design and methodology of water supply network distribution and sewer system. It includes the design of pipeline circulation network and the assumptions considered on particles, temperature, and speed that may impact the water and sewer properties.
Contribute Materials
Your contribution can guide someone’s learning journey. Share your
documents today.

Abstract
This report shows and surveys the extent of possible design for the water supply network
distribution and the design for sewer system. This report begins with the analysis of water supply
network distribution. The design methodology in this report for clean sewer framework will be
actualized in perspective of the conditions of pseudo-plastic fluids. At the point when profluent
is mixed with water, it acts as pseudo-plastic. This report shows the design of pipeline circulation
network. Assumption has been considered on the particles, temperature and speed and other
basic properties that may impact the water and sewer properties
This report shows and surveys the extent of possible design for the water supply network
distribution and the design for sewer system. This report begins with the analysis of water supply
network distribution. The design methodology in this report for clean sewer framework will be
actualized in perspective of the conditions of pseudo-plastic fluids. At the point when profluent
is mixed with water, it acts as pseudo-plastic. This report shows the design of pipeline circulation
network. Assumption has been considered on the particles, temperature and speed and other
basic properties that may impact the water and sewer properties
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

Introduction
The pipe network system for series and parallel each do have a point when the flows enters the
system and on the other point where the flow is exiting, permitting the flow course in each and
every pipe to be derived explicitly. For such a case, in the event that we are simply intrigued by
the connection between total flow and aggregate head loss, it is some of the time helpful to
improve the analysis by representation of the entire group of pipes as a solitary, using hydraulic
water identical pipe.
The piping system for the friction intensity constraint for every pipe should always be known,
and it should be free of the flow situations for the scope of flow states of intrigue. In the event
that the distribution water condition is utilized to relate head loss hL to velocity V or flow rate Q
(Spiliotis and Tsakiris, 2010), the representation of friction intensity factor is denoted by f, this
has a steady value on a specific pipe for completely turbulent flow, however it does not imply on
the transitional or laminar flow. In the event that the Hazen Williams equation (Kumar,
Narasimhan and Bhallamudi, 2010) is utilized, the friction intensity factor is CHW, which is
thought to be identified for a specific pipe.
The Hardy cross method used in analyzing the pipe network system illuminate the nonlinear
conditions associated with network investigation by making certain assumptions. The higher
power rectification terms can be dismissed and the loop number is little for a solitary loop
despite the fact that the underlying guess is weak. However, dismissing contiguous loops and
considering just a single amendment condition at once can influence the arrangement and
furthermore number of iteration required for joining increments as the measure of the system
increments.
Altered Hardy Cross strategy can be connected to enhance merging and lessen the quantity of
loops. In any case, this number can be very substantial for genuine systems. Consequently, rather
than considering just a single amendment condition at once, all the adjustment conditions can be
illuminated by thinking about the impact of every single contiguous circle. So joining can be
accomplished in fewer loops. Additionally, a portion of the conditions engaged with pipe
network investigation is nonlinear.
The pipe network system for series and parallel each do have a point when the flows enters the
system and on the other point where the flow is exiting, permitting the flow course in each and
every pipe to be derived explicitly. For such a case, in the event that we are simply intrigued by
the connection between total flow and aggregate head loss, it is some of the time helpful to
improve the analysis by representation of the entire group of pipes as a solitary, using hydraulic
water identical pipe.
The piping system for the friction intensity constraint for every pipe should always be known,
and it should be free of the flow situations for the scope of flow states of intrigue. In the event
that the distribution water condition is utilized to relate head loss hL to velocity V or flow rate Q
(Spiliotis and Tsakiris, 2010), the representation of friction intensity factor is denoted by f, this
has a steady value on a specific pipe for completely turbulent flow, however it does not imply on
the transitional or laminar flow. In the event that the Hazen Williams equation (Kumar,
Narasimhan and Bhallamudi, 2010) is utilized, the friction intensity factor is CHW, which is
thought to be identified for a specific pipe.
The Hardy cross method used in analyzing the pipe network system illuminate the nonlinear
conditions associated with network investigation by making certain assumptions. The higher
power rectification terms can be dismissed and the loop number is little for a solitary loop
despite the fact that the underlying guess is weak. However, dismissing contiguous loops and
considering just a single amendment condition at once can influence the arrangement and
furthermore number of iteration required for joining increments as the measure of the system
increments.
Altered Hardy Cross strategy can be connected to enhance merging and lessen the quantity of
loops. In any case, this number can be very substantial for genuine systems. Consequently, rather
than considering just a single amendment condition at once, all the adjustment conditions can be
illuminated by thinking about the impact of every single contiguous circle. So joining can be
accomplished in fewer loops. Additionally, a portion of the conditions engaged with pipe
network investigation is nonlinear.

Since there is no immediate technique for their answer, these conditions are linearized and after
that tackled.
Since the arrangement is estimated, it is remedied and the iterative method is proceeded until the
point when attractive precision is come to. The Newton-Raphson strategy and the Linear Theory
technique endeavor to comprehend all the concerned conditions all the while by applying the
iterative methodology.
The Newton-Raphson strategy (Kulworawanichpong., 2010) extends the nonlinear terms in
Taylor– s arrangement, considers just the direct terms by dismissing the deposits after two terms.
Since the nonlinearity in the conditions for pipe organize examination is uniform and basic , the
nonlinear term can be effectively linearized by consolidating a piece of the nonlinear term into
the pipe opposition consistent. This standard was first utilized by McIlroy Then, Wood and
Charles] created it and it is presently generally utilized as a part of training. This standard can be
utilized to linearize a wide range of conditions. Nonetheless, by and by it is connected to pipe-
release conditions and nodal-head conditions.
A node with a known head is generally a source hub, for example, a hoisted stockpiling tank. A
node with a known stream is a request node
•At the source node, the flow is for the most part unknown,
•While at the request node, the head is for the most part obscure.
Be that as it may, sometimes the stream additionally might be known notwithstanding the known
head at a source hub, while the stream may likewise be obscure notwithstanding the obscure
head at a request hub.
Specific objectives
1. To determine nodal heads, H
2. To determine pipe discharges, Q
3. To determine nodal inflows or outflows, q
4. To determine pipe resistance constants, R
that tackled.
Since the arrangement is estimated, it is remedied and the iterative method is proceeded until the
point when attractive precision is come to. The Newton-Raphson strategy and the Linear Theory
technique endeavor to comprehend all the concerned conditions all the while by applying the
iterative methodology.
The Newton-Raphson strategy (Kulworawanichpong., 2010) extends the nonlinear terms in
Taylor– s arrangement, considers just the direct terms by dismissing the deposits after two terms.
Since the nonlinearity in the conditions for pipe organize examination is uniform and basic , the
nonlinear term can be effectively linearized by consolidating a piece of the nonlinear term into
the pipe opposition consistent. This standard was first utilized by McIlroy Then, Wood and
Charles] created it and it is presently generally utilized as a part of training. This standard can be
utilized to linearize a wide range of conditions. Nonetheless, by and by it is connected to pipe-
release conditions and nodal-head conditions.
A node with a known head is generally a source hub, for example, a hoisted stockpiling tank. A
node with a known stream is a request node
•At the source node, the flow is for the most part unknown,
•While at the request node, the head is for the most part obscure.
Be that as it may, sometimes the stream additionally might be known notwithstanding the known
head at a source hub, while the stream may likewise be obscure notwithstanding the obscure
head at a request hub.
Specific objectives
1. To determine nodal heads, H
2. To determine pipe discharges, Q
3. To determine nodal inflows or outflows, q
4. To determine pipe resistance constants, R

Considering a network that has different loops, at normal circumstances there will be channels
regular to bordering loops with a clockwise stream in one loop showing up a countercurrent flow
to clockwise in the other. Each loop must be distinguished and the rectifications made efficiently
to each loop thus. The remedy to the flows must be made each time before proceeding onward to
the following loop. In the excess of two loops in a system, the procedure turns out to be
extremely intricate and computer strategies should be utilized.
regular to bordering loops with a clockwise stream in one loop showing up a countercurrent flow
to clockwise in the other. Each loop must be distinguished and the rectifications made efficiently
to each loop thus. The remedy to the flows must be made each time before proceeding onward to
the following loop. In the excess of two loops in a system, the procedure turns out to be
extremely intricate and computer strategies should be utilized.
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

FORMULA AND EQUATIONS USED
Continuity Formula
The sum of pipe amount of flows into and out of the respective nods equals to the amount of
flow that is entering or leaving the system through each node.
Hence, from the statement it means that the following equation will be resulted: QTotal = Q1 + Q2
Where,
Q = Total inflow, Q1 + Q2= Total outflow
Formula for energy conservation
The total algebraic Summation of head loss hf around any closed loop is zero.
Therefore, ∑ hf(loop) = 0 →∑ k ( Q+∆ Q )n=0
Where,
Q= Actual inflow,
ΔQ= Correction
K= Head loss coefficient,
n= Flow exponent.
Always the following formula should be used for general relationship between discharges and
head-losses for each pipe in loops:
hf = k*Qn
Exponential friction Equation (Hazen-William)
K = 10.67
C1.85 D4.87 , n = 1.87
Continuity Formula
The sum of pipe amount of flows into and out of the respective nods equals to the amount of
flow that is entering or leaving the system through each node.
Hence, from the statement it means that the following equation will be resulted: QTotal = Q1 + Q2
Where,
Q = Total inflow, Q1 + Q2= Total outflow
Formula for energy conservation
The total algebraic Summation of head loss hf around any closed loop is zero.
Therefore, ∑ hf(loop) = 0 →∑ k ( Q+∆ Q )n=0
Where,
Q= Actual inflow,
ΔQ= Correction
K= Head loss coefficient,
n= Flow exponent.
Always the following formula should be used for general relationship between discharges and
head-losses for each pipe in loops:
hf = k*Qn
Exponential friction Equation (Hazen-William)
K = 10.67
C1.85 D4.87 , n = 1.87

∆ Q= −∑ h
2∗∑( h
Q )
Methodology
With respect to pipe network investigation, the conventionally approach is known as the Hardy
Cross procedure (Huang, Vairavamoorthy and Tsegaye, 2010). This strategy is appropriate if the
entire pipe sizes (lengths and breadths) are settled, and either the head losses between the outlets
and inlets are known yet the flow are not, or the flow at each inflow and overflowing point are
known, yet the head losses are definitely not. This last case is investigated straightaway.
The system incorporates making a guess with respect to the flow to rate in each pipe, taking
consideration of making a guess to such an extent that the total flow into any crossing point
approaches the total flow out of that convergence. By then the head loss in each pipe is found
out, in perspective of the normal flow and the picked flow versus head loss relationship. Next,
the system is checked whether the head loss around each loop is zero. Since the fundamental
flow was speculated, this will undoubtedly not be the circumstance. The flow rates are then
adjusted with the end goal that continuity will in any case be fulfilled at each crossing point,
aside from the head loss around each loop is more similar to be zero. This strategy is repeated
until the point that the progressions are attractively little. The definite procedure is according to
the following
Procedure
Divided the network into loops
For each loops done the fallowing steps
1. Assumptions on the flow, , flow course in the pipes, direction of flow in the loop where
positive will be taken to be clockwise or negative will be taken to be counterclockwise,
with an application of equation of continuity condition at every node. Evaluated pipe
flows are associated with iteration until head loss in the clockwise direction is equivalent
to the counterclockwise bearing in each loop.
2∗∑( h
Q )
Methodology
With respect to pipe network investigation, the conventionally approach is known as the Hardy
Cross procedure (Huang, Vairavamoorthy and Tsegaye, 2010). This strategy is appropriate if the
entire pipe sizes (lengths and breadths) are settled, and either the head losses between the outlets
and inlets are known yet the flow are not, or the flow at each inflow and overflowing point are
known, yet the head losses are definitely not. This last case is investigated straightaway.
The system incorporates making a guess with respect to the flow to rate in each pipe, taking
consideration of making a guess to such an extent that the total flow into any crossing point
approaches the total flow out of that convergence. By then the head loss in each pipe is found
out, in perspective of the normal flow and the picked flow versus head loss relationship. Next,
the system is checked whether the head loss around each loop is zero. Since the fundamental
flow was speculated, this will undoubtedly not be the circumstance. The flow rates are then
adjusted with the end goal that continuity will in any case be fulfilled at each crossing point,
aside from the head loss around each loop is more similar to be zero. This strategy is repeated
until the point that the progressions are attractively little. The definite procedure is according to
the following
Procedure
Divided the network into loops
For each loops done the fallowing steps
1. Assumptions on the flow, , flow course in the pipes, direction of flow in the loop where
positive will be taken to be clockwise or negative will be taken to be counterclockwise,
with an application of equation of continuity condition at every node. Evaluated pipe
flows are associated with iteration until head loss in the clockwise direction is equivalent
to the counterclockwise bearing in each loop.
2. The equivalent resistance K for each pipe will be required to be calculated based on the
given parameters on the demand for each node, similarly pipe length and diameter,
together with temperature and finally pipe material are expected to be unique if not it is
assumed to be equal.
3. Calculation of hf = k*𝑄n for each pipe. The sign from procedure 1 is retained and
computation is done for the sum of the loops hf
4. Computation of hf ⁄Q for each and every pipe and the summation for each and every loop
that is∑ ¿hf ⁄𝑄|.
5. Calculation of the correction by the fallowing formula ∆𝑄 = − ∑ h f/( 𝑛∑ |hf ⁄𝑄 |
6. Application of correction to Qnew = Q+ΔQ
7. Repeat procedure (3) to (6) until Δh become very small.
8. Finally solving of the total pressure at each and every node using energy method
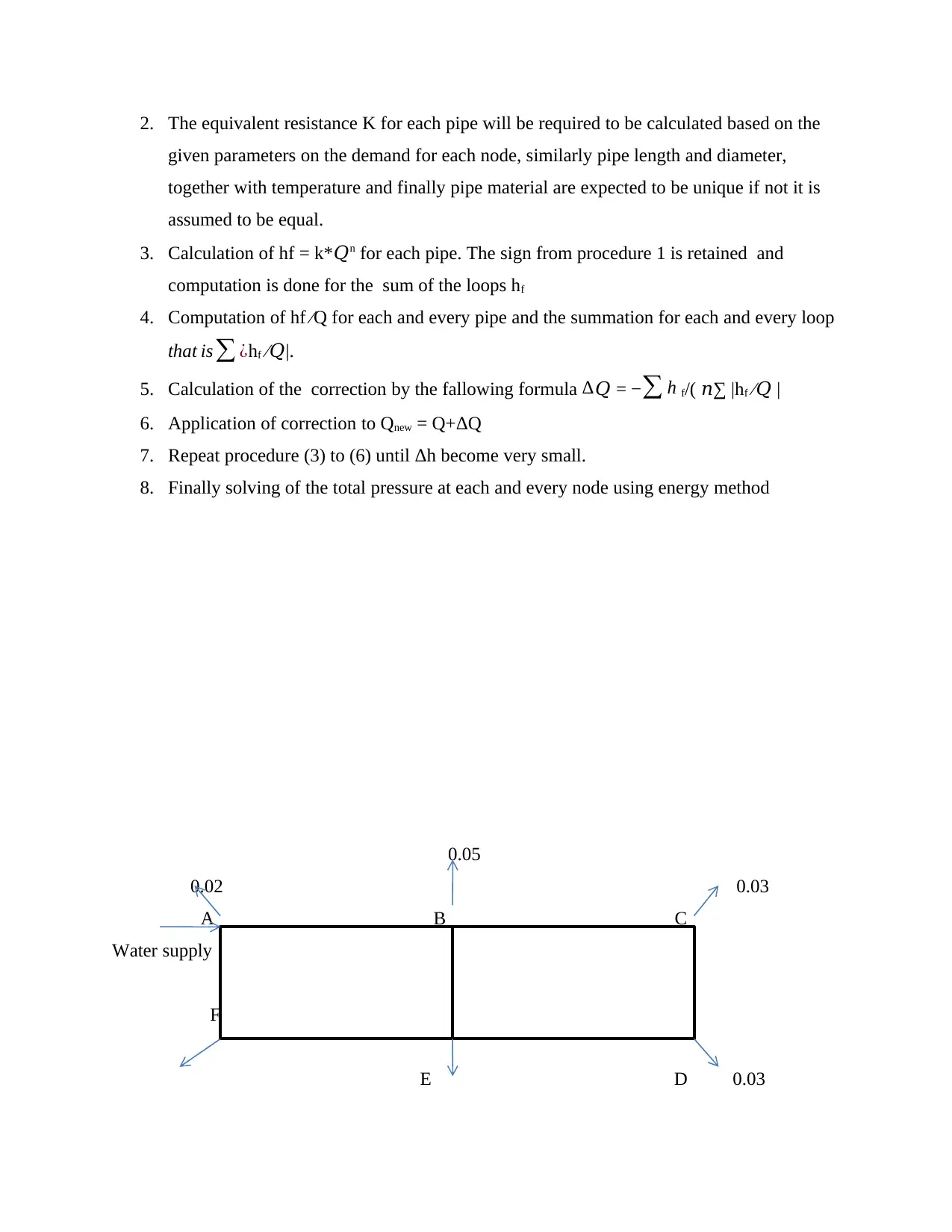
0.05
0.02 0.03
A B C
Water supply
F
E D 0.03
given parameters on the demand for each node, similarly pipe length and diameter,
together with temperature and finally pipe material are expected to be unique if not it is
assumed to be equal.
3. Calculation of hf = k*𝑄n for each pipe. The sign from procedure 1 is retained and
computation is done for the sum of the loops hf
4. Computation of hf ⁄Q for each and every pipe and the summation for each and every loop
that is∑ ¿hf ⁄𝑄|.
5. Calculation of the correction by the fallowing formula ∆𝑄 = − ∑ h f/( 𝑛∑ |hf ⁄𝑄 |
6. Application of correction to Qnew = Q+ΔQ
7. Repeat procedure (3) to (6) until Δh become very small.
8. Finally solving of the total pressure at each and every node using energy method
0.05
0.02 0.03
A B C
Water supply
F
E D 0.03
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
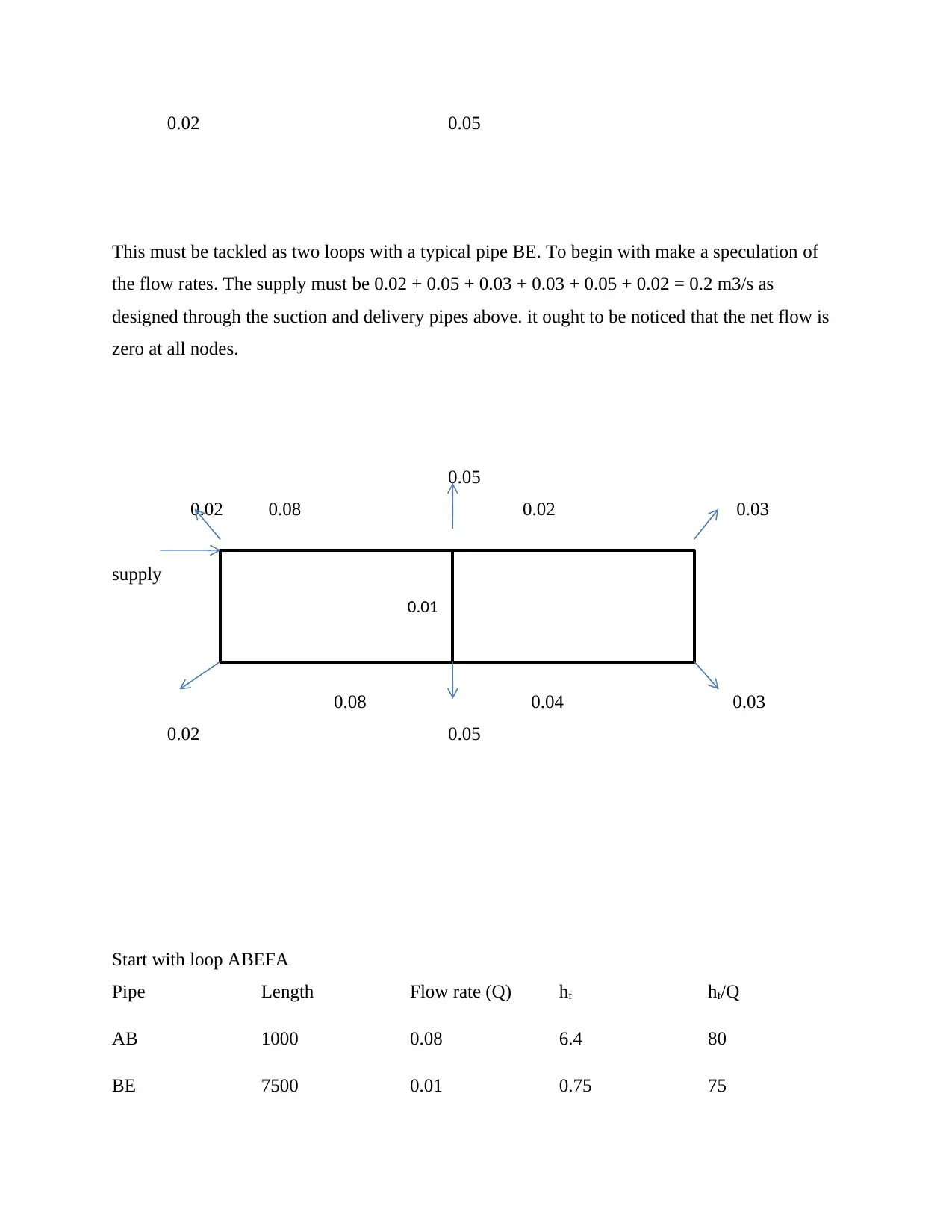
0.02 0.05
This must be tackled as two loops with a typical pipe BE. To begin with make a speculation of
the flow rates. The supply must be 0.02 + 0.05 + 0.03 + 0.03 + 0.05 + 0.02 = 0.2 m3/s as
designed through the suction and delivery pipes above. it ought to be noticed that the net flow is
zero at all nodes.
0.05
0.02 0.08 0.02 0.03
supply
0.08 0.04 0.03
0.02 0.05
Start with loop ABEFA
Pipe Length Flow rate (Q) hf hf/Q
AB 1000 0.08 6.4 80
BE 7500 0.01 0.75 75
0.01
This must be tackled as two loops with a typical pipe BE. To begin with make a speculation of
the flow rates. The supply must be 0.02 + 0.05 + 0.03 + 0.03 + 0.05 + 0.02 = 0.2 m3/s as
designed through the suction and delivery pipes above. it ought to be noticed that the net flow is
zero at all nodes.
0.05
0.02 0.08 0.02 0.03
supply
0.08 0.04 0.03
0.02 0.05
Start with loop ABEFA
Pipe Length Flow rate (Q) hf hf/Q
AB 1000 0.08 6.4 80
BE 7500 0.01 0.75 75
0.01
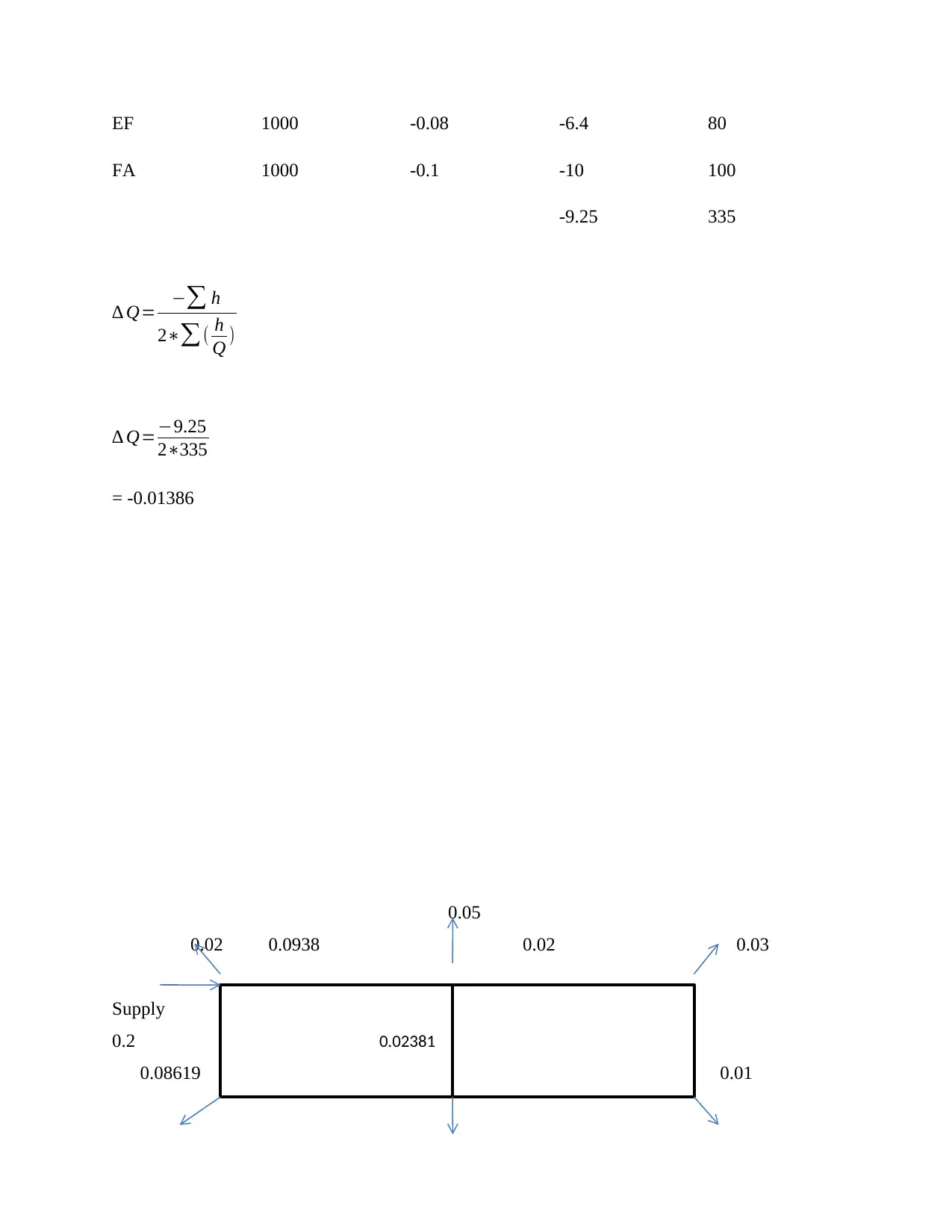
EF 1000 -0.08 -6.4 80
FA 1000 -0.1 -10 100
-9.25 335
∆ Q= −∑ h
2∗∑( h
Q )
∆ Q=−9.25
2∗335
= -0.01386
0.05
0.02 0.0938 0.02 0.03
Supply
0.2
0.08619 0.01
0.02381
FA 1000 -0.1 -10 100
-9.25 335
∆ Q= −∑ h
2∗∑( h
Q )
∆ Q=−9.25
2∗335
= -0.01386
0.05
0.02 0.0938 0.02 0.03
Supply
0.2
0.08619 0.01
0.02381
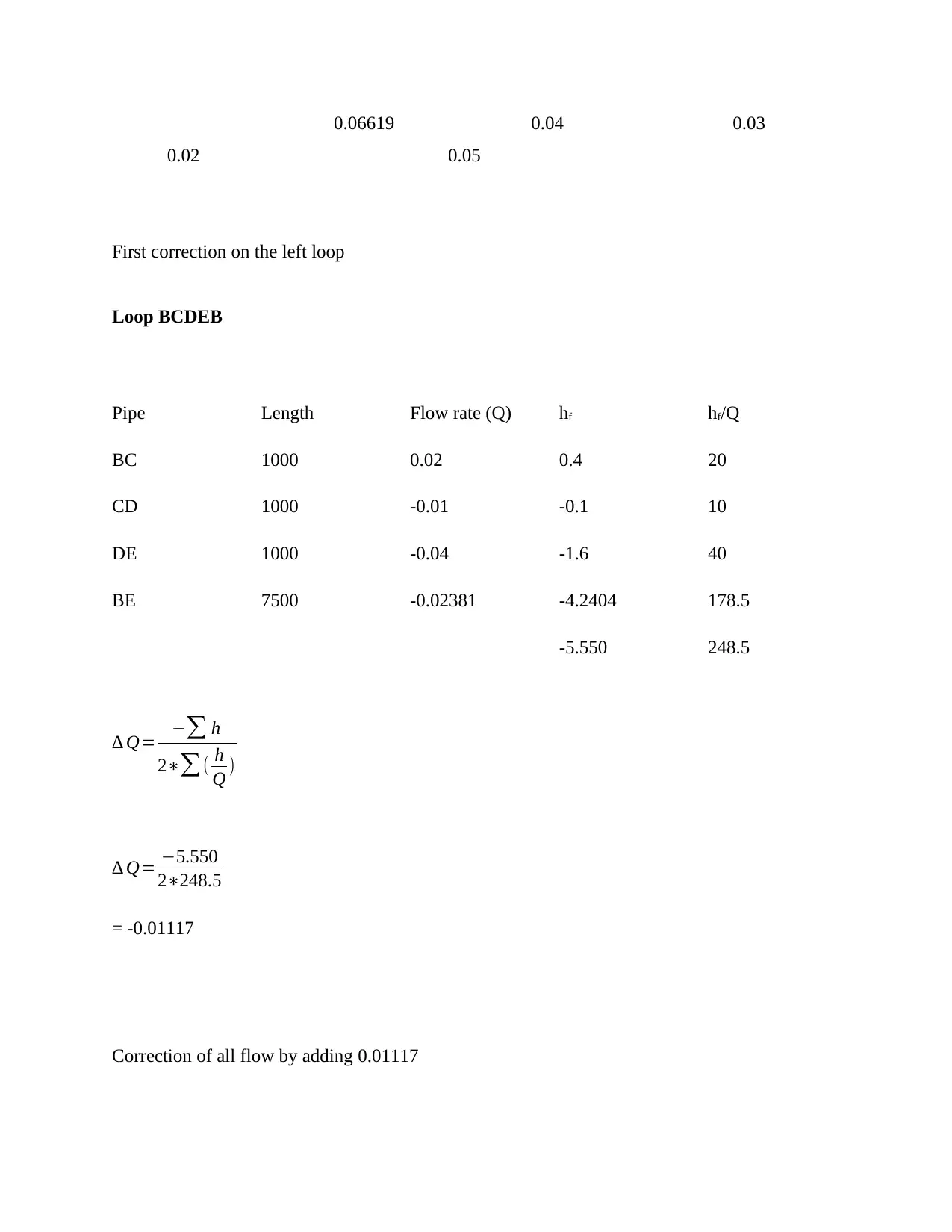
0.06619 0.04 0.03
0.02 0.05
First correction on the left loop
Loop BCDEB
Pipe Length Flow rate (Q) hf hf/Q
BC 1000 0.02 0.4 20
CD 1000 -0.01 -0.1 10
DE 1000 -0.04 -1.6 40
BE 7500 -0.02381 -4.2404 178.5
-5.550 248.5
∆ Q= −∑ h
2∗∑( h
Q )
∆ Q= −5.550
2∗248.5
= -0.01117
Correction of all flow by adding 0.01117
0.02 0.05
First correction on the left loop
Loop BCDEB
Pipe Length Flow rate (Q) hf hf/Q
BC 1000 0.02 0.4 20
CD 1000 -0.01 -0.1 10
DE 1000 -0.04 -1.6 40
BE 7500 -0.02381 -4.2404 178.5
-5.550 248.5
∆ Q= −∑ h
2∗∑( h
Q )
∆ Q= −5.550
2∗248.5
= -0.01117
Correction of all flow by adding 0.01117
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
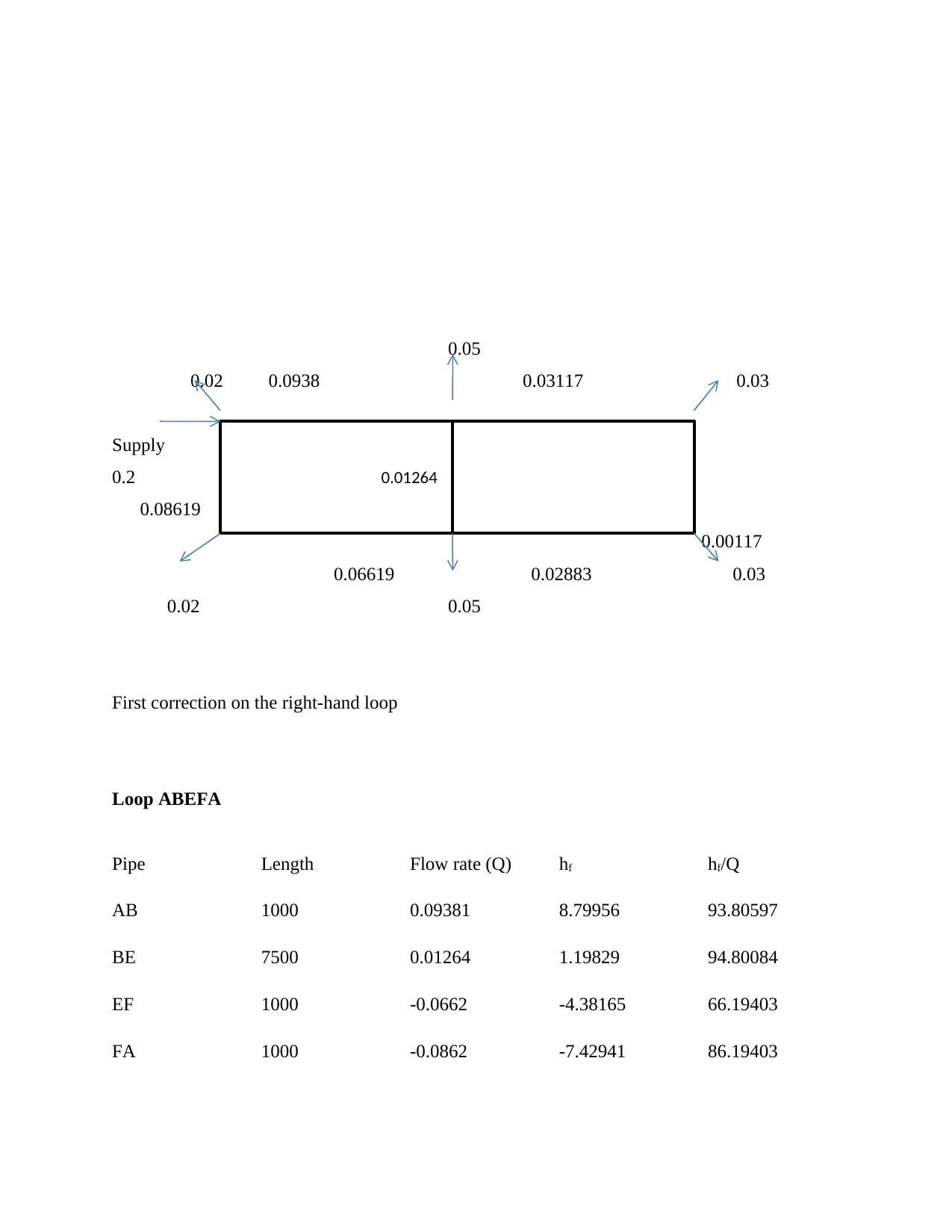
0.05
0.02 0.0938 0.03117 0.03
Supply
0.2
0.08619
0.00117
0.06619 0.02883 0.03
0.02 0.05
First correction on the right-hand loop
Loop ABEFA
Pipe Length Flow rate (Q) hf hf/Q
AB 1000 0.09381 8.79956 93.80597
BE 7500 0.01264 1.19829 94.80084
EF 1000 -0.0662 -4.38165 66.19403
FA 1000 -0.0862 -7.42941 86.19403
0.01264
0.02 0.0938 0.03117 0.03
Supply
0.2
0.08619
0.00117
0.06619 0.02883 0.03
0.02 0.05
First correction on the right-hand loop
Loop ABEFA
Pipe Length Flow rate (Q) hf hf/Q
AB 1000 0.09381 8.79956 93.80597
BE 7500 0.01264 1.19829 94.80084
EF 1000 -0.0662 -4.38165 66.19403
FA 1000 -0.0862 -7.42941 86.19403
0.01264
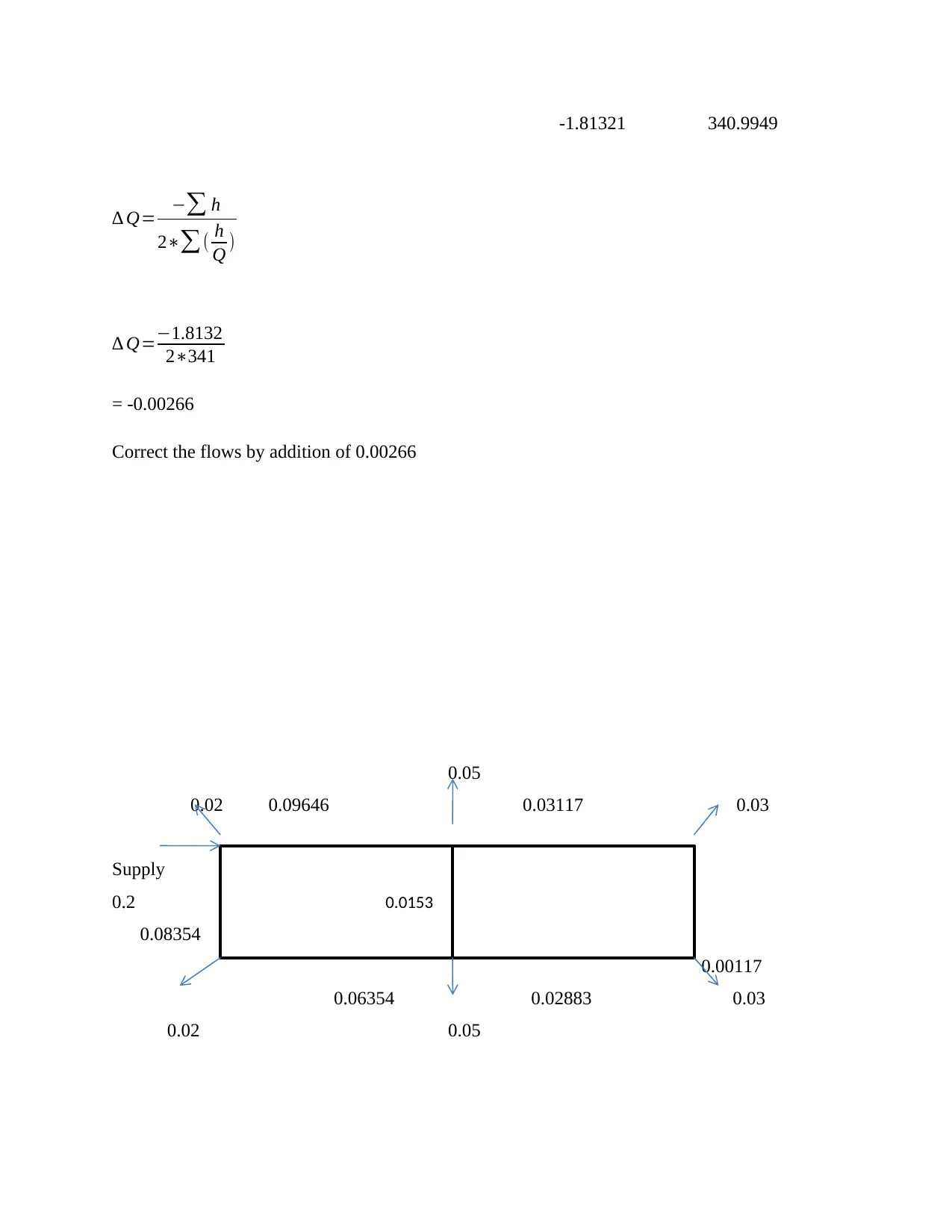
-1.81321 340.9949
∆ Q= −∑ h
2∗∑( h
Q )
∆ Q=−1.8132
2∗341
= -0.00266
Correct the flows by addition of 0.00266
0.05
0.02 0.09646 0.03117 0.03
Supply
0.2
0.08354
0.00117
0.06354 0.02883 0.03
0.02 0.05
0.0153
∆ Q= −∑ h
2∗∑( h
Q )
∆ Q=−1.8132
2∗341
= -0.00266
Correct the flows by addition of 0.00266
0.05
0.02 0.09646 0.03117 0.03
Supply
0.2
0.08354
0.00117
0.06354 0.02883 0.03
0.02 0.05
0.0153
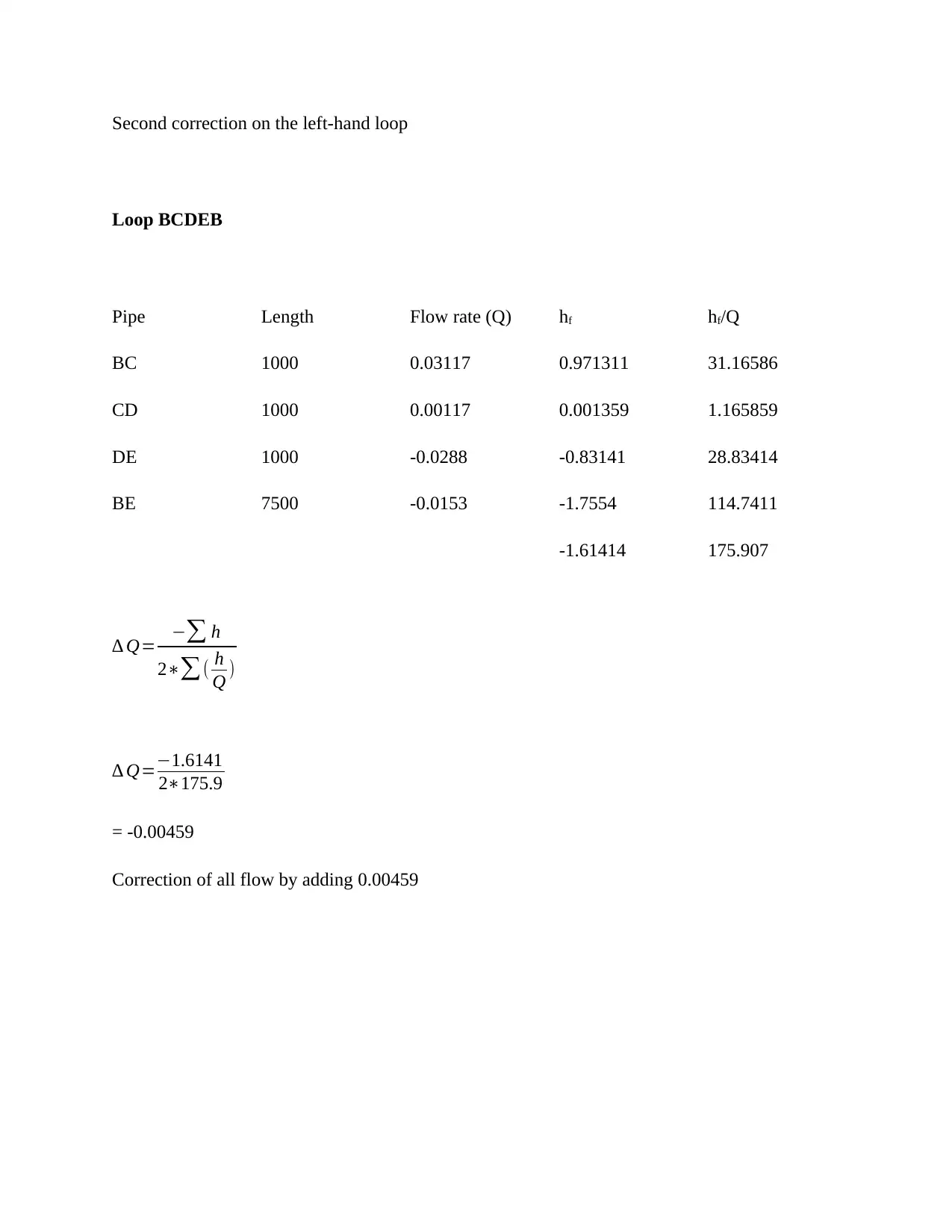
Second correction on the left-hand loop
Loop BCDEB
Pipe Length Flow rate (Q) hf hf/Q
BC 1000 0.03117 0.971311 31.16586
CD 1000 0.00117 0.001359 1.165859
DE 1000 -0.0288 -0.83141 28.83414
BE 7500 -0.0153 -1.7554 114.7411
-1.61414 175.907
∆ Q= −∑ h
2∗∑( h
Q )
∆ Q=−1.6141
2∗175.9
= -0.00459
Correction of all flow by adding 0.00459
Loop BCDEB
Pipe Length Flow rate (Q) hf hf/Q
BC 1000 0.03117 0.971311 31.16586
CD 1000 0.00117 0.001359 1.165859
DE 1000 -0.0288 -0.83141 28.83414
BE 7500 -0.0153 -1.7554 114.7411
-1.61414 175.907
∆ Q= −∑ h
2∗∑( h
Q )
∆ Q=−1.6141
2∗175.9
= -0.00459
Correction of all flow by adding 0.00459
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
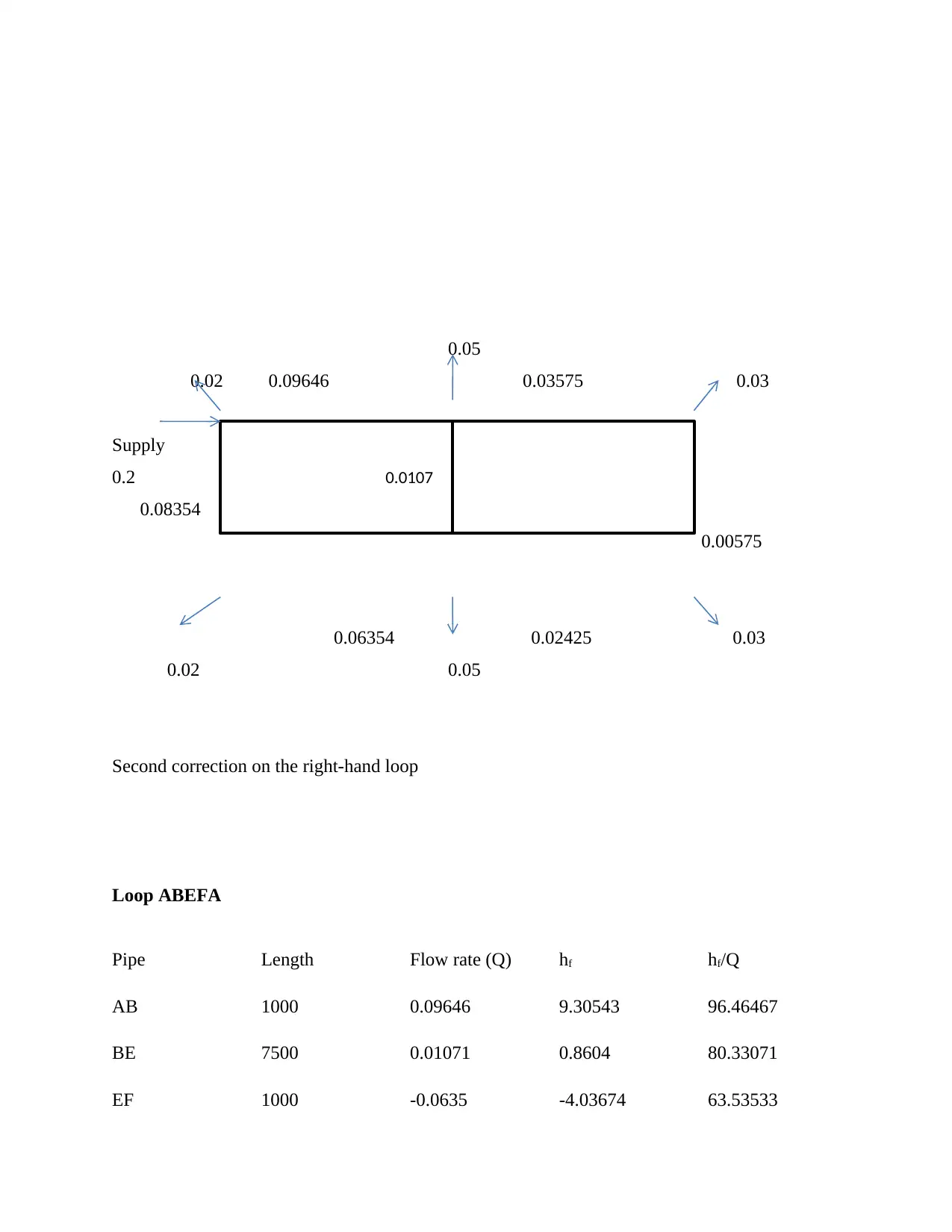
0.05
0.02 0.09646 0.03575 0.03
Supply
0.2
0.08354
0.00575
0.06354 0.02425 0.03
0.02 0.05
Second correction on the right-hand loop
Loop ABEFA
Pipe Length Flow rate (Q) hf hf/Q
AB 1000 0.09646 9.30543 96.46467
BE 7500 0.01071 0.8604 80.33071
EF 1000 -0.0635 -4.03674 63.53533
0.0107
0.02 0.09646 0.03575 0.03
Supply
0.2
0.08354
0.00575
0.06354 0.02425 0.03
0.02 0.05
Second correction on the right-hand loop
Loop ABEFA
Pipe Length Flow rate (Q) hf hf/Q
AB 1000 0.09646 9.30543 96.46467
BE 7500 0.01071 0.8604 80.33071
EF 1000 -0.0635 -4.03674 63.53533
0.0107
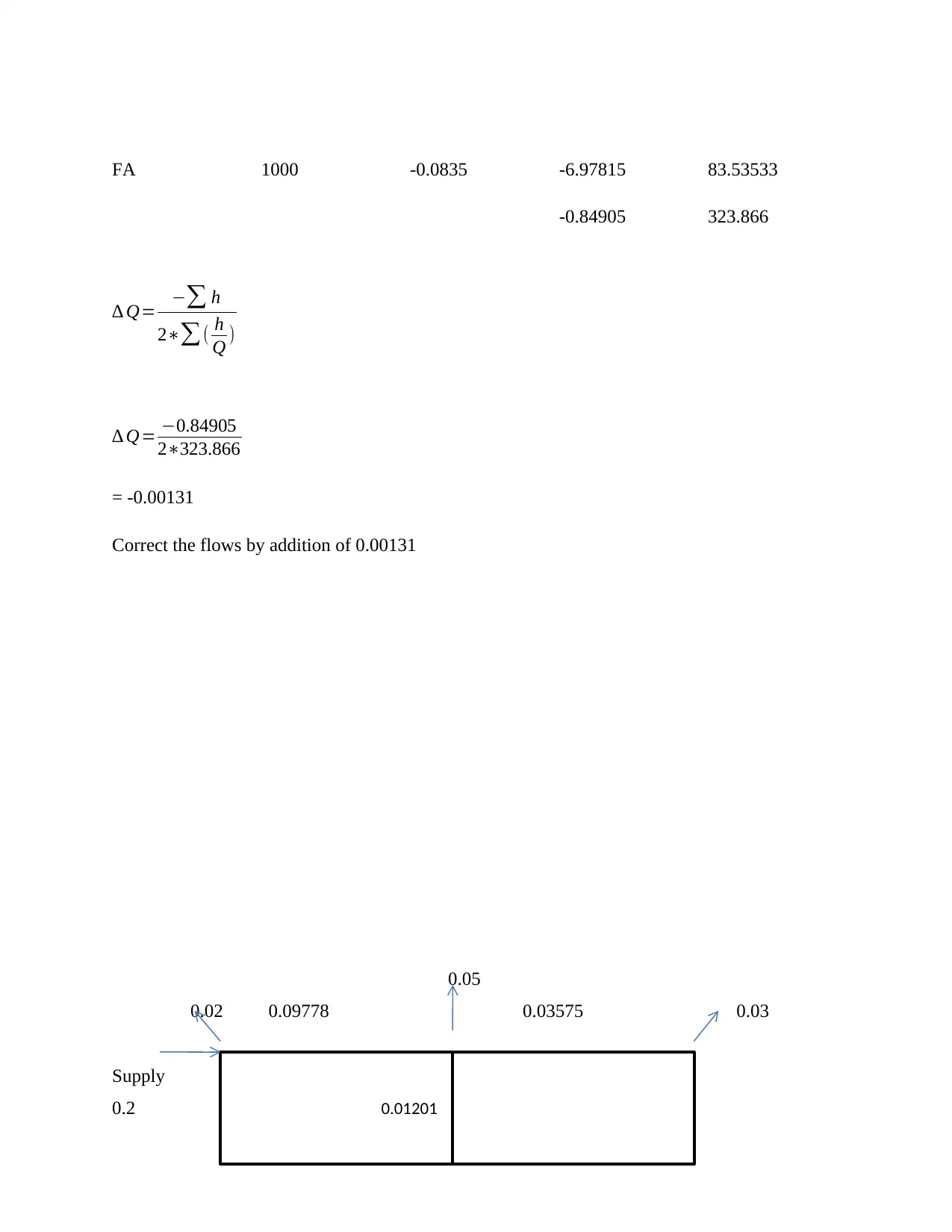
FA 1000 -0.0835 -6.97815 83.53533
-0.84905 323.866
∆ Q= −∑ h
2∗∑( h
Q )
∆ Q= −0.84905
2∗323.866
= -0.00131
Correct the flows by addition of 0.00131
0.05
0.02 0.09778 0.03575 0.03
Supply
0.2 0.01201
-0.84905 323.866
∆ Q= −∑ h
2∗∑( h
Q )
∆ Q= −0.84905
2∗323.866
= -0.00131
Correct the flows by addition of 0.00131
0.05
0.02 0.09778 0.03575 0.03
Supply
0.2 0.01201
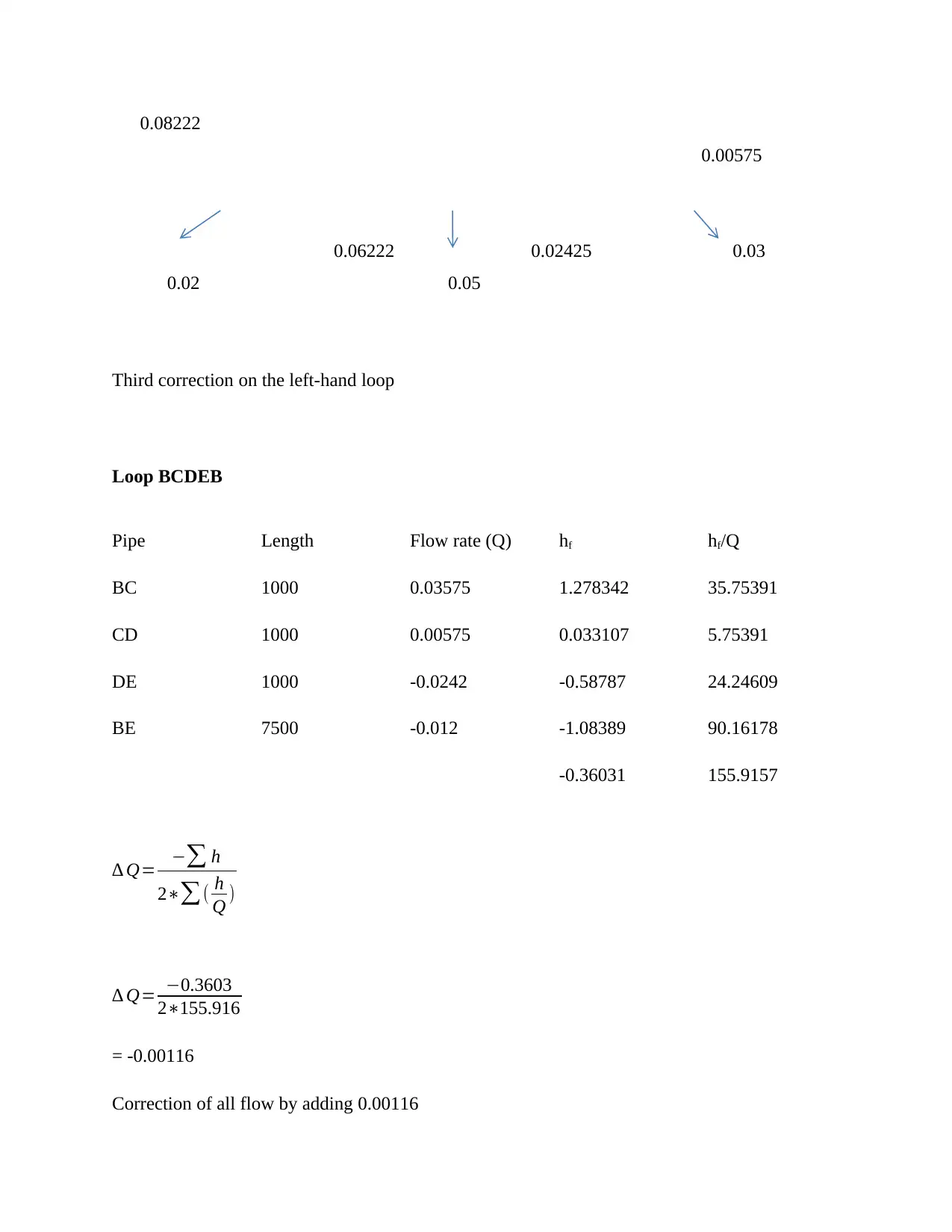
0.08222
0.00575
0.06222 0.02425 0.03
0.02 0.05
Third correction on the left-hand loop
Loop BCDEB
Pipe Length Flow rate (Q) hf hf/Q
BC 1000 0.03575 1.278342 35.75391
CD 1000 0.00575 0.033107 5.75391
DE 1000 -0.0242 -0.58787 24.24609
BE 7500 -0.012 -1.08389 90.16178
-0.36031 155.9157
∆ Q= −∑ h
2∗∑( h
Q )
∆ Q= −0.3603
2∗155.916
= -0.00116
Correction of all flow by adding 0.00116
0.00575
0.06222 0.02425 0.03
0.02 0.05
Third correction on the left-hand loop
Loop BCDEB
Pipe Length Flow rate (Q) hf hf/Q
BC 1000 0.03575 1.278342 35.75391
CD 1000 0.00575 0.033107 5.75391
DE 1000 -0.0242 -0.58787 24.24609
BE 7500 -0.012 -1.08389 90.16178
-0.36031 155.9157
∆ Q= −∑ h
2∗∑( h
Q )
∆ Q= −0.3603
2∗155.916
= -0.00116
Correction of all flow by adding 0.00116
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
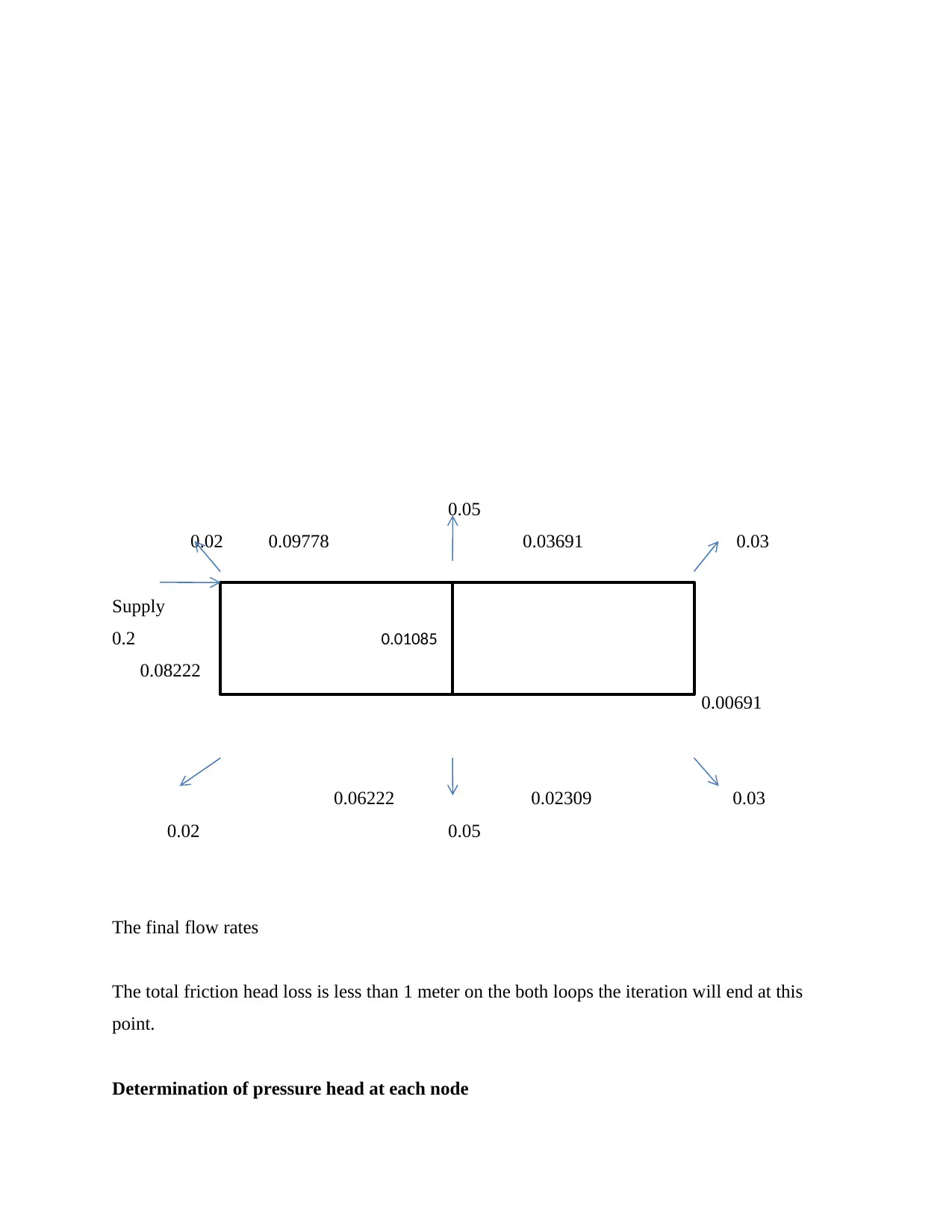
0.05
0.02 0.09778 0.03691 0.03
Supply
0.2
0.08222
0.00691
0.06222 0.02309 0.03
0.02 0.05
The final flow rates
The total friction head loss is less than 1 meter on the both loops the iteration will end at this
point.
Determination of pressure head at each node
0.01085
0.02 0.09778 0.03691 0.03
Supply
0.2
0.08222
0.00691
0.06222 0.02309 0.03
0.02 0.05
The final flow rates
The total friction head loss is less than 1 meter on the both loops the iteration will end at this
point.
Determination of pressure head at each node
0.01085
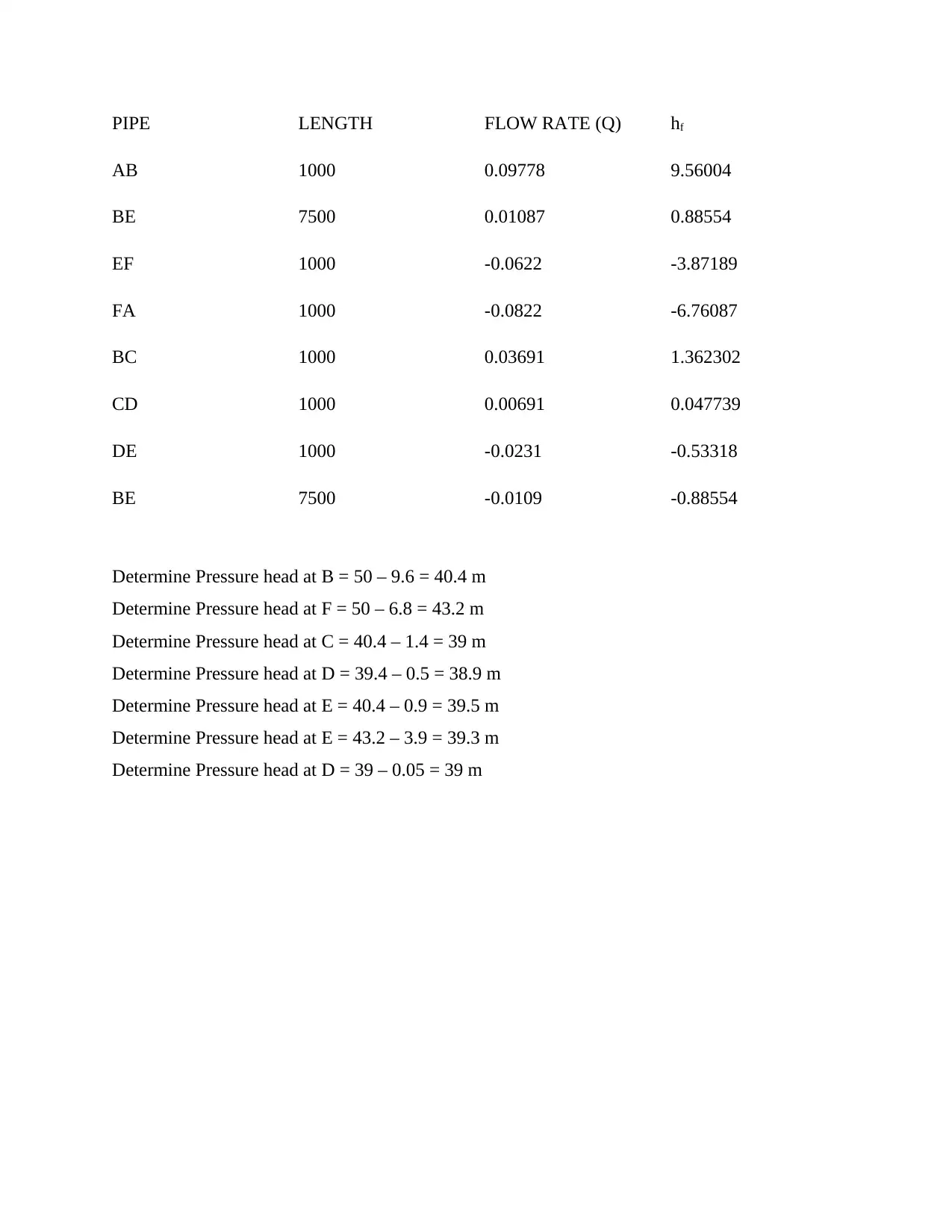
PIPE LENGTH FLOW RATE (Q) hf
AB 1000 0.09778 9.56004
BE 7500 0.01087 0.88554
EF 1000 -0.0622 -3.87189
FA 1000 -0.0822 -6.76087
BC 1000 0.03691 1.362302
CD 1000 0.00691 0.047739
DE 1000 -0.0231 -0.53318
BE 7500 -0.0109 -0.88554
Determine Pressure head at B = 50 – 9.6 = 40.4 m
Determine Pressure head at F = 50 – 6.8 = 43.2 m
Determine Pressure head at C = 40.4 – 1.4 = 39 m
Determine Pressure head at D = 39.4 – 0.5 = 38.9 m
Determine Pressure head at E = 40.4 – 0.9 = 39.5 m
Determine Pressure head at E = 43.2 – 3.9 = 39.3 m
Determine Pressure head at D = 39 – 0.05 = 39 m
AB 1000 0.09778 9.56004
BE 7500 0.01087 0.88554
EF 1000 -0.0622 -3.87189
FA 1000 -0.0822 -6.76087
BC 1000 0.03691 1.362302
CD 1000 0.00691 0.047739
DE 1000 -0.0231 -0.53318
BE 7500 -0.0109 -0.88554
Determine Pressure head at B = 50 – 9.6 = 40.4 m
Determine Pressure head at F = 50 – 6.8 = 43.2 m
Determine Pressure head at C = 40.4 – 1.4 = 39 m
Determine Pressure head at D = 39.4 – 0.5 = 38.9 m
Determine Pressure head at E = 40.4 – 0.9 = 39.5 m
Determine Pressure head at E = 43.2 – 3.9 = 39.3 m
Determine Pressure head at D = 39 – 0.05 = 39 m

PART B
DESIGN A SANITARY SEWER SYSTEM
Sewers are designed taking consideration of 20 years.
Assumptions
Population in the initial years of the design period are low compared to the design population at
the end of design period
Peak flow rate in the initial years is low compared to the designed peak flow rate
Sizing should be such that it will attain the self-cleansing velocity at the average flow rate or at
least at the maximum flow rate at the beginning of the design period.
For Partially-full flow v is not influenced by the diameter of the pipe, rather is much influenced
by the slope of the channel
Sewer line design considerations
Average sewer flow is calculated based on consumption and population
Average sewage flow Q = 0.8 * consumption
Qdesign = 2*peak factor * Q + infiltration (10%) + storm water (100% of peak flow)
DESIGN A SANITARY SEWER SYSTEM
Sewers are designed taking consideration of 20 years.
Assumptions
Population in the initial years of the design period are low compared to the design population at
the end of design period
Peak flow rate in the initial years is low compared to the designed peak flow rate
Sizing should be such that it will attain the self-cleansing velocity at the average flow rate or at
least at the maximum flow rate at the beginning of the design period.
For Partially-full flow v is not influenced by the diameter of the pipe, rather is much influenced
by the slope of the channel
Sewer line design considerations
Average sewer flow is calculated based on consumption and population
Average sewage flow Q = 0.8 * consumption
Qdesign = 2*peak factor * Q + infiltration (10%) + storm water (100% of peak flow)
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

Design equation using Manning`s formula (Vongvisessomjai, Tingsanchali and Babel, 2010) for
sewage flowing under gravity
V = 1
n∗¿ R2/3 * S1/2
Where,
V = velocity of flow in m/sec
R = hydraulic mean depth
S = slope of the sewer
n = coefficient of roughness for pipes (n = 0.013 for RCC pipes)
Cleansing velocity => for partially combined sewer = 0.7 m/sec
Maximum velocity used should not be greater than 2.4 m/sec, to avoid abrasion
Minimum sewer size to be used should not be less than 225 mm to avoid chocking of sewer with
bigger size objects through the man hole
Minimum cover to be used = 1 m to avoid damage by live loads on sewer
Design procedure
1. Determination of present population of projected area
2. Drawing of the system layout while considering the streets and road layout.
3. Identification of the sewer line and numbering of the manhole.
4. Allocate plots to each sewer line
5. Measurement of the sewer line length as per scale of the map provided
6. Adopt the per capita sewage flow as 70% of water consumption and calculate the average
sewage flow and infiltration for all the sewer line., at this point take infiltration as 10% of
the average sewage flow
7. Calculation of peak sewage flow and design flow for the sewer lines (Hvitved-Jacobsen,
Vollertsen, and Nielsen, 2010)
sewage flowing under gravity
V = 1
n∗¿ R2/3 * S1/2
Where,
V = velocity of flow in m/sec
R = hydraulic mean depth
S = slope of the sewer
n = coefficient of roughness for pipes (n = 0.013 for RCC pipes)
Cleansing velocity => for partially combined sewer = 0.7 m/sec
Maximum velocity used should not be greater than 2.4 m/sec, to avoid abrasion
Minimum sewer size to be used should not be less than 225 mm to avoid chocking of sewer with
bigger size objects through the man hole
Minimum cover to be used = 1 m to avoid damage by live loads on sewer
Design procedure
1. Determination of present population of projected area
2. Drawing of the system layout while considering the streets and road layout.
3. Identification of the sewer line and numbering of the manhole.
4. Allocate plots to each sewer line
5. Measurement of the sewer line length as per scale of the map provided
6. Adopt the per capita sewage flow as 70% of water consumption and calculate the average
sewage flow and infiltration for all the sewer line., at this point take infiltration as 10% of
the average sewage flow
7. Calculation of peak sewage flow and design flow for the sewer lines (Hvitved-Jacobsen,
Vollertsen, and Nielsen, 2010)

8. By the usage of back calculation determine the appropriate diagram and sewer in
assumption that the sewer is fully flowing
9. In the end find the invert levels for all the sewer
10. Draw the profile for all sewer line
Data for design
Present year 2018 Design period 2038
Plots 7 10
Apartments 400 600
Flats 200 400
Assume the number of plots in the area = 280
Assume the number of apartment in the area = 3
Number of flats in the area = 3
Take the design period to be = 20 years
Present population = 7 * 28 + 400 * 3 + 200 * = 3767
Annual population growth rate for Australia = 1.98%
Population density in 2038 Pd = pp(1 + 1.98%) – 20 = 3822
Pd = 10*281 + 600 * 3 + 400 * 3 = 5810
Per capita water consumption = 300 lpc + 103 plc = 403 plc
assumption that the sewer is fully flowing
9. In the end find the invert levels for all the sewer
10. Draw the profile for all sewer line
Data for design
Present year 2018 Design period 2038
Plots 7 10
Apartments 400 600
Flats 200 400
Assume the number of plots in the area = 280
Assume the number of apartment in the area = 3
Number of flats in the area = 3
Take the design period to be = 20 years
Present population = 7 * 28 + 400 * 3 + 200 * = 3767
Annual population growth rate for Australia = 1.98%
Population density in 2038 Pd = pp(1 + 1.98%) – 20 = 3822
Pd = 10*281 + 600 * 3 + 400 * 3 = 5810
Per capita water consumption = 300 lpc + 103 plc = 403 plc

Average sewer flow = pd * pcwc * 0.8/1000
= 5810 * 403 * 0.8/1000 = 1873 m3/day
= 0.0217 m3/s
Peak factor = 4
A = π∗¿(D/4)2
V = 1/N * R2/3 * S1/2
R = A/P = D/4
Q = A*V
Q=
π D2
4 ∗1
n ( D
4 )2
3∗S
1
2
Q = 0.0217 M3/S
S =0.001
N = 0.015
0.0217=
π D2
4 ∗1
0.015 ( D
4 ) 2
3∗0.001
1
2
D8/5 = 0.033022
D = 0.004267 M
D = 0.4 (TAKE)
V = Q/A
V = 0.0217/A
V = 1517 M/S which is greater than 0.6 m/s
= 5810 * 403 * 0.8/1000 = 1873 m3/day
= 0.0217 m3/s
Peak factor = 4
A = π∗¿(D/4)2
V = 1/N * R2/3 * S1/2
R = A/P = D/4
Q = A*V
Q=
π D2
4 ∗1
n ( D
4 )2
3∗S
1
2
Q = 0.0217 M3/S
S =0.001
N = 0.015
0.0217=
π D2
4 ∗1
0.015 ( D
4 ) 2
3∗0.001
1
2
D8/5 = 0.033022
D = 0.004267 M
D = 0.4 (TAKE)
V = Q/A
V = 0.0217/A
V = 1517 M/S which is greater than 0.6 m/s
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

Determination of manhole distance and pipe slope
Procedure
1. Demonstrate the inverted height on profile for each pipe entering and leaving the sewer manhole at
within sewer manhole wall.
2. Since the design sewer pipe has a diameter of 0.004267 m and is less than 48 inch then the following
will be considered, the distance between center to center is 95 m
a) The pipe distance will be determined by subtracting one half inside the dimension of on which the
sewer manhole, for both manhole from the total distance between the centerline of both manholes.
In the event that we take the two sewer manhole are having a most extreme of 1.2 m measurement, at
that point the aggregate separation distance between the two sewer manhole with respect to centerline
to centerline is 95 m, and the separation distance between the centerline of the sewer manhole to the
inside wall of the sewer manhole is 0.6 m.
Since the two sewer manhole are a similar width, subtract 1.2 m from the aggregate separation distance
95 – 1.2= 93.5 m, the pipe remove between sewer manhole will be 93.5 m however a separation
distance of 95 m ought to be appeared on the profile.
b) To decide the pipe slope, subtract the two sewer manhole inverts and divide the difference by the
pipe distance and multiply by one hundred (100) to acquire the percent review of the pipe.
If the manhole invert elevations are 75 m as per the contours for one manhole and 55 for the other,
then the difference between the two manhole inverts will be 15.00 m
Take the invert difference 15 .00 m and divide it by the pipe distance (93.5 m). The pipe slope will be
0.16042feet per hundred feet or 16.04%. Show the pipe slope on the profile.
SANITARY SEWER LINE PROFILE
1ST MANHOLE 2ND MANHOLE
PIPE SIZE = 0.51 M
Procedure
1. Demonstrate the inverted height on profile for each pipe entering and leaving the sewer manhole at
within sewer manhole wall.
2. Since the design sewer pipe has a diameter of 0.004267 m and is less than 48 inch then the following
will be considered, the distance between center to center is 95 m
a) The pipe distance will be determined by subtracting one half inside the dimension of on which the
sewer manhole, for both manhole from the total distance between the centerline of both manholes.
In the event that we take the two sewer manhole are having a most extreme of 1.2 m measurement, at
that point the aggregate separation distance between the two sewer manhole with respect to centerline
to centerline is 95 m, and the separation distance between the centerline of the sewer manhole to the
inside wall of the sewer manhole is 0.6 m.
Since the two sewer manhole are a similar width, subtract 1.2 m from the aggregate separation distance
95 – 1.2= 93.5 m, the pipe remove between sewer manhole will be 93.5 m however a separation
distance of 95 m ought to be appeared on the profile.
b) To decide the pipe slope, subtract the two sewer manhole inverts and divide the difference by the
pipe distance and multiply by one hundred (100) to acquire the percent review of the pipe.
If the manhole invert elevations are 75 m as per the contours for one manhole and 55 for the other,
then the difference between the two manhole inverts will be 15.00 m
Take the invert difference 15 .00 m and divide it by the pipe distance (93.5 m). The pipe slope will be
0.16042feet per hundred feet or 16.04%. Show the pipe slope on the profile.
SANITARY SEWER LINE PROFILE
1ST MANHOLE 2ND MANHOLE
PIPE SIZE = 0.51 M

15 SLOPE = 16.04% SIZE OF THE MAN HOLE EXTREEMS= 1.2
93.5 M
95 M
Reference
Huang, D., Vairavamoorthy, K. and Tsegaye, S., 2010. Flexible design of urban water
distribution networks. In World Environmental and Water Resources Congress 2010: Challenges
of Change (pp. 4225-4236).
Hvitved-Jacobsen, T., Vollertsen, J. and Nielsen, A.H., 2010. Urban and highway stormwater
pollution: Concepts and engineering. CRC press..
Spiliotis, M. and Tsakiris, G., 2010. Water distribution system analysis: Newton-Raphson
method revisited. Journal of Hydraulic Engineering, 137(8), pp.852-855.
Kulworawanichpong, T., 2010. Simplified Newton–Raphson power-flow solution
method. International Journal of Electrical Power & Energy Systems, 32(6), pp.551-558.
Vongvisessomjai, N., Tingsanchali, T. and Babel, M.S., 2010. Non-deposition design criteria for
sewers with part-full flow. Urban Water Journal, 7(1), pp.61.
93.5 M
95 M
Reference
Huang, D., Vairavamoorthy, K. and Tsegaye, S., 2010. Flexible design of urban water
distribution networks. In World Environmental and Water Resources Congress 2010: Challenges
of Change (pp. 4225-4236).
Hvitved-Jacobsen, T., Vollertsen, J. and Nielsen, A.H., 2010. Urban and highway stormwater
pollution: Concepts and engineering. CRC press..
Spiliotis, M. and Tsakiris, G., 2010. Water distribution system analysis: Newton-Raphson
method revisited. Journal of Hydraulic Engineering, 137(8), pp.852-855.
Kulworawanichpong, T., 2010. Simplified Newton–Raphson power-flow solution
method. International Journal of Electrical Power & Energy Systems, 32(6), pp.551-558.
Vongvisessomjai, N., Tingsanchali, T. and Babel, M.S., 2010. Non-deposition design criteria for
sewers with part-full flow. Urban Water Journal, 7(1), pp.61.
1 out of 24
Related Documents
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.


