Stress Analysis and Strain Calculation

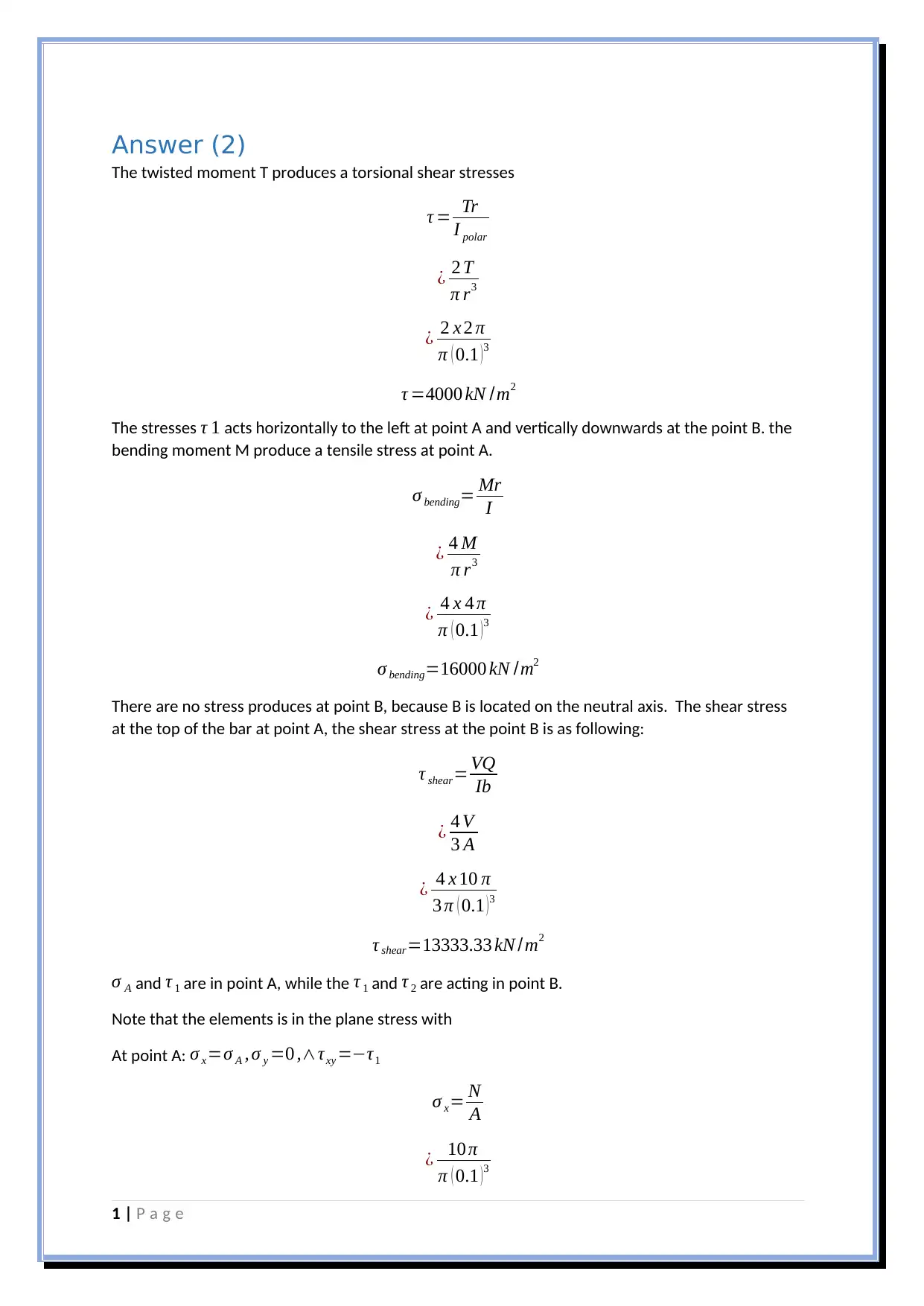
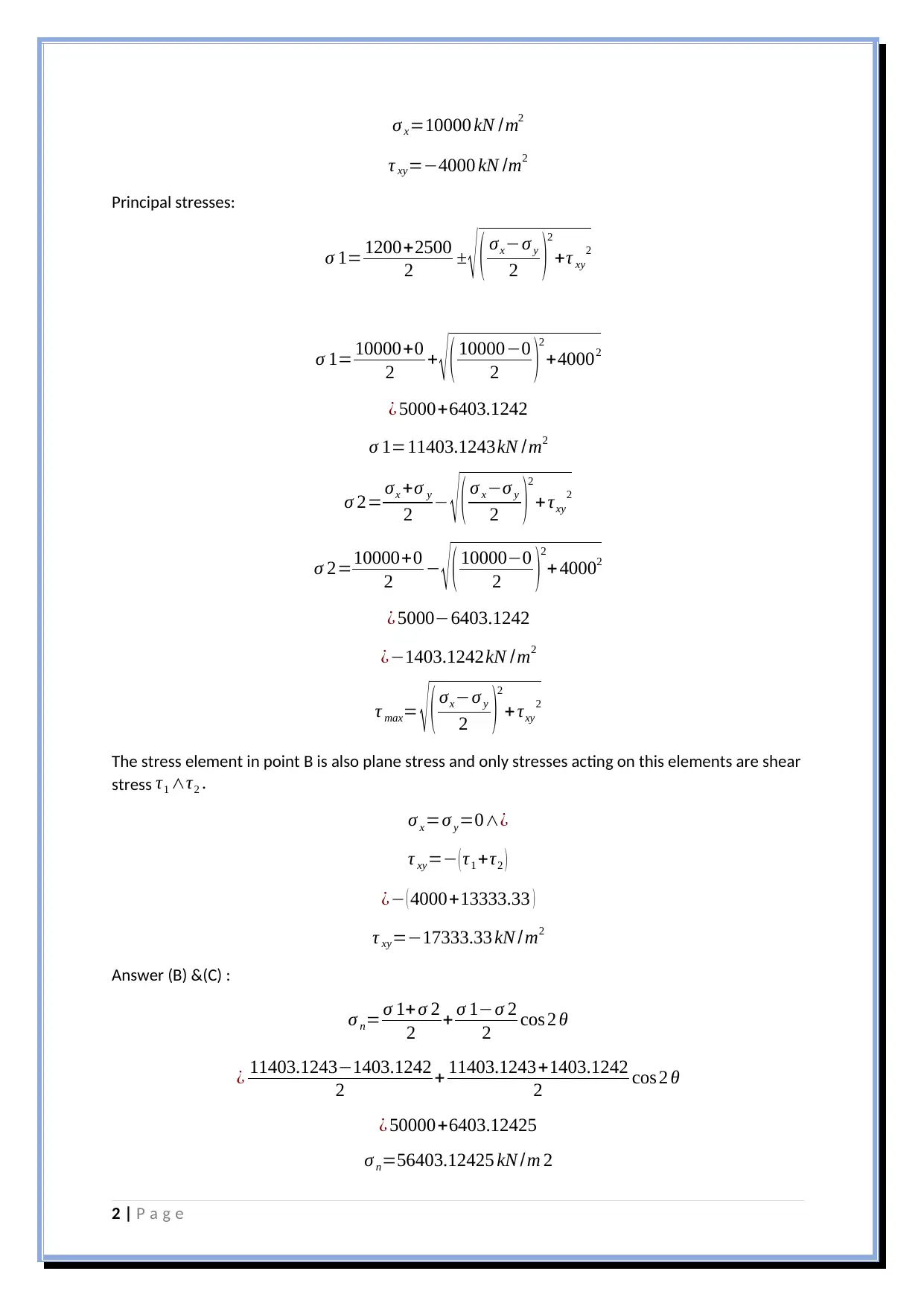
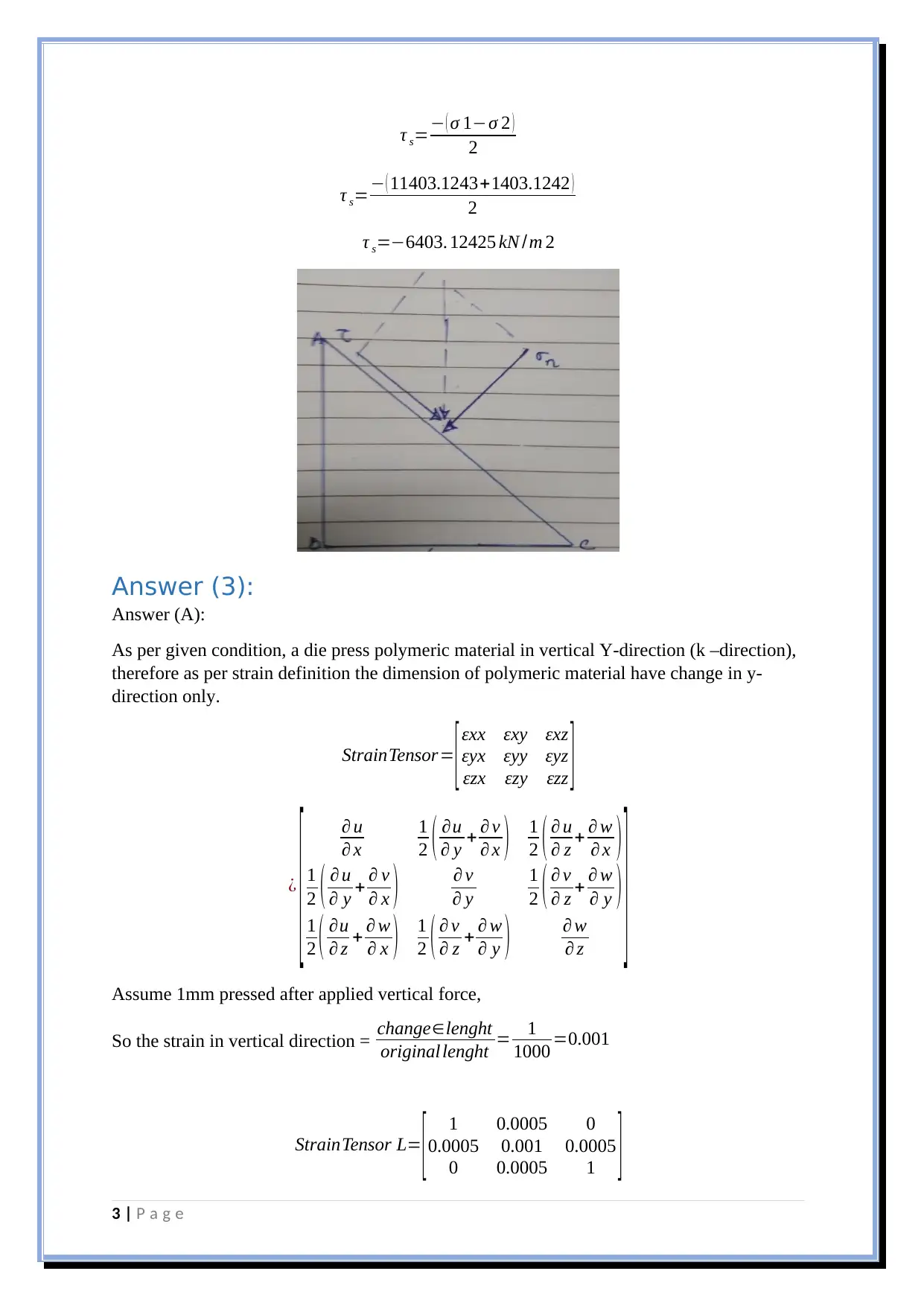
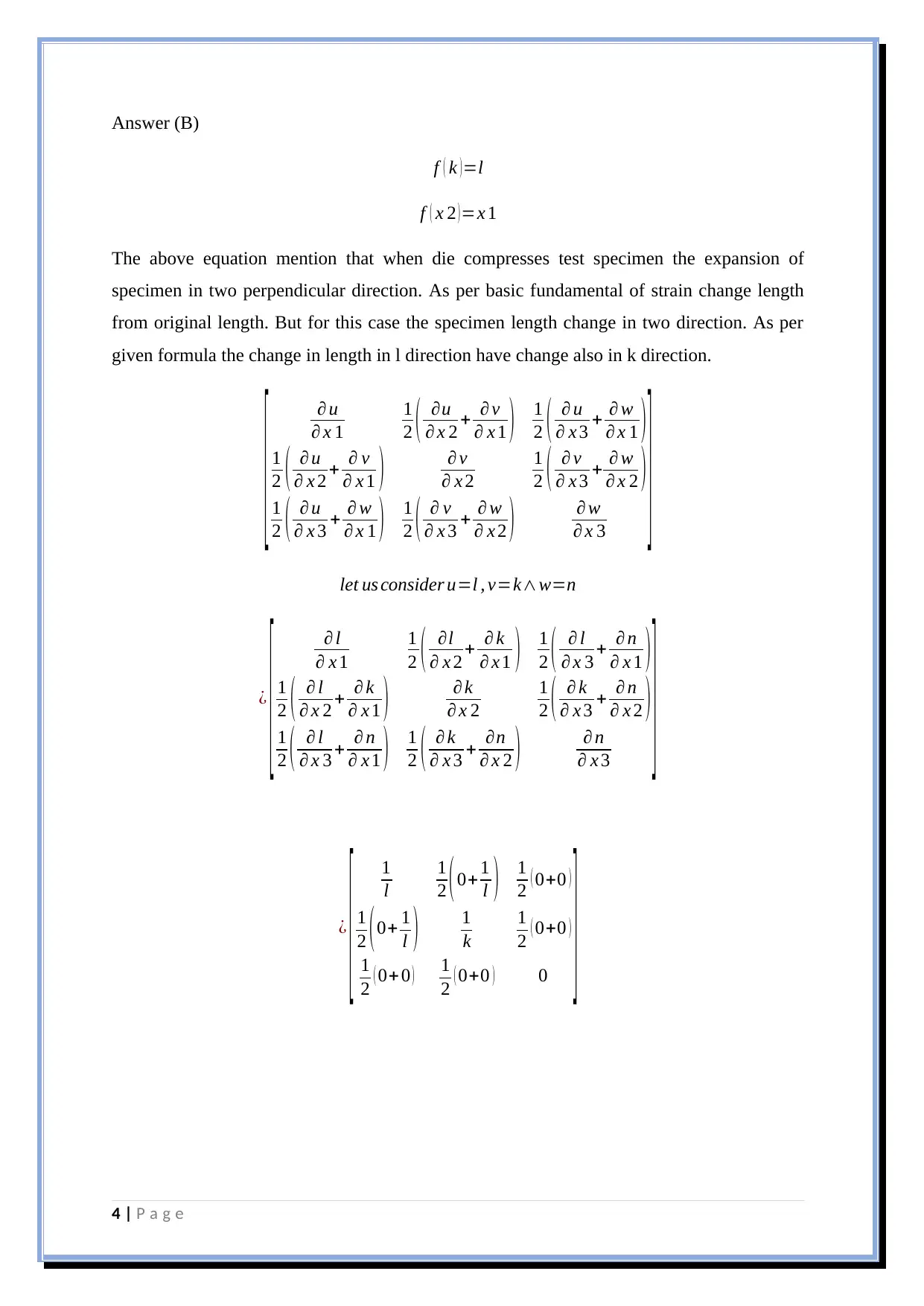
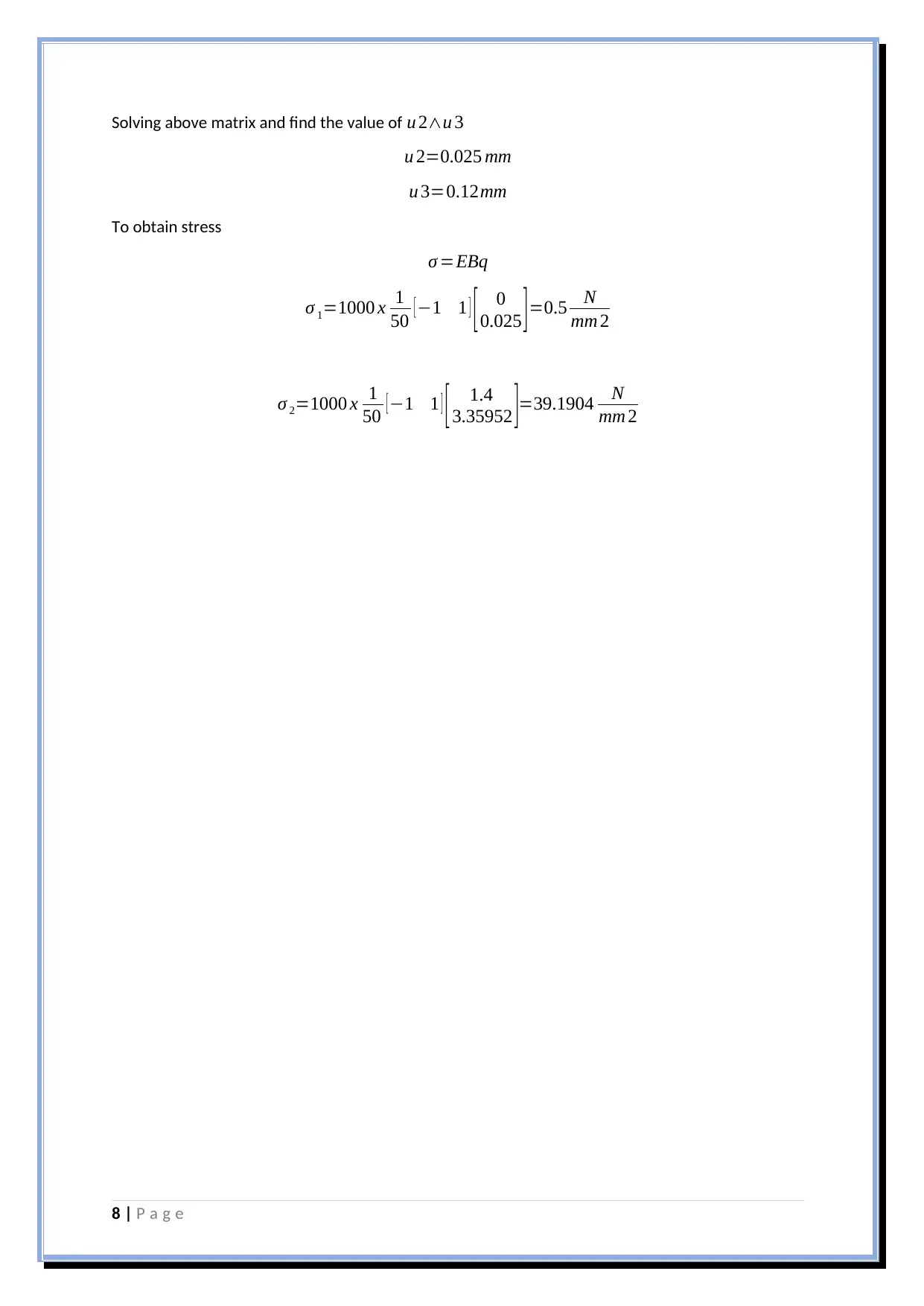
The assignment content discusses torsional shear stresses produced by a twisted moment T, which act horizontally at point A and vertically downwards at point B. The bending moment M produces tensile stress at point A. No stresses are produced at point B as it is on the neutral axis. The content also discusses plane stress in elements, principal stresses, and strain definition for a die press polymeric material. Additionally, it provides a finite element model to analyze the displacement and stress of an area under certain boundary conditions.

Answer (2)
The twisted moment T produces a torsional shear stresses
τ = Tr
I polar
¿ 2 T
π r3
¿ 2 x 2 π
π ( 0.1 )3
τ =4000 kN /m2
The stresses τ 1 acts horizontally to the left at point A and vertically downwards at the point B. the
bending moment M produce a tensile stress at point A.
σ bending= Mr
I
¿ 4 M
π r3
¿ 4 x 4 π
π ( 0.1 )3
σ bending=16000 kN /m2
There are no stress produces at point B, because B is located on the neutral axis. The shear stress
at the top of the bar at point A, the shear stress at the point B is as following:
τ shear= VQ
Ib
¿ 4 V
3 A
¿ 4 x 10 π
3 π ( 0.1 )3
τ shear=13333.33 kN /m2
σ A and τ 1 are in point A, while the τ 1 and τ 2 are acting in point B.
Note that the elements is in the plane stress with
At point A: σ x=σ A , σ y =0 ,∧τxy =−τ1
σ x= N
A
¿ 10 π
π ( 0.1 ) 3
1 | P a g e

σ x=10000 kN /m2
τ xy=−4000 kN /m2
Principal stresses:
σ 1= 1200+2500
2 ± √ ( σx−σ y
2 )
2
+τ xy
2
σ 1= 10000+0
2 + √ ( 10000−0
2 )2
+40002
¿ 5000+6403.1242
σ 1=11403.1243kN /m2
σ 2= σx +σ y
2 − √ ( σ x−σ y
2 )2
+ τxy
2
σ 2=10000+0
2 − √ ( 10000−0
2 )2
+ 40002
¿ 5000−6403.1242
¿−1403.1242kN /m2
τ max= √ ( σx−σ y
2 )
2
+ τxy
2
The stress element in point B is also plane stress and only stresses acting on this elements are shear
stress τ1 ∧τ2 .
σ x=σ y=0∧¿
τ xy=− ( τ1 +τ2 )
¿− ( 4000+13333.33 )
τ xy=−17333.33 kN /m2
Answer (B) &(C) :
σ n= σ 1+ σ 2
2 + σ 1−σ 2
2 cos 2 θ
¿ 11403.1243−1403.1242
2 + 11403.1243+1403.1242
2 cos 2 θ
¿ 50000+6403.12425
σ n=56403.12425 kN /m 2
2 | P a g e

τ s=− ( σ 1−σ 2 )
2
τ s=− ( 11403.1243+1403.1242 )
2
τ s=−6403. 12425 kN / m 2
Answer (3):
Answer (A):
As per given condition, a die press polymeric material in vertical Y-direction (k –direction),
therefore as per strain definition the dimension of polymeric material have change in y-
direction only.
StrainTensor= [εxx εxy εxz
εyx εyy εyz
εzx εzy εzz ]
¿
[ ∂ u
∂ x
1
2 ( ∂u
∂ y + ∂ v
∂ x ) 1
2 ( ∂ u
∂ z + ∂ w
∂ x )
1
2 ( ∂ u
∂ y + ∂ v
∂ x ) ∂ v
∂ y
1
2 ( ∂ v
∂ z + ∂ w
∂ y )
1
2 ( ∂u
∂ z + ∂ w
∂ x ) 1
2 ( ∂ v
∂ z + ∂ w
∂ y ) ∂ w
∂ z
]Assume 1mm pressed after applied vertical force,
So the strain in vertical direction = change∈lenght
originallenght = 1
1000 =0.001
StrainTensor L= [ 1 0.0005 0
0.0005 0.001 0.0005
0 0.0005 1 ]
3 | P a g e

Answer (B)
f ( k )=l
f ( x 2 ) =x 1
The above equation mention that when die compresses test specimen the expansion of
specimen in two perpendicular direction. As per basic fundamental of strain change length
from original length. But for this case the specimen length change in two direction. As per
given formula the change in length in l direction have change also in k direction.
[ ∂ u
∂ x 1
1
2 ( ∂u
∂ x 2 + ∂ v
∂ x 1 ) 1
2 ( ∂ u
∂ x 3 + ∂ w
∂ x 1 )
1
2 ( ∂ u
∂ x 2 + ∂ v
∂ x 1 ) ∂ v
∂ x 2
1
2 ( ∂ v
∂ x 3 + ∂ w
∂ x 2 )
1
2 ( ∂ u
∂ x 3 + ∂ w
∂ x 1 ) 1
2 ( ∂ v
∂ x 3 + ∂ w
∂ x 2 ) ∂ w
∂ x 3
]let us consider u=l , v=k∧w=n
¿
[ ∂ l
∂ x 1
1
2 ( ∂l
∂ x 2 + ∂ k
∂ x 1 ) 1
2 ( ∂ l
∂ x 3 + ∂ n
∂ x 1 )
1
2 ( ∂ l
∂ x 2 + ∂ k
∂ x 1 ) ∂ k
∂ x 2
1
2 ( ∂ k
∂ x 3 + ∂ n
∂ x 2 )
1
2 ( ∂ l
∂ x 3 + ∂ n
∂ x 1 ) 1
2 ( ∂ k
∂ x 3 + ∂n
∂ x 2 ) ∂ n
∂ x 3
]
¿
[ 1
l
1
2 ( 0+ 1
l ) 1
2 ( 0+0 )
1
2 ( 0+ 1
l ) 1
k
1
2 ( 0+0 )
1
2 ( 0+ 0 ) 1
2 ( 0+0 ) 0 ]
4 | P a g e

¿
[ 1
l
1
2l 0
1
2l
1
k 0
0 0 0 ]
For compressive stress σ =Eε
σ com=E
[ 1
l
1
2l 0
1
2l
1
k 0
0 0 0 ] N /mm2
Answer (C)
As given m = 9500mm so the change in length = 500mm so that following strain tensor
obtained
ε = 500
1000 =0.5
Following matrix obtained
ε ij= [ 0.5 0.25 0.25
0.25 1 0
0.25 0 1 ]
σ =ε ij E
Converting into meter and obtained following equation
¿ 1000 x [ 0.5 0.25 0.25
0.25 1 0
0.25 0 1 ]
σ ij= [500 250 250
250 1000 0
0 25 1000 ] N /mm 2
F=σ 3 x Area
5 | P a g e

Fx 1 x 1=26 x ( 10 x 10 )
Fx 3=2600 MPa
Answer (4)
Using two element each of 50 mm long in length, the finite element model in figure below. Nodes
and elements are numbered as shown. Noted that the area at the mid-point of the plate is as
discussed following:
A ( x )= A ( 0 ) (1− x
2 L )
A 1 ( 0 ) =10 mm2
L=100 mm
Let us take area X = L,
A ( L )=10 (1− 1
2 )
A 2 ( L )=5 mm 2
So, the area at mid span A 3= A 1+ A 2
2 =10+5
2 = 7.5mm2
Areaof top most ¿ position= 10+7.5
2 =8.75 mm 2
Area of bottom most position= 7.5+5
2 =6.25 mm 2
A ( x )= A ( 0 ) (1− x
2 L )
A ( 150 )=10 (1− 150
2 x 100 )
A ( 150 )=2.5 mm 2
Boundary condition F 1=0 , F 3=1 N
Element stiffness matrix Ke= EA
L [ 1 −1
−1 1 ]
K1= 1000 x 8.75
50 [ 1 −1
−1 1 ]
6 | P a g e

K2= 1000 x 6.25
50 [ 1 −1
−1 1 ]
K3= 1000 x 2.5
50 [ 1 −1
−1 1 ]
The global stiffness matrix K assembled
K= 1000
50 [ 8.75 −8.75 0
−8.75 15 −6.25
0 −6.25 8.75
0
0
−2.5
0 0 ¿−2.5 2.5 ]
The element body force vector are
Fe= Alρ
2 [ 1
1 ]
F1= 8.75 x 50 x 1000
2 x 106 [ 1
1 ]
F1=
[ 0.218750
0.218750 ]
F2=6.25 x 50 x 1000
2 x 106 [ 1
1 ]
F2=
[ 0.156250
0.156250 ]
F3= 2.5 x 50 x 1000
2 x 106 [ 1
1 ]
F3=
[ 0.0625
0.0625 ]
Now using formula in order to obtained displacement F=kx
1000
50 [ 8.75 −8.75 0
−8.75 15 −6.25
0 −6.25 8.75
0
0
−2.5
0 0 ¿−2.5 2.5 ] [ u1
u2
u 3
u 4 ] =
[ 0.218750
0.375
0.21875
0.0625 ]
Displacement at u 1=0 , u 4=50 mm
7 | P a g e

Solving above matrix and find the value of u 2∧u 3
u 2=0.025 mm
u 3=0.12mm
To obtain stress
σ =EBq
σ 1=1000 x 1
50 [ −1 1 ] [ 0
0.025 ] =0.5 N
mm 2
σ 2=1000 x 1
50 [−1 1 ] [ 1.4
3.35952 ]=39.1904 N
mm 2
8 | P a g e