Application of Mechanical Control for Quad Bike: ENEM20001

This project focuses on the application of mechanical control for quad bike in ENEM20001 course. It covers problem investigation, scope, assumptions, limitations, mathematical model, Simulink design, controller, and more. The aim is to design a drive-line control for the quad bike to reduce misfortunes and produce consistent stunts while having a mechanized control of the wheel stand disarray.

PROJECT TWO:
APPLICATION OF MECHANICAL CONTROL
ENEM20001
INSTITUTIONAL AFFILIATION
LOCATION (STATE, COUNTRY)
STUDENT NAME
STUDENT ID NUMBER
PROFESSOR (TUTOR)
DATE OF SUBMISSION
1

2

3

TABLE OF CONTENTS
4

APPLICATION OF MECHANICAL CONTROL
PART A: PROBLEM INVESTIGATION, SCOPE, ASSUMPTIONS AND LIMITATIONS
Problem Statement & Scope
The aim of the project is to progress or design a drive-line control for the quad bike. The
controller seeks to reduce misfortunes and produce consistent stunts while it is anticipated to
have a mechanized control of the wheel stand disarray. The controller tests the operation of
the second degree of freedom of the quad bike using a rotational and translational model of
the Simulink designed in the prior analysis.
Limitations
The project focuses on the second degree of freedom only for the analysis of the quad bikes
drive train performance and engine operation in terms of maximum torque, power, and gear
ratios.
Assumptions
a) This is the all-terrain version of Quad bike that operates on the basis of vehicle
propagation for translational and rotary motion. The gravitational acceleration is given
as 9.81 ms-2.
b) The quad bike has a single rider at every analysis. The chassis and the wheels
constitute the entire weight of the quad bike.
c) Road surface is uniform throughout the analysis.
PART B: HORIZONTAL SPEED AND POSITION MODEL
B1 Mathematical model schematic of the quad bike with traction provided by the
engine
5

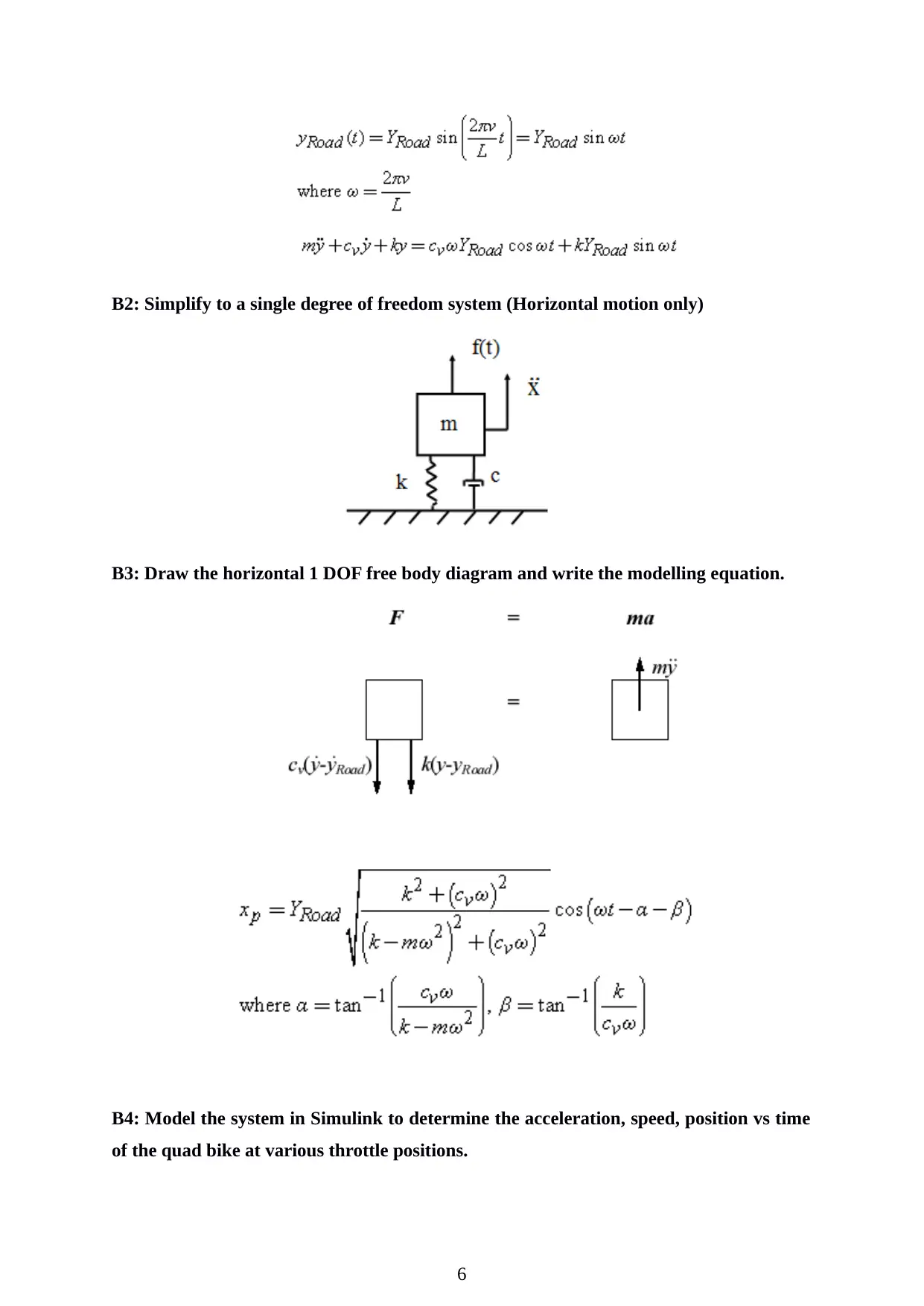
B2: Simplify to a single degree of freedom system (Horizontal motion only)
B3: Draw the horizontal 1 DOF free body diagram and write the modelling equation.
B4: Model the system in Simulink to determine the acceleration, speed, position vs time
of the quad bike at various throttle positions.
6

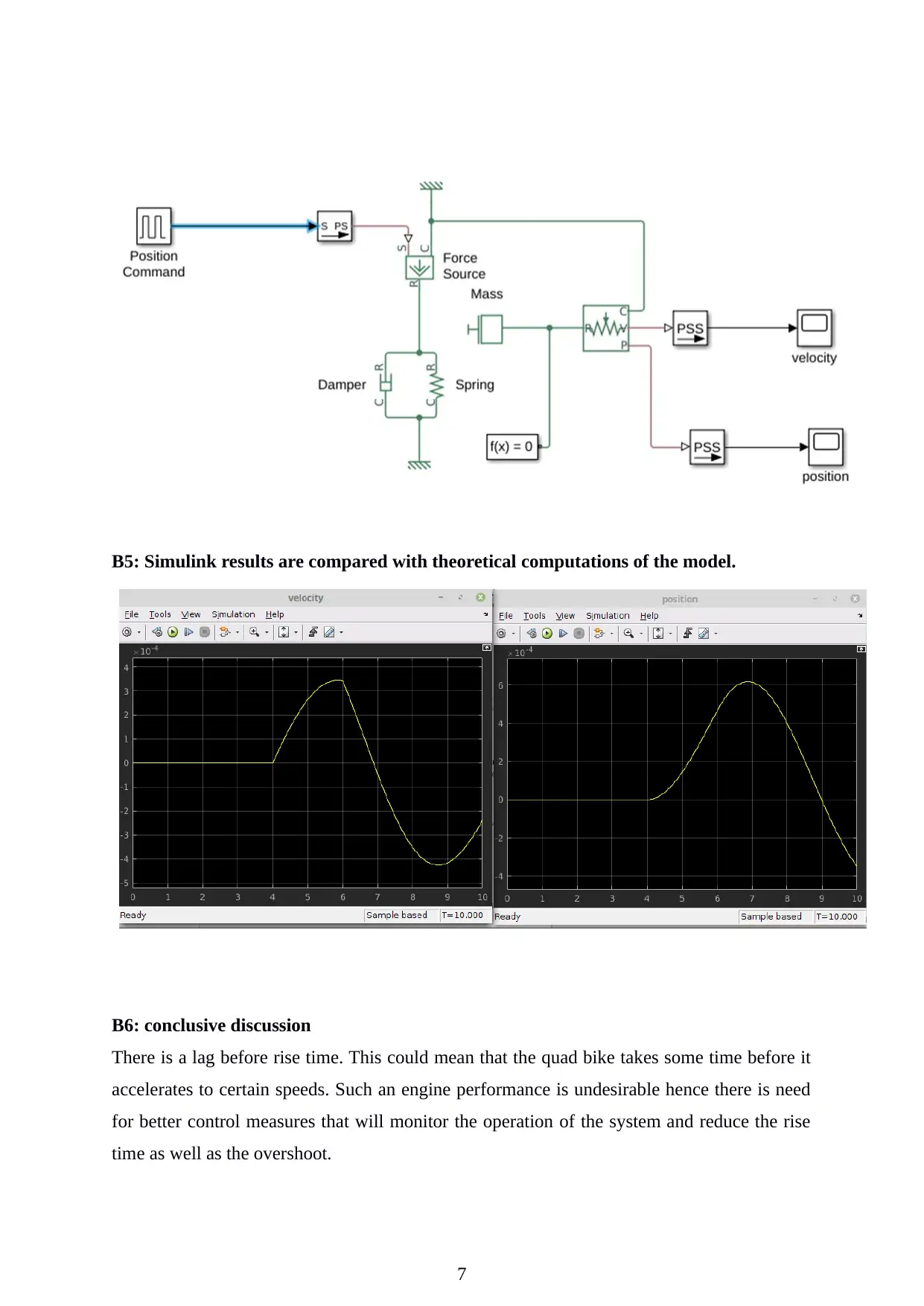
B5: Simulink results are compared with theoretical computations of the model.
B6: conclusive discussion
There is a lag before rise time. This could mean that the quad bike takes some time before it
accelerates to certain speeds. Such an engine performance is undesirable hence there is need
for better control measures that will monitor the operation of the system and reduce the rise
time as well as the overshoot.
7

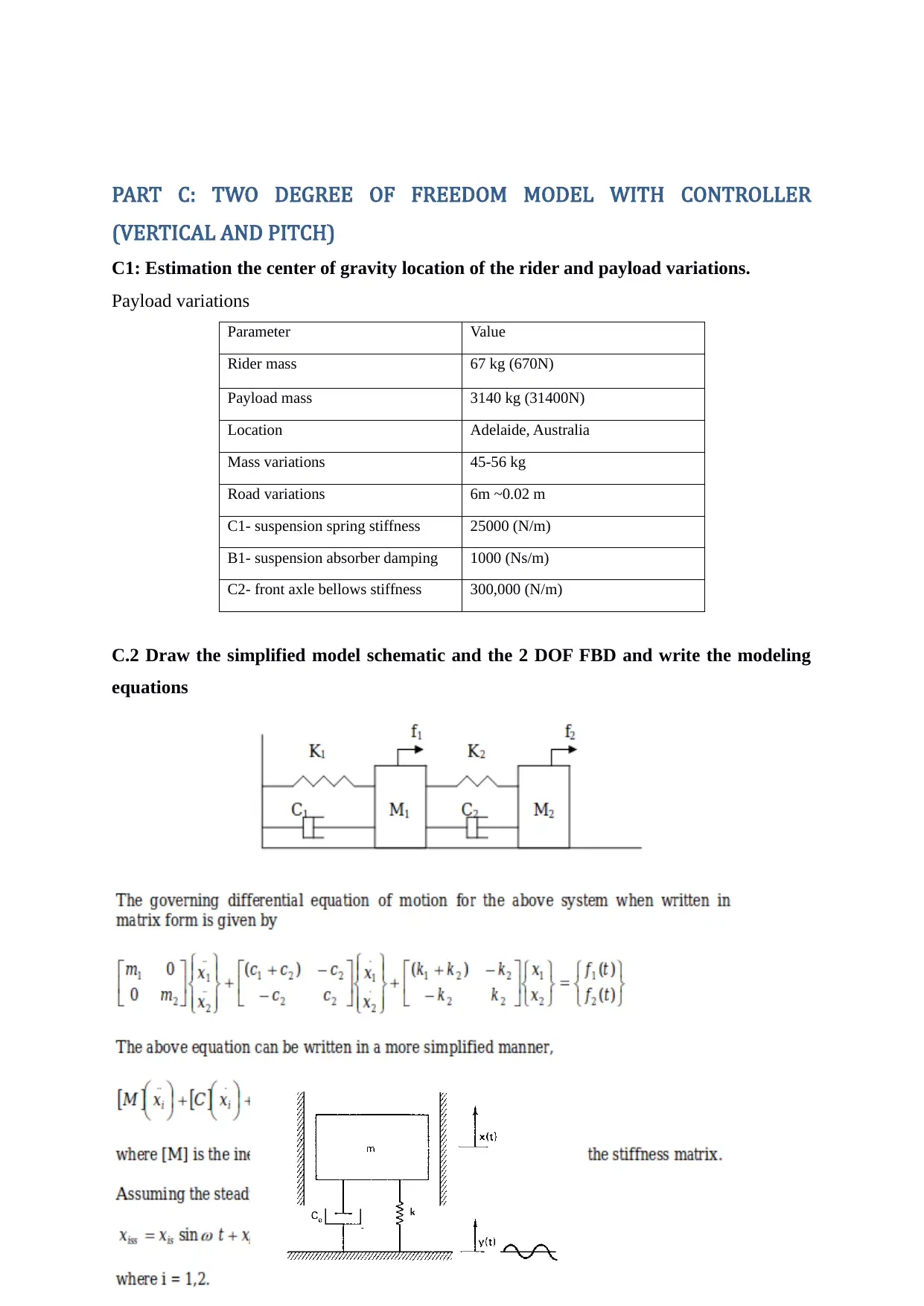
PART C: TWO DEGREE OF FREEDOM MODEL WITH CONTROLLER
(VERTICAL AND PITCH)
C1: Estimation the center of gravity location of the rider and payload variations.
Payload variations
Parameter Value
Rider mass 67 kg (670N)
Payload mass 3140 kg (31400N)
Location Adelaide, Australia
Mass variations 45-56 kg
Road variations 6m ~0.02 m
C1- suspension spring stiffness 25000 (N/m)
B1- suspension absorber damping 1000 (Ns/m)
C2- front axle bellows stiffness 300,000 (N/m)
C.2 Draw the simplified model schematic and the 2 DOF FBD and write the modeling
equations
8

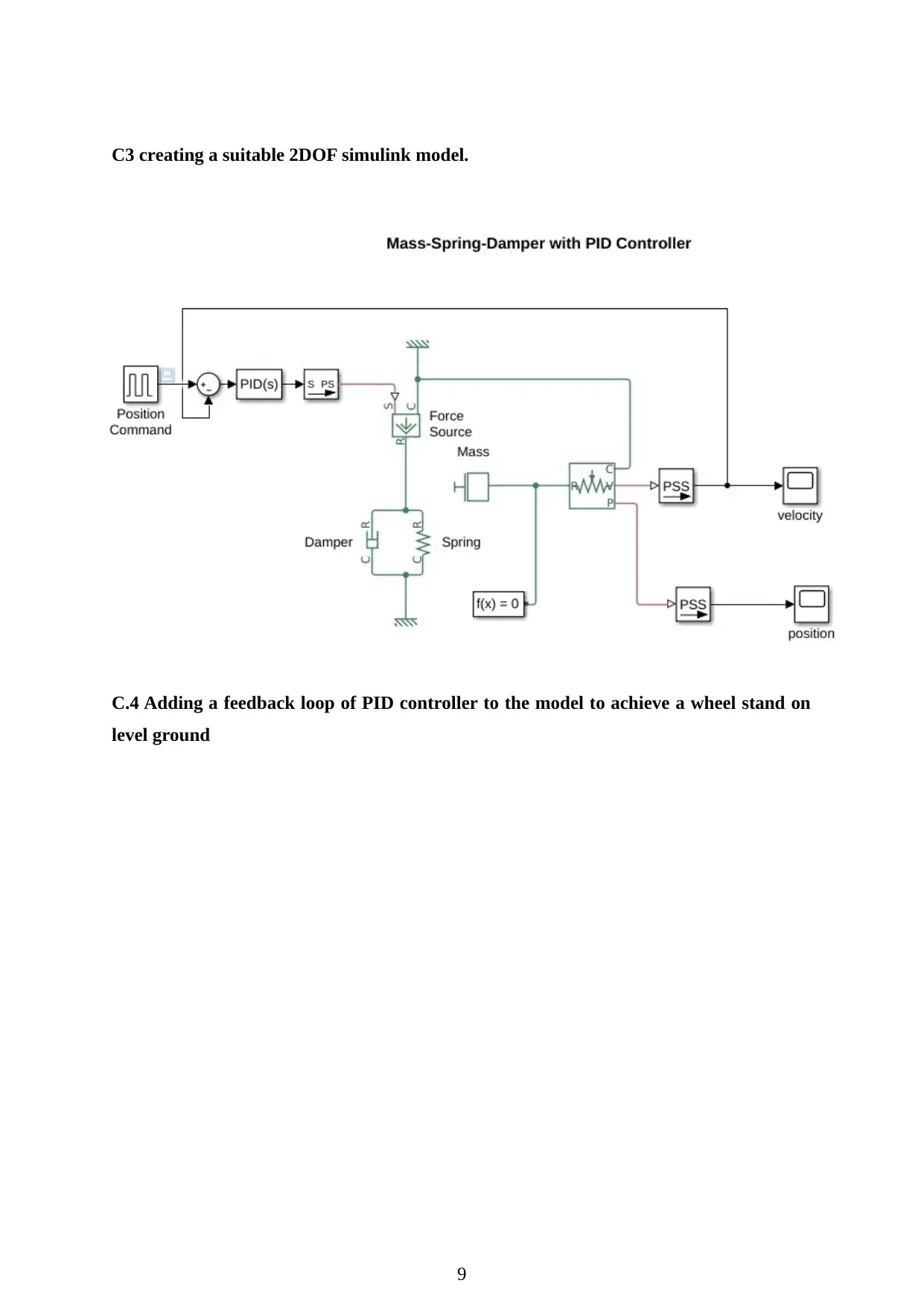
C3 creating a suitable 2DOF simulink model.
C.4 Adding a feedback loop of PID controller to the model to achieve a wheel stand on
level ground
9

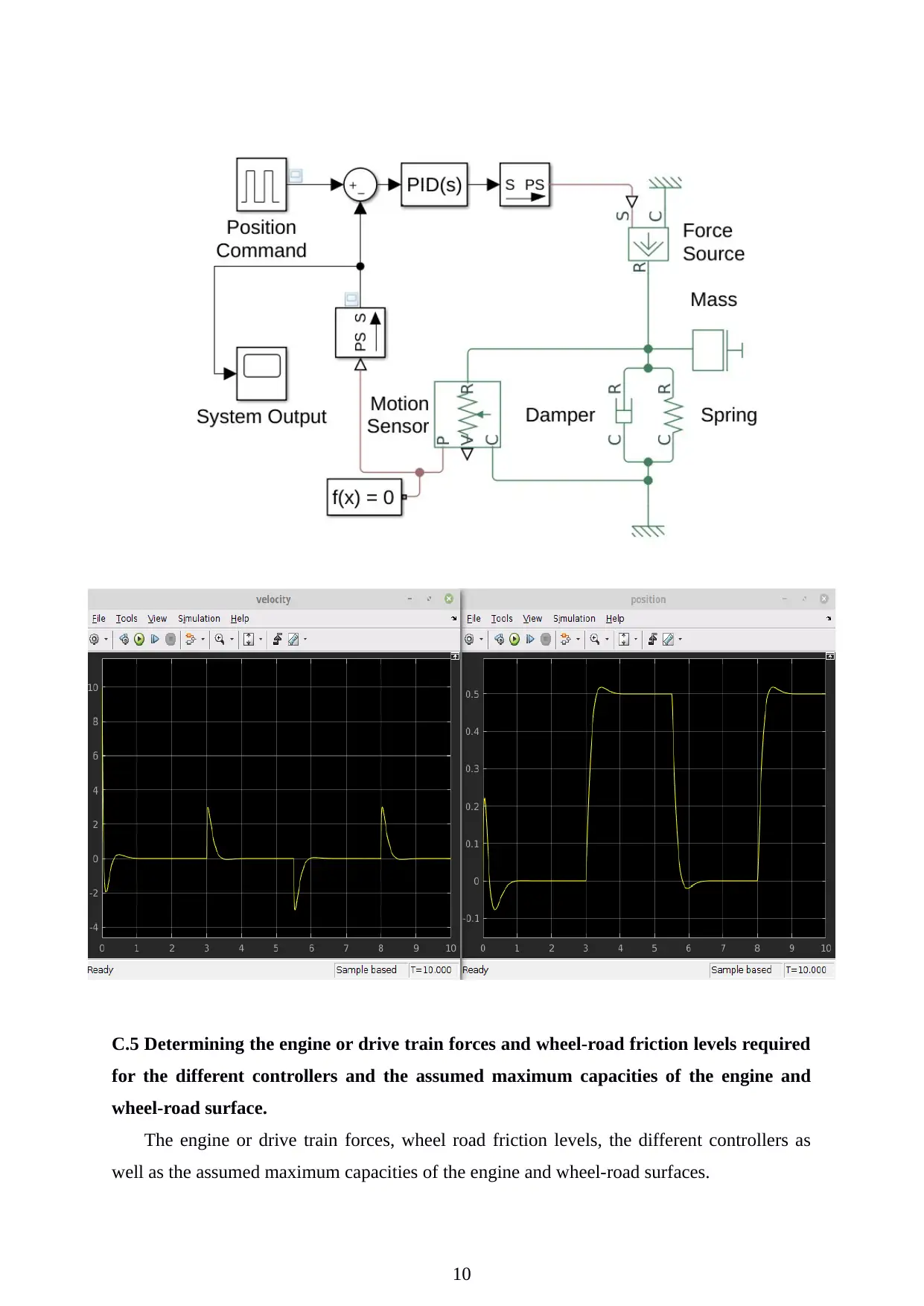
C.5 Determining the engine or drive train forces and wheel-road friction levels required
for the different controllers and the assumed maximum capacities of the engine and
wheel-road surface.
The engine or drive train forces, wheel road friction levels, the different controllers as
well as the assumed maximum capacities of the engine and wheel-road surfaces.
10

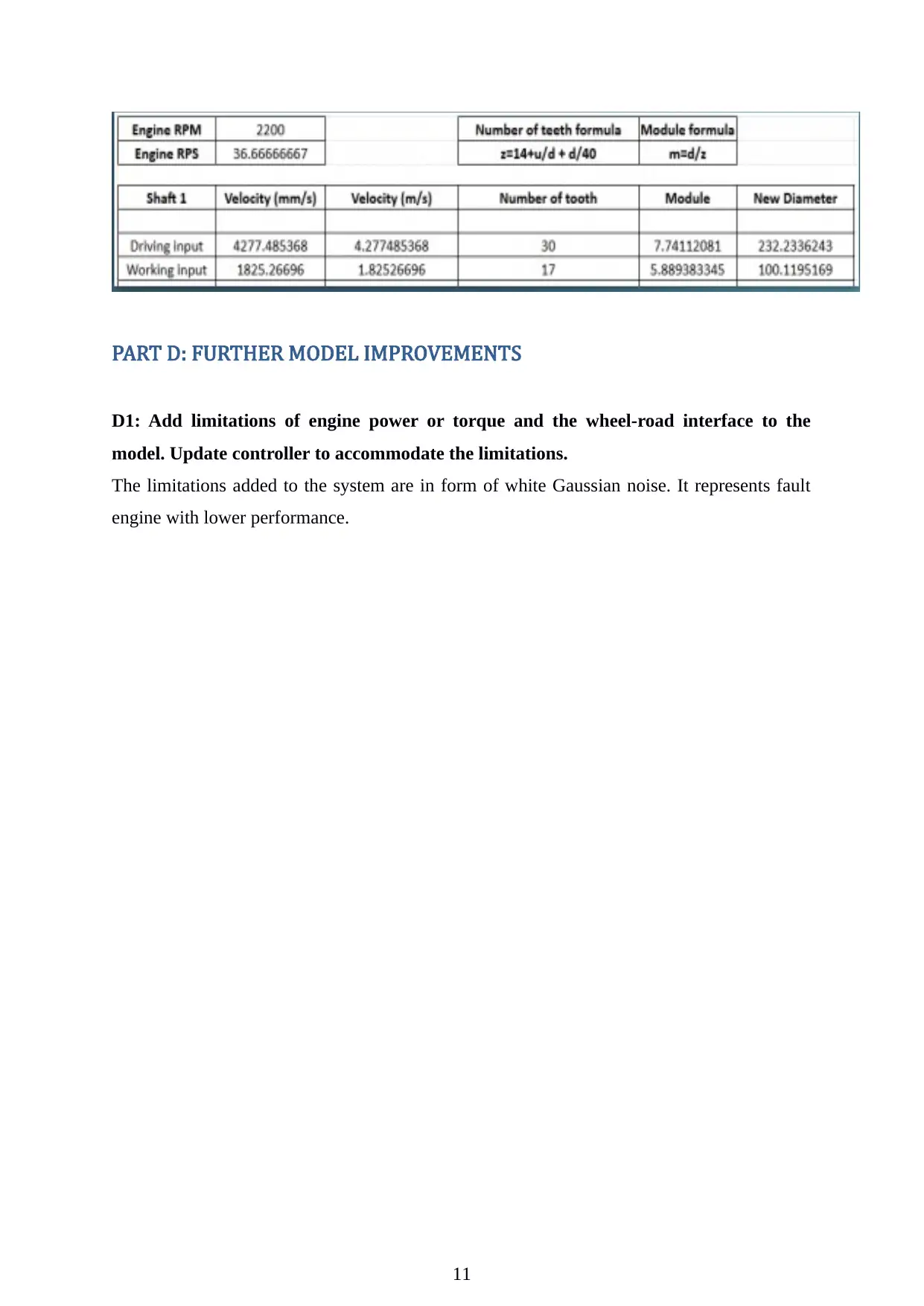
PART D: FURTHER MODEL IMPROVEMENTS
D1: Add limitations of engine power or torque and the wheel-road interface to the
model. Update controller to accommodate the limitations.
The limitations added to the system are in form of white Gaussian noise. It represents fault
engine with lower performance.
11

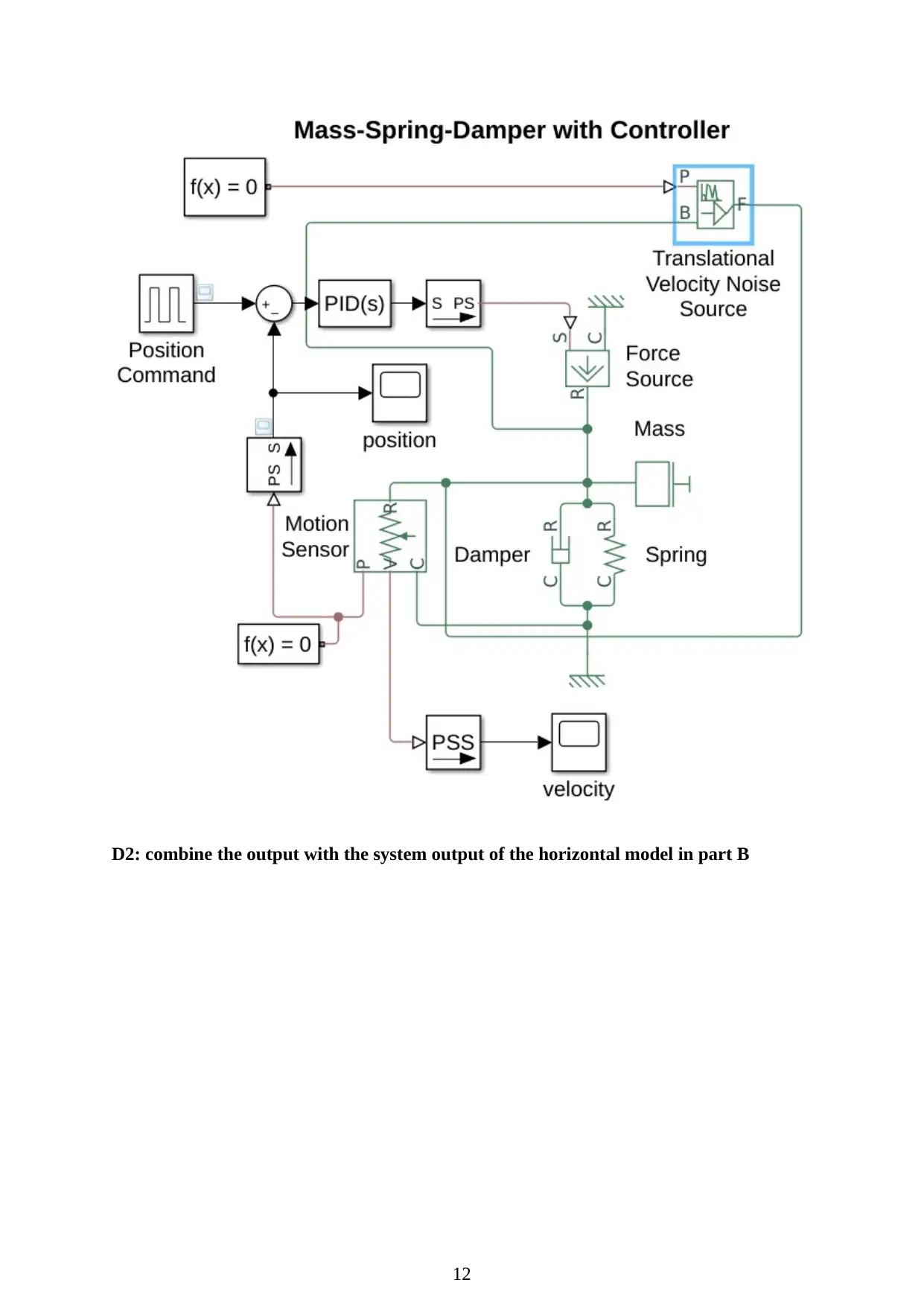
D2: combine the output with the system output of the horizontal model in part B
12

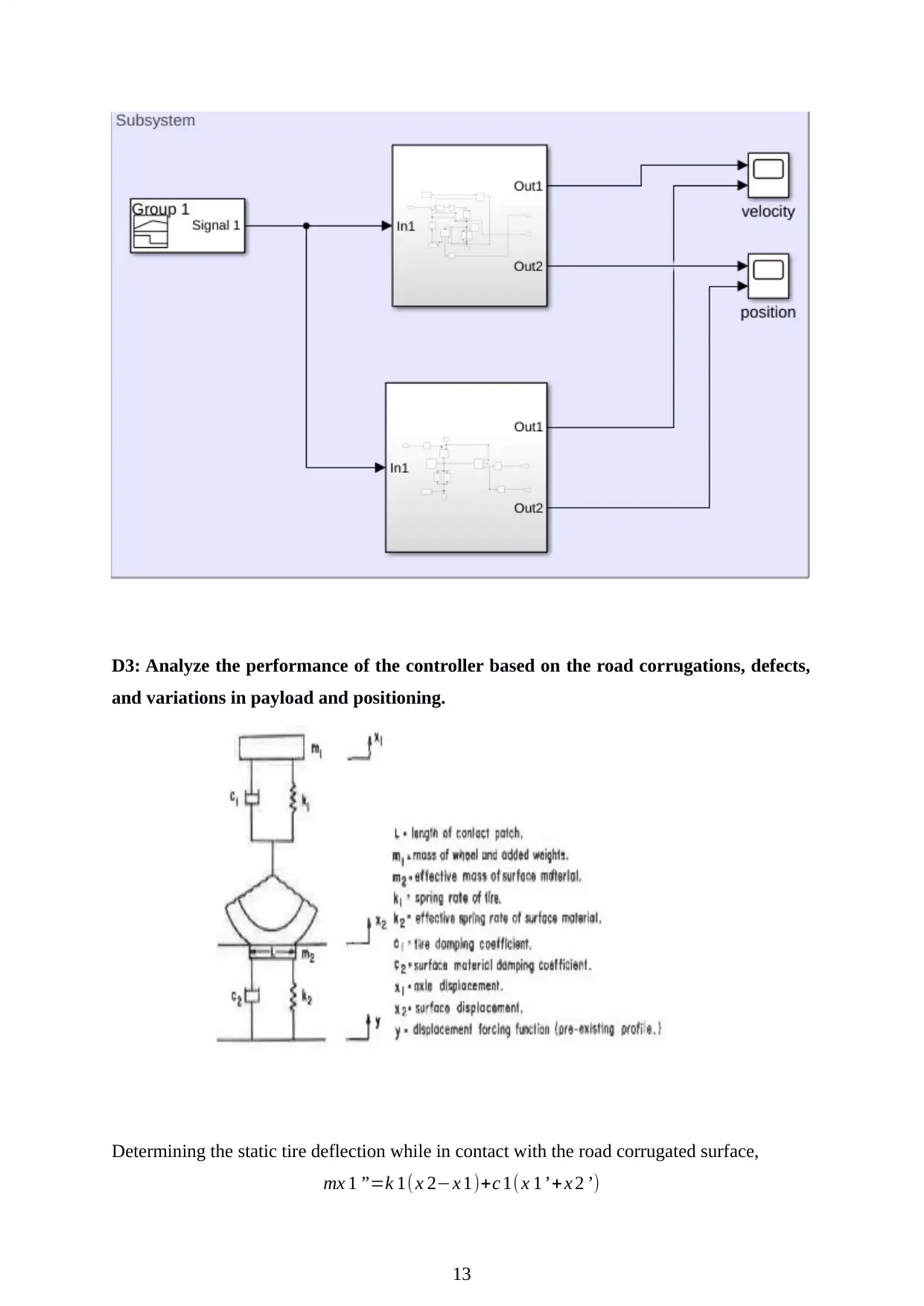
D3: Analyze the performance of the controller based on the road corrugations, defects,
and variations in payload and positioning.
Determining the static tire deflection while in contact with the road corrugated surface,
mx 1 ”=k 1( x 2−x 1)+c 1( x 1’ +x 2 ’)
13

m 2 x 2”=k 1( x 1−x 2)+c 1( x 1 ’−x 2 ’)+k 2( y −x 2)+c 2( y ’−x 2’ )
for (x 1−x 2)≤static tire deflection
Neglecting the air resistance, the wheels encounter the surface and develop a trajectory whose
path is defined as,
X 1 ’=X 1 p ’−¿
for all the cases where(x 1−x 2)> static tire deflection
When analyzing the system on a horizontal plane, the vertical reactive forces that act on the
tire keep changing which affects the rolling radius. It is also important to consider the
slippage which is defined as,
i=1− ( R
Rst )
which defines effective rolling radius and the static rolling radius.
The Bekker equation shows that the relationship between the horizontal and the vertical loads
are based on the corresponding deformations and could be expressed by,
14

D4: re-assess the quad bike spring and damper values based on the chosen values in
prospect 1.
The values chosen for the Quad bike Spring and damper in the first analysis were
spring coefficient=25 0,000 N /m
Damping coefficient =25,500 Ns /m
For the case with a PID controller, the system stabilizes at a short duration. The system is
able to develop consistency and access to the system parameters and it deflects the noises in
its environment.
spring coefficient=25,545 N /m
Damping coefficient =2950 Ns /m
15

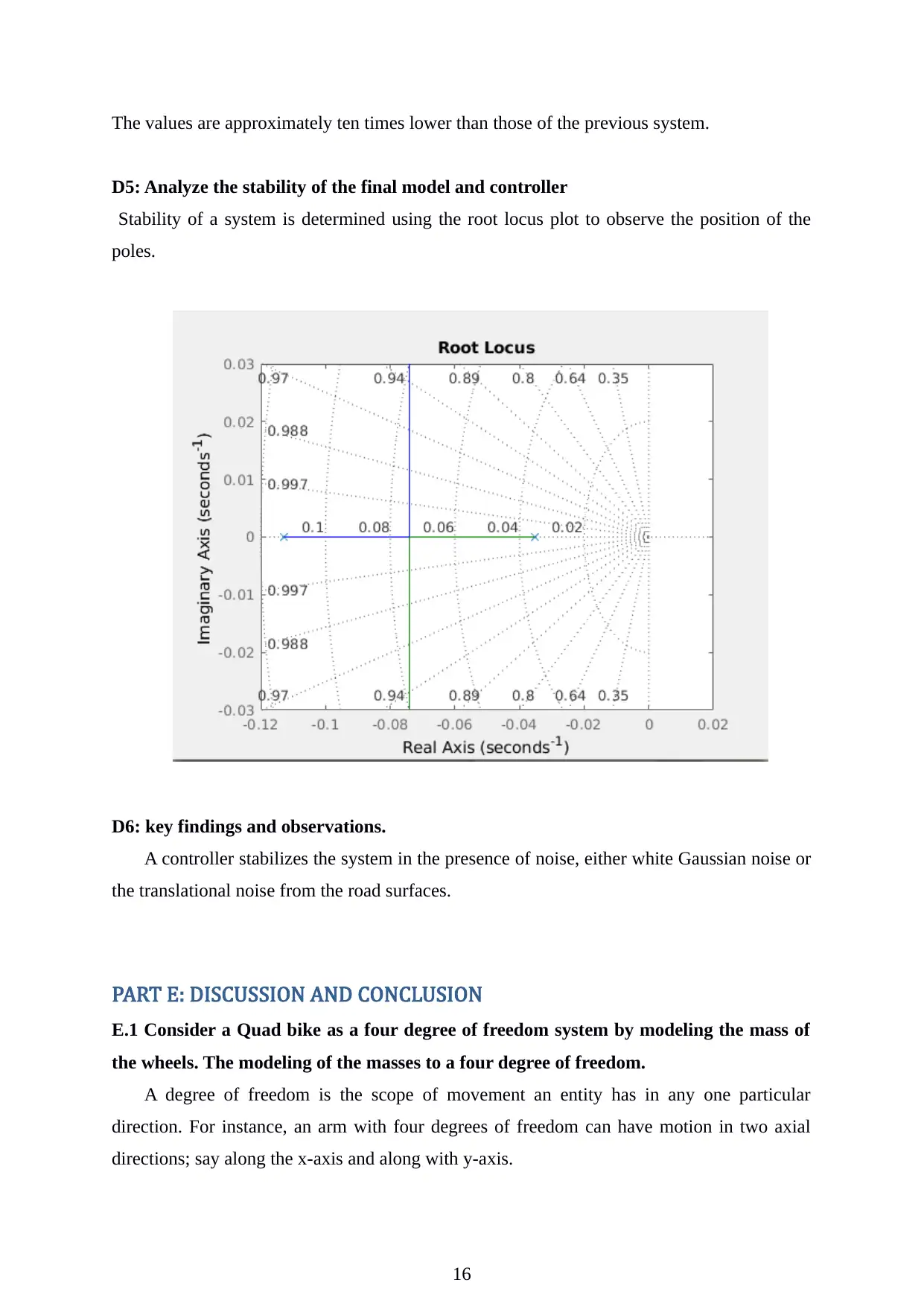
The values are approximately ten times lower than those of the previous system.
D5: Analyze the stability of the final model and controller
Stability of a system is determined using the root locus plot to observe the position of the
poles.
D6: key findings and observations.
A controller stabilizes the system in the presence of noise, either white Gaussian noise or
the translational noise from the road surfaces.
PART E: DISCUSSION AND CONCLUSION
E.1 Consider a Quad bike as a four degree of freedom system by modeling the mass of
the wheels. The modeling of the masses to a four degree of freedom.
A degree of freedom is the scope of movement an entity has in any one particular
direction. For instance, an arm with four degrees of freedom can have motion in two axial
directions; say along the x-axis and along with y-axis.
16

E2 Benefits in relation to both dynamic analysis and accuracy of the controller
Controller is able to ensure that only the anticipated yield is obtained at the output
despite the system errors that may arise.
E3 Final design for the quad bike suspension stiffness, damper values and
recommendations for a controller.
Design for quad bike suspension stiffness, damper values, and recommendations
spring coefficient = 30,000 N/m
Damping coefficient = 2950 Ns/m
The controller gain parameters for the PID controller were adjusted throughout the testing
phase as shown in the table below,
Parameter Test 1 Test 2 Test 3 Test 4 Test 5
Kp 650 750 1000 1250 850
Kd 15 35 10 20 25
17

Ki 4500 4800 4000 3500 5000
Recommendations for the controller.
The controller should be embedded on a chip and installed in the electrical connection
point in the quad bike. At that point, it can control the system and ensure that that quad bike
deals with rough terrain as well as abnormalities during the drive train. In modern era, the use
of artificial intelligence fuzzy technique is quite prevalent. The fuzzy systems monitor a
systems operations and determines certain patterns in the system. Such patterns can provide
additional information to the controller to improve its performance.
E4. Discuss about the controller.
The proportional controller is used in the first order systems. It manipulates a
proportional gain constant which is used to alter the value of the output. The gain parameter
is multiplied with the system first order system to give a new yield to the system. The gain
parameter minimizes the steady state error but it does not eliminate the errors. The gain
parameter is denoted as, Kp. Adjusting the value increasingly minimizes the steady state
error. The value may be increased to a given value which is consider the optimum value
which results in a reduced amplitude and phase margin. Exceeding the value may cause the
output to oscillate during dead time or lag. The proportional-integral controller, on the other
hand, eliminates the steady state error completely. The control parameters are denoted as Kp
and Ki.
The controller is implemented in areas where speed is not a performance factor.
Unfortunately, the controller is not able to predict future errors in the system and it can
neither reduce the rise time nor eliminate the oscillations that may result when the value of
the proportional gain parameter is very high. The PID controller solves the issues that other
controllers are unable to solve. It guarantees optimum control dynamics to obtain zero steady
state error, faster response which implies a shorter rise time, no oscillations, no overshoots,
and higher stability. The controller can be implemented in systems of higher orders unlike the
proportional controller which is limited to first order systems only.
E5 Steps to implement the controller on a real quad bike
18

a) Determine the electrical connection section of the system.
b) Study the integration of the electrical part of the Quad Bike with the wheel system at
the axle and the engine. At the point of connection, embed the controller.
c) The best method to embed the controller is done using PLC, PIC or an Arduino-
programmed chip which is programmed and inserted on the device.
d) Tests are carried out to ensure that the system functions as expected. The feedback
loop collect information from the environmental noise and incorrect yields, processes
the error and makes adjustments to ensure that the system performs as it is intended
to.
E6 summarize the key findings and observations
Operating a system as an open loop exposes it to a lot of external and internal
disturbances which in turn affect the system’s performance. The system’s performance is
given on the basis where the system output reflects the intended system output.
19

REFERENCES
A.G. Keskar. "Robotic Arm with Four Degrees of Freedom" , 2008 First International
Conference on Emerging Trends in Engineering and Technology, 07/2008.
Kerchmann, V. & Hohman, R. E. T., 2009. Dynamic tire friction models for Quad Bikes
Traction control. Decision and Control: Proceedings of the 38th IEEE conference, 13(4), pp.
3746-3751.
Seoung-On, K. et al., 2012. Actively translating a rear diffuser device for the aerodynamic
drag reduction of a passenger car. International l Journal of Automotive Technology, 13(4),
pp. 583-5
20