Applied Biostatistics Assignment

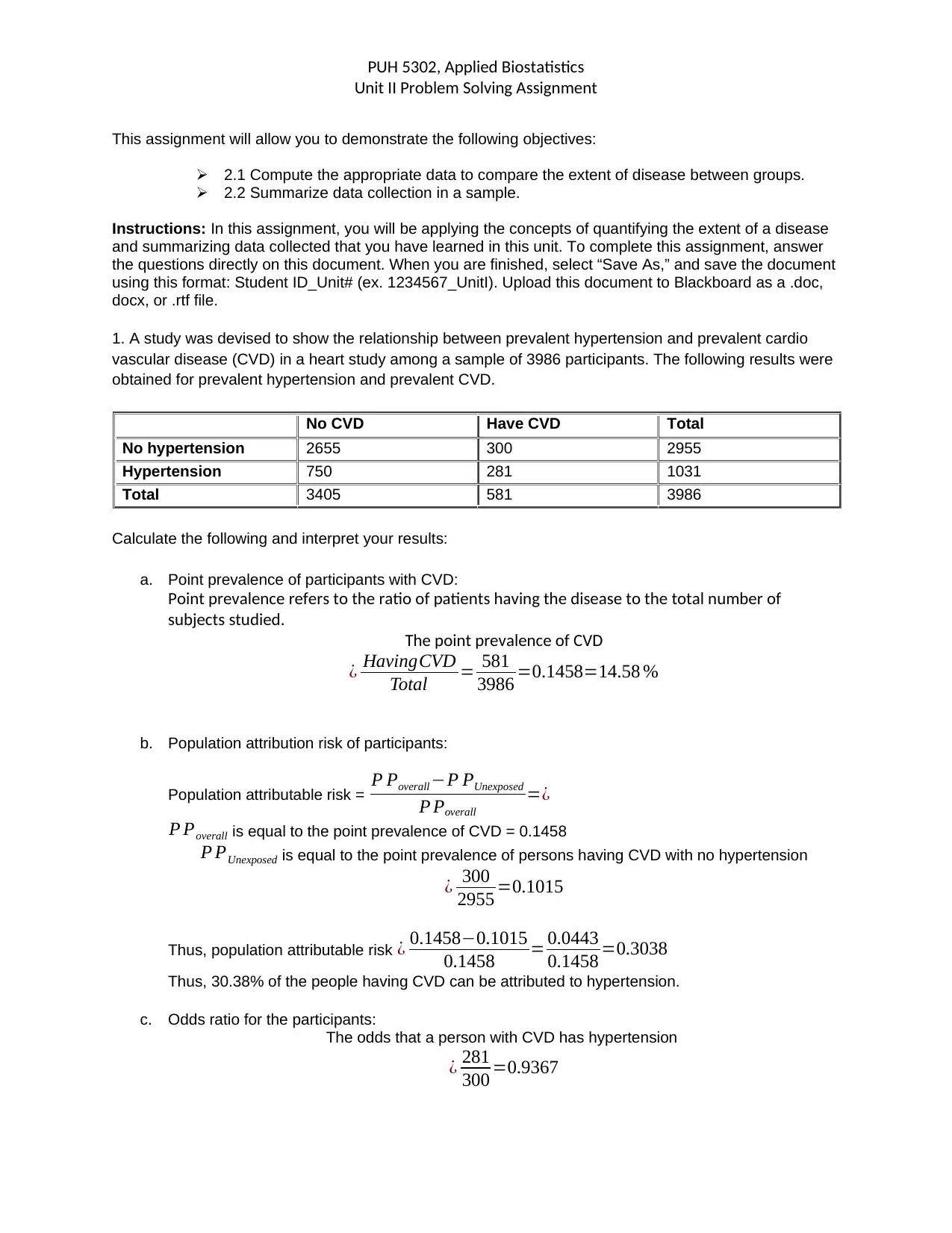
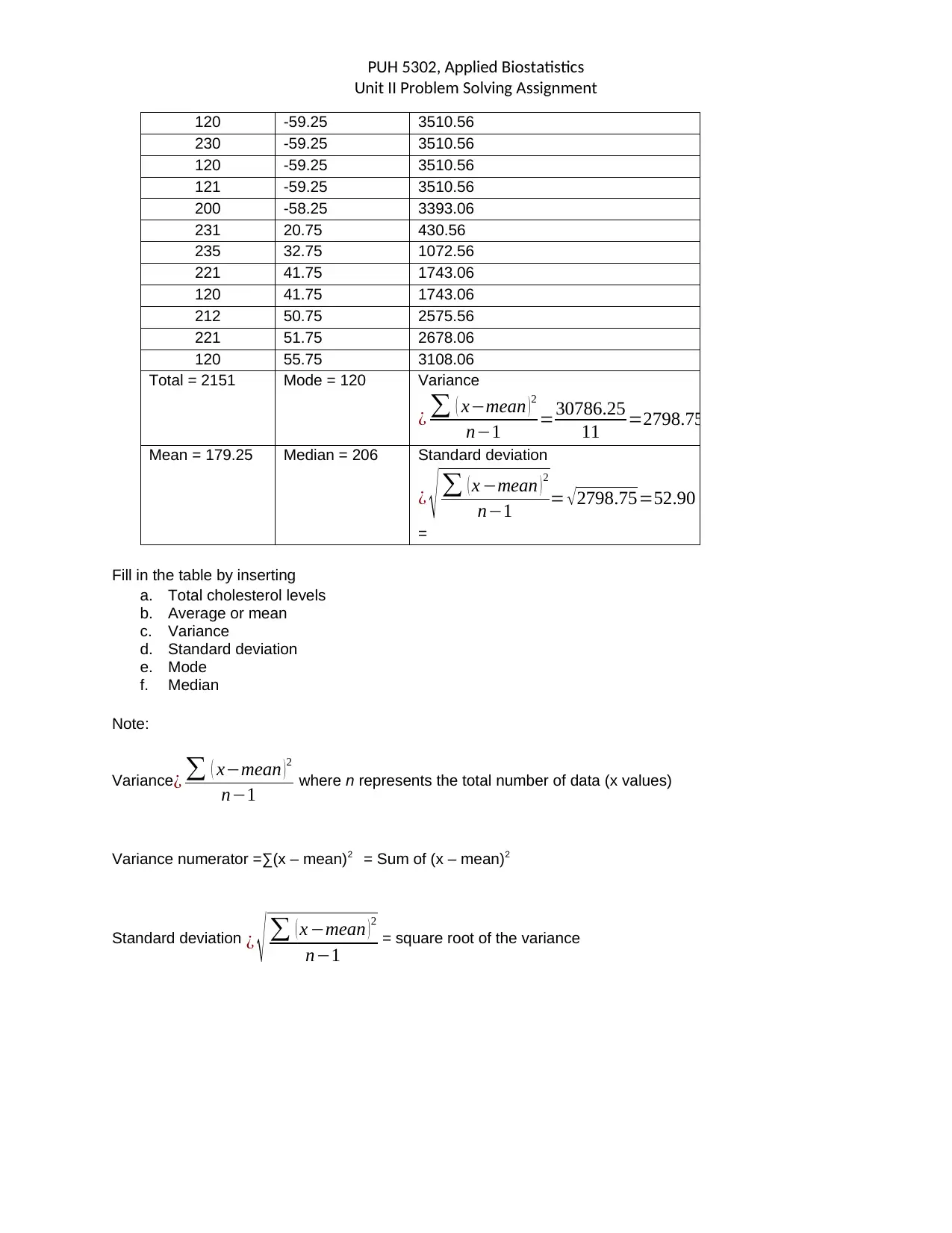
This assignment focuses on applying the concepts of quantifying the extent of a disease and summarizing data collected in the field of biostatistics. It includes calculations and interpretations of point prevalence, population attribution risk, odds ratio, risk difference, and relative risk.